Unveiling The Importance Of Volume In Geometry And Its Practical Applications

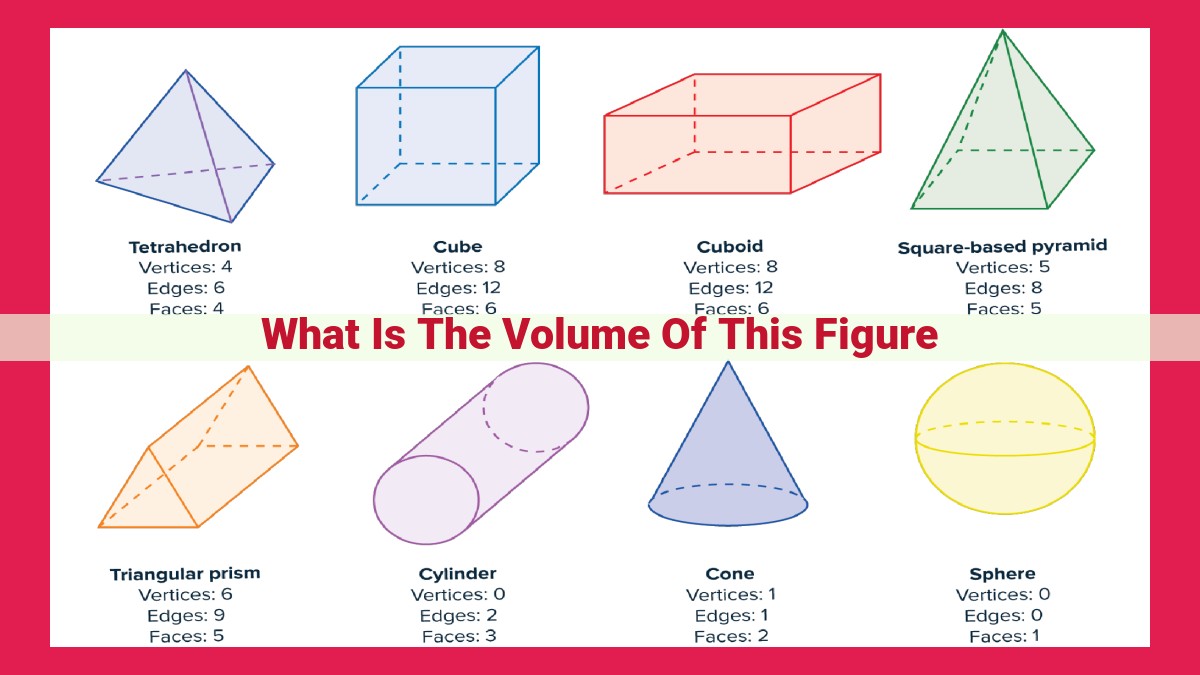
Volume, a key concept in geometry, signifies the amount of three-dimensional space occupied by an object. Understanding volume plays a pivotal role in numerous real-world applications, from scientific calculations to engineering designs. It’s defined as the product of length, width, and height and is measured in cubic units such as cubic meters or liters. By accurately calculating volume, we gain valuable insights into the size, density, and behavior of 3D objects, facilitating informed decisions across diverse fields, including architecture, construction, medicine, and more.

The Significance of Volume: Understanding the Dimensions of Our World

In the intricate tapestry of our three-dimensional reality, understanding volume is paramount. Far beyond a mere mathematical concept, volume plays a crucial role in our everyday lives, shaping our perception of the world and enabling us to navigate its vast expanse.

From the grandiose structures that grace our skylines to the humble objects that fill our homes, volume defines the size and shape of every tangible thing around us. It allows us to estimate the capacity of containers, predict the behavior of fluids, and comprehend the density of materials. In essence, volume provides the foundation for understanding the physical world we inhabit.

In the realm of architecture and engineering, volume determines the spaciousness of buildings, bridges, and other structures. It influences factors such as ventilation, lighting, and structural integrity, ensuring our safety and comfort. In the world of commerce, volume plays a vital role in packaging, inventory management, and logistics, determining the efficiency of storage and transportation. For artists and designers, volume lends depth and dimension to their creations, allowing them to evoke emotion and create a sense of presence in their work.

The significance of volume extends far beyond its practical applications. It is an essential element of scientific inquiry, enabling us to quantify the three-dimensional properties of substances and materials. In medicine, volume measurements are vital diagnostic tools, aiding in the evaluation of organ function and tissue density. In environmental science, volume calculations are crucial for understanding ecosystems, managing water resources, and assessing the impact of human activities.

In short, volume is the fundamental concept that allows us to grasp the size and shape of our three-dimensional world. It is the key to unlocking the mysteries of the physical universe, shaping our understanding of everything from the macro to the micro level. By delving into the depths of volume, we gain a deeper appreciation for the complexity and beauty that surrounds us.

Understanding Volume: Definition and Related Concepts

Let’s embark on a journey to unravel the intriguing concept of volume, a fundamental property that defines the three-dimensional space occupied by objects. Volume plays a pivotal role in our daily lives, from comprehending the size of our homes to understanding the function of various containers.

To fully grasp volume, it’s important to distinguish it from other related terms such as capacity, space, and displacement. While these terms often overlap in everyday language, they each carry distinct meanings. Capacity refers to the amount of substance that can be contained within an object, while space simply refers to the empty area surrounding or within an object. Displacement, on the other hand, measures the volume of fluid displaced by an object when it is submerged.

Volume, in contrast to these terms, specifically measures the amount of three-dimensional space enclosed within the boundaries of an object. It provides a quantitative measure of the object’s size and is expressed in units such as cubic meters (m³), liters (L), or gallons (gal). Understanding volume is crucial for understanding the size and shape of objects, as well as for calculating their mass and density.

Measurement Units and Techniques for Volume Calculation

The Standard Units of Volume

When measuring volume, standardization is crucial. The most commonly used standard units for volume are cubic meters (m³), liters (L), milliliters (mL), and cubic centimeters (cm³). These units provide a consistent and precise way of quantifying volume, allowing for accurate comparisons and calculations across different contexts.

Calibration for Accuracy

The accuracy of volume measurements is essential for a wide range of applications, from scientific research to industrial manufacturing. To ensure accurate measurements, it is important to calibrate the equipment used to measure volume. Calibration involves comparing the readings obtained from the equipment with known standard volumes. This process helps identify any errors or deviations and allows for necessary adjustments to obtain accurate results.

Types of Volume Measurement Equipment

A variety of equipment can be used to measure volume, each with its own strengths and limitations. Some common examples include:

• Graduated cylinders: These are typically used to measure the volume of liquids, with markings indicating the volume contained at different levels.
• Pipettes: Pipettes are used to accurately dispense precise volumes of liquids, often in microliter (µL) or milliliter (mL) increments.
• Volumetric flasks: Volumetric flasks are designed to hold a specific volume of liquid, typically for preparing solutions or dilutions. They have a narrow neck with a graduation mark indicating the calibrated volume.

Understanding the appropriate equipment and calibration techniques ensures accurate volume measurements, which are essential for reliable data and successful outcomes in various fields of science, engineering, and everyday applications.

Key Dimensions: Length, Width, and Height

Understanding the concept of volume is crucial in numerous real-world applications and for comprehending three-dimensional objects. The key to unraveling volume lies in grasping the dimensions of length, width, and height. These dimensions play a pivotal role in calculating volume and are applicable to a diverse range of shapes.

Length represents the extent of an object from one end to the other. For example, the length of a rectangular prism is the distance between its two parallel faces.

Width signifies the measure of an object from side to side, perpendicular to its length. In the case of a rectangular prism, the width is the distance between its two faces that are perpendicular to its length.

Height denotes the vertical dimension of an object, extending from its base to its top. For a rectangular prism, the height is the distance between its two parallel faces that are perpendicular to its length and width.

The formula for the volume of a rectangular prism highlights the significance of length, width, and height: V = L x W x H. This formula underscores that the volume is the product of these three crucial dimensions.

By understanding the concepts of length, width, and height, you gain the ability to calculate the volume of numerous shapes. For instance, a cube has equal length, width, and height, making its volume equal to the cube of its side length: V = s³. A cylinder, on the other hand, possesses a circular base and two parallel circular faces. Its volume is calculated as V = πr²h, where r represents the radius of the base and h denotes the height of the cylinder.

Mastering the dimensions of length, width, and height empowers you to unravel the mysteries of volume, enabling you to navigate various fields that heavily rely on volume calculations. These dimensions are indispensable in architecture, engineering, construction, cooking, and countless other disciplines. By wielding this knowledge, you unlock the door to a deeper comprehension of the three-dimensional world around you.

The Formula for Volume: V = L x W x H

Understanding the concept of volume is essential in our everyday lives. From measuring the ingredients for a cake to calculating the fuel capacity of a vehicle, volume plays a significant role. The formula for calculating volume, V = L x W x H, provides the mathematical foundation for these measurements.

In this formula, V represents the volume of the object, L is its length, W is its width, and H is its height. It is important to note that the units of measurement for these dimensions must be consistent, typically using cubic meters (m³) or cubic centimeters (cm³).

The formula is based on the principle that the volume of an object is equal to the product of its three dimensions. For example, a rectangular prism with a length of 5 cm, a width of 3 cm, and a height of 2 cm would have a volume of 5 cm x 3 cm x 2 cm = 30 cm³.

This formula can be applied to a variety of shapes, including regular and irregular objects. By breaking down an irregular object into smaller, regular shapes and calculating the volume of each shape individually, we can determine the total volume of the object.

The formula for volume is an indispensable tool in various fields, such as architecture, engineering, and medicine. It is used to calculate the volume of buildings, design storage spaces, and determine the dosage of medications. Understanding and applying this formula empower us to interact with the three-dimensional world around us.

Example Calculations for Common Shapes

Unveiling the Volume of Everyday Objects

In our day-to-day lives, we often encounter objects of various shapes and sizes. Understanding their volume is crucial for tasks ranging from packaging and construction to cooking and scientific experiments. Let’s embark on a journey to calculate the volume of some common shapes and simplify the process for you.

Calculating the Volume of a Cube

A cube is a three-dimensional object with all sides equal in length. Imagine a dice or a sugar cube. To find the volume of a cube, we measure the length of one side, represented by l. Using the formula V = l x l x l, we simply cube the side length.

For example, if each side of the cube measures 5 centimeters, its volume would be V = 5 cm x 5 cm x 5 cm = 125 cubic centimeters (cm³)**.

Calculating the Volume of a Rectangular Prism

A rectangular prism has three pairs of parallel faces, with each face being a rectangle. Think of a shoebox or a brick. To calculate its volume, we measure three dimensions: length (l), width (w), and height (h). The formula for the volume of a rectangular prism is V = l x w x h.

Suppose we have a rectangular prism with a length of 10 centimeters, a width of 5 centimeters, and a height of 3 centimeters. Its volume would be V = 10 cm x 5 cm x 3 cm = 150 cubic centimeters (cm³)**.

Calculating the Volume of a Cylinder

A cylinder has two circular bases connected by a curved surface. Picture a can of soda or a garden hose. To calculate the volume of a cylinder, we measure its radius (r), which is the distance from the center of the base to its edge, and its height (h). The formula for the volume of a cylinder is V = πr²h, where π (pi) is a mathematical constant approximately equal to 3.14.

For instance, if a cylinder has a radius of 2 centimeters and a height of 5 centimeters, its volume would be V = 3.14 x 2 cm² x 5 cm = 62.8 cubic centimeters (cm³)**.

By following these simple formulas and understanding the key dimensions of common shapes, you can confidently and accurately calculate their volumes, unlocking a deeper understanding of the world around you.