Visualizing Numerical Relationships with Double Number Lines: A Comprehensive Guide

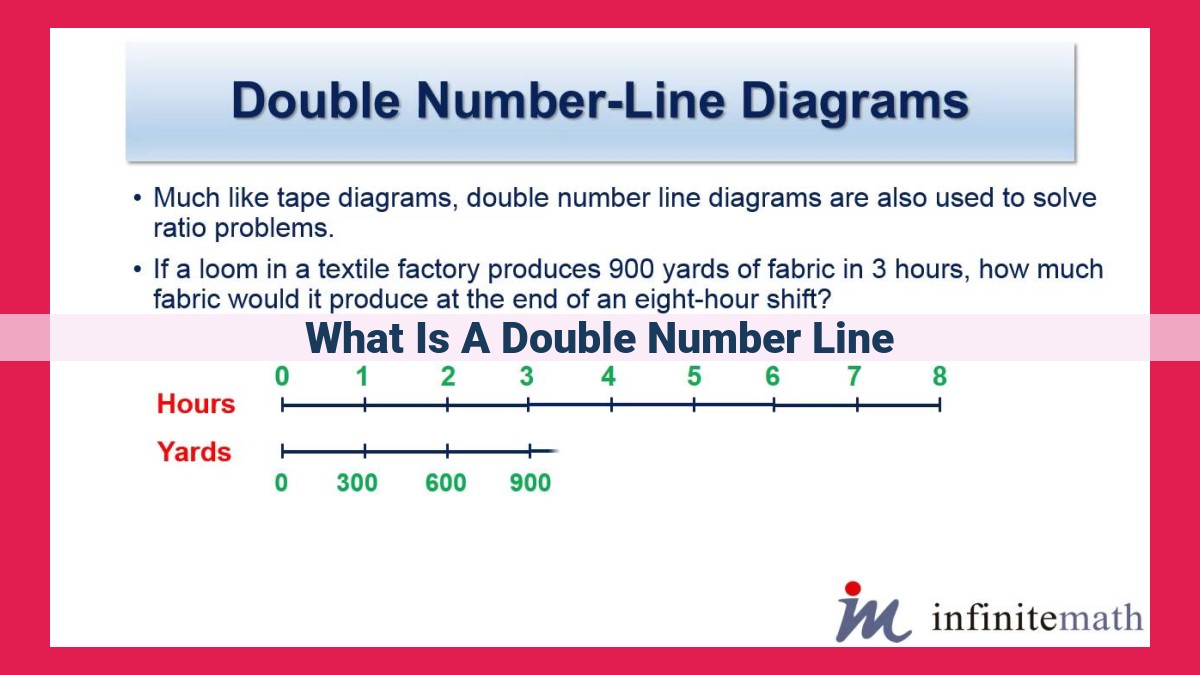
A double number line is a visual representation that utilizes two parallel number lines to depict both positive and negative values. The origin, or zero point, is located at the intersection of the two lines, acting as the reference for measuring distances. Positive numbers extend to the right, while negative numbers extend to the left. This arrangement allows for the visualization of numerical relationships, making it particularly useful in mathematical operations involving integers, fractions, and rational numbers.

Contents

Understanding the Double Number Line: A Visual Aid for Positive and Negative Values

In the realm of mathematics, the double number line emerges as an invaluable tool that allows us to explore the intricate world of both positive and negative values. It serves as a visual representation, painting a clear picture of the numerical landscape and its inhabitants.

Definition and Purpose

A double number line is essentially two parallel number lines placed side by side. It’s designed to help learners visualize the concepts of positive and negative numbers in a tangible way. By plotting values on both lines, the double number line offers a vivid illustration of how these numbers relate to zero and to each other.

Visualizing Positive and Negative Values

The double number line is a dynamic tool that enables us to see how positive and negative values interact. Positive numbers extend to the right of zero, while negative numbers stretch to the left. This arrangement allows us to grasp the concept of opposites in a tangible manner. For instance, the number +5 is located five units to the right of zero, while -5 lies five units to the left.

Elements of a Double Number Line

Parallel Number Lines

A double number line consists of two parallel number lines placed side by side, representing both positive and negative values. These parallel lines extend infinitely in both directions, visualizing the entire range of real numbers.

Zero as the Origin

The point where these parallel lines intersect is called the origin, represented by the number zero. Zero serves as the reference point for all distances and values on the number line. It is neither positive nor negative and divides the line into two separate halves: the positive half and the negative half.

Representation of Positive and Negative Numbers

Positive numbers lie to the right of zero, indicating values greater than zero. They are typically represented in black or red and are used to measure distances, amounts, or quantities that are present or gained.

Negative numbers, on the other hand, lie to the left of zero, representing values less than zero. They are usually colored blue or green and indicate absences, debts, or downward movement. The farther a number is from zero, either to the left or right, the greater its absolute value (magnitude).

Understanding Parallel Lines in Double Number Lines

In the realm of mathematics, double number lines serve as powerful tools for visualizing and understanding both positive and negative values. At the core of double number lines lies the concept of parallel lines, pivotal in creating a cohesive and intuitive representation of numerical domains.

Definition of Parallel Lines in Geometry:

In geometry, parallel lines are defined as lines that never intersect, extending indefinitely in the same direction. This characteristic of not crossing paths is crucial for the construction and operation of double number lines.

Role in Double Number Lines:

Double number lines consist of two parallel number lines, placed side-by-side. Each number line represents a different sign range – positive numbers on one line and negative numbers on the other. The parallel orientation of these lines allows for the seamless comparison of values and the visualization of numerical relationships.

Parallelism Ensures Clarity:

By using parallel lines, double number lines eliminate any ambiguity in representing opposite signs. The non-intersecting nature of these lines ensures that positive and negative values are clearly distinguished, preventing confusion and facilitating easier comprehension.

The concept of parallel lines in double number lines is fundamental in creating a clear and effective visual representation of numerical domains. By separating positive and negative values onto distinct, non-intersecting lines, double number lines empower learners with an intuitive understanding of various mathematical concepts, fostering confidence in working with both positive and negative numbers.

Breaking Down the Concept of Zero in a Double Number Line

In the realm of mathematics, double number lines serve as visual aids that help us understand the intricacies of positive and negative values. The concept of zero plays a pivotal role in these number lines.

Zero as the Reference Point

Imagine a double number line as a pair of parallel number lines, each extending indefinitely in opposite directions. Zero is the starting point, the reference point from which all other distances are measured. It’s like the starting line of a race, the point from which positive numbers run to the right and negative numbers run to the left.

Intersection Point of Positive and Negative Numbers

Zero is not merely the beginning; it also marks the meeting point of positive and negative numbers. Picture the positive number line as a beam of sunlight, extending to the right. The negative number line is like a shadow, stretching to the left. Zero is the fulcrum, the point where light and darkness converge.

This intersection point symbolizes the duality of numbers. Positive numbers represent quantities that increase or are above zero, while negative numbers represent quantities that decrease or are below zero. Zero itself stands as the neutral ground, neither positive nor negative.

The concept of zero in a double number line is fundamental to our understanding of positive and negative values. It provides a reference point for measurements, representing neither a gain nor a loss. As the intersection point of positive and negative numbers, it highlights the duality inherent in numbers, allowing us to grasp the intricacies of addition, subtraction, and other mathematical operations with greater ease.

Understanding the Concept of Positive Numbers on a Double Number Line

In the realm of mathematics, a double number line is a crucial tool for visualizing and manipulating numeric values, especially when working with both positive and negative numbers. Positive numbers are an essential component of this representation, playing a fundamental role in understanding the concept of a double number line and its applications.

Characteristics of Positive Numbers

Positive numbers are characterized by their magnitude and direction. The magnitude refers to the absolute value or size of the number, while the direction indicates its placement on the number line. Positive numbers are represented to the right of zero on the double number line. This rightward direction signifies an increase in value from the origin or reference point.

Representation of Positive Numbers

Positive numbers can be represented in various ways, including:

Whole numbers: These are positive integers without any fractional or decimal components. Examples include 1, 2, 3, and so on.
Decimals: Positive numbers can also be written as decimals, which represent fractional values. For instance, 0.5, 1.25, and 2.7 are all positive decimals.
Mixed numbers: Mixed numbers combine a whole number with a fraction. For example, 1 1/2, 2 3/4, and 3 5/8 are all mixed numbers that represent positive values.

Related Concepts

Two related concepts often associated with positive numbers are non-negative numbers and natural numbers.

Non-negative numbers: Non-negative numbers include both positive numbers and zero. They are represented on the double number line from zero and to the right.
Natural numbers: Natural numbers, also known as counting numbers, are a subset of positive integers that start from 1 and increase infinitely. They are used for counting and ordering objects.

Understanding the concept of positive numbers on a double number line provides a deeper comprehension of numeric values and their relationships. This knowledge forms the basis for more advanced mathematical operations and concepts, enabling students and professionals to tackle complex problems involving both positive and negative values.

Negative Numbers: The Dark Side of the Double Number Line

In the realm of mathematics, numbers extend beyond the familiar realm of positive integers into the enigmatic world of negative numbers. These enigmatic entities inhabit the left side of the double number line, a visual representation that guides our mathematical escapades.

Distinguishing Negative Numbers

Unlike their positive counterparts, negative numbers carry a special characteristic that sets them apart. They are represented by a minus sign (–) placed before the number, signifying their numerical _opposite. For instance, -5 is the numerical antithesis of 5.

Location on the Double Number Line

On the double number line, negative numbers reside left of zero, the mathematical equilibrium point. This placement reflects their less than relationship with positive numbers. As we venture further to the left, the negative numbers grow more negative, indicating an increasing distance from zero.