Understanding Velocity And Its Si Unit: Meters Per Second (M/S)

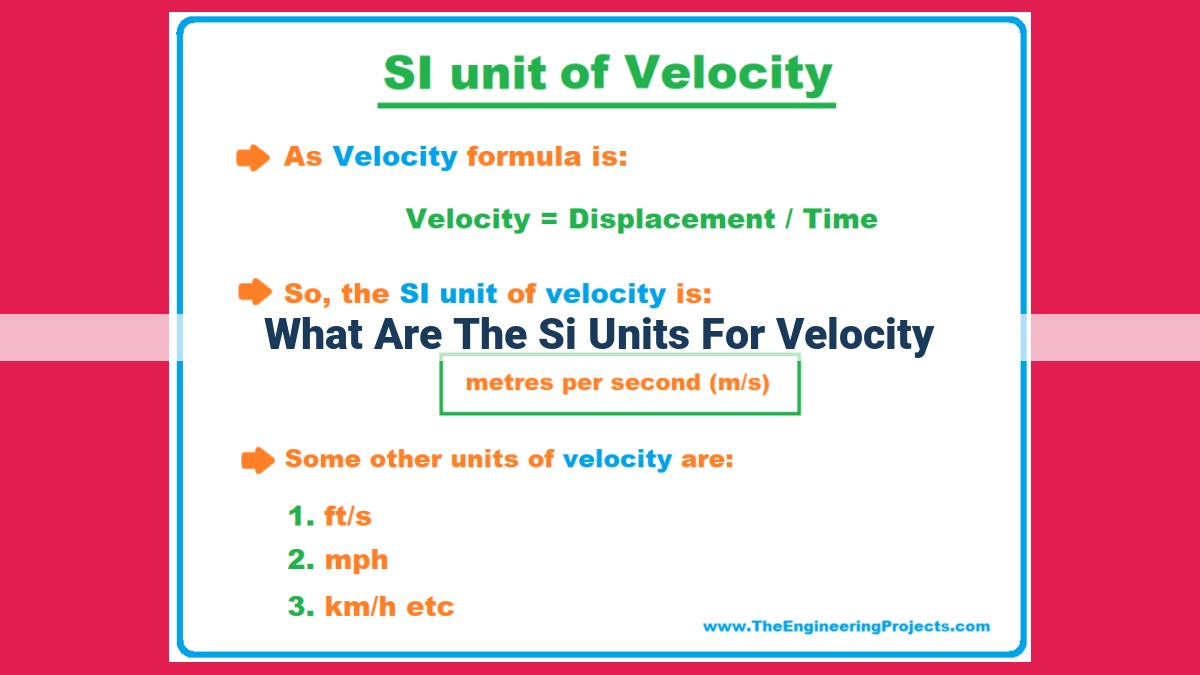
The SI unit for velocity is meters per second (m/s). Velocity measures an object’s speed and direction. Distance, measured in meters (m), is the length traveled, while time, measured in seconds (s), is the duration. Using the formula v = d / t, where v is velocity, d is distance, and t is time, we derive m/s as the SI unit for velocity. This unit represents the distance covered in one second, providing a precise measure of an object’s motion.

Velocity and SI Units: A Journey into the Dynamics of Motion

In our dynamic world, objects move at varying rates and directions. Understanding the measure of this motion, known as velocity, is crucial for comprehending the physical phenomena that surround us. To ensure precision and clarity in this measurement, we rely upon the International System of Units (SI), a standardized system of units used worldwide.

Unveiling Velocity: A Tale of Position and Time

Velocity is the rate at which an object changes its position over time. It quantifies how quickly and in what direction an object is moving. The SI unit of velocity is meters per second (m/s), indicating the distance traveled in one second.

Introducing the SI Family: A Unified Language of Measurement

The SI system provides a unified language for scientific and engineering communication, ensuring precision and clarity in measurements across borders and disciplines. When measuring velocity, we adhere to the SI units of meters (m) for distance and seconds (s) for time.

Distance: The Journey Traveled

Distance measures the length or extent of an object’s movement. In the SI system, distance is expressed in meters (m). Whether it’s a microscopic shift or a cosmic voyage, distance quantifies the path traversed.

Time: A Tapestry of Moments

Time measures the duration or interval between events. The SI unit of time is the second (s). From the fleeting blink of an eye to the vast expanse of geological ages, time serves as the canvas upon which motion unfolds.

Understanding the Velocity Formula: Unraveling the Equation of Motion

Velocity, a fundamental concept in physics, quantifies how quickly an object moves, capturing the speed and direction of its displacement over time. Its formula, v = d / t, serves as a mathematical cornerstone for understanding these aspects of motion.

The formula is composed of three key components:

• Distance (d): The total length traversed by the object. Visualize this as the ground you cover during a journey.

• Time (t): The duration for which the object is in motion. Think of it as the clock ticking as the object moves.

• Velocity (v): The object’s speed combined with its direction. Speed is the rate at which the object covers distance, while direction specifies the path it takes.

The formula expresses the intuitive relationship between these quantities: v = d / t. Velocity is directly proportional to distance and inversely proportional to time, meaning that a greater distance covered in less time results in a higher velocity.

Example:

Suppose you drive your car for 60 kilometers in 1 hour. Your velocity is calculated as:

v = 60 km / 1 hr
v = 60 km/hr

This value indicates that you traveled 60 kilometers during each hour of driving. The “km/hr” unit represents the distance you cover per unit of time.

By understanding the velocity formula, you can not only calculate an object’s velocity but also gain insights into how its motion varies with distance and time. This knowledge finds applications in various fields, from engineering to sports, where accurate measurements of speed and direction are crucial.

Measurement of Distance

• Define distance as the length traveled by an object.
• Discuss the SI unit of distance (meter) and other common units (km, cm).

Measurement of Distance: The Foundation of Velocity

Distance, a fundamental concept in physics, represents the length traveled by an object. Understanding this concept is crucial for comprehending velocity, a measure of how fast an object moves.

In the scientific community, the International System of Units (SI) serves as the standardized system for all measurements, including distance. The SI unit of distance is the meter (m). A meter is defined as the distance light travels in a vacuum in 1/299,792,458 of a second.

While the meter is the preferred unit in SI, other common units of distance include the kilometer (km) and centimeter (cm). The kilometer is equal to 1,000 meters, and the centimeter is 1/100 of a meter.

By understanding these units of distance, scientists can accurately measure and communicate the lengths objects travel, laying the foundation for determining their velocity.

Measurement of Time

• Define time as the duration of an event.
• Discuss the SI unit of time (second) and other common units (min, hr).

Understanding Time Measurement in Physics

Time is an essential concept in physics, serving as the measure of the duration of events. In scientific and engineering contexts, time is precisely measured using the International System of Units (SI), which defines the second as its fundamental unit.

The second is defined as the duration of 9,192,631,770 oscillations of a specific type of electromagnetic radiation emitted by cesium atoms. This definition ensures the utmost accuracy and consistency in timekeeping across the globe.

Apart from the second, other common units of time include minutes (min) and hours (hr). The relationship between these units is as follows:

• 1 min = 60 seconds
• 1 hr = 60 minutes = 3600 seconds

Understanding these time units is crucial for expressing and interpreting time-related information accurately. For example, a race that lasts for 10 minutes and 30 seconds would be written as 10.5 minutes.

Precise time measurement is essential in scientific research, engineering applications, and everyday life. From timing chemical reactions to monitoring traffic flow, the accurate measurement of time is ubiquitous in modern society.

Unveiling the SI Unit of Velocity: Meters per Second

In our quest to quantify motion, velocity stands as a crucial parameter, measuring the rate at which an object’s position changes over time. The International System of Units (SI), a global standard, plays an indispensable role in this measurement, providing a consistent and precise framework.

To unravel the SI unit of velocity, let’s delve into the fundamental formula that defines it: v = d / t. Here, v represents velocity, d denotes distance, and t signifies time.

Distance, measured in meters, quantifies the length traversed by an object. A meter, the SI unit of distance, constitutes a standardized measurement known worldwide. Similarly, time, expressed in seconds, gauges the duration of an event. A second, the SI unit of time, provides a precise and universal reference for temporal measurements.

Substituting meters for d and seconds for t in the velocity formula, we arrive at the SI unit of velocity: meters per second (m/s). This unit captures a profound concept: the distance covered by an object in one second. It’s a tangible representation of the object’s speed and the direction of its motion.

The significance of meters per second as a velocity unit is profound. It enables us to compare velocities across different objects and contexts, regardless of their size or trajectory. This standardized measurement ensures clarity and facilitates scientific collaboration.

Speed vs. Velocity: Unveiling the Key Distinction

In the realm of motion, understanding the difference between speed and velocity is crucial. While both terms describe the rate of an object’s movement, they differ in one fundamental aspect: direction. Velocity, a vector quantity, encompasses both the speed and the direction of motion, while speed, a scalar quantity, only measures the magnitude of motion.

Imagine a car traveling down the highway. Its speedometer displays its speed, which indicates how fast it’s moving but doesn’t tell us in which direction it’s headed. Velocity, on the other hand, provides a more complete picture by incorporating this missing information. It tells us not only how fast the car is going but also whether it’s moving towards the east, west, north, or south.

This distinction is essential in real-world applications. For instance, in navigation, knowing an object’s velocity allows us to precisely determine its position and predict its path. Engineers rely on velocity measurements to design vehicles and structures that can withstand specific forces and directions of motion.

Moreover, understanding the difference between speed and velocity is vital for accurate communication in science and engineering. Without precise definitions and standardized units, researchers and professionals would struggle to convey their findings and collaborate effectively.

Acceleration: A Related Concept

• Define acceleration as the rate of change of velocity.
• Explain how acceleration is related to velocity.

Acceleration: A Related Concept

Acceleration is another important concept related to velocity. It refers to the rate of change in velocity. This means that acceleration measures how quickly an object’s velocity is changing. Acceleration can be either positive or negative. Positive acceleration indicates that the velocity is increasing, while negative acceleration indicates that the velocity is decreasing.

Acceleration is directly proportional to the force acting on an object and inversely proportional to its mass. This relationship is expressed by Newton’s second law of motion, which states that F = ma, where F is the force, m is the mass, and a is the acceleration.

Acceleration is a vector quantity, meaning that it has both magnitude and direction. The direction of acceleration is the same as the direction of the net force acting on the object. For example, if a car is accelerating forward, the acceleration vector will point forward.

Acceleration is an important concept in physics, as it helps us to understand how objects move and interact with their environment. It is used in a wide variety of applications, including engineering, transportation, and sports.

Importance of SI Units in Velocity Measurements

Understanding velocity is crucial in various fields, from physics to engineering. Accurate velocity measurements rely heavily on standardized units, making the International System of Units (SI) indispensable.

Precision and Clarity: Imagine a team of scientists collaborating on a research project. If each scientist uses different units to measure velocity, confusion and misinterpretation can arise. SI units provide a common language, ensuring that all measurements are consistent and precise. This eliminates errors and allows for seamless data sharing and comparison.

International Collaboration: The scientific community is global, with researchers from diverse backgrounds working together. SI units serve as a universal standard, facilitating communication and collaboration across borders. By adopting SI units, scientists can exchange data and ideas confidently, knowing that they are using the same frame of reference.

Engineering Applications: In engineering, precise velocity measurements are essential for designing and constructing structures, vehicles, and machinery. Standardized units ensure that engineers can accurately calculate forces, stresses, and other factors that depend on velocity. Deviations from SI units can lead to costly mistakes and safety hazards.

In conclusion, SI units are not just arbitrary measurements; they are the foundation of accurate and reliable velocity measurements. Their use promotes precision, clarity, and international collaboration, enabling scientific advancements and engineering breakthroughs. Embracing SI units is crucial for ensuring that velocity measurements are meaningful, comparable, and universally understood.