Unveiling The Value Of X: A Comprehensive Guide To Geometric Theorems

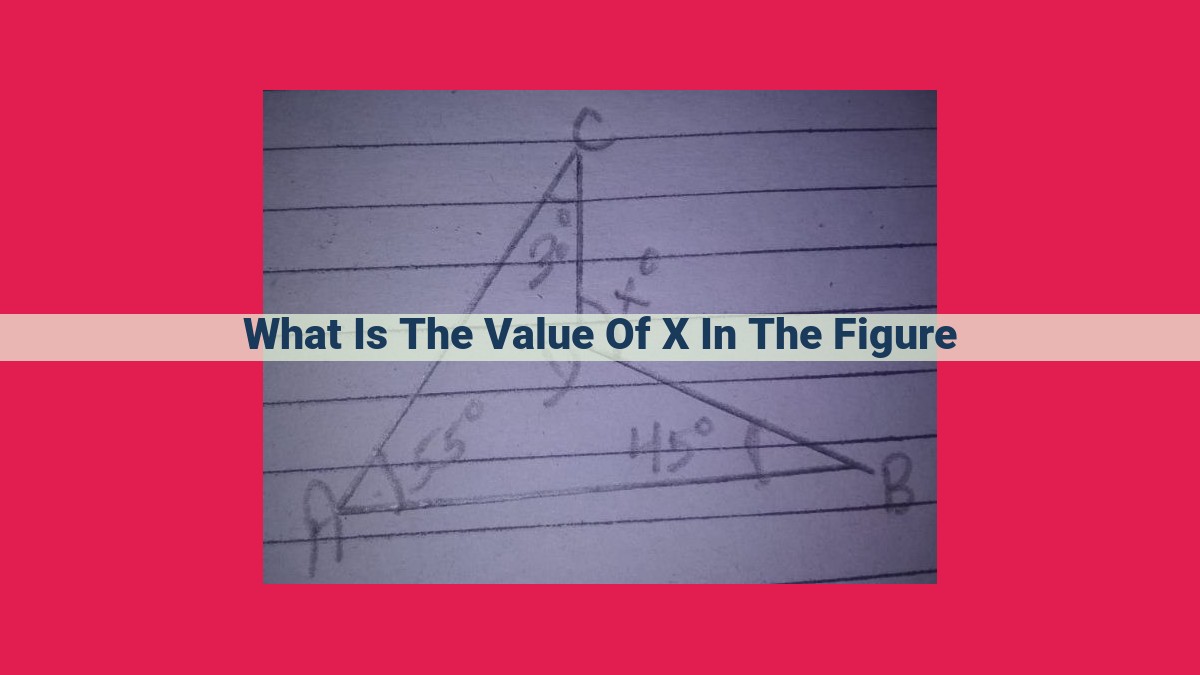
To find the value of x in a given figure, we utilize various geometric concepts. The angle bisector theorem helps determine the ratios of segments created by an angle bisector. The triangle proportionality theorem establishes relationships between segments in similar triangles. If applicable, the Pythagorean theorem can be employed to find missing side lengths in right triangles. By analyzing the figure to identify geometric relationships, we can apply these theorems in a step-by-step process to arrive at the value of x.

Unveiling the Angle Bisector Theorem: A Journey into Angle Division

In the realm of geometry, the Angle Bisector Theorem emerges as a profound tool that empowers us to comprehend the intricate relationships within angles and triangles. An angle bisector is like a fair and impartial ruler, gracefully dividing an angle into two congruent halves.

Constructing the Angle Bisector: A Step-by-Step Guide

To construct an angle bisector, we embark on a geometric adventure. Using a compass and a straight edge, we create two arcs of equal length with centers on each side of the angle. The intersection point of these arcs becomes the نقطة, from which we draw a line segment that intersects the angle’s vertex, elegantly bisecting it.

Properties of Angle Bisectors: Unveiling Hidden Truths

Angle bisectors possess remarkable properties that illuminate the secrets of triangles. For instance, the Angle Bisector Theorem states that any point on an angle bisector is equidistant from the sides of the angle. This means that the bisector acts as a neutral zone, ensuring equal access to both sides.

Moreover, if an angle bisector intersects the opposite side of a triangle, it divides that side into segments that are proportional to the adjacent sides. This fascinating property provides a powerful tool for exploring triangle relationships.

Triangle Bisectors: A Gateway to Triangle Division

In the realm of triangles, triangle bisectors play a pivotal role. A triangle bisector is a line segment that connects a vertex to the midpoint of the opposite side, effectively dividing the triangle into two smaller triangles. Interestingly, triangle bisectors exhibit properties similar to those of angle bisectors, ensuring equal access to the sides of the triangle and proportionality among the segments they create.

As we delve deeper into the enchanting world of geometry, the Angle Bisector Theorem and its associated concepts become invaluable tools for unlocking the secrets of angles and triangles. These principles empower us to navigate the intricacies of geometric figures with confidence and precision.

Triangle Proportionality Theorem: A Window into Geometric Harmony

In the realm of geometry, the Triangle Proportionality Theorem unlocks a hidden world of harmonious relationships between triangles. This theorem is a cornerstone of geometric understanding, enabling us to uncover the secrets of proportional segments, similar triangles, and the interplay of proportions within them.

The Concept of Proportional Segments

Imagine two parallel lines intersected by a transversal. The segments created by the transversal are in proportion. This means that the ratio of the lengths of one pair of segments is equal to the ratio of the lengths of the other pair.

Defining Similar Triangles

Two triangles are defined as similar if they have the same shape but not necessarily the same size. This means that their corresponding angles are congruent and their corresponding sides are proportional. The Triangle Proportionality Theorem provides a way to determine if triangles are similar.

Applications of Ratio and Proportion in Similar Triangles

The Triangle Proportionality Theorem has numerous applications in geometric problem solving. One of its key uses is to establish ratios and proportions between the segments of a triangle. This information can then be used to find the lengths of unknown segments.

For example, if we know that two sides of a triangle are in a ratio of 3:4 and the shorter side is 9 cm, we can use the proportion to find the length of the longer side. The proportion would be:

3/4 = 9 cm / x

Solving for x, we get:

x = 9 cm * 4/3 = 12 cm

Therefore, the length of the longer side is 12 cm.

The Triangle Proportionality Theorem is a fundamental concept in geometry that unlocks a deeper understanding of proportional segments, similar triangles, and the intricate relationships that exist within them. By mastering this theorem, we gain the ability to solve a wide range of geometric problems and uncover the hidden harmony that underlies geometric shapes.

The Enigmatic Pythagorean Theorem: Unlocking the Secrets of Right Triangles

In the realm of mathematics, where numbers dance and figures intertwine, there exists a theorem that has captivated minds for centuries: the Pythagorean Theorem. This legendary formula holds the key to unlocking the secrets of right triangles, those enigmatic shapes where one corner forms a perfect 90-degree angle.

A Journey into Square Roots

Before we unveil the theorem’s profound implications, let’s embark on a brief detour into the fascinating world of square roots. A square root is the number that, when multiplied by itself, yields the original number. For instance, the square root of 16 is 4, because 4 x 4 = 16.

Pythagorean Triples: The Perfect Threesomes

Now, let’s meet the Pythagorean triples, special sets of three numbers that form the lengths of the sides of a right triangle. The most famous Pythagorean triple is 3-4-5, where 3 represents the length of the shortest side (the opposite side of the right angle), 4 is the length of the middle side (the adjacent side to the right angle), and 5 is the length of the longest side (the hypotenuse). Other Pythagorean triples include 6-8-10, 5-12-13, and countless more.

The Essence of the Pythagorean Theorem

And now, the moment we’ve all been waiting for: the Pythagorean Theorem! This remarkable equation states that in a right triangle, the square of the hypotenuse (the longest side) is equal to the sum of the squares of the other two sides. In other words, a² + b² = c², where a and b are the lengths of the shorter sides and c is the length of the hypotenuse.

Unveiling the Power of the Theorem

The Pythagorean Theorem is a mathematical superpower that empowers us to solve a multitude of problems involving right triangles. For example, if we know the lengths of two sides, we can find the length of the third side. This knowledge is essential in fields ranging from architecture to surveying.

Furthermore, the Pythagorean Theorem provides a foundation for other important mathematical concepts, such as trigonometry and calculus. It’s a cornerstone of geometric understanding and a tool that continues to inspire and enlighten generations of students.

Unlocking the Secrets of Similar Triangles

In the realm of geometry, there exists a fascinating class of triangles known as similar triangles. These enchanting shapes share a mystical bond that unlocks a treasure trove of knowledge about proportions and relationships within their graceful forms.

Defining Triangle Similarity

Similar triangles, like kindred spirits, possess the same shape but may vary in size. This mesmerizing characteristic stems from their corresponding angles being congruent, meaning they have identical measures. Moreover, the ratios of their respective side lengths remain constant, revealing an intriguing pattern of proportionality.

The Magic of Scale Factor

A fundamental concept in the study of similar triangles is the scale factor. This enigmatic number represents the ratio of the corresponding side lengths of two similar triangles. Imagine two triangles, one nestled comfortably within the other, like a Russian doll’s intricate embrace. The scale factor serves as a bridge between these two geometric worlds, connecting them through a web of proportional relationships.

Properties and Relationships in Similar Triangles

The enchantment of similar triangles extends to a multitude of remarkable properties and relationships that govern their existence:

• Corresponding Side Ratios: The ratios of the lengths of corresponding sides are equal to the scale factor. This magical connection ensures that similar triangles maintain their harmonious shape despite their varying sizes.
• Area Ratio: The ratio of the areas of two similar triangles is equal to the square of the scale factor. This profound relationship reveals how the spaces encompassed by similar triangles grow proportionally.
• Ratio of Incenters: The ratio of the incenter radii of two similar triangles equals the scale factor. This hidden connection unveils the proportional relationship between the incenters, the points equidistant from all sides of a triangle.

Unraveling the Mystery of “x” with Geometry’s Helping Hand

In the realm of geometry, uncovering the value of the enigmatic variable “x” is a common quest. By weaving together concepts like angle bisectors, triangle proportionality, and the Pythagorean Theorem, we can embark on a geometric adventure to find the elusive “x.”

Step 1: Deciphering the Clues

Our journey begins with a curious figure, concealing geometric relationships. Like a detective scrutinizing the scene, we meticulously examine the lines, angles, and triangles within it. As we uncover these connections, we lay the groundwork for our mathematical expedition.

Step 2: Harnessing the Power of Angle Bisectors and Triangle Proportionality

With our geometric landscape mapped out, we summon the angle bisector theorem and triangle proportionality theorem as our trusty companions. Angle bisectors, like impartial mediators, divide angles into equal parts. They provide us with insights into relationships between line segments. Triangle proportionality, on the other hand, reveals the secrets of similar triangles. By establishing ratios and proportions, we unlock hidden connections within our figure.

Step 3: The Pythagorean Theorem: A Geometrical Lifeline

In certain cases, the Pythagorean Theorem, the cornerstone of right-triangle geometry, lends its support. This theorem enables us to establish relationships between the lengths of sides in right triangles. By harnessing its power, we can bridge the gap between seemingly disconnected segments.

Step 4: The Grand Finale: Unveiling the Value of “x”

Armed with our geometric arsenal, we embark on the final step of our quest: solving for “x.” Through a meticulous sequence of calculations, we apply the concepts we’ve explored to systematically unravel the value of the unknown. Each step takes us closer to our destination, as we navigate the labyrinth of lines and angles.

With patience and geometric prowess, we emerge victorious, having determined the elusive value of “x.” This journey through the world of geometry not only reveals a hidden truth but also empowers us to tackle future mathematical challenges with confidence. By embracing the synergy between concepts, we become masters of geometric problem-solving, unlocking the secrets hidden within the pages of our textbooks and the world around us.