Unlock The Significance Of Frequency: Measurement, Applications, And Importance In Science And Engineering

Frequency, a crucial parameter in science and engineering, measures the number of occurrences of a repetitive event over a specific time interval. It is quantified in Hertz (Hz), the SI unit representing one cycle per second. Frequency is inversely proportional to period, with a higher frequency indicating a shorter duration between events. In the context of electromagnetic radiation, frequency correlates with wavelength, as higher frequencies correspond to shorter wavelengths. Related concepts like periods per second, cycles per second, and vibrations per second all depict frequency in different contexts. The accurate measurement of frequency is essential in various fields, including physics, electronics, and signal processing.

Frequency: The Rhythm of the Universe

In the tapestry of our cosmos, events unfold at a myriad of paces. From the rapid oscillations of light to the stately orbit of planets, each movement possesses a characteristic beat—a frequency. Frequency, the rate at which a repetitive event occurs, is a fundamental concept in scientific and engineering disciplines, helping us decipher the rhythm of the universe.

Imagine a child swinging on a playground. The back-and-forth motion repeats itself at a steady rate, creating a cyclical pattern. The number of cycles completed in a given time, such as one second, defines the frequency of the swing. This same principle extends to every vibrating object, from the strings of a guitar to the atoms in a molecule.

Frequency is not merely an abstract concept; it has immense practical significance. In the realm of engineering, it governs the operation of oscillating circuits, determining the pitch of musical instruments, and synchronizing communication systems. In the medical field, it aids in diagnosing conditions like heart arrhythmias and brainwave patterns.

Hertz: The Maestro of Frequency

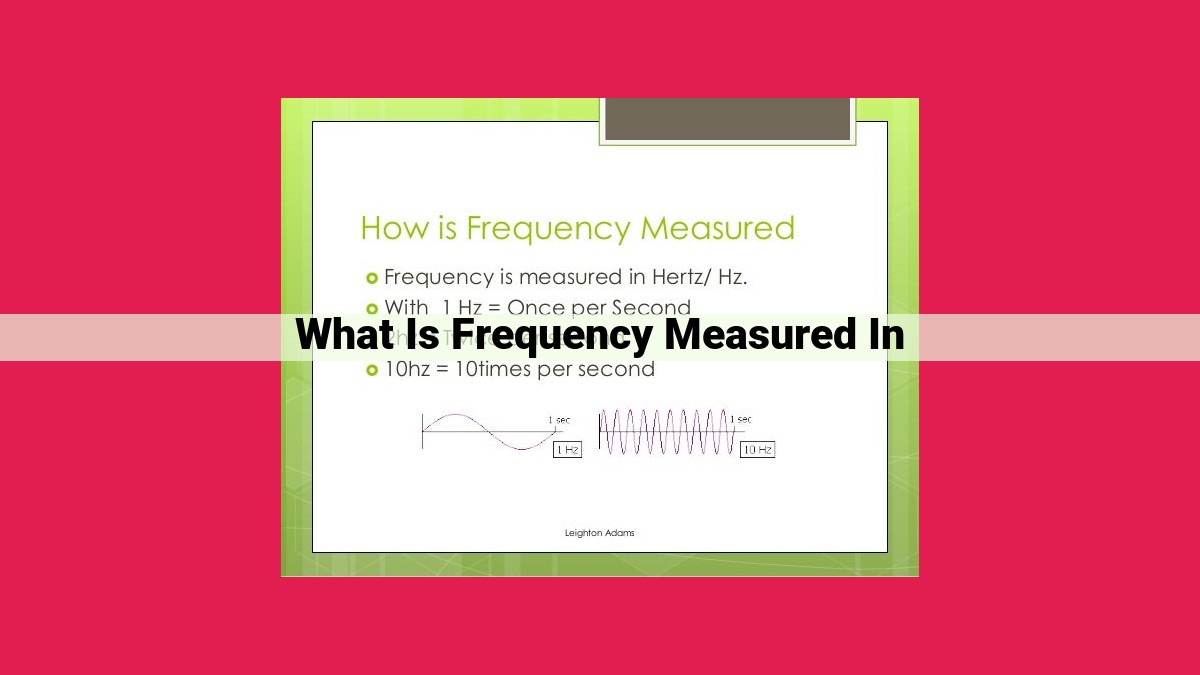
To quantify frequency, scientists have adopted the unit hertz (Hz), named after the renowned physicist Heinrich Hertz. One hertz corresponds to one cycle per second. A spinning fan rotating at 60 times per second, for instance, has a frequency of 60 Hz.

The inverse of frequency is known as period, the time it takes to complete one cycle. Measuring the duration between successive peaks or troughs of a wave, we can derive the period. Frequency and period are thus closely intertwined, offering complementary perspectives on the rhythm of events.

Beyond Hertz: Frequency in Diverse Realms

Frequency permeates far beyond the confines of hertz. In the realm of electromagnetism, it determines the energy of photons, with higher frequencies corresponding to higher energy. Sound waves, on the other hand, are characterized by their frequency, which dictates the pitch we hear.

The concept of frequency even extends to the human experience. Our brains process information in cycles, known as brainwaves, with different frequencies associated with different states of consciousness, from deep sleep to heightened alertness.

Frequency stands as an indispensable tool in our quest to understand the universe. It quantifies the rhythmic motion of objects, events, and even our own minds. From the pulsations of stars to the vibrations of molecules, frequency provides a unifying thread that weaves through the tapestry of existence. Whether in the laboratory or the concert hall, the concept of frequency empowers us to comprehend the intricate dance of the cosmos.

Hertz: The Universal Unit of Frequency

In the symphony of science and engineering, frequency plays a pivotal role, quantifying the rhythmic oscillations that govern our world. At its heart lies the Hertz (Hz), the SI unit of frequency, a testament to the groundbreaking work of Heinrich Hertz.

Cycles, Periods, and Vibrations: The Rhythm of Waves

Frequency measures the number of cycles completed per second, each cycle representing a complete oscillation. For instance, a sound wave oscillating at 1000 Hz completes 1000 cycles in one second. This measure is closely related to period, which represents the time taken for a single cycle to complete. The inverse relationship between frequency (f) and period (T) is expressed by the equation f = 1/T.

Wavelength and Frequency: The Dance of Light

Frequency also dances with wavelength, the distance between two consecutive peaks or troughs of a wave. In the realm of electromagnetic waves, such as light, higher frequencies correspond to shorter wavelengths. This relationship is elegantly summarized by the equation c = fλ, where c is the speed of light.

Hertz: Unveiling the Rhythm of the Universe

The Hertz, named after Heinrich Hertz, the pioneer of electromagnetic waves, has become the universal unit for measuring frequency. It unifies the diverse fields of science and engineering, from acoustics to electronics, providing a common language for understanding rhythmic phenomena.

Essential Concepts for Frequency Mastery

To fully grasp the concept of frequency, several related terms are essential:

• Periods per second: Equivalent to frequency, expressed in units of seconds.
• Cycles per second: Another name for frequency.
• Vibrations per second: Also equivalent to frequency.
• Wavelength: The distance between consecutive peaks or troughs of a wave.
• Time: The duration of a cycle or period.

Mastering these concepts enables a deeper understanding of the rhythmic patterns that shape our world, from the hum of a guitar string to the flicker of a fluorescent bulb.

Frequency and Periodicity: The Inverse Relationship

In the realm of science and engineering, frequency plays a crucial role, quantifying the rhythmic or oscillatory nature of phenomena. It measures the number of occurrences of a periodic event within a specified time interval. The SI unit of frequency is hertz (Hz), defined as one cycle per second.

Periodicity, on the other hand, describes the duration between successive occurrences of an event, expressed in seconds (s). Frequency and period are inversely related, signifying a fundamental connection between the two concepts. A simple formula, f = 1/T, elucidates this relationship, where f represents frequency and T stands for period.

Imagine a pendulum swinging back and forth. Its frequency, measured in Hz, is directly proportional to the number of complete oscillations it makes in a given number of seconds. Conversely, its period, measured in seconds, is the time it takes to complete one full cycle. As the frequency increases, the period decreases, and vice versa.

Frequency and Wavelength: A Tale of Inverse Proportionality

In the realm of science and engineering, frequency reigns supreme as a fundamental concept that measures the rhythmic repetition of a phenomenon. Often expressed in Hertz (Hz), the SI unit denoting one cycle per second, frequency unveils a world of rhythmic occurrences.

Just as a heartbeat has a period – the time between consecutive beats – every cyclical process possesses a wavelength. It represents the distance over which the pattern repeats itself. Now, here’s the fascinating connection: frequency and wavelength are inversely proportional.

Imagine a rhythmic wave propagating through a medium. High-frequency waves, characterized by rapid oscillations, squeeze into a shorter wavelength. On the other hand, low-frequency waves, with their leisurely gait, occupy a more extended wavelength. This inverse relationship is beautifully captured by the formula:

Speed of light (c) = Frequency (f) x Wavelength (λ)

As frequency increases, the wavelength must decrease to maintain a constant speed of light. This dance between frequency and wavelength holds true across the electromagnetic spectrum, from radio waves to gamma rays. Higher frequency radiation, like gamma rays, sports shorter wavelengths, while lower frequency waves, such as radio waves, stretch out over longer wavelengths.

Understanding this relationship empowers us to decode the secrets of the universe. From analyzing the frequency of light emitted by distant stars to mapping the brain’s electrical rhythms, this concept unlocks a wealth of scientific knowledge. So, as you witness the rhythmic ebb and flow of the world around you, remember the dance between frequency and wavelength – an invisible ballet that governs the very fabric of reality.

Related Concepts

In the realm of frequency, several related concepts play crucial roles:

Period: The duration of one complete cycle, measured in seconds (s). It represents the time it takes for a periodic motion to repeat itself.

Periods per Second: A measure of the frequency, indicating how many complete cycles occur within one second. It corresponds to the inverse of the period.

Cycles per Second: Synonymous with frequency, it quantifies the number of repetitions of a periodic event within one second. This term is interchangeable with hertz (Hz) and reflects the rate at which cycles occur.

Vibrations per Second: Similar to frequency, it denotes the number of vibrations or oscillations per second. This concept is often encountered in the context of acoustics, vibrations, and waveforms.

Wavelength: The spatial distance between two consecutive corresponding points, such as crests or troughs, in a periodic wave. It is inversely related to frequency: higher frequencies correspond to shorter wavelengths.

Time: The fundamental measure of duration, often expressed in seconds (s). It serves as the reference for period and frequency calculations.