Unlock The Power Of Unit Rates: A Comprehensive Guide

A unit rate describes the relationship between two quantities by comparing the value of one unit of one quantity to the corresponding value of the other quantity. It is a ratio that simplifies complex ratios by expressing the rate as a constant value. Unit rates allow for easy comparison between different quantities, determination of the best value for a given unit, and prediction of future values based on existing data. They are frequently represented as fractions or the slope of a line and have applications in various fields, including speed, cost, and population density.

Unit Rate: Making Sense of Ratios

In our daily lives, we often encounter situations where we need to compare quantities with different units. This is where unit rates come into play, serving as a powerful tool to help us understand and make sense of these comparisons.

What is a Unit Rate?

A unit rate is a ratio that compares the value of one unit of a quantity to the value of one unit of another quantity. It expresses the relationship between two quantities, allowing us to measure how much of one quantity we get for a given amount of the other.

For example, if you buy 12 apples for $6, the unit rate would be $6 ÷ 12 = $0.50 per apple. This means that for every apple you buy, it costs you $0.50. Unit rates are particularly useful when you want to compare prices or find the best value for your money.

**Understanding Unit Rate**

Ratio Concept and Unit Rates

Unit rates are special ratios that compare two quantities with different units. They simplify comparisons by expressing a value per unit, making it easier to compare quantities that may not otherwise be directly comparable. For example, if you’re comparing the speed of two cars, you need to consider both the distance traveled and the time taken. Expressing the speed as “miles per hour” creates a unit rate that allows for a fair comparison.

Fractions as Unit Rates

Fractions are another way to represent unit rates. A fraction, such as 2/5, represents the division of the numerator (2) by the denominator (5). In this case, 2/5 can be interpreted as a unit rate of 2 units per 5 units. This means that for every 5 units of the denominator quantity, there are 2 units of the numerator quantity.

Relationship between Unit Rates and Slope

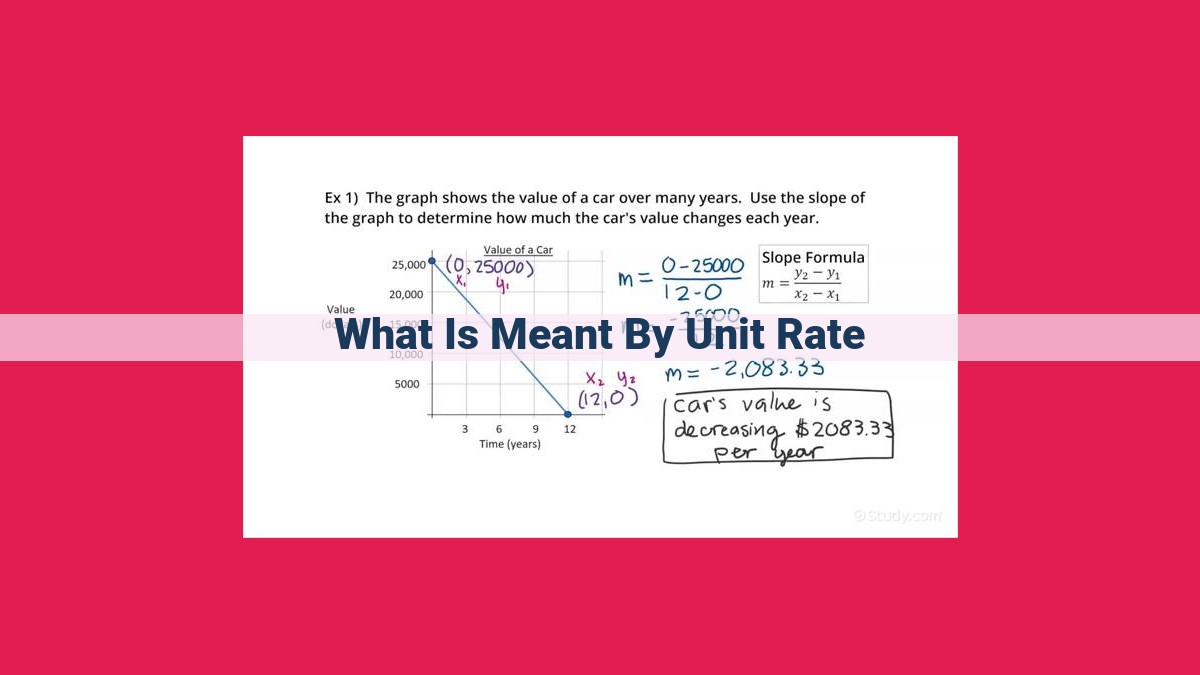
Unit rates are closely related to the slope of a line. In a linear relationship, the slope represents the rate of change between two variables. This rate of change can be expressed as a unit rate, where the numerator represents the change in the dependent variable and the denominator represents the change in the independent variable. By understanding the relationship between unit rates and slope, you can easily analyze and interpret linear relationships.

Examples of Unit Rate

Understanding unit rates is crucial in everyday life as they help us compare quantities with different units. Here are some practical examples to illustrate how unit rates work:

Speed:

Imagine you’re driving at a constant speed of 60 miles per hour. This means you’re covering _60 miles for every hour (_or_ 60 miles per 1 hour) you travel. The unit rate here is 60 miles per hour, representing how far you travel in one hour.

Cost:

Let’s say you’re buying apples at a grocery store. They cost $3 for 5 apples. To find the unit rate, you need to determine the cost per apple. Dividing the total cost ($3) by the number of apples (5) gives us $0.60 per apple. This unit rate tells you that each apple costs $0.60.

Population Density:

Consider a city with a population of 100,000 people living in an area of 100 square miles. To calculate the population density, you divide the population (100,000) by the area (100 square miles), resulting in 1,000 people per square mile. This unit rate indicates the average number of people living in each square mile of the city.

Applications of Unit Rate

Unit rates aren’t just abstract concepts; they have practical applications in our everyday lives. Let’s delve into how unit rates help us make informed decisions and understand the world around us:

Comparing Quantities with Different Units

Imagine you’re shopping for groceries and want to compare the prices of different brands of cereal. Brand A costs $3.50 for a 10-ounce box, while Brand B costs $4.20 for a 12-ounce box. Which brand is the better value?

To compare them, we need to find the unit rate, which is the price per ounce. For Brand A, the unit rate is $3.50 / 10 oz = $0.35 per ounce. For Brand B, the unit rate is $4.20 / 12 oz = $0.35 per ounce. They both have the same unit rate, so neither brand is a better value.

Determining the Best Value for a Given Unit

Now, let’s say you’re buying gasoline. One gas station offers regular unleaded for $3.20 per gallon, while another offers premium unleaded for $3.60 per gallon. You drive your car an average of 25 miles per gallon on regular unleaded. Which gas is the better choice?

To find out, we need to calculate how much it will cost you to drive a certain distance. Let’s say you want to drive 200 miles. With regular unleaded, it will cost 200 miles / 25 miles per gallon = 8 gallons. At $3.20 per gallon, it will cost you 8 gallons x $3.20 = $25.60.

With premium unleaded, it will cost you 200 miles / 25 miles per gallon = 8 gallons. At $3.60 per gallon, it will cost you 8 gallons x $3.60 = $28.80.

Using Unit Rates to Make Predictions

Unit rates can also help us predict future outcomes based on past trends. For example, if you know the unit rate of population growth in a certain city, you can estimate the population in the future years.

Let’s say the population of a city is currently 100,000 and the unit rate of growth is 2% per year. To predict the population in 5 years, we can use the formula:

Predicted population = Current population x (1 + unit rate of growth)^(number of years)

Plugging in the values, we get:

Predicted population = 100,000 x (1 + 0.02)^5 = 110,408

Therefore, we can estimate that the population of the city will be approximately 110,408 in 5 years, assuming the unit rate of growth remains constant.

Unit rates are a powerful tool for understanding and making decisions about the world around us. By comparing quantities, determining the best value, and making predictions, we can use unit rates to make informed choices and gain a deeper understanding of everyday situations.

Unlocking the Power of Unit Rates and Related Concepts

In the realm of mathematics, the concept of unit rate shines as a beacon of clarity, empowering us to compare seemingly disparate quantities with ease. From understanding the speed of a car to comparing the value of different products, unit rates provide a universal language that bridges the gap between units.

Related Concepts

Exploring the world of unit rates also leads us to other indispensable mathematical concepts:

• Rate of Change: Unit rates are the building blocks of rate of change, which measures how quickly a quantity changes over time. Understanding unit rates is crucial for calculating rates of change in various scenarios.

• Proportions: Unit rates are intimately connected to proportions, which state that two ratios are equal. This relationship allows us to make comparisons between quantities with different units, such as comparing the speed of two cars or the population density of two cities.

• Equations: Unit rates can be expressed as equations, such as distance = rate × time. These equations provide a powerful tool for solving real-world problems involving rates.

• Conversion Factors: Unit rates also play a vital role in converting between units. For instance, a conversion factor of 1 kilometer per 0.6214 miles allows us to convert distances between kilometers and miles.

Mastering Proportions and Equations with Unit Rates

To illustrate the significance of these related concepts, consider the following example:

Imagine you’re driving a car at a constant speed and notice that you travel 60 miles in 2 hours. The unit rate for speed is calculated as follows:

Unit Rate = Distance ÷ Time = 60 miles ÷ 2 hours = 30 miles per hour

This unit rate can then be used to answer various questions:

• What is the rate of change in distance with respect to time?

  • The rate of change is 30 miles per hour, which means that for every hour you drive, you travel 30 miles.

• If you continue driving at the same speed for another 4 hours, how far will you travel?

  • Using a proportion, we can set up the following equation:

  30 miles/hour = distance / 4 hours
  

  Solving for distance, we get:

  Distance = 30 miles/hour * 4 hours = 120 miles
  

This example demonstrates the power of unit rates in real-world problem-solving. By understanding the related concepts of rate of change, proportions, and equations, we can unlock the full potential of unit rates in various mathematical applications.

The Constant of Proportionality: Unveiling the Secret Behind Proportional Relationships

In the realm of mathematics, where numbers dance and relationships unfold, we encounter the intriguing concept of the constant of proportionality. This elusive entity plays a pivotal role in proportional relationships, especially when unit rates are at play. Let’s embark on a storytelling journey to unravel the mysteries surrounding this fascinating concept.

Imagine a world where all things move in harmony, where the distance traveled is always directly proportional to the time spent traveling. This proportional relationship can be expressed mathematically as:

distance = speed x time

In this equation, the constant of proportionality is speed. It represents the unit rate at which distance is covered per unit of time. The constant of proportionality remains constant throughout the relationship, ensuring that the two quantities remain in perfect balance.

The constant of proportionality can be thought of as a magical multiplier that transforms one quantity into the other. In our example, speed is the constant of proportionality that converts time into distance. This conversion factor allows us to predict the distance traveled based on the given time and vice versa.

For instance, if the speed is 10 miles per hour, then every hour spent traveling will result in 10 miles of ground covered. This predictability makes proportional relationships incredibly useful in various fields, including science, engineering, and economics.

The constant of proportionality is not limited to speed. It can represent any constant ratio that connects two or more quantities. In a grocery store, the constant of proportionality might be the unit price that relates the total cost of an item to the number of items purchased. In a medical setting, the constant of proportionality might be the dosage rate that determines the amount of medication administered based on the patient’s weight.

Understanding the constant of proportionality is key to interpreting and solving proportional relationships. It provides a deep insight into the nature of these relationships, allowing us to predict, compare, and make informed decisions based on the available data. As we continue to explore the fascinating world of math, the constant of proportionality will undoubtedly remain a guiding light, unraveling the secrets of many a proportional mystery.