Understanding The Slope-Intercept Equation: Unlocking The Relationship Between Slope And Y-Intercept
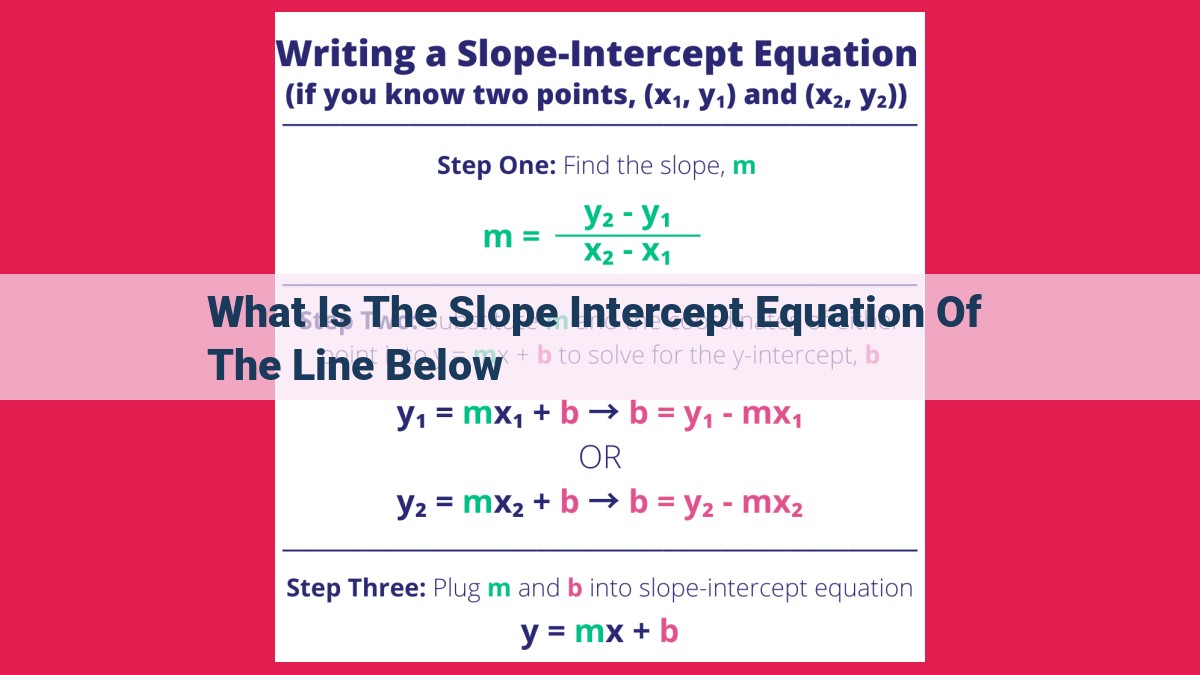
The slope-intercept equation of a line, y = mx + b, describes the relationship between the slope (m) and the y-intercept (b) of a line. The slope represents the steepness of the line, while the y-intercept is the point where the line intersects the y-axis. The equation can be used to write equations of lines given their slope and y-intercept, or to find the slope and y-intercept of a line from its equation.
Unveiling the Secrets of Slope: Measuring the Gradient of Lines
In the realm of mathematics, understanding the concept of slope is fundamental for deciphering the intricate world of lines. It’s a measure of how steep a line is, revealing the secrets of its angle and gradient.
Defining Slope: A Ratio of Change
At its core, slope represents the ratio between the vertical (up/down) change over the horizontal (left/right) change along a line. Imagine climbing a hill; the steeper the hill, the greater the slope. Conversely, a gentle slope indicates a more gradual change in elevation.
Significance of Slope: Angles and Gradients
Understanding slope is crucial for describing line angles and gradients. A positive slope indicates an upward-sloping line, while a negative slope denotes a downward-sloping line. The absolute value of the slope quantifies the steepness of the line, with larger values corresponding to more pronounced angles.
Understanding the Y-Intercept: The Key to Line Positioning
In the realm of mathematics, a line is a crucial concept that forms the foundation of geometry and higher-level math. Just like a path leads to a destination, a line is defined by its path, which is determined by its slope and y-intercept. While slope describes the line’s inclination, the y-intercept holds the key to its vertical positioning.
The y-intercept is the point where the line intersects the y-axis. It is represented by the letter “b” in the standard slope-intercept equation: y = mx + b. This point is crucial because it determines the vertical displacement of the line. In other words, it tells us where the line starts on the y-axis.
To understand the role of the y-intercept, imagine a line passing through two points: the origin (0,0) and another point (5,3). The line starts at the origin and rises to reach the point (5,3). The y-intercept in this case is 0, which means the line begins right on the y-axis.
Now, let’s consider a different line that passes through the same point (5,3) but has a different y-intercept. Let’s say the new y-intercept is 2. This means the line will start 2 units above the y-axis at the point (0,2). As the line extends, it will maintain the same slope as the previous line, but its vertical displacement will be different.
The y-intercept provides valuable information about the line’s origin. It tells us where the line intersects the y-axis, which can be particularly useful when graphing or analyzing equations. By understanding the y-intercept, we gain insight into the vertical position of the line and how it relates to other points on the line.
Understanding the Slope-Intercept Equation (y = mx + b): A Comprehensive Guide
In the realm of mathematics, lines play a pivotal role in describing various relationships. To accurately represent these lines, we use the slope-intercept equation, which provides a concise and informative description of their behavior and key characteristics.
Defining the Slope-Intercept Equation
The slope-intercept equation, expressed as y = mx + b, is a mathematical representation of a straight line. It consists of four components:
- y represents the dependent variable, whose value is determined by the values of x and the other constants.
- x is the independent variable, which can take on any value within the domain of the line.
- m is the slope, which indicates the steepness or gradient of the line. It is calculated as the ratio of the change in y to the change in x.
- b is the y-intercept, which represents the point where the line crosses the y-axis.
The Slope: Quantifying Line Steepness
The slope, denoted by m, is a crucial parameter that determines the line’s inclination. A positive slope means the line rises from left to right, while a negative slope indicates a line that falls from left to right. The magnitude of the slope quantifies the steepness of the line: a higher absolute value of m corresponds to a steeper line.
The Y-Intercept: A Vertical Milestone
The y-intercept, denoted by b, is the point where the line intersects the y-axis, which means when x = 0. It represents the initial vertical displacement of the line from the origin. A positive y-intercept means the line starts above the origin, while a negative y-intercept indicates a starting point below the origin.
Interplay of Slope and Intercept
The slope and y-intercept provide a comprehensive picture of a line’s behavior. The slope determines the angle at which the line rises or falls, while the y-intercept indicates its vertical position. By knowing both m and b, we can accurately graph the line and predict its course.
In summary, the slope-intercept equation, y = mx + b, is a powerful tool for representing and analyzing straight lines. It allows us to quantify their steepness, vertical displacement, and overall behavior, making it an essential equation in the study of mathematics and its applications.
Equation of a Line: The Math Behind Line Relationships
In the world of geometry and algebra, understanding the equation of a line is crucial for describing and analyzing line graphs. It’s a mathematical representation that captures the unique characteristics of a line, allowing us to explore its slope, intercept, and behavior.
An equation of a line is a mathematical statement that defines a line in the coordinate plane. It describes the relationship between the dependent and independent variables, which are typically represented by y and x, respectively. There are several different forms of line equations, each with its own advantages for different purposes.
The most common form of line equation is the slope-intercept form, which is written as y = mx + b. Here, m represents the slope of the line, which measures its steepness or angle of inclination. The variable b represents the y-intercept, which is the point where the line crosses the y-axis. The slope and y-intercept are two key features that uniquely characterize a line.
Other forms of line equations include point-slope form, two-point form, and standard form. Each of these forms has its own specific applications and advantages. For example, point-slope form is useful for writing the equation of a line when you know a specific point on the line and its slope. Two-point form is commonly used when you know the coordinates of two points on the line.
Understanding the different forms of line equations empowers you to describe, analyze, and solve problems related to lines in various contexts. Whether you’re a student studying geometry, a researcher exploring data, or an engineer designing structures, the equation of a line is a powerful tool that unlocks the secrets of line relationships.
Point-Slope Form: Unlocking the Equation with Known Points
In the realm of mathematics, equations of lines play a pivotal role in describing the behavior and relationships between variables. Among the various forms of line equations, the point-slope form stands out as a powerful tool for effortlessly writing an equation when armed with a known point and the slope of the line.
Imagine you’re an explorer embarking on a trail, and you come across a signpost that reads: “Point (3, 5), Slope = 2”. This signpost provides you with a point on the line, (3, 5), denoted as (x1, y1), and the slope of the line, m. Your task is to decipher the equation of this line using the point-slope form.
To achieve this, we embark on a step-by-step journey:
- Summon the Magical Formula: The point-slope form reads as follows:
y - y1 = m(x - x1). In this formula, (x1, y1) represents the given point, and m symbolizes the slope of the line. - Substituting the Known Values: Plug the values of (x1, y1) and m into the formula, yielding
y - 5 = 2(x - 3). - Simplifying the Equation: Distribute the slope to get
y - 5 = 2x - 6. - Rearranging for y: Adding 5 to both sides, we obtain the final equation of the line in slope-intercept form:
y = 2x - 1.
And there you have it! Using the point-slope form, you’ve successfully transformed the given information into a mathematical equation that precisely describes the line passing through point (3, 5) with a slope of 2.
Next time you encounter a situation where you know a point and the slope of a line, remember the point-slope form. It’s a handy tool that will effortlessly guide you to the equation that captures the essence of that line.
Equation of a Line: Unlocking the Two-Point Form
When it comes to describing a line, the slope-intercept form is a familiar face. But what if you’re given two points instead? Enter the two-point form, a powerful tool that empowers you to craft an equation with ease.
Let’s break it down. Consider two distinct points on a line: (x1, y1) and (x2, y2). The slope (m) between these points represents the line’s steepness and can be calculated using the formula:
m = (y2 - y1) / (x2 - x1)
Now, with slope in hand, we can construct the two-point form equation:
y - y1 = m(x - x1)
By substituting the values of x1, y1, and m, we can write the equation of the line in a heartbeat.
For instance, given points (2, 5) and (6, 11), we calculate the slope:
m = (11 - 5) / (6 - 2) = 2
Plugging this into the two-point form, we get:
y - 5 = 2(x - 2)
This equation accurately captures the relationship between the given points, allowing us to describe the line’s path through the coordinate plane.
Remember, the two-point form is particularly handy when working with lines that don’t intersect the y-axis, making it an indispensable tool in your linear equation toolbox.