Understanding The S Sublevel: A Comprehensive Guide

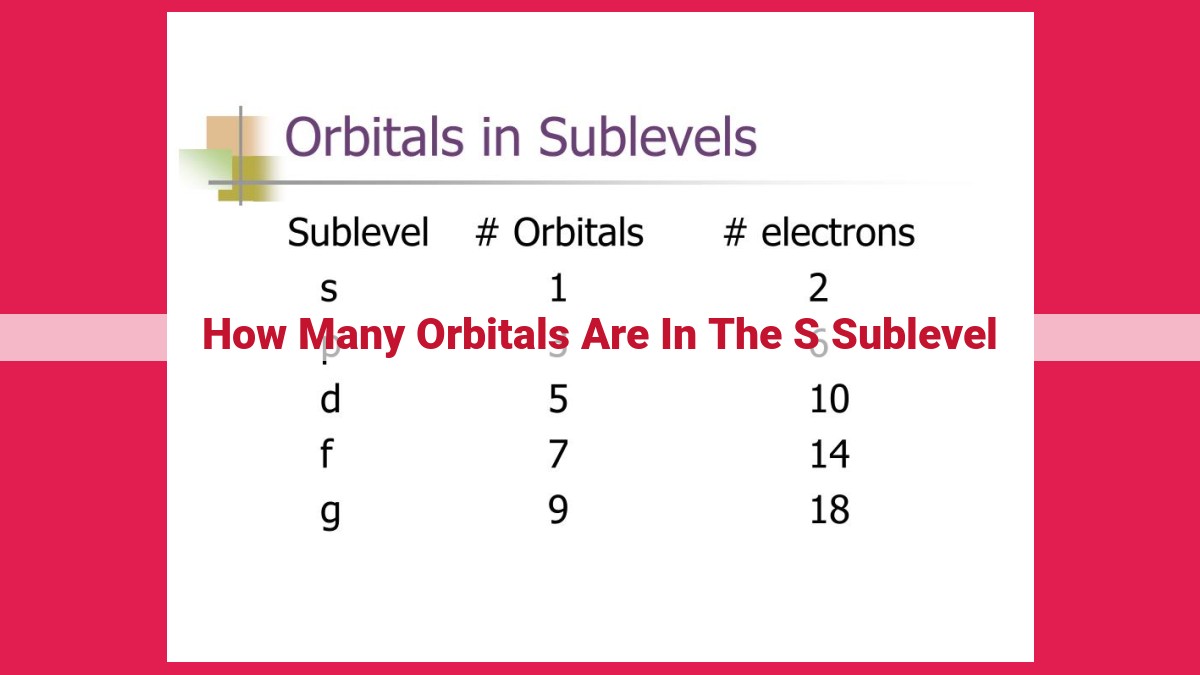
The s sublevel, designated by l = 0, has a spherical shape and represents the lowest energy level within an atom. It corresponds to s orbitals, which are characterized by their spherical symmetry. Each principal quantum number (n) value has one s sublevel, meaning that for n = 1, there is only one s orbital in the s sublevel. Therefore, the s sublevel contains only one orbital.

Quantum Mechanics and Atomic Orbitals:

• Introduce the basic concepts of quantum mechanics.
• Define atomic orbitals as regions around the nucleus where electrons are likely to be found.

Quantum Mechanics and Atomic Orbitals: A Journey into the Microscopic Realm

Prepare to embark on an extraordinary journey into the fascinating world of quantum mechanics, where the fundamental principles governing the universe unfold. In the realm of atoms, electrons dance around the nucleus in specific patterns, occupying distinct regions known as atomic orbitals.

An atomic orbital represents the probable location of an electron around the nucleus. It is not like a solid sphere but rather a cloud-like region where an electron is most likely to be found. Quantum mechanics provides the framework for understanding these orbitals and their properties.

Shells and Subshells: The Architecture of Atoms

Imagine the atom as a tiny solar system, with the nucleus as the sun and electrons orbiting around it. The electrons are organized into shells, like the planets orbiting a star, with each shell corresponding to a different energy level. Within each shell lie subshells, which are designated by the letters s, p, d, and f. Each subshell has a specific shape and energy.

The s sublevel is the first and simplest subshell, with a spherical shape. It is analogous to a planet orbiting in a perfectly circular path around a star. The s sublevel can only accommodate a maximum of two electrons, which must have opposite spins.

Quantum Numbers: Unlocking the Mysteries of Orbitals

To fully understand atomic orbitals, we need to introduce the concept of quantum numbers. These numbers describe the specific properties of each orbital:

• Principal quantum number (n): Indicates the energy level of the shell.
• Azimuthal quantum number (l): Determines the shape of the subshell.
• Magnetic quantum number (ml): Specifies the orientation of the orbital within the subshell.

Delving into the s Sublevel

The s sublevel has a value of l = 0, which corresponds to a spherical shape. Since there is only one possible orientation for an orbital with l = 0, there is only one orbital in the s sublevel. This orbital is denoted as 1s, where “1” represents the principal quantum number.

In the tapestry of atomic structure, the s sublevel stands out as a unique entity, housing only a single electron. This solitary orbital serves as a testament to the fundamental principles of quantum mechanics, which govern the intricate dance of electrons around the atomic nucleus.

The Enigmatic s Sublevel: Delving into the Heart of Atomic Orbitals

Embark on a Quantum Journey

As we dive into the captivating world of quantum mechanics, let’s unravel the secrets of atomic orbitals, the enigmatic spaces where electrons reside. These ethereal realms govern the chemical behavior of elements, shaping their properties and interactions.

The s Sublevel: A Spherical Sanctuary

Within the atom’s energy ladder, the s sublevel occupies a special place. It’s a single energy level with a unique characteristic: its orbitals are spherical. Imagine electrons dancing around the nucleus in a cloud of equal probability, like celestial bodies circling a star.

Principal Quantum Number: Distance from the Nucleus

The principal quantum number (n) dictates the energy level of an orbital. The higher the n, the further the orbital resides from the nucleus. The s sublevel corresponds to the n = 1 energy level, meaning its orbitals are the closest to the nucleus.

A Journey into Shapes

The azimuthal quantum number (l) governs the shape of an orbital. For the s sublevel, l is always 0. This means that s orbitals are always spherical. Their spherical symmetry makes them the most compact and lowest-energy orbitals within a given energy level.

The Principal Quantum Number: Unveiling the Atomic Energy Ladder

In the realm of quantum mechanics, the principal quantum number (n) reigns as a governing force, dictating the energy levels of electrons within an atom. Like rungs on a ladder, each value of n represents a distinct energy level, with higher values corresponding to greater distances from the nucleus.

n and the Orbital Hierarchy

From the innermost energy level outward, n assumes values starting with 1. Each level, known as a principal energy shell, accommodates a specific number of orbitals. These orbitals, geometric regions where electrons reside, come in various shapes and orientations, designated by the azimuthal and magnetic quantum numbers.

n and the Orbital Types

The principal quantum number also plays a crucial role in determining the type of orbital an electron occupies. As n increases, the number of possible orbital types expands. The first energy shell (n = 1) can only accommodate s orbitals, characterized by their spherical shape. The second energy shell (n = 2) introduces p orbitals, with dumbbell-shaped lobes, along with s orbitals. The pattern continues, with each successive energy shell adding new orbital types: d orbitals (n = 3), f orbitals (n = 4), and so forth.

n and the Electron Distribution

The principal quantum number influences the distribution of electrons within an atom. Electrons naturally seek the lowest energy levels available. Thus, electrons in the first energy shell (n = 1) are closer to the nucleus and possess the lowest energy. As electrons occupy higher energy shells (n = 2, 3, etc.), they become progressively farther from the nucleus and acquire higher energies.

The principal quantum number (n) serves as a fundamental parameter in understanding atomic structure. It governs the energy levels of electrons, determines the types of orbitals they occupy, and influences the overall electron distribution within an atom. By unraveling the mysteries of n, we gain deeper insights into the intricate world of quantum mechanics and the behavior of matter at its most fundamental level.

Azimuthal Quantum Number (l):

• Define the azimuthal quantum number as a number that indicates the shape of an orbital.
• Explain that it determines whether an orbital is s, p, d, or f type.
• Describe the subshells associated with different l values.

The Azimuthal Quantum Number: Shaping the Orbitals

As we delve deeper into the fascinating realm of quantum mechanics, we encounter the concept of the azimuthal quantum number, also known as the orbital angular momentum quantum number or l. This enigmatic number plays a pivotal role in shaping the geometry of atomic orbitals, the ethereal spaces where electrons dance around the nucleus.

Imagine an atom as a miniature solar system, with the nucleus as the central sun and electrons orbiting it like planets. The principal quantum number (n), which we discussed earlier, determines the electron’s energy level, akin to the distance of a planet from the sun. The azimuthal quantum number, on the other hand, governs the shape of the electron’s orbital, just as the eccentricity of a planet’s orbit determines its shape.

The azimuthal quantum number assumes values from 0 to n-1. Each value of l corresponds to a specific type of orbital:

• l = 0: s orbital, characterized by a spherical shape.
• l = 1: p orbital, with a dumbbell-shaped, three-dimensional representation.
• l = 2: d orbital, possessing a more complex, four-lobed shape.
• l = 3: f orbital, featuring even more intricate, multi-lobed geometries.

These different orbital shapes are essential for understanding the chemical properties of elements. s orbitals are closest to the nucleus and have the lowest energy, while higher l values indicate orbitals that are farther from the nucleus and have higher energy.

The azimuthal quantum number not only determines the orbital shape but also gives rise to subshells. Each l value corresponds to a specific number of subshells, which are denoted by the letters s, p, d, and f. For example, l = 0 corresponds to the s subshell, l = 1 corresponds to three p subshells (labeled px, py, and pz), and so on.

The combination of the principal quantum number (n) and the azimuthal quantum number (l) provides a precise description of each atomic orbital. For instance, the 1s orbital indicates an electron in the first energy level (n = 1) and the spherical s subshell (l = 0).

In summary, the azimuthal quantum number (l) is a crucial factor in shaping the geometry of atomic orbitals. It governs the type of orbital (s, p, d, or f) and determines the number of associated subshells. Together with the principal quantum number, it forms the foundation for understanding the intricate dance of electrons in atoms.

The Magnetic Quantum Number: Unraveling the Orientation of Orbitals

Quantum mechanics, with its baffling world of atomic orbitals, can seem like a daunting enigma. But fear not, for we’re here to shed light on one of its enigmatic components: the magnetic quantum number.

Picture this: An electron, like a tiny dancer, pirouettes within an atomic orbital. Imagine the orbital as a stage, where the electron’s pirouette is determined by three factors – its energy level, shape, and orientation. The magnetic quantum number governs this last crucial element – the orientation of the electron’s pirouette.

The magnetic quantum number, denoted by ml, behaves like a compass needle, pointing the way for the electron’s dance. For a given energy level and shape, it specifies the number of possible orientations the electron can adopt. Each orientation is like a unique step in the electron’s pirouette, giving rise to a different orbital within a sublevel.

For instance, in the p sublevel (l = 1), three orientations are possible, leading to three p orbitals labeled px, py, and pz. These orbitals resemble dumbbells oriented along the x, y, and z axes, respectively.

Hund’s rule plays a pivotal role here, dictating how electrons fill orbitals. It asserts that electrons prefer to occupy orbitals with the same spin before pairing up. This dance of electrons influences the magnetic properties of atoms and molecules.

So, to unravel the mysteries of quantum mechanics, remember the magnetic quantum number. It’s the compass that guides electrons’ pirouettes, determining their orientation within the atomic dance. Embrace its enigmatic charm, and the world of quantum realms will gracefully unveil its secrets.

The Number of Orbitals in the S Sublevel: Unveiling Quantum Mechanics’ Secrets

In the captivating realm of atomic structure, the s sublevel emerges as a fundamental building block, shaping the distribution of electrons around the nucleus. To grasp the intricate nature of this sublevel, let’s delve into the fascinating world of quantum mechanics.

The s sublevel, denoted by the azimuthal quantum number l = 0, represents a unique energy level within an atom. Its peculiar characteristic lies in the spherical shape of its associated orbitals. These orbitals, like tiny electron clouds, envelop the nucleus, defining regions where electrons are most likely to reside.

Crucially, the value of l also dictates the number of orbitals within a sublevel. For the s sublevel, with l = 0, there exists only one possible value for the magnetic quantum number ml. This enigmatic number, ml, governs the orientation of orbitals in space. For l = 0, ml can only be 0.

Consequently, the s sublevel possesses a single orbital, often labeled as the 1s orbital. This solitary orbital stands alone, distinct from its p, d, and f counterparts, which boast multiple orbitals due to higher l values.

In conclusion, the s sublevel, characterized by l = 0 and ml = 0, harbors only one orbital. This fundamental understanding unveils the intricate tapestry of atomic structure, guiding us deeper into the enigmatic realm of quantum mechanics.