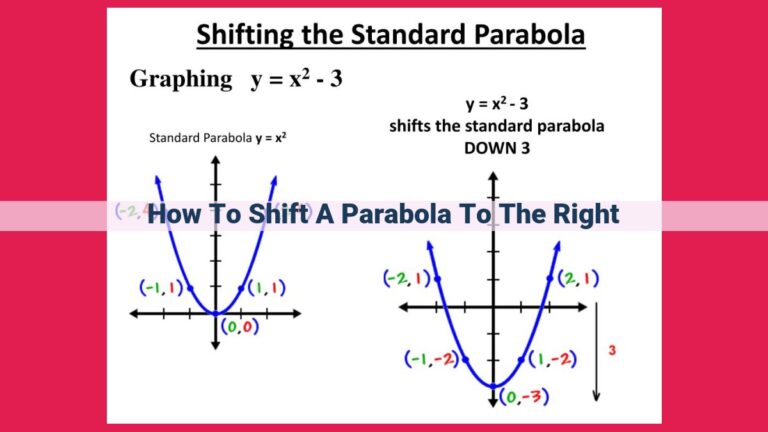
Understanding Root Types In Polynomial Equations: Real, Complex, Rational, And Non-Conjugate
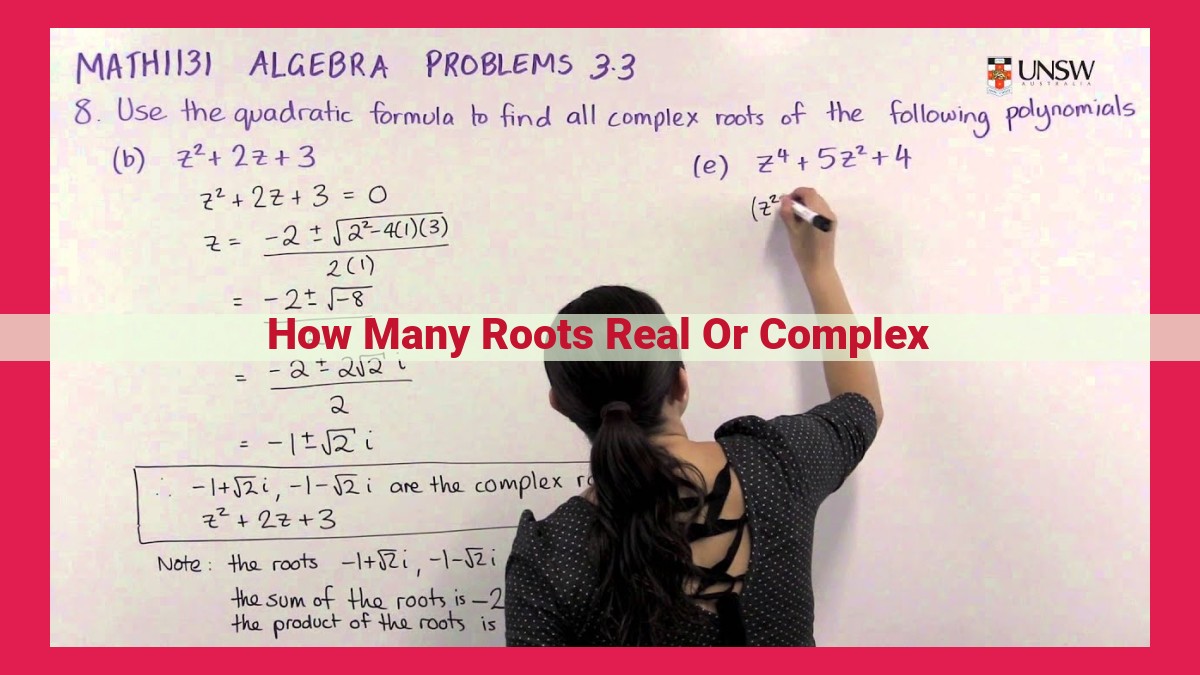
Real roots are those that fall on the real number line, while complex roots involve imaginary numbers and occur in conjugate pairs. The number of roots for a polynomial equation is determined by the Fundamental Theorem of Algebra, which states that the number of roots equals the degree of the polynomial. Rational roots can be found using the Rational Roots Theorem, and they are either rational or irrational. Non-conjugate real roots are independent and unrelated, while conjugate real roots occur in pairs with the same magnitude but opposite signs. Complex conjugate roots also exist in pairs, featuring the same magnitude but differ in the sign of their imaginary parts. Understanding these root types helps in solving polynomial equations by narrowing down potential solutions and identifying the characteristics of the equation based on the nature of its roots.
Delving into the Enigmatic World of Root Types
In the realm of mathematics, roots hold immense power, unlocking hidden truths within polynomial equations. Embark on this captivating journey as we delve into the diverse types of roots, exploring their unique characteristics and profound implications.
Unraveling the Mystery of Complex Roots
Venture into the uncharted waters of complex numbers, where roots take on a new dimension. Complex roots emerge in conjugate pairs, like inseparable twins. Each root is the mirror image of the other across the real-number axis. This mystical duality stems from the fundamental nature of polynomial equations, governed by the enigmatic Fundamental Theorem of Algebra. It whispers a profound truth: every polynomial equation, no matter how grand or humble, holds within it an equal number of roots.
Navigating the Rational Roots Maze
Step into the realm of rational roots, where order and logic reign supreme. The Rational Roots Theorem serves as our guiding beacon in this quest, revealing a method to uncover the rational roots that lurk within polynomial equations. These rational souls, clad in the cloak of fractions, often hold the key to unlocking the equation’s hidden secrets. Moreover, their presence, or lack thereof, whispers volumes about the equation’s temperament.
Discerning Real Roots: Conjugate and Beyond
In the tapestry of roots, real roots stand tall, either rational or irrational in nature. Some real roots form enchanting pairs, known as real conjugate roots, waltzing with perfect symmetry across the real-number axis. Their enchanting presence hints at the polynomial equation’s amiable nature, possessing no imaginary components.
Unveiling Complex Conjugate Roots
Now, let us venture into the ethereal realm of complex conjugate roots. These roots dance in pairs, sharing the same magnitude but opposite in sign. Like ethereal echoes, they mirror each other across the imaginary axis. Their harmonious presence reveals the polynomial equation’s deep connection with the complex plane, a realm where imaginary numbers dance and unravel secrets.
Synthesizing Root Types: A Path to Enlightenment
As we conclude our odyssey, we gather the threads of our knowledge, weaving a tapestry of root types. Each type – complex, real, rational, and conjugate – holds a unique allure, contributing to the intricate symphony of polynomial equations. Understanding their nature empowers us to unravel the equations’ hidden stories, revealing their true essence.
Locating Rational Roots
- Describe the Rational Roots Theorem and how it helps find rational roots of polynomial equations.
- Explain the significance of real and rational roots.
Locating Rational Roots: Unlocking the Secrets of Polynomial Equations
In the realm of mathematics, polynomial equations reign supreme, holding the key to solving complex problems. Understanding the types of roots these equations possess is crucial for unlocking their secrets. Among the various root types, rational roots hold a special significance.
Rational Roots Theorem: A Guiding Light
The Rational Roots Theorem is a powerful tool that empowers us to locate rational roots of a polynomial equation. It states that if a polynomial has rational coefficients, any rational root must be expressible as a fraction of the form p/q, where p is a factor of the constant term and q is a factor of the leading coefficient.
Finding Rational Roots: A Step-by-Step Approach
To find rational roots using the theorem, follow these steps:
- Identify potential rational roots: List all possible factors of the constant term and leading coefficient.
- Check for valid roots: For each potential root, divide the constant term by the numerator and the leading coefficient by the denominator. If the remainders are zero, then the root is valid.
Significance of Real and Rational Roots
Rational roots are of particular importance because they represent real roots. Real roots are values that lie on the real number line, making them easier to interpret and apply in real-world situations. Rational roots, being a subset of real roots, are especially valuable as they can be expressed in fractional form, providing a clear and concise representation.
The Rational Roots Theorem serves as a guiding light in the quest to locate rational roots of polynomial equations. By understanding this theorem and its implications, we gain a deeper insight into the nature of polynomials and their solutions. Moreover, identifying rational roots allows us to make informed decisions and solve complex problems with greater precision and confidence.
Real Roots: Conjugate and Non-Conjugate
- Define real roots and their classification as rational or irrational.
- Introduce the concept of real conjugate roots and their occurrence in pairs.
Real Roots: The Two Sides of the Root World
In the realm of mathematics, roots are like the keys that unlock the secrets of polynomial equations. And when it comes to real roots, we enter a captivating world of numbers that can be rational, irrational, conjugate, or even non-conjugate.
Defining Real Roots
Real roots are simply the roots of polynomial equations that produce real numbers. They can be either rational (can be expressed as a fraction of two integers) or irrational (cannot be expressed as a fraction). Irrational roots are like elusive shadows, forever lurking on the fringes of the rational world.
Conjugates: The Inseparable Root Twins
Among real roots, conjugate roots occupy a special place. They are pairs of roots that are mirror images of each other across the real number line. Conjugate roots always appear in duets, with one root being positive and the other negative.
The reason for this symmetry lies in the Fundamental Theorem of Algebra, which states that every polynomial equation with real coefficients has at least one complex root. And when a root is complex, its conjugate must also be a root, ensuring perfect balance and harmony within the polynomial equation.
Understanding different root types is not just an academic exercise. It’s a crucial step in solving polynomial equations. By recognizing the presence of rational, irrational, and conjugate roots, we can break down complex equations into simpler components, making them more manageable and revealing hidden insights into the equation’s behavior.
Complex Conjugate Roots: Unlocking the Secrets of Polynomial Equations
Imagine a world where numbers could exist beyond the realm of reality, where they dance with imaginary friends. Complex roots are the magical creatures that inhabit this enigmatic realm, and they come in pairs that are mirror images of each other – like eerie echoes from another dimension.
When a polynomial equation whispers its secrets, it often conceals complex conjugate roots within its enigmatic folds. These roots are like two sides of the same coin – they share the same numerical value but reside on opposite sides of the imaginary divide. They’re like ghostly twins, forever bound together in mathematical harmony.
The presence of complex conjugate roots has a profound impact on the nature of the polynomial equation. Just as a mirror reflects an object, these roots create a symmetry in the equation’s graph. The polynomial will dance around the x-axis, with its peaks and valleys mirroring each other with uncanny precision.
This symmetry reveals a hidden truth – the sum of complex conjugate roots is always a real number. It’s as if the equation seeks balance, finding solace in the harmony of its complex roots. Furthermore, the product of complex conjugate roots is invariably a positive real number. This unyielding positivity suggests that complex roots, despite their imaginary nature, bring a sense of order and stability to the equation.
The presence of complex conjugate roots is a testament to the intricate beauty of mathematics. They’re like the enigmatic guardians of polynomial equations, protecting the secrets of their graphs. By understanding the nature of complex conjugate roots, we unlock a deeper appreciation for the enchanting world of mathematics.
Types of Roots in Polynomial Equations
Embark on a Mathematical Journey
In the world of mathematics, polynomial equations hold a central place, describing countless phenomena in nature and our daily lives. Understanding the roots of these equations is paramount, as they provide crucial insights into the behavior and solutions of the equations. Join us as we delve into the fascinating realm of root types, exploring their diverse characteristics and their profound implications in solving polynomial equations.
Complex and Real Roots: A Balancing Act
Polynomial equations can have both complex and real roots. Complex roots occur in conjugate pairs, meaning they are mirror images of each other across the real axis. This phenomenon arises from the Fundamental Theorem of Algebra, which guarantees that every polynomial equation has at least one root.
Rational Roots: Uncovering the Hidden Treasures
Within the realm of real roots lies a special subset known as rational roots. These roots can be expressed as fractions with integer numerators and denominators. To uncover these hidden treasures, we invoke the Rational Roots Theorem. This theorem guides us in identifying potential rational roots by examining the coefficients of the polynomial equation.
Real Roots: Unveiling the Interplay
Real roots can be further classified into two types: conjugate and non-conjugate. Conjugate real roots occur in pairs with opposite signs, while non-conjugate real roots stand alone. The presence of conjugate real roots indicates that the polynomial equation has a quadratic factor, providing valuable insights into its structure.
Complex Conjugate Roots: A Symphony of Pairs
Complex conjugate roots manifest as pairs of roots that are mirror images of each other across the imaginary axis. They coexist in polynomial equations with real coefficients, introducing an element of symmetry and greatly influencing the nature of the equation.
Additional Insights: Harnessing Root Types
By grasping the complexities and interplay of root types, we gain a powerful tool for solving polynomial equations. Different root types dictate specific strategies and approaches, empowering us to unravel even the most intricate equations. This knowledge equips us to extract meaningful information from polynomial equations, paving the way for a deeper understanding of their mathematical essence and their applications in various fields.