Understanding The Integral Of Force: A Fundamental Concept In Motion And Energy Transformations

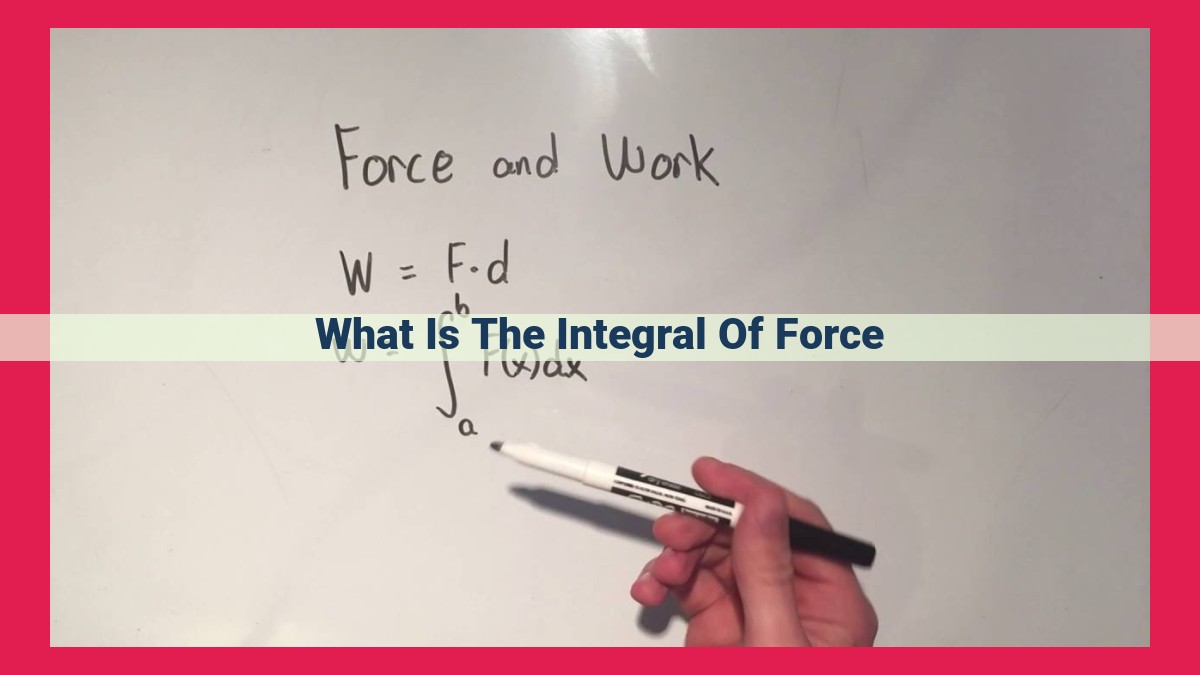
The integral of force over time is impulse, which is the change in momentum. Work, or energy transferred, is the integral of force over displacement. As force is related to momentum and energy changes, understanding its integral is fundamental to comprehending motion, collisions, and energy transformations.

Force and Its Mathematical Representation: Unraveling the Power to Move

In our everyday interactions, we encounter forces in countless ways, from the gentle push of a door to the powerful propulsion of a rocket soaring towards the stars. What is force, and how can we mathematically describe its effects on the world around us?

Force and Its Math: A Deeper Dive

Force, a fundamental concept in physics, represents an interaction that can cause a change in the motion of an object. It is a vector quantity, meaning that it has both a magnitude and a direction. The mathematical representation of force is given by the equation:

Force = mass × acceleration

Where:
– Force is measured in newtons (N)
– Mass is measured in kilograms (kg)
– Acceleration is measured in meters per second squared (m/s²)

This equation tells us that the force applied to an object is directly proportional to its inertia, or resistance to changes in its motion, and the rate at which its velocity is changing. In other words, the heavier an object is and the faster it is accelerating, the greater the force required to move it.

Understanding the Significance of Force

Comprehending force and its mathematical representation is crucial in various fields of science and engineering. It allows us to:

• Predict how objects will move under the influence of different forces.
• Design structures and machines that can withstand and utilize forces effectively.
• Explain a wide range of physical phenomena, from the motion of celestial bodies to the behavior of fluids.

Understanding Impulse and Work: Concepts Related to Force

Prologue:

In the realm of physics, force plays a central role in understanding the motion and interactions of objects. But force is not alone; it has close companions: impulse and work. These concepts are intricately connected, forming a web of principles that govern our physical world.

Impulse: Force’s Timed Interplay

Impulse is the product of force and the time it acts for. It is a vector quantity, meaning it has both magnitude and direction. Imagine pushing an object with a constant force for a few seconds. The impulse you impart is directly proportional to the force and the duration of your push.

Impulse’s significance lies in its ability to alter an object’s momentum. According to Newton’s second law, the rate of change of momentum is equal to the net force acting on an object. In other words, impulse is the “currency” of momentum changes. When an impulse is applied, it can change an object’s velocity or direction of motion.

Work: Force’s Displacement Partner

Work, on the other hand, is the product of force and the displacement of an object in the direction of the force. It is a scalar quantity, meaning it has only magnitude. Think of lifting a box against the force of gravity. The work you do is proportional to the force you apply and the vertical height through which you lift the box.

Work is crucial for understanding energy transformations. Energy is the ability to do work, and work can change the energy of an object. For example, when you lift a box, you transfer energy from your body to the box, increasing its potential energy.

Force, impulse, and work form an indispensable trio in the study of motion. Impulse quantifies the time-dependent nature of force, while work captures the energy transfer associated with force. Together, they provide a comprehensive framework for understanding the interactions and dynamics of objects in our physical world.

Momentum: Definition and Its Significance

In the realm of physics, momentum stands as a fundamental concept that encapsulates the essence of an object’s motion. It embodies the interplay between an object’s mass and its velocity, providing a quantitative measure of its inertia.

Momentum, denoted by the symbol p, is calculated as the product of an object’s mass m and its velocity v:

p = m * v

This mathematical expression unveils the profound relationship between an object’s inherent matter and its movement. An object with a greater mass possesses a correspondingly greater momentum, reflecting its resistance to changes in motion. Conversely, an object with a higher velocity exhibits a proportionally greater momentum, highlighting its tendency to maintain its state of motion.

The significance of momentum lies in its ability to describe the behavior of objects in collisions. Momentum is a conserved quantity, meaning that the total momentum of a closed system remains constant regardless of the internal interactions within the system. This principle provides a powerful tool for analyzing the dynamics of collisions, enabling physicists to predict the post-collision outcomes of objects.

By understanding momentum, scientists can gain invaluable insights into the motion of planets, the trajectories of projectiles, and the behavior of particles in subatomic interactions. It serves as a cornerstone for exploring the intricate workings of our physical world, from the macroscopic scale to the quantum realm.

Energy and Its Forms: Kinetic and Potential Energy

In the realm of physics, energy reigns supreme, driving the motion and interactions of matter. Two fundamental forms of energy, kinetic and potential, play a crucial role in shaping our universe.

Kinetic Energy: The Energy of Motion

Imagine a speeding car hurtling down the road. Its motion is a testament to its kinetic energy, the energy possessed by an object due to its movement. Kinetic energy is directly proportional to an object’s mass and the square of its velocity. The heavier an object is, or the faster it travels, the greater its kinetic energy.

Potential Energy: Stored Energy Waiting to Be Released

In contrast to kinetic energy, potential energy is stored energy awaiting release. It’s like a coiled spring, ready to unleash its power when the time is right. Potential energy is often associated with an object’s position or condition. For instance, a rock perched high atop a cliff possesses gravitational potential energy, the energy due to its position relative to the Earth’s gravitational field. The higher the rock, the greater its potential energy.

Types of Potential Energy

The world of potential energy is diverse, with different forms emerging depending on the situation.

• Gravitational potential energy: Exists due to an object’s position in a gravitational field.
• Elastic potential energy: Stored in stretched or compressed objects like springs or rubber bands.
• Chemical potential energy: Resides within chemical bonds, providing the energy for reactions.
• Electrical potential energy: Associated with the separation of electrical charges.

The Interplay of Kinetic and Potential Energy

Kinetic and potential energies are not isolated entities; they interact constantly, transforming from one form to another. Consider a roller coaster car climbing the first hill. As it ascends, its kinetic energy gradually converts into gravitational potential energy. At the highest point, all kinetic energy is lost, replaced by maximum potential energy. As the car plummets down the track, the stored potential energy is released, transforming back into kinetic energy, reaching its peak speed at the bottom of the hill.

Understanding kinetic and potential energy is essential for comprehending the dynamics of our world. From the flight of a bird to the workings of a hydroelectric plant, these energy forms drive countless phenomena. By grasping their principles, we unlock a deeper appreciation for the intricate workings of the physical universe.

The Enduring Principle: Conservation of Energy

In the realm of physics, the principle of conservation of energy emerges as an indispensable concept, guiding our understanding of the intricate tapestry of energy transformations. This principle asserts that within isolated systems, the total amount of energy remains constant, unwavering amidst myriad transitions. Energy, in its various forms, can neither be created from nothingness nor destroyed into oblivion; it is merely transformed into different incarnations.

Closed systems, such as isolated containers or planetary systems, serve as ideal paradigms for showcasing this principle. Within such systems, energy may metamorphose from one form to another, such as from chemical energy stored in batteries to electrical energy powering devices. Yet, the grand total of all energy within the system remains steadfast, impervious to manipulation.

This principle plays a pivotal role in comprehending the ceaseless flux of energy in our universe. It guides us in predicting and comprehending the behavior of physical systems, from the tiniest atoms to the grandeur of cosmic phenomena. The principle of conservation of energy provides a fundamental scaffolding upon which a countless array of scientific discoveries have been erected, shaping our understanding of the world around us.