Understanding Included Angles: From Basic Concepts To Advanced Applications
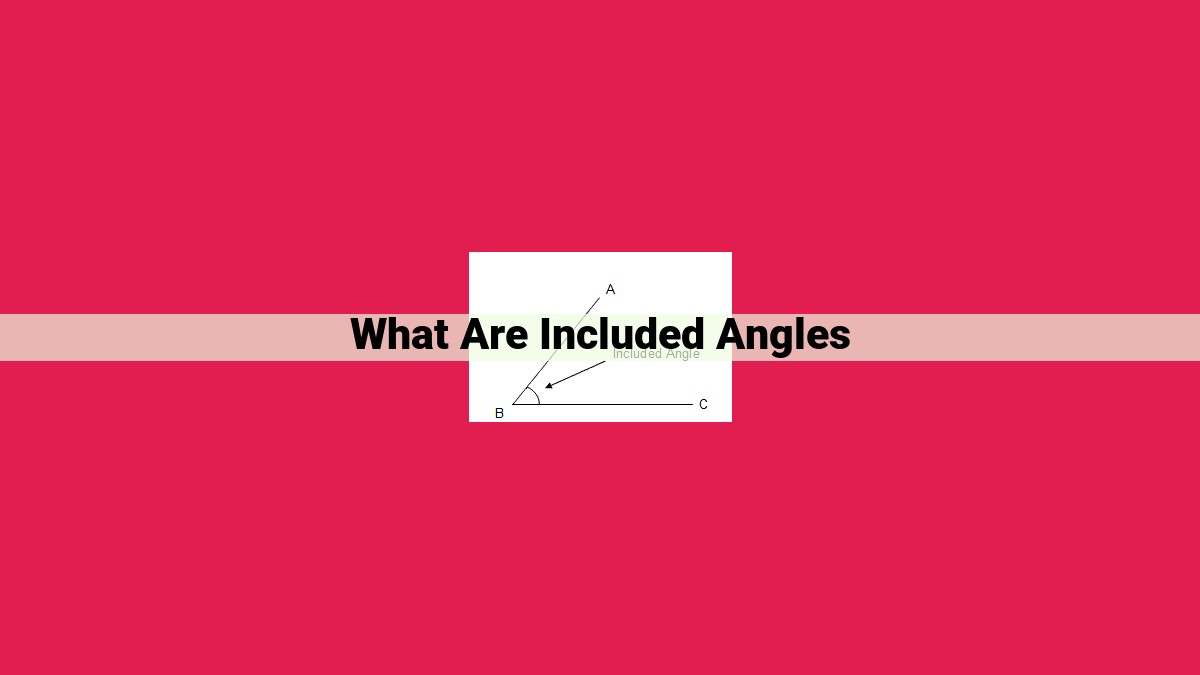
Included angles are formed when two lines intersect, with the vertex at the intersection point and the common side shared by both lines. Key concepts related to included angles include vertices, common sides, and common vertices. They can be classified as acute, obtuse, right, or reflex based on their measures. Included angles have relationships with supplementary and complementary angles, allowing the calculation of unknown angles. They find applications in geometry, such as determining interior angles of polygons and proving their properties. Advanced applications exist in trigonometry, physics, and engineering, where included angles are used for analyzing vectors, calculating forces, and solving various problems.
Definition of Included Angles
- Explain what included angles are and how they are formed when two lines intersect.
What are Included Angles?
Imagine two lines that intersect like roads crossing in the town. At the point where they meet, they form a “vertex,” like the town square. The included angles are the two angles that are formed on either side of the vertex, just like the roads going in opposite directions. These angles are like the angles you make when you spread your arms apart, with your body representing the vertex.
Each included angle is named by the three points that define it. For example, if the two lines are called line AB and line CD, the included angle would be called angle BCD.
Key Features of Included Angles
There are a few important concepts that go hand-in-hand with included angles:
- Adjacent angles: These are angles that share a common side and a vertex. In our town square example, the two included angles would be adjacent angles.
- Vertical angles: These are angles that are formed by two intersecting lines and are opposite each other. They’re like twins, always having the same measure.
- Supplementary angles: These are angles whose measures add up to 180 degrees. If you think of a straight line, the angles on either side are supplementary.
- Complementary angles: These are angles whose measures add up to 90 degrees. They’re like the angles you make when you bend your arm at a right angle.
Measuring and Classifying Included Angles
To measure an included angle, you can use a protractor, which is like a ruler for angles. Simply place the protractor on the vertex of the angle and align its zero mark with one of the lines. The number where the other line intersects the protractor is the measure of the angle.
Included angles can be classified based on their measures:
- Acute angles: These angles are less than 90 degrees, like the angle made by a pencil tip.
- Obtuse angles: These angles are between 90 and 180 degrees, like the angle made by the hour and minute hands on a clock at 3 o’clock.
- Right angles: These angles are exactly 90 degrees, like the angle made by a book lying flat on a table.
- Reflex angles: These angles are greater than 180 degrees, like the angle made by a boomerang.
Key Concepts Related to Included Angles
When two lines intersect, they form four angles, two of which are called included angles. Included angles share a common vertex and a common side.
Vertex: The vertex is the point where the two lines intersect and is the common point of both included angles.
Common Side: The common side is the line segment that is shared by both included angles.
Common Vertex: Both included angles share a common vertex.
Linear Pair:
When two included angles form a straight line, they are known as a linear pair. The sum of the angles in a linear pair is always 180 degrees.
Supplementary Angles:
Supplementary angles are two angles whose sum is 180 degrees. In the case of included angles, if the two included angles are supplementary, then they form a linear pair.
Complementary Angles:
Complementary angles are two angles whose sum is 90 degrees. Included angles can never be complementary unless they are both right angles.
Measuring and Classifying Included Angles
When two lines intersect, they form four angles around the point of intersection. The two angles opposite each other are called included angles. Measuring and classifying included angles are essential to understanding many concepts in geometry.
Measuring Included Angles:
One common tool for measuring included angles is a protractor. Place the protractor on the point of intersection with its center at the vertex. Align one edge of the protractor with one of the intersecting lines and read the measurement at the other intersecting line. The angle between the lines is the included angle.
Classifying Included Angles:
Included angles can be classified based on their measures:
- Acute angles: Less than 90 degrees (e.g., 30°, 60°)
- Right angles: Exactly 90 degrees
- Obtuse angles: Greater than 90 degrees but less than 180 degrees (e.g., 100°, 150°)
- Reflex angles: Greater than 180 degrees but less than 360 degrees (e.g., 210°, 300°)
Understanding how to measure and classify included angles is crucial for solving geometry problems involving angles and lines.
Relationships Between Included Angles
Understanding the Connection
Included angles, formed when two lines intersect, play a significant role in geometry and have intriguing relationships with other types of angles. Two such angles that share a special connection are supplementary angles and complementary angles.
Supplementary Angles: A Perfect Pair
Supplementary angles are two angles that add up to 180 degrees. When two included angles are supplementary, they form a straight line. This means that the sum of their measures equals the angle of a full circle. For instance, if one included angle measures 120 degrees, the other must measure 60 degrees to be supplementary.
Complementary Angles: A Harmonious Duo
On the other hand, complementary angles are two angles that add up to 90 degrees. They are often found adjacent to each other, forming a right angle. For example, if one included angle measures 30 degrees, its complementary angle measures 60 degrees, creating a right angle.
Using Relationships to Solve for Unknowns
These relationships between included angles, supplementary angles, and complementary angles provide powerful tools for solving geometry problems. By knowing the measure of one included angle, we can determine the measure of its supplementary or complementary angle.
If you have an included angle with a measure of x, then:
- Its supplementary angle measures 180 – x
- Its complementary angle measures 90 – x
By using these formulas, you can easily find the unknown angles and deepen your understanding of geometry.
Applications of Included Angles in Geometry
Unlocking the Secrets of Shapes
In the realm of geometry, included angles play a pivotal role in deciphering the intricate relationships between lines and shapes. They provide a key to uncovering the hidden secrets of polygons, triangles, and quadrilaterals, revealing their angles and properties.
Finding Interior Angles in Polygons
Imagine a bustling town where streets intersect at every corner. Each intersection forms two included angles, which we can use to unravel the sum of the interior angles of any polygon. For a triangle, the sum of the included angles is always 180 degrees. This concept extends to quadrilaterals and other polygons, providing a valuable tool for solving geometry problems.
Determining Shape Properties
Included angles also hold the power to determine the very nature of shapes. Two lines that intersect at a right angle form perpendicular lines, while parallel lines maintain a constant included angle of 180 degrees. By measuring and analyzing included angles, we can readily establish the properties of shapes, unraveling their parallel, perpendicular, or other characteristics.
Unifying Supplementary and Complementary Angles
The world of geometry is filled with interconnected concepts, and the relationship between included angles and supplementary and complementary angles is no exception. Supplementary angles add up to 180 degrees, while complementary angles sum up to 90 degrees. Understanding this interplay empowers us to find the measures of unknown included angles with ease.
Included angles are indispensable tools in the geometer’s toolbox, unlocking the mysteries of shapes and revealing their hidden relationships. By understanding their role in polygons, properties, and the interplay of angles, we open the door to a deeper appreciation of the intricate tapestry that is geometry.
Advanced Applications of Included Angles
Beyond their use in basic geometry, included angles play crucial roles in more complex mathematical disciplines and real-world applications. Let’s delve into some of these advanced scenarios:
Trigonometry and Vector Analysis
In trigonometry, included angles are pivotal for understanding the relationships between sides and angles in triangles. The Law of Sines and Law of Cosines use included angles to determine unknown side lengths and angles. Included angles also find use in vector analysis, where they help calculate the angle between vectors and determine the direction of forces.
Physics and Engineering
In physics and engineering, included angles are vital in analyzing force vectors and resolving forces. The equilibrium of forces often depends on the angles formed between these vectors. Included angles are also used in projectile motion to calculate the trajectory and range of objects.
Advanced Geometry
In advanced geometry, included angles are crucial for understanding properties of complex shapes. For instance, the interior angle sum of a polygon is determined by the number of sides and the included angles. Included angles also help classify conic sections, such as ellipses, hyperbolas, and parabolas.
While included angles may seem like a simple concept at first, their applications extend far beyond basic geometry. From trigonometry and vector analysis to physics and advanced geometry, included angles play a fundamental role in solving a wide range of mathematical and real-world problems. Understanding their properties and relationships is essential for anyone who wants to delve deeper into these complex fields.