Seo-Optimized Title: Understanding Division Expressions: A Comprehensive Guide
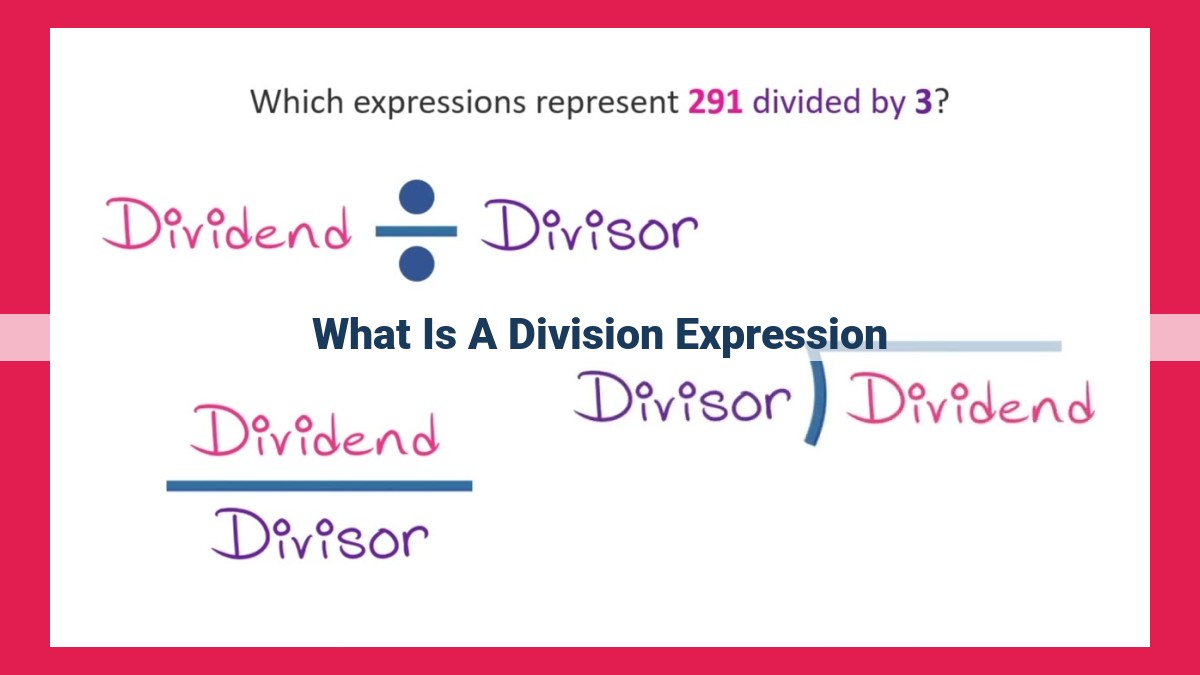
What is a Division Expression?
A division expression is a mathematical equation that indicates the process of dividing one number (the dividend) by another number (the divisor). The result of the division can be a whole number, a fraction, or a decimal. The division symbol is represented by the ÷ symbol, the slash (/) symbol, or the fraction bar. Division expresses the operation of splitting a quantity into equal parts or determining how many times a smaller quantity (the divisor) is contained within a larger quantity (the dividend).
Division Expressions: The Power of Sharing
In the realm of mathematics, we encounter a fundamental concept known as the division expression. Imagine you have a bag of treats, and you need to share them equally among your friends. How do you determine how many treats each friend receives? Enter the division expression, a mathematical tool that helps us divide one number (dividend) by another (divisor) to find the equal share (quotient).
Division expressions are represented by the division symbol (÷) or the fraction notation (dividend/divisor). The dividend is the number being divided, while the divisor is the number by which we’re dividing the dividend. For instance, in the expression “10 ÷ 2,” 10 is the dividend, and 2 is the divisor.
The purpose of a division expression is to find the number of times the divisor can be subtracted from the dividend without leaving a remainder. For example, if you have 10 treats and need to share them equally among 2 friends, you would divide 10 by 2, which gives you 5 treats per friend. In this case, the divisor (2) fits evenly into the dividend (10), resulting in a quotient of 5 with no remainder.
Explanation of the division symbol
Headline: Demystifying Division Expressions: Understanding the Magic of Dividing Numbers
Have you ever wondered how we make sense of the world around us? Mathematics plays a crucial role, and one of its most fundamental operations is division. Division expressions are powerful tools that help us understand concepts like sharing, comparison, and distribution. This blog post will embark on a journey to unravel the mysteries of division expressions, making this mathematical concept more approachable than ever before.
What is a Division Expression?
Imagine you have a delicious pizza and want to share it equally with your friends. How do you determine how much each person gets? That’s where division comes in! A division expression is a mathematical phrase that represents this action. It consists of three key parts:
- Dividend: The total amount to be divided (the pizza)
- Divisor: The number that divides the dividend (the number of friends)
- Quotient: The resulting quantity (the equal slices)
Explanation of the Division Symbol
The division symbol, commonly represented by a forward slash (/), is the gatekeeper of division expressions. It acts as a mathematical bridge, signifying that the dividend is being divided by the divisor. Think of it as a magical potion that transforms the dividend into equal parts.
Components of Division Expressions
Just as a car has different parts that make it function, division expressions consist of several components:
Dividend – The Number Being Divided
The dividend, like a bountiful treasure chest, holds the total amount that needs to be distributed or compared.
Divisor – The Number by Which the Dividend is Divided
The divisor, akin to a master chef, wields its power to slice the dividend into equal portions or measure its relative size.
Remainder – The Leftover Number After Division
In some cases, the division process may not result in a perfect split. The remainder, like a crumb of bread, represents the quantity left over after the dividend is divided by the divisor.
Related Concepts
Division expressions are not isolated concepts; they intertwine with other mathematical ideas, creating a harmonious equation of knowledge.
Factors – Numbers that Evenly Divide Another Number
Factors are like best friends who share a special bond with the dividend. They can divide it evenly without leaving a remainder, making the division process a breeze.
Numerator and Denominator – In Fractions, Dividend and Divisor, Respectively
When division expresses itself as fractions, the dividend transforms into the numerator, and the divisor becomes the denominator. They work together to represent the quotient as a fraction.
Equations and Inequalities – Using Division Expressions to Represent Equality or Inequality
Division expressions can play a starring role in equations and inequalities. They help us understand relationships between different quantities, determining whether they are equal or not.
Mathematical Formulas – Division Expressions in Mathematical Formulas
Division expressions often find their place in mathematical formulas, acting as essential ingredients in complex calculations. They bring balance and precision to the world of math.
Applications of Division Expressions
Division expressions are not just mathematical curiosities; they have real-world applications in our daily lives:
Distributing Resources Equally
From dividing candy among friends to allocating materials in a factory, division expressions ensure fair and equal distribution.
Comparing Ratios and Proportions
Division expressions allow us to compare the relative proportions of different quantities, helping us make informed decisions.
Solving Problems Involving Rates and Percentages
Division expressions become the key to unlocking problems that involve rates and percentages, enabling us to calculate speeds, discounts, and much more.
By understanding division expressions and their components, you have gained a powerful tool to navigate the mathematical world. Whether it’s sharing resources, comparing quantities, or solving complex equations, division expressions will guide you through the labyrinth of numbers, making your journey more meaningful and enjoyable.
Division Expressions: A Comprehensive Guide
What is a Division Expression?
In the realm of mathematics, a division expression serves as a powerful tool for understanding the concept of dividing one number by another. It’s like a mathematical recipe, where we take a number, known as the dividend, and split it equally into shares based on the divisor. The division symbol, represented by the slash (/) or the colon (:), is the culinary knife that carves out these equal parts.
Dividend: The Number to be Divided
The dividend, the protagonist of our division expression, is the number that we aim to dissect. It represents the whole that needs to be distributed or split into equal parts. For instance, if we want to divide 12 apples among 4 friends, 12 would be our dividend.
Examples:
- 10 / 2
- 45 : 5
- 1234 / 10
Key Takeaway:
A division expression is a mathematical operation that divides one number (dividend) by another (divisor) to determine the number of equal parts and any remaining amount (remainder).
Division Expressions: Unlocking the Mystery of Distributing and Dividing
What is a Division Expression?
Division expressions are mathematical equations that represent the distribution or separation of a quantity into equal parts. They involve two main components: the dividend (the number being distributed) and the divisor (the number by which we distribute).
The Divisor: The Key to Equal Division
Imagine you have a delicious pizza to share with a group of friends. The divisor in this case is the number of friends you have. The goal is to cut the pizza into equal slices so that each friend gets the same amount. The divisor helps us determine the size of each slice and ensures that everyone gets a fair share.
Dividend and Divisor: A Symbiotic Relationship
The dividend and divisor work together to determine the outcome of the division expression. The larger the divisor, the smaller the slices (or the quotient). Conversely, a smaller divisor results in larger slices. This relationship highlights the importance of choosing the divisor carefully to achieve the desired distribution or comparison.
Beyond Pizza: Applications in Real Life
Division expressions extend far beyond distributing pizza. They have numerous applications in our daily lives, such as:
- Distributing Resources: Division helps us distribute resources fairly, such as allocating funds among different departments or splitting chores among family members.
- Comparing Ratios: Division allows us to compare different ratios and proportions, such as comparing the speed of two vehicles or the concentration of two solutions.
- Solving Practical Problems: Division is essential for solving problems involving rates, percentages, and other numerical relationships.
In summary, division expressions are a fundamental tool for distributing and dividing quantities. By understanding the role of the divisor, we can effectively use these expressions to solve problems and make fair distributions in various real-life scenarios.
Delving into the Remainder: The Unsung Hero of Division
In the realm of mathematics, division expressions reign supreme, providing us with an invaluable tool for understanding the relationships between numbers. At the heart of these expressions lies the concept of a remainder—a crucial element that often goes unnoticed.
Imagine a scenario where you have a delicious pizza to share among your friends. You divide the pizza into equal slices, ensuring fairness and harmony. However, there might be a pesky slice left over—the remainder. This leftover slice represents the amount that cannot be evenly divided among the intended recipients.
In a division expression, the dividend is the pizza you’re sharing, the divisor is the number of friends you’re sharing it with, and the remainder is the slice that’s left over. This leftover slice plays a significant role in determining the result of the division and can provide insights into the relationship between the dividend and divisor.
The remainder serves as a testament to the fact that division is not always a clean-cut process. It reminds us that there may be instances where we cannot evenly distribute a quantity, leaving us with a fractional or decimal result. This leftover portion can be crucial in certain applications, such as calculating the area of a circle or determining the percentage of tax on a particular amount.
In conclusion, the remainder, often overlooked in the grand scheme of division expressions, holds immense importance. It signifies the unavoidable reality that division may not always yield a perfect outcome, and it provides valuable insights into the nature of the numbers we’re dealing with. Embracing the role of the remainder can empower us to wield division expressions with greater precision and understanding.
Division Expressions: A Mathematical Concept with Real-World Applications
Division expressions play a crucial role in our mathematical understanding and everyday lives. They help us distribute resources fairly, compare ratios and proportions, and solve problems involving rates and percentages.
Components of Division Expressions
A division expression consists of three key components:
- Dividend: The number being divided.
- Divisor: The number by which the dividend is divided.
- Remainder: The leftover number after division.
Factors: A Special Case
Factors are numbers that evenly divide another number. For instance, the factors of 12 are 1, 2, 3, 4, 6, and 12. These factors can be used to simplify division expressions and make calculations easier.
Related Concepts and Applications
Division expressions are closely related to other mathematical concepts:
- Fractions: The dividend and divisor in a fraction represent the numerator and denominator, respectively.
- Equations and Inequalities: Division expressions can be used to express equality or inequality.
- Mathematical Formulas: Division expressions are commonly found in mathematical formulas and equations.
Practical Applications
Division expressions have numerous practical applications in everyday situations:
- Distributing Resources Equally: When distributing resources among a group of people, we can use division to ensure fair and equal distribution.
- Comparing Ratios and Proportions: Division expressions help us compare ratios and proportions, such as when comparing the density of two substances or the speed of two objects.
- Solving Problems Involving Rates and Percentages: Division is essential for solving problems involving rates, such as speed or flow rates, and percentages, such as discounts or sales tax calculations.
Division expressions are a fundamental mathematical concept with a wide range of applications in both theoretical mathematics and practical life. Understanding how to work with division expressions is essential for problem-solving, logical reasoning, and navigating everyday situations.
Division Expressions: Unveiling the Mathematical Treasure
What is a Division Expression?
A division expression is a mathematical equation that represents the division of one number by another. It is denoted by the division symbol, “÷” or “/”, and serves to find the quotient, the result of the division.
Components of Division Expressions
The dividend, the number being divided, is like the “treat” to be shared among friends. The divisor, the number by which the dividend is divided, acts as the “friends” receiving the treat. Division results in the quotient, the number that indicates how many equal shares each “friend” gets. Sometimes, there might be a remainder, the leftover “treat” that cannot be equally distributed.
Exploring Related Concepts
Numerator and Denominator:
In fractions, the dividend is the numerator, residing up top, and the divisor is the denominator, seated below. This relationship is akin to a recipe, where the numerator represents the amount of ingredient needed, and the denominator indicates the number of portions.
Other related concepts include:
- Factors: Numbers that divide another number evenly, like the “friends” who can evenly share a treat.
- Equations and Inequalities: Division expressions can represent equality (“=”) or inequality (“≠”), such as “5 ÷ 2 = 2.5”.
- Mathematical Formulas: Division is often found in formulas, like “v = d/t”, where “v” is speed, “d” is distance, and “t” is time.
Applications in Real Life
Division expressions play a vital role in everyday life:
- Distributing Resources: To ensure fair distribution of goods, like dividing a pizza among friends.
- Comparing Ratios: To compare different quantities, such as the ratio of boys to girls in a class.
- Solving Rates and Percentages: For calculating rates, like speed or interest rates, and percentages, such as discounts or taxes.
By understanding division expressions, we unlock a powerful mathematical tool that helps us navigate and solve countless real-world problems.
Using Division Expressions in Equations and Inequalities
Imagine a baker who needs to divide a batch of dough equally among 12 muffin cups. This scenario can be represented by the division expression:
Number of dough / Number of cups = Number of dough per cup
Translating this into an equation, we get:
**Dough / Cups = Dough Per Cup**
The equation states that the number of dough units divided by the number of cups equals the number of dough units in each cup. This equation can be used to determine the equal distribution of dough.
Similarly, division expressions can be used in inequalities to compare the distribution of quantities. For instance, if the baker wants to ensure that each muffin cup receives at least 2 units of dough, the inequality would be:
**Dough / Cups ≥ 2**
This inequality guarantees that the number of dough units per cup is greater than or equal to 2, ensuring fair distribution.
Division expressions in equations and inequalities provide a concise and clear way to represent the relationships between quantities. They allow us to express the division of one quantity by another and to compare the results of such divisions. By understanding these concepts, we can effectively solve problems involving fair distribution, comparisons, and other mathematical operations.
Division Expressions: Unveiling the Mathematical Secrets
In the realm of mathematics, division expressions hold a pivotal role, enabling us to dissect numerical problems and unravel their hidden solutions. These expressions, denoted by the enigmatic symbol /, possess a profound significance in our understanding of quantitative relationships.
Components of a Division Expression: A Mathematical Trio
Every division expression comprises an intricate trio of elements:
- Dividend: The number that yields the answer when divided.
- Divisor: The number by which the dividend is divided.
- Remainder: The residual amount remaining after division.
These three components dance together, creating a harmonious equilibrium within the expression, much like the three parts of a delicate puzzle.
Mathematical Formulas: Division Expressions Unmasked
Division expressions often masquerade within the intricate tapestry of mathematical formulas. They serve as the invisible threads that bind together diverse variables and constants, revealing the underlying logic behind complex calculations.
For instance, the formula for calculating the average speed (v) of an object reads as follows: v = d/t. Here, the division expression d/t reveals that the average speed is determined by dividing the distance (d) by the time (t) taken to cover that distance. This simple expression encapsulates a profound relationship between speed, distance, and time, a testament to the power of division in unveiling mathematical truths.
By dissecting division expressions, we uncover their hidden potential and gain a deeper appreciation for the intricate language of mathematics. So, let us embark on this journey of exploration, unraveling the secrets of division expressions, one step at a time.
Division Expressions: A Guide to the Basics
Division expressions are a fundamental concept in mathematics, used to represent the process of dividing one number by another. In this blog post, we’ll dive into the world of division expressions, exploring their components, related concepts, and practical applications.
Components of a Division Expression
A division expression consists of three main parts:
- Dividend: The number being divided.
- Divisor: The number by which the dividend is divided.
- Remainder: The number left over after division (not always present).
Related Concepts
Division expressions are closely linked to other mathematical concepts, including:
- Factors: Numbers that evenly divide another number, without leaving a remainder.
- Numerator and Denominator: In fractions, the dividend is the numerator and the divisor is the denominator.
- Equations and Inequalities: Division expressions can be used to represent equality or inequality between two expressions.
- Mathematical Formulas: Division expressions often appear in mathematical formulas to represent various operations.
Applications of Division Expressions
Division expressions have numerous practical applications in everyday life, such as:
- Distributing resources equally: Division is used to ensure that resources, such as food or materials, are distributed fairly among individuals or groups.
For example, if a pizza is to be shared equally among 8 friends, we can use division to find out how many slices each person gets:
Pizza slices = total slices / number of people
= 12 slices / 8 people
= 1.5 slices per person
By dividing the total number of slices by the number of people, we can determine the equal distribution.
- Comparing ratios and proportions: Division helps us compare ratios and proportions by determining the relative sizes of two quantities.
For example, if a recipe calls for 3 cups of flour to 2 cups of sugar, we can use division to find the ratio of flour to sugar:
Flour : Sugar
= 3 cups / 2 cups
= 3 : 2
The ratio 3:2 indicates that for every 3 cups of flour, we need 2 cups of sugar.
- Solving problems involving rates and percentages: Division is essential for solving problems involving rates and percentages.
For example, if a car travels 240 miles in 4 hours, we can use division to find its average speed:
Average speed = distance traveled / time taken
= 240 miles / 4 hours
= 60 miles per hour
By dividing the distance traveled by the time taken, we can calculate the car’s average speed.
Division Expressions: Beyond Arithmetic
What is a Division Expression?
Division expressions help us understand how many times one number fits inside another. Imagine sharing a pizza among friends. The number of pizzas being shared is the dividend, while the number of friends is the divisor. The division expression tells us how many slices each person gets.
Components of Division Expressions
- Dividend: The number being shared
- Divisor: The number by which we divide
- Remainder: Any leftover after division
Related Concepts
Division expressions connect to other mathematical ideas:
- Factors: Numbers we can multiply to get another
- Numerator and Denominator: In fractions, the dividend and divisor
- Equations and Inequalities: Expressing equality or inequality using division
Applications of Division Expressions
Division is not just about sharing pizza. It has real-world applications:
- Distributing Resources: Dividing supplies evenly among participants
- Comparing Ratios and Proportions: Determining if two quantities are in proportion
- Solving Problems: Calculating rates, percentages, and more
Comparing Ratios and Proportions
Ratios compare the size of two quantities, while proportions show equality between two ratios. For example:
- Ratio: If you have 3 red apples and 2 green apples, the ratio of red apples to green apples is 3:2.
- Proportion: If you have 6 red apples and 4 green apples, the proportion is 3:2, indicating the same ratio as before.
By using division expressions, we can calculate the ratios and proportions that describe our world. From sharing resources to understanding real-world relationships, division expressions are a versatile tool in our mathematical toolbox.
Division Expressions: A Comprehensive Guide to Understanding and Solving Problems
Division is a fundamental operation in mathematics that involves splitting a quantity into equal parts or comparing two quantities by dividing one by the other. In this blog post, we will delve into the world of division expressions, exploring their definition, components, and various applications.
Understanding Division Expressions
A division expression is a mathematical phrase that represents the operation of division. It consists of two numbers being divided by a division symbol. The number being divided is called the dividend, and the number dividing it is called the divisor. The result of the division is known as the quotient.
Components of Division Expressions
Every division expression has three main components:
- Dividend: The number being divided.
- Divisor: The number by which the dividend is divided.
- Remainder: The leftover number after division that cannot be further divided evenly by the divisor.
Related Concepts
Division expressions are closely related to several other mathematical concepts:
- Factors: Numbers that divide evenly into another number.
- Numerator and Denominator: In fractions, the dividend and divisor are referred to as the numerator and denominator, respectively.
- Equations and Inequalities: Division expressions can be used to represent equality or inequality in mathematical statements.
- Mathematical Formulas: Division expressions are often used in mathematical formulas to represent relationships between variables.
Applications of Division Expressions
Division expressions have a wide range of practical applications in various fields, including:
- Distributing resources equally: Dividing a quantity among a group of people or objects ensures fair distribution.
- Comparing ratios and proportions: By dividing two quantities, we can determine their relative sizes and make comparisons.
- Solving problems involving rates and percentages: Division expressions play a crucial role in solving problems related to speed, time, distances, and percentages.
For example, if a car travels 180 miles in 3 hours, the average speed can be calculated by dividing the distance (180 miles) by the time (3 hours):
Average speed = Distance / Time
Average speed = 180 miles / 3 hours
Average speed = 60 miles per hour
By understanding and applying division expressions effectively, we can solve a wide range of mathematical problems and gain a deeper understanding of the world around us.