Understand The Integrand: Key To Unlocking Integration
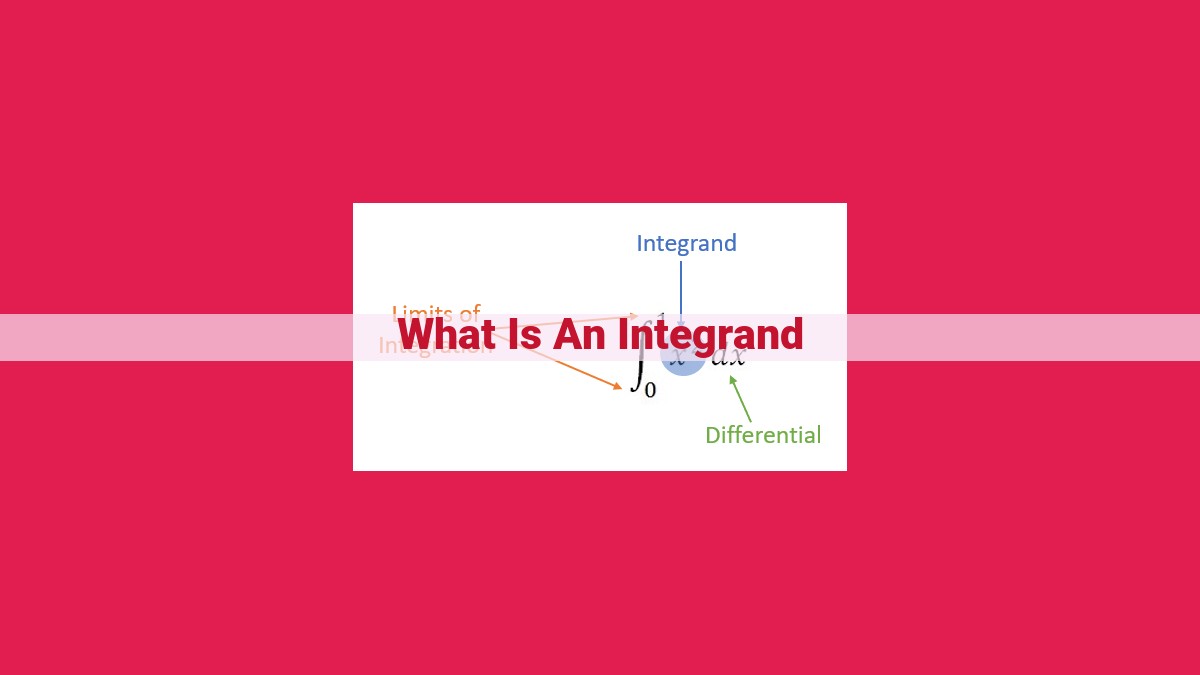
An integrand is the mathematical expression that is being integrated. It can be a variable, function, or complex expression. Integration is the process of finding the area under the curve represented by the integrand. The integral of an integrand is a family of antiderivatives, which are functions whose derivatives are equal to the integrand. The definite integral, which is a specific numerical value, represents the area under the curve over a specific interval. The integrand is a crucial element in integration and has applications in various real-world scenarios.
What is an Integrand?
- Definition and description of an integrand as the mathematical expression being integrated.
What is an Integrand?
In the captivating world of mathematics, the concept of integration plays a pivotal role. At the heart of integration lies the enigmatic entity known as the integrand_a mathematical expression that undergoes a fascinating transformation to unveil its secrets.
Delving into the Integrand
The integrand, by its very essence, embodies the core of integration. It represents the mathematical building block upon which the process of integration rests. Envision the integrand as the protagonist of an intricate puzzle, embodying the essence of the solution that lies ahead.
Unraveling the Nature of the Integrand
The integrand can take on various guises, each possessing its own unique characteristics. Sometimes, it manifests as a simple variable, a single entity that stands alone. In other instances, it transforms into a function, a dynamic tapestry of values woven together by a governing rule. The integrand can even don the mantle of a complex expression, a symphony of mathematical symbols intertwined in an intricate dance.
The Integral: A Tale of Unfolding the Integrand
The integral, the culmination of the integration process, emerges as the integral of the integrand. This numerical treasure is likened to the area beneath the curve that the integrand so gracefully traces. Imagine the integrand as a landscape, a rolling terrain of values. The integral, in this analogy, captures the essence of this landscape, quantifying its vastness.
Integration: The Symphony of the Integrand
Integration, a mathematical masterpiece, revolves around the intricate relationship between the integrand and its integral. Just as a tapestry is woven thread by thread, the integral gradually unfolds, unraveling the secrets embedded within the integrand. The process of integration acts as a guiding force, leading us from the enigmatic integrand to the enlightening integral.
The Nature of the Integrand: Unveiling the Essence of Integration
In the realm of calculus, the integrand stands as the heart and soul of integration, the process of finding the area under a curve. This enigmatic mathematical expression can take various forms, each holding its own significance and complexities.
An integrand can be a variable, such as x, representing a function’s rate of change. It can also manifest as a function, such as sin(x), describing a specific curve’s behavior. Moreover, the integrand can be a complex expression, involving a combination of variables, functions, and operators.
Consider the function y = x^2. When we integrate this function, its integrand is x^2. This expression captures the essence of the curve, telling us how the curve’s height varies with respect to x.
The nature of the integrand influences the complexity and techniques used in integration. For instance, integrating a polynomial integrand, such as x^2, is relatively straightforward. However, integrating a more complex integrand, such as e^(x^2), requires specialized techniques like u-substitution.
Understanding the nature of the integrand is crucial for successful integration. It guides us in selecting the appropriate integration method and helps us comprehend the significance of the result. Whether it’s a simple variable, a complex function, or a combination thereof, the integrand serves as the foundation for unlocking the secrets of integration.
The Integral of an Integrand: Uncovering the Essence of Integration
In the realm of mathematics, integration unfolds as a captivating dance between two mathematical entities: the integrand and its integral. The integrand, often denoted by f(x), stands as a mathematical expression that holds the key to unlocking the elusive area under the curve, a fundamental concept in calculus.
When we integrate an integrand, we embark on a journey to unveil the integral, a function represented by ∫f(x)dx. This integral embodies the area bounded above by the graph of f(x) and below by the x-axis. The boundaries of this area define the limits of integration, which determine the specific segment of the curve where the area is calculated.
The integral serves as a powerful tool that enables us to quantify the net area, whether positive or negative, enclosed by the curve over the given interval. A positive net area represents the actual area beneath the curve, while a negative net area indicates the area lying below the x-axis but above the curve.
The integral plays a pivotal role in various scientific disciplines and real-world applications. It finds its use in calculating volumes, surface areas, work, and many other physical quantities. By delving into the integral of an integrand, we gain a deeper understanding of the nature of functions and their geometric interpretations, opening up a myriad of possibilities in mathematical exploration and practical problem-solving.
Integration and the Integrand: Unraveling the Essence of Calculus
Integration: A Journey to Discover Underlying Areas
In the realm of calculus, integration stands tall as a fundamental operation that unravels the mystery of finding areas under curves. At the heart of this intricate process lies the integrand, a curious mathematical expression that whispers the secrets of the area it holds.
Imagine a curve, a playful dance of numbers, etched onto a graph. Beneath this curve lies a hidden treasure, an area that integration seeks to uncover. This is where the integrand steps onto the stage, its enigmatic presence promising to reveal the area under the curve.
Embracing the Integrand: A Variable, Function, or Complex Companion
The integrand, a versatile entity, can take on various forms. It may manifest as a humble variable, a _function brimming with equations, or even a _complex expression teeming with mathematical intricacies. Regardless of its guise, the integrand holds the key to unlocking the area beneath the curve.
Integration and the Integrand: A Symbiotic Dance
Integration, the masterful artist, transforms the integrand into a work of mathematical beauty – the integral. This integral, a symphony of numbers, represents the _area under the curve the integrand so eloquently described. The integrand and the integral, two sides of the same mathematical coin, engage in a harmonious dance, each revealing the secrets of the other.
Indefinite Integral: A Family of Possible Solutions
In the world of mathematics, indefinite integrals are like enigmatic treasure maps, leading us to a family of antiderivatives that hold the key to unlocking the unknown. When we encounter an integrand, the mathematical expression we seek to conquer, we embark on a quest to find its indefinite integral.
Unlike definite integrals, which yield a single numerical value, indefinite integrals present us with a wider vista of possibilities. Each antiderivative represents a different path to the same destination, like parallel trails leading to the summit of a mountain. And to navigate this landscape, we wield the power of integration constants.
These constants, denoted by symbols like C, serve as adjustable dials that allow us to shift our solutions up or down the vertical axis. They represent the freedom to explore different branches of the antiderivative family, each corresponding to a unique curve.
In essence, the indefinite integral is not a solitary solution but a constellation of potential solutions, a kaleidoscope of curves that share a common trajectory. It embodies the notion that in mathematics, as in life, there is often not just one right answer, but a spectrum of possibilities waiting to be discovered.
Definite Integral: Unveiling the Fixed Value of Area Under the Curve
In the vast realm of calculus, integrals hold a pivotal position, and at the heart of integration lies the integrand. An integrand, as its name suggests, is the mathematical expression that undergoes integration. But what exactly is its significance in the world of definite integrals?
A definite integral is no mere abstract concept; it embodies a fixed numerical value that quantifies the area under the curve of the integrand over a specific interval. This interval is precisely defined by two points, often labeled as “a” and “b.”
Imagine a graph where the integrand’s function is plotted. The definite integral calculates the net area between the curve and the horizontal axis, bounded by the specified interval. This area can be positive, negative, or even zero, depending on the function’s behavior within the interval.
The symbol for a definite integral is ∫, placed before the integrand. The interval is denoted as [a, b], indicating the starting and ending points of the integration. The definite integral is expressed as:
∫[a, b] f(x) dx
where f(x) represents the integrand.
In essence, the definite integral provides a precise measurement of the area under the curve, offering valuable insights into the function’s behavior within a specific range. Its applications extend far beyond theoretical mathematics, finding practical uses in fields such as physics, engineering, and economics.
Antiderivatives and the Integrand: Unlocking the Secrets of Integration
In the realm of calculus, the concept of an antiderivative plays a pivotal role in understanding integration, the process of finding the area under a curve. An antiderivative is essentially the inverse operation of the derivative, and its relationship with the integrand, the mathematical expression being integrated, is crucial for comprehending the essence of integration.
An antiderivative, often denoted by the symbol F(x), represents a function whose derivative is equal to the original integrand, f(x). In other words, it is a function that undoes the differentiation process. Consider the example of the integrand f(x) = 2x. Its antiderivative is F(x) = x^2 + C, where C is an arbitrary constant. Taking the derivative of F(x), we get F'(x) = 2x, which is the original integrand.
The significance of antiderivatives lies in their ability to provide a family of functions that share the same derivative. The constant C in the antiderivative represents this family, allowing for the exploration of all possible functions that satisfy the given derivative. In the case of f(x) = 2x, the family of antiderivatives is F(x) = x^2 + C, where C can take on any real value.
The relationship between the integrand and its antiderivative is fundamental to understanding integration. Integration is essentially the process of finding the antiderivative of an integrand, and the resulting function represents the area under the curve defined by the integrand. For example, if we want to find the area under the curve of f(x) = 2x over the interval [0, 1], we would first find its antiderivative, F(x) = x^2 + C. Then, we would evaluate F(x) at the endpoints of the interval, F(1) – F(0), to obtain the area as 1.
In conclusion, antiderivatives are the key to unlocking the secrets of integration. Their relationship with the integrand enables us to find the area under curves and explore the family of functions that share the same derivative. Understanding this concept is essential for mastering the art of integration and applying it to real-world problems.