Ultimate Guide To Constant Rate Of Change: Calculate Slope For Linear Equations
Best Blog Post Outline
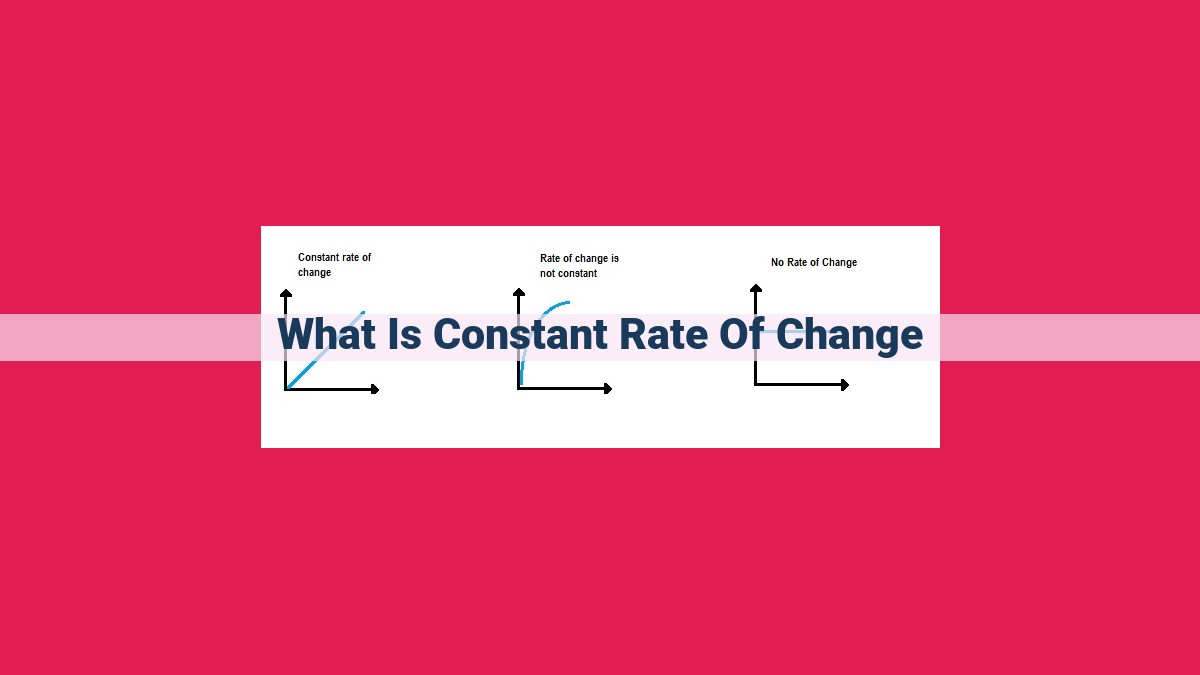
Introduction to Constant Rate of Change
Constant rate of change is a measure of how much a linear equation’s output (y-value) changes for each unit increase in input (x-value). It is represented by the slope of the line, which is calculated by dividing the vertical change (rise) by the horizontal change (run). The constant rate of change determines the steepness of a line and is essential for understanding linear relationships in various fields.
Understanding Constant Rate of Change: The Heartbeat of Linear Equations
Imagine you’re designing a new rollercoaster. The thrill comes from its ups and downs, its variable speeds and angles of descent. But amidst the chaotic excitement, there’s a hidden order: the constant rate of change.
In mathematics, constant rate of change describes the consistent change in a variable as another variable changes. Like the rollercoaster’s steady acceleration, it’s a measure of the linear relationship between two variables. In linear equations, this rate of change forms the backbone of our understanding.
Significance in Linear Equations
Linear equations are like blueprints for lines on a graph. The constant rate of change determines the slope of the line, which tells us how steep or inclined the line is. A positive rate of change indicates a line that climbs upward, while a negative rate of change shows a line that descends.
Understanding constant rate of change allows us to:
- Predict the value of a dependent variable (y) based on the independent variable (x)
- Determine the intercepts where the line crosses the x- and y-axes
- Write the equation of a line in various forms (point-slope, slope-intercept)
In essence, constant rate of change is the key that unlocks the secrets of linear equations, enabling us to describe and analyze the patterns in data.
Related Concepts: Slope, Gradient, and Inclination
In the realm of linear functions, constant rate of change takes center stage, and it goes hand-in-hand with a trio of related concepts: slope, gradient, and inclination. These terms are interchangeable and play a critical role in understanding the behavior of linear equations.
Slope: A Measure of Steepness
Imagine a mountain path ascending steadily towards a peak. The slope of the path represents how steeply or gradually it rises for every unit of horizontal distance traveled. Mathematically, slope is defined as the ratio of the vertical change (rise) to the horizontal change (run) along a line.
Gradient: A Synonym for Slope
The term gradient is often used interchangeably with slope. It also quantifies the steepness or inclination of a line. A line with a positive gradient rises from left to right, while a line with a negative gradient descends.
Inclination: The Angle of Ascent
Inclination measures the angle at which a line is tilted with respect to the horizontal. It is closely related to slope, as the steeper the slope, the greater the inclination. An inclination of 0 degrees indicates a horizontal line, while an inclination of 90 degrees represents a vertical line.
By understanding these related concepts, you gain a deeper appreciation for the behavior of linear functions and their constant rate of change.
Rise and Run: Understanding the Anatomy of Constant Rate of Change
In the world of linear equations, there’s a concept that breathes life into these mathematical expressions: constant rate of change. Just like a car’s speedometer measures its speed over distance, the constant rate of change tells us how fast a line is climbing or descending as it moves along.
Central to understanding this concept is the duo of rise and run. Rise refers to the vertical change a line makes, while run is its horizontal movement. Imagine a hiker ascending a mountain trail. The vertical distance she climbs, from the trailhead to a certain point, is the rise. The horizontal distance she covers along the trail is the run.
Now, here’s the magic: By dividing the rise by the run, you get the constant rate of change. This ratio tells you how much the line changes vertically for every unit of horizontal change. It’s like the car’s speedometer, but for lines!
This ratio also has another important name: slope. The slope of a line tells us not only the constant rate of change but also how steep or gentle it is. A higher slope indicates a steeper line, while a lower slope suggests a more gradual climb or descent.
For example, consider a line that rises 3 units vertically and moves 2 units horizontally. The constant rate of change, or slope, would be 3/2. This means that for every 2 units you move along the line horizontally, it rises by 3 units vertically.
Understanding rise and run is crucial for grasping the concept of constant rate of change. It’s like having a roadmap to navigate the ups and downs of linear equations. So, next time you encounter a line, pause and consider its rise and run. They’ll tell you a story about its constant rate of change and reveal its true nature.
Constant Rate of Change and the Elusive Y-Intercept
In the world of linear equations, the constant rate of change reigns supreme. It tells us how a line is sloping up or down, giving us valuable insights into its behavior. And at the heart of this slope-defining equation lies a mysterious point known as the y-intercept.
The y-intercept is a unique coordinate on the y-axis where our line decides to take a break from its journey across the plane. It’s the point where the line intersects the y-axis, with no x-coordinate influencing its position.
Unveiling the Y-Intercept’s Role
The y-intercept plays a crucial role in determining the constant rate of change. It’s like the seed from which the slope grows. You see, the slope, that measure of the line’s steepness, is calculated by dividing the “rise” (the vertical change) by the “run” (the horizontal change). But here’s the catch: when the horizontal change is zero, which happens when the line is vertical, the slope becomes undefined.
However, even in this vertical world, the y-intercept shines through. It becomes the sole determinant of the line’s position, as the line essentially becomes a vertical column passing through that y-intercept coordinate.
The Y-Intercept and Point-Slope Form
The y-intercept also holds significance in the equation-writing game. When we use the point-slope form to define a line, the y-intercept makes its presence felt in the constant term. This term represents the vertical shift from the given point to the intercept, providing a glimpse into the line’s overall behavior.
For instance, in the equation y = 2x + 5, the constant term 5 represents the y-intercept, telling us that the line crosses the y-axis at the point (0, 5).
Point-Slope Form and Slope-Intercept Form: Unraveling the Secrets of Constant Rate of Change
In the enchanting realm of linear equations, where lines dance gracefully across the coordinate plane, understanding the constant rate of change is paramount. This elusive concept holds the key to unlocking the secrets of these equations, and two indispensable tools that guide us on this journey are the point-slope form and slope-intercept form.
Point-Slope Form: The Foundation of Understanding
Imagine yourself standing at a specific point on a line, like a brave explorer navigating an uncharted territory. The point-slope form is your trusty compass, allowing you to determine the line’s constant rate of change based on this single point. It whispers to you an equation that represents the line’s path, using the point’s coordinates and the line’s slope:
y - y₁ = m(x - x₁)
Where (x₁, y₁) is the given point, and m is the slope, the magical number that measures the line’s inclination.
Derivation of Slope-Intercept Form: A Bridge to Convenience
The slope-intercept form emerges as a simplified yet powerful representation of the line’s equation, unveiling its constant rate of change with unmatched clarity:
y = mx + b
Intriguingly, the slope-intercept form is a metamorphosis of the point-slope form. By substituting y – y₁ with y and then rearranging the terms, we arrive at this elegant expression.
Convenience and Clarity: The Essence of Slope-Intercept Form
The slope-intercept form shines with unparalleled convenience. Its coefficient before x, the elusive m, is none other than the line’s constant rate of change, the holy grail of our quest. The intercept, represented by b, reveals the line’s intersection with the y-axis, providing an additional layer of insight.
In the vast tapestry of linear equations, understanding the constant rate of change is a tapestry’s thread, connecting points and weaving the fabric of knowledge. The point-slope form and slope-intercept form are the needles that guide this intricate embroidery, empowering us to unravel the mysteries of linear relationships.