Ultimate Guide To Counting Dots: Master Dot-Counting In Triangles, Squares, Rectangles, And Circles

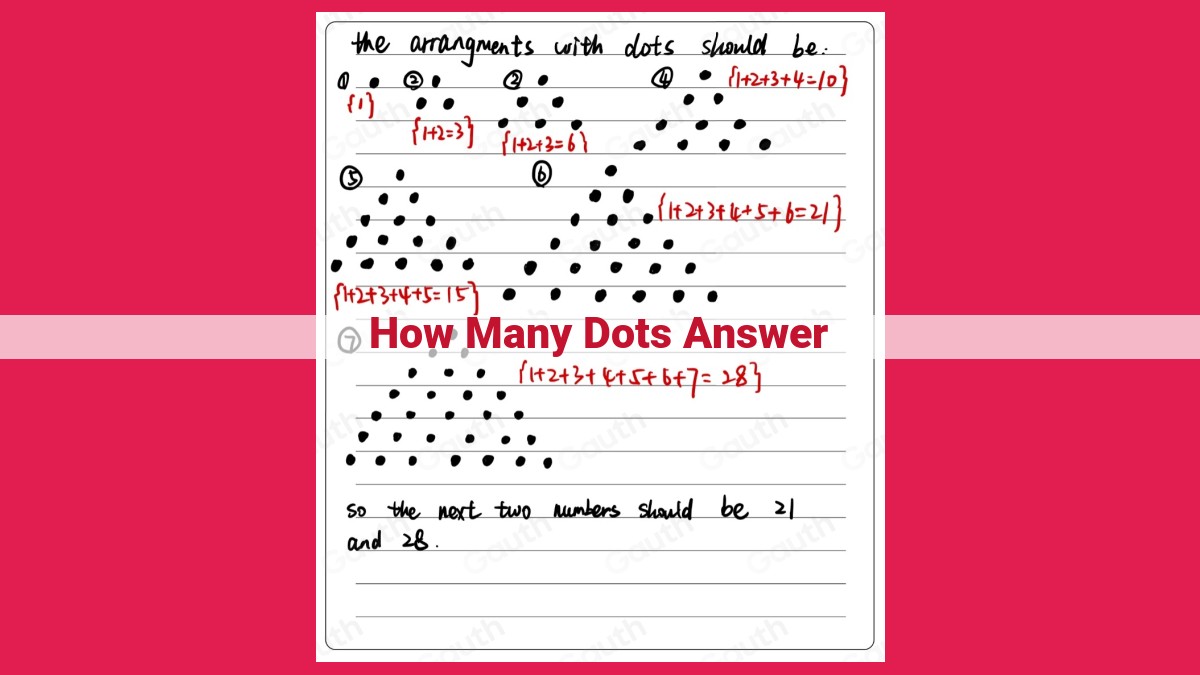
This comprehensive guide covers everything related to counting dots in various patterns. It explains the basics of counting dots individually and provides efficient tips. For triangular patterns, the concept of triangular numbers and the related formula are introduced. For square and rectangular patterns, formulas based on area are provided. Lastly, the guide details the process of counting dots in circle patterns, including dividing the pattern into sectors and calculating the fraction of dots in each sector. This guide is a valuable resource for anyone who needs to count dots accurately and efficiently in a variety of patterns.

Counting Dots: A Comprehensive Guide to Mastering the Art

In the world of patterns, understanding how to count dots is an essential skill that extends beyond mere mathematics. It’s a journey that takes us from simple counting to unraveling the mysteries of intricate designs. Let’s embark on this adventure, starting with the most fundamental step: counting dots individually and adding them together.

Imagine a scattered field of dots, like a playful frolic across a blank canvas. Each dot is a tiny universe in itself, waiting to be explored. Our task is to traverse this field, carefully examining each dot and meticulously tallying their numbers. As you embark on this counting quest, a rhythm may emerge. You may find yourself humming a tune or tapping your foot, synchronizing your movements with the repetitive counting. Embrace this rhythm, for it is the heartbeat of your journey.

The key to success lies in staying organized. Divide the field into smaller sections, making it easier to conquer. Tackle each section one at a time, ensuring that every dot is accounted for. As you add up the dots in each section, the total number begins to take shape. It’s a gradual process, but with each addition, the puzzle pieces start to fit together, revealing the true extent of the pattern.

A Comprehensive Guide to Counting Dots in Various Patterns

1. Total Dots in a Pattern

Counting dots in a pattern may seem like a basic task, but there are efficient strategies to streamline the process. Imagine a game of “Connect the Dots” where each dot represents a piece of your puzzle. Connecting them one by one, individually counting them as you go, is a straightforward approach. However, for larger patterns or scattered dots, this method can be tedious.

Instead, try visualizing the groups of dots. Look for rectangular or triangular shapes within the pattern that you can quickly calculate. For example, if you have a group of 5 dots arranged in a rectangle, simply multiply 5 x 2 to get 10 dots. This chunk counting technique saves time and reduces error.

2. Dots in a Triangular Pattern

Triangles have a special pattern when it comes to dots. The number of dots in each row increases by 1, forming a triangular number. The formula for triangular numbers is n(n+1)/2, where n is the number of rows. For instance, a triangle with 5 rows would have 15 dots (5(5+1)/2 = 15).

3. Dots in a Square Pattern

Squares are all about area. The number of dots in a square pattern is directly related to its side length. The formula is Side Length x Side Length. If you have a square with a side length of 5, it will have 25 dots (5 x 5 = 25).

4. Dots in a Rectangular Pattern

Rectangles follow a similar principle to squares, but with different dimensions. The formula for rectangular patterns is Length x Width. For example, a rectangle with a length of 6 and a width of 4 would have 24 dots (6 x 4 = 24).

5. Dots in a Circle Pattern

Circles can be a bit trickier, but with a little geometry, you can master dot counting. Imagine dividing the circle into equal sectors like a pie and counting the dots in each sector. The fraction of dots in one sector represents the fraction of dots in the entire circle. Multiply this fraction by the total number of dots in the pattern to get your final count.

Comprehensive Guide to Counting Dots in Various Patterns: A Storytelling Approach

Have you ever found yourself gazing upon a field of polka dots, wondering just how many little circles adorned the fabric? Or perhaps you’ve marveled at the intricate patterns of a stained-glass window, each piece containing countless dots? If so, fear not! This guide will embark us on a fantastical journey to demystify the art of counting dots.

From the humble counting of individual dots to the intricate patterns of triangles, squares, rectangles, and circles, we shall explore the secrets that lie within these seemingly simple shapes. Along the way, we’ll uncover mathematical formulas and techniques that will make dot counting a breeze.

Triangular Patterns: The Secrets of Triangular Numbers

Imagine a world where dots form perfect triangles. In this realm, there exists a magical formula known as the triangular number. It whispers to us the number of dots that reside in a triangle with a given number of rows. Prepare yourself, for this mystical formula is none other than:

Tn = n * (n + 1) / 2

Where Tn represents the triangular number for n rows.

Now, let’s delve into the enchanting world of square and rectangular patterns, where dots dance in geometric formations, just waiting to be counted!

Comprehensive Guide to Counting Dots in Various Patterns

Counting Dots in a Triangular Pattern

Triangular numbers fascinate with their intricate patterns and hidden mathematical secrets. Each row resembles a triangle, with the number of dots increasing steadily. To decode the mystery of counting dots in these mesmerizing formations, we embark on a mathematical journey.

The Triangular Number Formula

A triangular number, T(n), represents the sum of all positive integers up to the nth number. It is calculated using the formula:

T(n) = n * (n + 1) / 2

Applying the Formula

Unveiling the number of dots in a triangular pattern becomes a breeze with this formula. Let’s say you have a triangular pattern with 5 rows. Substituting n = 5 into the formula, we get:

T(5) = 5 * (5 + 1) / 2 = 5 * 6 / 2 = 15 dots

Similarly, for a triangular pattern with 10 rows:

T(10) = 10 * (10 + 1) / 2 = 10 * 11 / 2 = 55 dots

This formula empowers you to determine the precise number of dots in any triangular pattern with ease and precision.

Counting Dots in Square Patterns: Unraveling the Dotty Enigma

In the realm of dot-counting, square patterns present a unique challenge. Unlike linear patterns, where dots are neatly lined up, dots in squares dance in a playful dance across the Cartesian plane. But fear not, for I shall guide you through this dotty maze, revealing the secrets to unlocking the mysteries of square dot patterns.

The Area-Dot Dance

At the heart of counting dots in squares lies an intriguing relationship: the **area of the square and the number of dots it contains are intimately linked. Think of it as a grand dotty ballet, where the stage (the square) determines the number of dancers (the dots).

The Magic Formula: A Mathematical Dance

To unveil this dotty secret, let us don our mathematical hats and delve into the magical formula:

Number of Dots = Side Length × Side Length

This formula is like a magic wand, transforming the square’s side length into the number of dots it holds. For instance, a square with a side length of 5 would have a whopping 25 dots gracefully adorning its surface.

Example: The 3×3 Dotty Delight

Imagine a 3×3 square, a modest stage for our dotty adventure. Plugging it into our formula, we get:

Number of Dots = 3 × 3 = 9

And voila! Nine mischievous dots frolic gracefully within the confines of our 3×3 square.

Tips for a Seamless Dot-Counting Soiree

As you embark on your dot-counting journey, keep these tips close at hand:

• Grid it Up: Divide the square into smaller squares or rectangles to simplify the counting process.
• Split and Conquer: Break larger squares into smaller ones and count their dots individually before adding them up.
• Odd Side Lengths: For squares with odd side lengths, imagine a central dot and count only half of the dots on each side. Then, double your count to get the total.

With these tips in your arsenal, you’ll become a dot-counting maestro, effortlessly navigating the world of square patterns. So, go forth and embrace the joy of dot-discovery!

Master the Art of Dot Counting: A Comprehensive Guide

Dots, those tiny, unassuming specks, can often pose a puzzling challenge when it comes to counting them accurately. Whether it’s for a game, a puzzle, or simply satisfying your curiosity, this comprehensive guide will equip you with the essential formulas and techniques for counting dots in various patterns.

Counting the Basics: Adding Dots One by One

In its simplest form, counting dots involves patiently counting each one individually and then adding them together. This method works well for small patterns with a limited number of dots. For instance, if you have a pattern with 5 rows and 4 columns, simply multiply 5 × 4 to get a total of 20 dots.

Dots in Triangles: Uncovering Triangular Numbers

When it comes to counting dots in triangular patterns, there’s a clever mathematical shortcut called triangular numbers. These numbers follow a simple formula:

Tn = n(n+1)/2

Where Tn is the triangular number, and n is the row number. For example, to find the number of dots in a triangular pattern with 4 rows, use the formula: 4(4+1)/2 = 10.

Dots in Squares: Area and Dots Hand in Hand

Square patterns have a close relationship between their area and the number of dots they contain. The formula for counting dots in squares based on side length is:

Dn = S^2

Where Dn is the number of dots, and S is the side length. For instance, a square with a side length of 3 will have 3^2 = 9 dots.

Dots in Rectangles: Length, Width, and Dot Count

Rectangular patterns also rely on the concept of area. The formula for counting dots in rectangles is:

Dn = L * W

Where Dn is the number of dots, L is the length, and W is the width. If a rectangle has a length of 5 and a width of 3, it will have 5 × 3 = 15 dots.

Explain the concept of the area of a rectangle and how it relates to the number of dots.

Counting Dots in Rectangular Patterns: Unveiling the Connection Between Area and Dot Density

Imagine yourself standing in a vast field, dotted with countless pebbles. You’re tasked with counting them all, but there’s a rectangular patch in the middle that seems overwhelming. How do you approach this challenge?

The secret lies in understanding the relationship between the area of the rectangle and the number of dots it contains. Just like you can calculate the area of a rectangle by multiplying its length and width, you can also use this area to estimate the number of dots inside.

Let’s say you have a rectangular pattern with a length of 5 units and a width of 3 units. The area of this rectangle is 5 x 3 = 15 square units. Now, assume that the dots are evenly distributed within this rectangular boundary.

The number of dots per square unit can be considered as the dot density. Let’s call it “d”. To find the total number of dots, we can simply multiply the area of the rectangle by the dot density. That is:

Total Dots = Area of Rectangle x Dot Density
Total Dots = 15 square units x d

This formula allows you to quickly estimate the number of dots in a rectangular pattern without having to count them individually. However, it’s important to note that the accuracy of this estimate depends on the assumption of uniform dot distribution. If the dots are not evenly spaced, the formula may not provide an exact count.

Understanding this connection between area and dot density not only simplifies the counting process but also provides insights into the dot distribution and the overall arrangement of the pattern.

Comprehensive Guide to Counting Dots in Various Patterns

Have you ever found yourself staring at a pattern of dots, wondering just how many there are? Fear not, for this comprehensive guide will equip you with the knowledge and techniques to count dots like a pro, no matter the pattern they may form.

Total Dots in a Pattern:

The simplest approach is to count the dots individually and add them together. Start from one corner and methodically work your way across, ensuring you don’t double-count or skip any dots. If the pattern is particularly dense, you can divide it into smaller sections for easier counting.

Dots in a Triangular Pattern:

Triangular patterns are special because they follow a mathematical formula that helps us determine the number of dots they contain. The “triangular number” formula is given by:

T_n = (n * (n + 1)) / 2

where T_n represents the triangular number for row n. Simply plug in the row number and you’ll have the exact count of dots in the triangle.

Dots in a Square Pattern:

Square patterns are another common type. The number of dots in a square pattern is directly related to its area. The formula for the number of dots in a square is:

D_n = n^2

where D_n is the number of dots and n is the length of one side of the square.

Dots in a Rectangular Pattern:

Rectangular patterns are similar to square patterns, but with different side lengths. To find the number of dots in a rectangle, first calculate its area:

A = length * width

where A is the area, length is the length of the longer side, and width is the length of the shorter side. Then, simply use the same formula as for squares:

D_n = A = length * width

Remember, in this case, A represents the area of the rectangle.

Dots in a Circle Pattern:

Circle patterns can be a bit more challenging, but a clever trick can make counting them easier. Divide the circle into equal sectors like a pie, and count the dots in one sector. To get the total number of dots, multiply the number of dots in the sector by the number of sectors.

Now, you’re armed with the knowledge to conquer any dot counting challenge. From simple patterns to intricate circles, you can count them all with ease and confidence. And the next time someone asks, “Excuse me, how many dots are there?” you’ll be the dot-counting hero!

The Enigmatic World of Dots: A Comprehensive Guide to Counting Dots in Various Patterns

In the realm of mathematics, where patterns dance and numbers whisper secrets, lies a fascinating enigma: the art of counting dots. From the simplest arrays to intricate geometric shapes, the ability to accurately determine the number of dots hidden within these patterns is an essential skill that unlocks the doors to a world of mathematical wonders.

Total Dots in a Pattern: The Foundation

Embarking on this journey, we begin with the most basic concept: counting dots individually and diligently adding them together. Just like a meticulous explorer navigating an uncharted terrain, we traverse the pattern, marking each dot with a stroke of our counting pen.

Dots in a Triangular Pattern: Unveiling Triangular Numbers

As we delve deeper, we encounter triangular patterns, where rows of dots form an alluring pyramid-like structure. Enter the magical realm of triangular numbers, a special sequence where each number represents the total dots in a triangular pattern with that corresponding row count. Armed with the formula for triangular numbers, we can effortlessly determine the number of dots in these enchanting formations.

Dots in a Square Pattern: Geometry’s Canvas

Next, we turn our attention to square patterns, where dots form a perfectly aligned grid. Here, we uncover the intimate connection between the area of a square and the number of dots it harbors. By employing a clever formula, we can swiftly calculate the dot count in square patterns of varying side lengths.

Dots in a Rectangular Pattern: Exploring the Quadrilateral Realm

Expanding our horizons, we encounter rectangular patterns, where dots dance within the confines of parallel lines. Just as in the case of squares, the area of a rectangle holds the key to unlocking the riddle of dot counting. With a modified formula, we can conquer this quadrilateral challenge, determining the dot count in rectangular patterns of various lengths and widths.

Dots in a Circle Pattern: Breaking the Circular Enigma

Finally, we venture into the mystical world of circle patterns, where dots orbit a central point like celestial bodies. Here, we embrace a more nuanced approach, dividing the circle into equal sectors and counting the dots within each sector. By calculating the fraction of dots in each sector, we can deduce the total number of dots in the circular pattern.

Mastering the art of counting dots in various patterns not only sharpens your mathematical prowess but also reveals the hidden beauty and order that underlies our world. From the simple elegance of rows and columns to the captivating allure of circles and triangles, the ability to count dots unlocks a door to a universe of mathematical discovery.

Comprehensive Guide to Counting Dots in Various Patterns

Welcome to the ultimate guide to counting dots! From simple patterns to complex arrangements, we’ll unravel the secrets of dot counting, empowering you to conquer this mathematical challenge with ease.

1. Total Dots in a Pattern

Let’s start with the basics. Counting dots in a pattern simply involves tallying up each dot individually. For larger patterns, group counting can help: break the pattern into smaller sections and count the dots within each.

2. Dots in a Triangular Pattern

Triangular patterns reveal a fascinating pattern: the number of dots in each row follows a sequence known as triangular numbers. The formula for the nth triangular number is n(n+1)/2, where n is the row number.

3. Dots in a Square Pattern

Squares are all about geometry! The number of dots in a square pattern is directly related to its area. The formula to calculate the number of dots is side length * side length.

4. Dots in a Rectangular Pattern

Rectangles, like squares, involve area calculation. To find the number of dots, simply multiply the length by the width of the rectangle.

5. Dots in a Circle Pattern

Circles pose a unique challenge. We’ll divide the circle into equal sectors and count the dots within each. Since we can’t always count all the dots in a sector, we’ll calculate the fraction of dots in a sector and multiply it by the total number of sectors to determine the grand total.

Tips for Accuracy:

• Use a magnifying glass for small patterns.
• Mark off counted dots to avoid double-counting.
• Estimate the number of dots and then count to verify.
• If possible, use a grid or coordinate system to guide your counting.

With these techniques, you’ll be an expert dot counter, ready to tackle any pattern that comes your way!

Tips for Accurate Dot Counting in Circle Patterns: A Comprehensive Guide

Circle patterns, with their intricate curves, can be notoriously tricky to count. But fear not, dear reader! With the right techniques, you can conquer this challenge and emerge victorious.

1. Sectorize:

Imagine slicing your circle into pie-like wedges, called sectors. By focusing on one sector at a time, you can simplify the counting process significantly.

2. Calculate Sector Fraction:

To find the fraction of dots in a sector, divide the sector’s angle by 360 degrees (the total angle of the circle). This will give you a percentage representing the proportion of dots within that sector.

3. Mark Your Dots:

As you count dots within each sector, mark them with a pen or marker to avoid recounting. This will help you keep track of your progress and avoid any confusion.

4. Multiply by Fraction:

Once you’ve counted the dots in a single sector, multiply that number by the fraction you calculated in step 2. This will give you the total number of dots in that sector.

5. Sum Up the Sectors:

Repeat this process for each sector of your circle. Then, add up all the individual sector counts to obtain the grand total of dots in your pattern.

Bonus Tip:

For patterns with many dots, consider using a digital tool or app that automates the counting process. This can save you time and eliminate potential errors.

Remember, patience and attention to detail are key. With these tips, you’ll be a dot-counting virtuoso in no time!