Unveiling The Midsegment Of A Triangle: A Comprehensive Guide For Understanding, Construction, And Applications

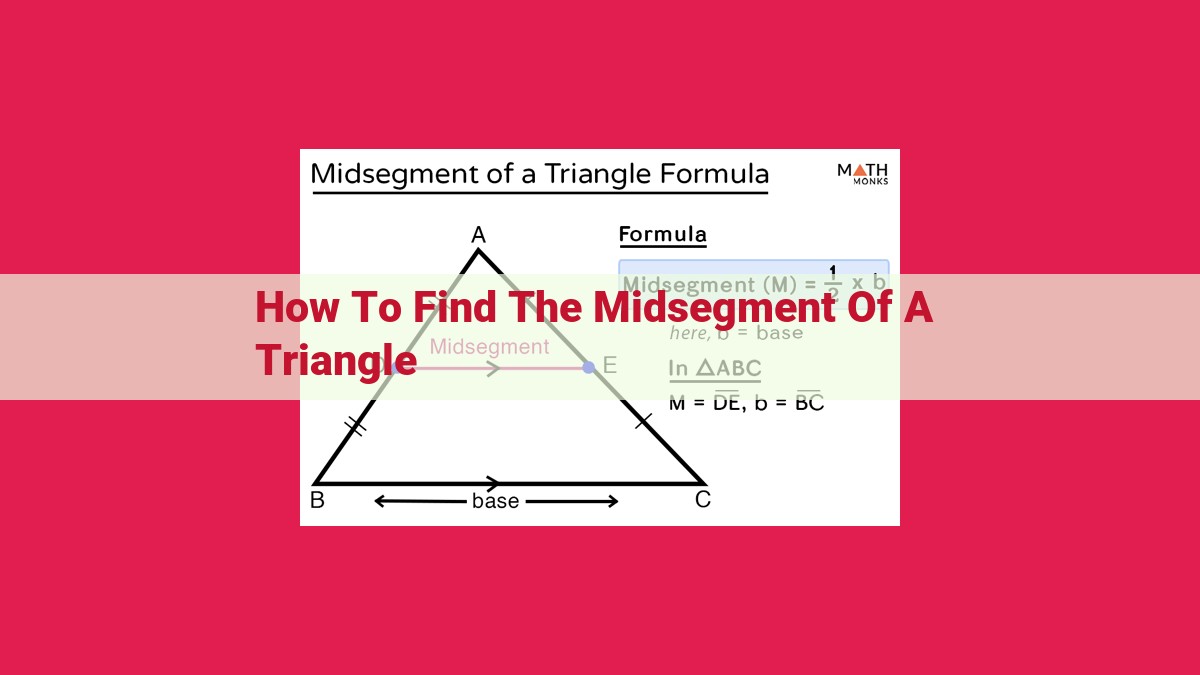
To find the midsegment of a triangle: identify the midpoints of two sides using the midpoint formula. These midpoints divide the sides into two equal segments. Draw a line connecting the midpoints to form the midsegment. This line segment runs parallel to the third side of the triangle and is half its length. Midsegments divide the triangle into two congruent triangles, and their properties can be used in various applications, such as finding side lengths and solving geometric problems.

Understanding the Basics of Midsegments: A Triangle’s Secret Ingredient

When it comes to triangles, one of the most intriguing concepts is the midsegment. To understand this geometric gem, let’s start with the basics. A triangle is a polygon with three sides, while a side of a triangle is one of its three straight edges.

Now, let’s talk about midpoints. A midpoint is a special point on a line segment that divides the segment into two equal parts. It’s like finding the perfect balance point on a seesaw! Understanding midpoints is crucial for grasping the elusive midsegment.

Midsegment of a Triangle

• Define “midsegment” as a line segment connecting midpoints of two sides.

The Midsegment of a Triangle: Unlocking Triangular Secrets

Triangles, with their enchanting angles and enigmatic sides, have enthralled mathematicians for centuries. Among their intriguing features lies the elusive midsegment, a line segment that connects the midpoints of two sides. Join us on an adventure to unravel the mysteries of this fascinating geometric entity.

Understanding the Midsegment

A midsegment is a line segment that connects the midpoints of two sides of a triangle. In essence, it bisects the third side, creating two smaller triangles that are congruent. This remarkable property makes midsegments instrumental in unlocking the secrets of triangles.

Benefits of the Midsegment

The midsegment offers a treasure-trove of benefits that enhance our understanding of triangles. For instance, it allows us to:

• Divide triangles into congruent triangles: Midsegments partition triangles into congruent triangles, simplifying calculations and proofs.
• Establish parallelism to the third side: Midsegments are always parallel to the third side of the triangle, providing valuable information about the figure’s shape and properties.
• Apply the Pythagorean Theorem: Midsegments can assist in applying the Pythagorean Theorem to solve complex triangle problems.

Finding the Midsegment

To find the midsegment of a triangle, simply follow these steps:

• Identify the midpoints of the two sides using the midpoint formula: x = (x1 + x2) / 2 and y = (y1 + y2) / 2.
• Connect the midpoints using a line segment.

The midsegment of a triangle is a powerful tool that reveals the hidden treasures of triangles. Its ability to divide triangles, establish parallelism, and facilitate Pythagorean Theorem calculations makes it an indispensable concept that enriches our understanding of this fascinating shape. Embrace the midsegment and discover the wonders that await within the world of triangles.

Finding Midsegments of Triangles: A Step-by-Step Guide

Understanding midsegments of triangles is crucial for exploring the geometry of these fundamental shapes. Midsegments offer valuable insights into the properties and relationships within triangles. Let’s dive into the steps to find the elusive midsegment:

Identifying Midpoints

The first step in determining the midsegment of a triangle is to identify the midpoints of two specific sides. A midpoint is the exact middle point of a line segment, dividing it into two equal parts. To find the midpoint of a line segment, we employ the midpoint formula:

Midpoint = ((x1 + x2) / 2, (y1 + y2) / 2)

Where (x1, y1) and (x2, y2) represent the coordinates of the endpoints of the line segment. By applying this formula, we reveal the coordinates of the midpoints.

Connecting Midpoints

Once we have identified the midpoints of the chosen sides, the next step is to connect these points. The line segment that joins the midpoints is the elusive midsegment of the triangle. This line segment runs parallel to the third side of the triangle.

Tips for Success

• Accuracy in Measurements: Determine the coordinates of the endpoints precisely to ensure accurate midpoint calculations.
• Midpoint Formula: Remember to use the midpoint formula correctly to avoid errors in identifying midpoints.
• Parallel Lines: Keep in mind that midsegments are parallel to the third side of the triangle. This relationship is a critical geometric feature to grasp.

Related Concepts

• Discuss parallel lines and the Pythagorean Theorem as related to midsegments.

Related Concepts: Parallel Lines and the Pythagorean Theorem

Understanding the relationship between midsegments and parallel lines is crucial. Midsegments are always parallel to the third side of the triangle. This property arises from the definition of a midpoint. When the midpoints of two sides are connected, they form a line segment that is parallel to the line segment connecting the opposite vertices.

The Pythagorean Theorem can also be applied to midsegments to find side lengths. If you know the lengths of the three sides of a triangle, you can use the Pythagorean Theorem to find the length of the midsegment. This principle is especially useful when the entire triangle is not present, and only side lengths are available.

For instance, suppose you have a triangle with side lengths a, b, and c. The length of the midsegment m that connects midpoints of sides a and b can be determined using the Pythagorean Theorem:

m² = (a² + b² - c²) / 4

This formula holds because the midsegment divides the triangle into two congruent right triangles. Each right triangle has legs equal to half of the sides of the original triangle.

Comprehending these related concepts empowers you to solve challenging geometry problems. Midsegments play a significant role in triangle geometry, offering insights into parallelism, proportionality, and the calculation of side lengths.

Benefits of Understanding Midsegments

Understanding midsegments not only unveils the intriguing properties of triangles but also equips you with practical tools to solve geometric puzzles.

Dividing Triangles into Congruent Parts

Midsegments, as their name suggests, serve as “middle” segments, connecting midpoints of two sides of a triangle. This unique placement has a remarkable consequence: it partitions the triangle into two congruent triangles.

For instance, consider triangle ABC. If M and N are the midpoints of sides AB and AC, the midsegment MN will divide the triangle into two triangles, AMN and MNC. These triangles are pairwise congruent, meaning they are identical in shape and size.

Parallelism to the Third Side

Another fascinating property of midsegments lies in their parallelism to the third side of the triangle. This observation stems from the Midsegment Theorem: In a triangle, a line segment connecting the midpoints of two sides is parallel to the third side and half its length.

Using the same triangle as before, the midsegment MN is parallel to side BC. Moreover, its length is exactly one-half of BC’s length. This parallelism and length relationship simplify many geometric calculations.

Calculating Side Lengths with the Pythagorean Theorem

Finally, midsegments play a crucial role in determining side lengths using the Pythagorean Theorem. For right triangles, the Pythagorean Theorem states that the square of the hypotenuse (the longest side) is equal to the sum of the squares of the other two sides.

Midsegments, when used in conjunction with the Pythagorean Theorem, can simplify the process of finding unknown side lengths. By leveraging the parallelism of midsegments and the fact that they divide triangles into right triangles, you can cleverly apply the Pythagorean Theorem to solve for unknown side lengths.

Understanding midsegments unlocks a wealth of geometric knowledge, empowering you to tackle geometric problems with ease. From dividing triangles into congruent parts to calculating side lengths with the Pythagorean Theorem, midsegments prove to be an essential tool in the arsenal of any geometry enthusiast.