Theoretical Probability: A Mathematical Guide For Calculating Event Probabilities
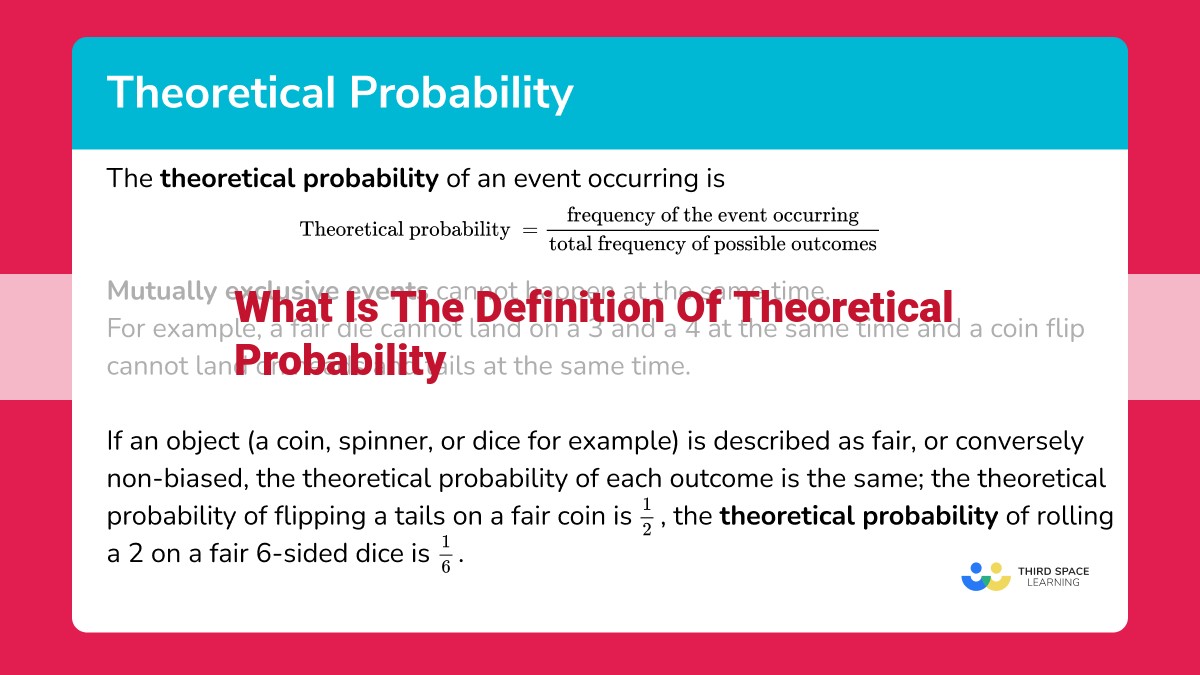
Theoretical probability is a mathematical framework that calculates the likelihood of specific outcomes based on the concept of sample space. It involves defining the set of all possible outcomes, called a sample space, and assigns probabilities to individual events, which are subsets of the sample space. The probability of an event is a numerical value indicating its likelihood, often determined through empirical observations or prior knowledge. This approach allows for the prediction of probabilities in various scenarios and provides a basis for understanding randomness and decision-making under uncertainty.
Understanding Sample Space: The Foundation of Probability
Delving into the enigmatic realm of probability, we embark on a journey to comprehend its fundamental building blocks. Sample space forms the cornerstone of this mathematical odyssey. It embodies the complete collection of all potential outcomes from a given experiment or trial. Imagine tossing a coin; the sample space would encompass both heads and tails.
Within the sample space resides a concept known as sigma-algebra. This formidable term refers to a collection of subsets of the sample space that possess specific properties, such as being closed under complementation and countable unions. Sigma-algebra is the backbone of probability theory, enabling us to define and quantify the likelihood of events.
Elementary Events and Outcomes: The Building Blocks of Probability
Understanding probability requires a firm grasp of the sample space, which represents the set of all possible outcomes from an experiment or trial. Within this sample space, we encounter elementary events – individual elements that make up the overall outcomes. These elementary events stand as the atomic units of probability, much like the protons and neutrons that compose an atom.
For instance, consider rolling a dice. The sample space for this experiment consists of six possible outcomes: {1, 2, 3, 4, 5, 6}. Each of these numbers represents an elementary event. Now, we can define singletons – events that consist of only a single elementary event. For example, the event “rolling a 2” would be represented as {2}.
Understanding elementary events and outcomes forms the cornerstone of probability theory. It allows us to break down complex experiments into simpler components, enabling us to calculate the likelihood of specific events occurring.
Events: Subsets of the Sample Space
Embarking on the Adventure of Probability
In the realm of probability, we embark on a journey to unravel the secrets of chance and uncertainty. To navigate this fascinating world, it’s essential to grasp the concept of events, the building blocks of this mathematical realm.
Events as Subsets: The Canvas of Outcomes
An event is simply a collection of outcomes from the sample space. Imagine spinning a coin; the sample space comprises two outcomes: heads or tails. An event could be obtaining heads, represented by the subset {H}.
Occurrence of Events: The Dance of Chance
The occurrence of an event is like a performance on the stage of chance. When an outcome within the event’s subset materializes, the event is said to have occurred. In our coin toss example, if heads appear, the event {H} has come to life.
Events: The Palette of Probability
Events paint the vibrant colors of probability. By understanding the occurrence of events, scientists and analysts can measure the likelihood of future outcomes. As you delve deeper into the world of probability, you’ll discover that events hold the key to unlocking the mysteries of chance and uncertainty.
The Probability of an Event: Unveiling the Likelihood of Outcomes
In the realm of probability theory, the concept of sample space and elementary events forms the cornerstone upon which the edifice of probability rests. But what happens when we want to analyze the likelihood of a specific outcome or event? This is where the pivotal concept of probability takes center stage.
Defining Probability: A Gateway to Likelihood
Probability, in essence, is a numerical expression that quantifies the likelihood or chance of an event occurring. It assigns a value between 0 and 1, where 0 indicates impossibility and 1 represents certainty.
Relative Frequency: A Tale of Repeated Trials
One way to calculate probability is through relative frequency. Imagine flipping a coin multiple times and counting the number of heads that appear. As the number of flips increases, the ratio of heads to total flips approaches a stable value. This value is the relative frequency of heads, which serves as an estimate of the probability of getting heads on a single coin flip.
Bayesian Probability: Embracing Prior Knowledge
In certain scenarios, we may possess additional information or prior knowledge that can influence our assessment of probability. This is where Bayesian probability comes into play. Bayesian probability takes into account both the relative frequency of an event and any relevant prior knowledge, combining them to provide a more refined estimate of probability.
Delving Deeper: Advanced Probability Concepts
While we have explored the fundamental concept of probability, the field of probability theory extends far beyond these introductory ideas. Researchers and practitioners delve into complex concepts such as conditional probability, independence, and random variables, each unlocking new layers of understanding in the realm of chance and uncertainty.