The Circumcenter Of A Triangle: Unveiling Geometric Relationships

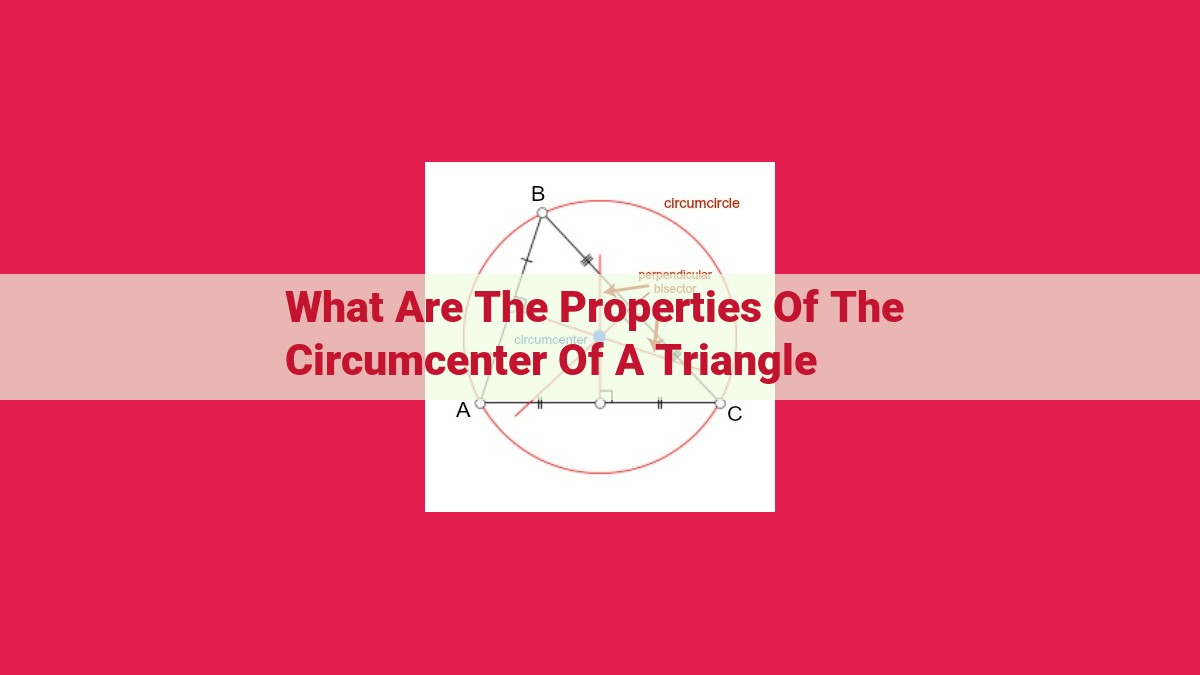
The circumcenter of a triangle is the center of the circumcircle, a circle passing through all three vertices. It is equidistant from all vertices and lies on the perpendicular bisector of any side. The circumcenter is connected to the incenter, centroid, and orthocenter by the Euler line, a special line in triangle geometry. It represents the point of concurrence of the altitudes, the perpendicular lines drawn from the vertices to their opposite sides. Understanding the circumcenter’s properties and its relationship with other triangle centers provides insights into the geometric relationships within a triangle.

The Circumcenter: A Definition

• Explain the concept of a circumcenter as the center of a circle passing through the triangle’s vertices.

The Circumcenter: The Heart of a Triangle’s Geometry

In the realm of geometry, triangles have captivated mathematicians for centuries. Among the fascinating properties of a triangle is its circumcenter, a point that holds a unique and significant role.

Imagine a triangle with vertices A, B, and C. Now, envision a circle that gracefully arcs around these points, touching them all. The center of this circle, the magical point that keeps the triangle’s dance in perfect harmony, is known as the circumcenter.

This enigmatic point possesses a remarkable attribute: _** equidistance from all three vertices**. No matter how the triangle is positioned, the circumcenter remains steadfastly equidistant from A, B, and C. This enchanting property sets the circumcenter apart from other geometric marvels.

The circumcenter’s embrace extends beyond the triangle’s boundaries, touching upon lines that dissect its sides. For any side, be it AB, BC, or CA, the circumcenter rests contentedly on its perpendicular bisector. These lines, perpendicular to their respective sides and bisecting them at their midpoints, form a tight-knit network with the circumcenter as their common thread.

Unveiling the Secrets of the Triangle’s Circumcenter

Embark on an enchanting journey into the realm of geometry, where we unravel the mysteries of the enigmatic circumcenter, the heart of every triangle.

As you navigate through this article, you’ll discover the fascinating tapestry of interconnected concepts that revolve around the circumcenter. Like a cosmic dance, these concepts intertwine, creating a mesmerizing symphony of geometry.

At its inception, the circumcenter emerges as the radiant center of a circle that gracefully encircles the triangle’s three vertices. With an air of impartiality, it equidistantly embraces each vertex, forging an unbreakable bond.

Venture further into our exploration, and you’ll encounter the perpendicular bisector, a line that cleaves a triangle’s side in two, perpendicularly. Lo and behold, the circumcenter stands sentinel on this bisector, a testament to its pivotal role in maintaining the triangle’s equilibrium.

But the circumcenter’s cosmic dance is not confined to the triangle’s interior. It reaches out, connecting with other enigmatic figures like the incenter and centroid. Together, they form the hallowed Euler line, a celestial pathway that weaves through the heart of the triangle.

As we delve into the depths of geometry, we encounter the enigmatic orthocenter, a point where the altitudes, those perpendicular lines drawn from each vertex to its opposite side, converge. However, unlike its celestial companion, the circumcenter, the orthocenter does not adorn the Euler line. It stands aloof, a solitary figure in the triangle’s celestial tapestry.

Unravel the captivating tale of the circumcenter, a concept that illuminates the intricate beauty of geometry. As you immerse yourself in this exploration, may you discover the wonder and awe that lies at the heart of mathematics.

Equidistance from Vertices

• Discuss the property that the circumcenter is equidistant from all three vertices of the triangle.

Equidistance from Vertices: A Unique Property of the Circumcenter

In the realm of geometry, the circumcenter plays a significant role in understanding the intricate relationships within a triangle. This enigmatic point, often denoted as the center of the circumscribed circle, possesses a remarkable property that sets it apart: it stands equidistant from all three vertices of the triangle.

Imagine you have a triangle, like a slice of a pizza. The vertices represent the tips of the slices, where you’d take your first bite. Now, think of a circle that goes around the entire triangle, touching each vertex like a ring around a finger. The circumcenter is the very center of this circle, like the hub of a wheel.

The beauty of the circumcenter lies in its equal distance from all vertices. It’s as if it’s the heart of the triangle, sending out equal love and affection to each corner. This special property gives the triangle a sense of balance and harmony.

Understanding the equidistance of the circumcenter is crucial because it unlocks many insights into the triangle’s geometry. For instance, it helps us calculate the radius of the circumscribed circle, which represents the distance from the circumcenter to any vertex. It also aids in determining the triangle’s circumference, the length of the path around the circumscribed circle, like the perimeter of a circle.

So, the next time you stumble upon a triangle, remember the circumcenter, the master of equidistance. Its presence brings order and symmetry to the shape, making it a fundamental aspect of geometric explorations.

The Circumcenter: A Perpendicular Bisector of Triangle Sides

In the realm of geometry, understanding triangle properties is crucial. Among them, the circumcenter stands out as a fascinating point with unique characteristics. Imagine a circle gracefully enveloping a triangle, its contours gently grazing each vertex. The circumcenter, like a conductor orchestrating this harmonious union, resides at the very **heart of this circle.**

Unveiling the Circumcenter’s Essence

Delving deeper into the circumcenter’s secrets, we uncover its defining quality: equidistance. It maintains an impartial distance from all three vertices of the triangle, ensuring a perfect balance of relationships. This remarkable property stems from the circumcenter’s strategic position on the perpendicular bisector of any side.

Unfolding the Perpendicular Bisector

Visualize a line perpendicular to a side of the triangle, slicing it in two perfect halves. This line, aptly named the perpendicular bisector, possesses a remarkable connection with the circumcenter. In a mesmerizing interplay of geometry, the circumcenter gracefully resides on this bisector, establishing a pivotal role in the triangle’s intricate architecture.

The circumcenter, a point of pivotal significance in a triangle, unveils its captivating nature through its equidistance from the vertices and its intimate relationship with the perpendicular bisectors. As we delve deeper into the fascinating tapestry of geometry, the circumcenter continues to enchant, revealing its intricate beauty and profound impact on triangle properties.

The Euler Line: A Path of Notable Triangle Points

The Euler line is an intriguing geometric feature associated with triangles. It forms a captivating connection between three significant points within the triangle’s realm: the circumcenter, the incenter, and the centroid. Embark on this journey as we delve into the captivating world of the Euler line.

Connecting the Circumcenter, Incenter, and Centroid

Visualize a triangle’s three vertices as beacon lights illuminating the geometric landscape. The circumcenter, nestled comfortably within the triangle’s realm, commands respect as the undisputed center of the triangle’s circumcircle, a sentinel of perfect symmetry. The incenter, on the other hand, takes center stage within the incircle, a smaller circle harmoniously touching the triangle’s interior sides. Finally, the centroid, a geometric balancing act, resides at the triangle’s heart, where its medians meet.

The Euler line emerges as a mystical thread weaving together these three celestial bodies. It traces a path, commencing at the circumcenter, descending through the triangle’s interior, and culminating at the centroid. This line serves as a testament to the triangle’s inherent order, a testament to geometry’s unwavering precision.

Unveiling the Euler Line’s Secrets

The Euler line holds secrets that amplify its allure. One such secret lies in its intrinsic characteristic: the circumcenter, incenter, and centroid align in perfect harmony along this geometric pathway. It’s as if nature has decreed that these points shall forever be intertwined, destined to dance along a single celestial path.

Another secret unfolds when examining the triangle’s perpendicular bisectors. These lines, emanating from the midpoint of each side and bisecting it perpendicularly, intersect the Euler line. This remarkable property further cements the Euler line’s significance within the triangle’s architecture.

A Glimpse Beyond the Euler Line

While the Euler line reigns supreme in connecting these three triangle points, it is not without a challenger. The orthocenter, an enigmatic point where the triangle’s altitudes converge, holds sway in another realm. Unlike the circumcenter, incenter, and centroid, the orthocenter stands aloof from the Euler line, maintaining its own unique place within the triangle’s cosmic dance.

The Euler line is a geometric marvel, a testament to the interconnectedness of triangle points. It serves as a beacon, guiding us through the depths of triangle geometry, revealing the harmonious relationships that govern these captivating shapes. As we witness the circumcenter, incenter, and centroid waltz along this celestial path, we cannot help but marvel at the intricate beauty of geometry, a testament to the wonders that lie hidden within mathematical realms.

Orthocenter

• Explain the concept of the orthocenter as the intersection point of the triangle’s altitudes.
• Note that the orthocenter, unlike the circumcenter, does not lie on the Euler line.

The Elusive Orthocenter: The Point Where Altitudes Intersect

In the world of triangles, there’s a special point that captures the essence of height and geometry: the orthocenter. Unlike its counterpart, the circumcenter, which lies equidistant from the triangle’s vertices, the orthocenter takes a different approach.

The orthocenter is the point where the altitudes of a triangle meet. Altitudes are lines that extend perpendicular from each vertex to the opposite side. As these lines intersect, they create a unique point that provides insights into the triangle’s geometry.

The Orthocenter’s Independence

While the circumcenter lies on the Euler line, a line connecting the triangle’s circumcenter, incenter, and centroid, the orthocenter takes its own path. It stands independently, not confined to the confines of the Euler line. This distinction makes it a point of interest for geometers and enthusiasts alike.

Applications in Real Life

The orthocenter has practical applications beyond the world of pure mathematics. In architectural design, for example, it can be used to determine the point where support structures intersect, ensuring stability and load bearing capacity. It also plays a role in surveying, where it helps establish precise measurements and boundaries.

A Point of Intrigue

The orthocenter is a fascinating point in the geometry of triangles. Its independence from the Euler line and its practical applications make it an object of wonder and analysis. Whether you’re a student of mathematics, an aspiring architect, or simply curious about the hidden wonders of geometry, the orthocenter is sure to spark your interest and expand your understanding of this intriguing shape.