Isolating The Variable N In Equations: A Guide To Solving For N

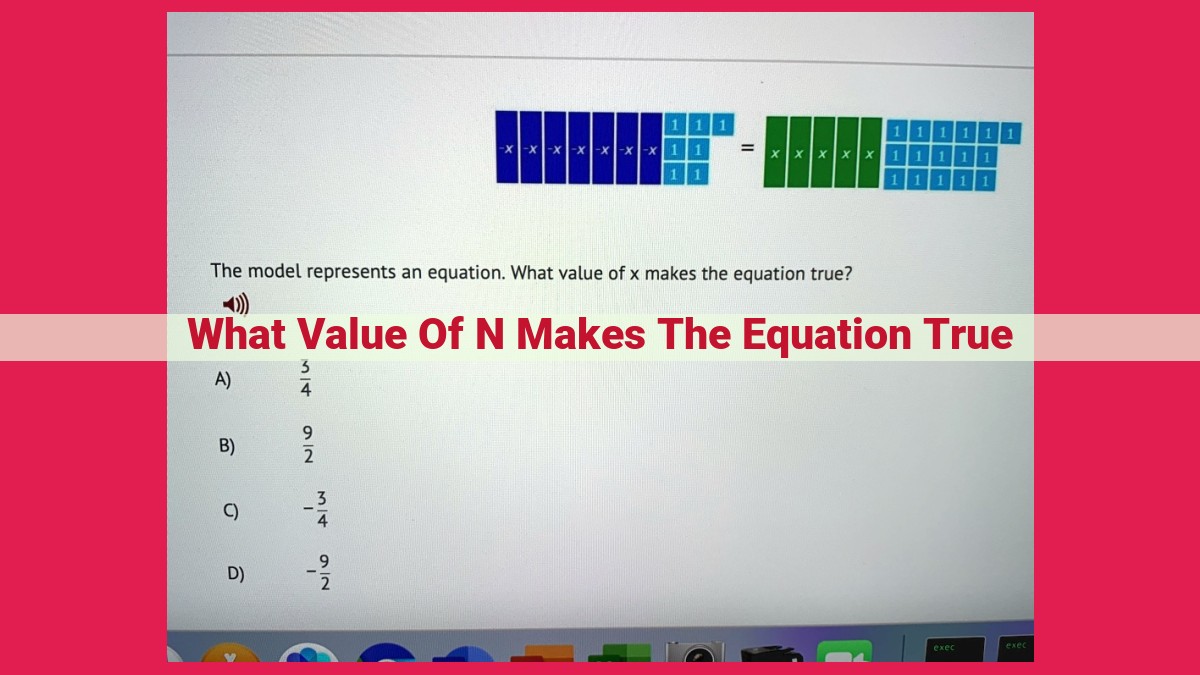
To determine the value of n in an equation, we isolate n on one side of the equation. This involves using algebraic properties such as the identity property (replacing n with n), multiplication and division properties (multiplying or dividing both sides by a constant), and addition and subtraction properties (adding or subtracting the same constant from both sides). By simplifying the equation and performing these operations, we can isolate n and find its value that satisfies the given equation.

Mastering the Art of Isolating n: An Essential Guide

In the realm of algebra, isolating the unknown variable, often denoted as “n,” is a crucial step towards solving equations. This process involves using various algebraic properties to manipulate the equation and ultimately determine the value of n. Let’s delve into the intricacies of isolating n and explore the techniques that will empower you to tackle any algebraic puzzle with confidence.

The Significance of Isolating n

The ultimate goal of solving an equation is to find the value of the unknown variable. However, in many cases, the variable is buried within a complex expression, making it difficult to determine its precise value. Isolating n involves transforming the equation into a form where n stands alone on one side of the equation. This allows us to read off its value directly.

Algebraic Properties: Your Equation Transformers

Like master builders with tools, we employ a toolbox of algebraic properties to isolate n. These properties serve as the levers and gears that enable us to manipulate the equation without altering its solution.

• Additive Identity Property: This property states that adding or subtracting zero to an expression does not change its value. It allows us to add or subtract the same value to both sides of the equation to simplify it.

• Multiplicative Identity Property: Similarly, multiplying or dividing an expression by one does not alter its value. This property empowers us to multiply or divide both sides of the equation by the same non-zero number to simplify it.

• Commutative Property of Addition and Multiplication: These properties allow us to rearrange terms within the equation without changing its overall value. They are like flexible building blocks, giving us freedom to manipulate the equation’s structure.

• Distributive Property: This property governs the multiplication of a sum by a factor. It is akin to a blueprint that guides us in distributing values across multiple terms within an expression.

By skillfully combining these algebraic properties, we can gradually transform the equation and isolate n, making its value clear and accessible.

Unraveling the Enigma of Algebra: A Guide to Isolating n

In the enigmatic world of algebra, the enigmatic variable n often holds the key to unlocking complex equations. To decipher these mathematical mysteries, we must embark on a journey of isolating n – a process that unravels the unknown and unveils the secrets it conceals.

Substitution: A Path of Simplification

One potent weapon in our arsenal of algebraic tools is substitution, a technique that transforms equations into simpler forms. Its essence lies in replacing an unknown variable with a more manageable expression. Consider the equation:

2x + 5 = 15

To isolate x, we can substitute 2y for x, as it is given that x = 2y:

2(2y) + 5 = 15

This substitution simplifies the equation, making it easier to solve for y and subsequently find the value of x.

Examples of Substitution

• Simplifying: Replace x with 2y in the equation 3x – 4 = 7 to obtain 6y – 4 = 7.
• Solving: Substitute y for x in the equation 2x + 5 = 15 to find y = 5. Using the fact that x = 2y, we can conclude that x = 10.

Substitution breathes new life into complex equations, streamlining and elucidating their paths to solutions.

Unlocking the Identity Property: A Key to Isolating n

Introduction:
Navigating the world of equations can be daunting, especially when you encounter the elusive variable n. To solve for n, we must delve into a secret arsenal of algebraic tools, including the ever-reliable Identity Property.

Identity Property: Unveiling the Truth
The Identity Property proclaims that any number or expression added to or subtracted from itself remains unchanged. In other words, a + 0 = a or a – 0 = a. This seemingly simple concept holds immense power when it comes to isolating n.

Unlocking n through the Identity Property:
Let’s consider an equation:

n - 5 = 10

To isolate n, we need to remove the subtraction of 5 from the equation. According to the Identity Property, we can add 5 to both sides of the equation without altering its balance. Doing so, we get:

n - 5 + 5 = 10 + 5

Simplifying further, we obtain:

n = 15

Conclusion:
The Identity Property is a fundamental principle in algebra, empowering us to manipulate equations and isolate variables like n. By understanding and applying this property, we gain control over complex-looking equations, revealing their hidden solutions. So, next time you encounter an equation with a hidden variable, remember the Identity Property – it holds the key to unlocking the unknown.

Multiplicative and Divisive Properties in Isolating n

When solving equations, isolating n is crucial to determine its value. Among the fundamental algebraic properties, the Multiplication and Division Properties of Equality play a pivotal role in this process.

The Multiplication Property of Equality states that when both sides of an equation are multiplied by the same nonzero number, the equality remains intact. This property is applied to eliminate a fractional coefficient or a decimal from the equation. Multiplying both sides by the least common denominator or inverse of the decimal results in an equation with integer coefficients, simplifying the solution process.

For instance, consider the equation: 2n / 5 = 4. To isolate n, multiply both sides by 5, the denominator of the fraction: 2n / 5 * 5 = 4 * 5, which simplifies to 2n = 20.

The Division Property of Equality states that when both sides of an equation are divided by the same nonzero number, the equality holds true. This property is used to remove coefficients and isolate n. Dividing both sides by the coefficient of n results in an equation with n as the only unknown, making it easy to solve for its value.

For example, consider the equation: 6n + 4 = 10. To isolate n, divide both sides by 6, the coefficient of n: (6n + 4) / 6 = 10 / 6, which simplifies to n + 2/3 = 5/3. Subtracting 2/3 from both sides gives n = 1.

Conquering the Enigma of Equations: Unveiling the Secrets of Isolating n

Embarking on a mathematical adventure, we venture into the captivating realm of solving equations. Our mission? To isolate the elusive variable n, revealing its hidden value. Along this path, we’ll uncover the tools and strategies that will empower us to conquer this algebraic enigma.

One potent weapon in our arsenal is the Addition and Subtraction Properties of Equality. These properties provide a powerful means of simplifying equations and bringing us closer to our goal.

Just as adding the same number to both sides of an equation leaves the equation unaltered, subtracting the same number from both sides also preserves equality. This concept is fundamental for isolating n.

Let’s unravel a simple example:

Suppose we encounter the equation: 3n + 5 = 14

To isolate n, we need to free it from the clutches of the numbers. Using the Subtraction Property of Equality, we subtract 5 from both sides:

3n + 5 - 5 = 14 - 5

Simplifying, we get:

3n = 9

Now, to complete the isolation, we employ the Division Property of Equality. Dividing both sides by 3, we finally liberate n:

3n / 3 = 9 / 3

Eureka! We’ve isolated n and determined its value:

n = 3

The Addition and Subtraction Properties of Equality are indispensable tools in our mathematical arsenal. They empower us to simplify equations, untether variables, and reveal their hidden secrets. Embrace their power and unlock the infinite possibilities of algebraic exploration!