Simplify Finding The Midpoint Of A Class In Frequency Tables
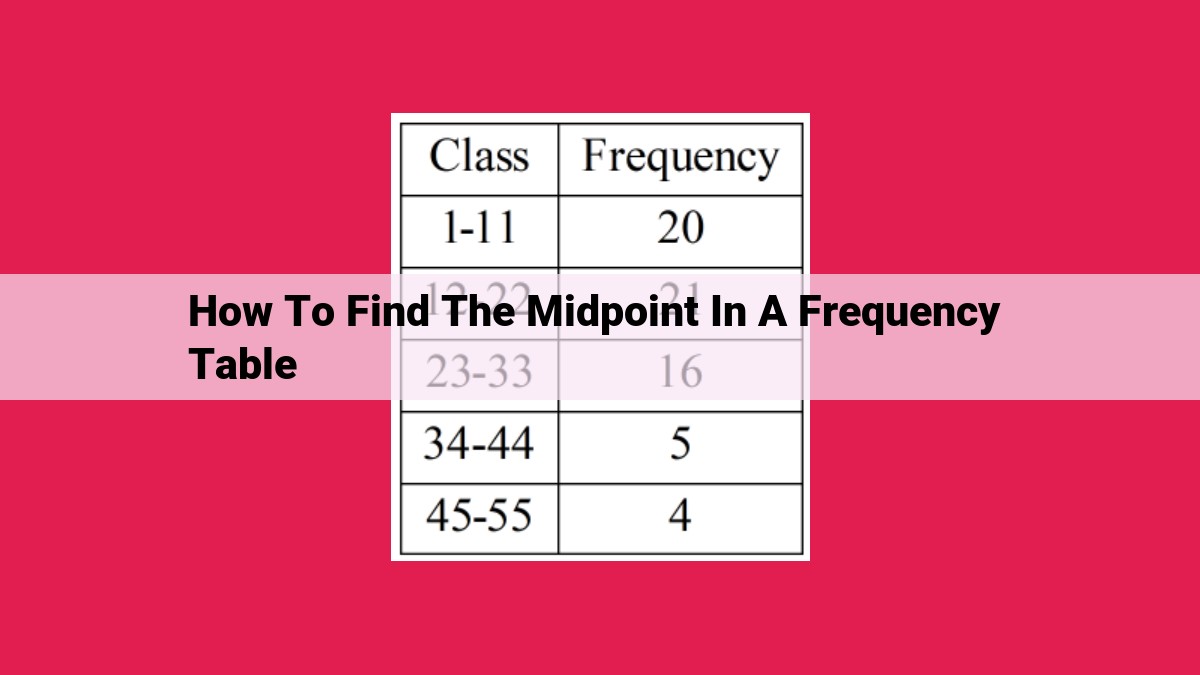
To find the midpoint of a class in a frequency table, determine the class boundaries by adding and subtracting half the class size from the upper and lower limits of the class. For example, if the upper limit is 10 and the lower limit is 5, and the class size is 5, the class boundaries are 12.5 and 2.5. The midpoint is then calculated by averaging the class boundaries, which in this case would be 7.5.
Unlocking the Secrets of Midpoint: A Comprehensive Guide for Statistical Analysis
In the realm of data analysis, unraveling the intricacies of midpoint holds immense significance. It serves as a fundamental concept upon which many other statistical measures hinge, providing valuable insights into real-world phenomena.
Midpoint represents the middle point of a range of values in a frequency table. Grasping this concept is paramount for comprehending the central tendencies and distributions of data, empowering researchers and analysts to draw insightful conclusions.
Understanding midpoint extends beyond mere calculation; it requires a nuanced comprehension of its relationship with other statistical measures, such as mean, median, mode, quartiles, and interquartile range. With this knowledge, statisticians can harness the power of midpoint to unearth hidden patterns and derive informed decisions.
By delving into this comprehensive guide, you will embark on an enlightening journey that unravels the secrets of midpoint. We will demystify its formula and explore how it fits into the context of frequency tables, providing you with a robust understanding of this pivotal statistical tool.
Understanding Midpoint
- Discuss the concept of midpoint and its relation to mean, median, mode, quartile, and interquartile range.
- Include the formula for calculating midpoint: (Upper limit + Lower limit) / 2.
Understanding Midpoint: A Statistical Journey
In the realm of statistics, the concept of midpoint occupies a pivotal position, providing a valuable statistical measure to explore the central value of a dataset. As you navigate this blog post, let’s delve into the fascinating world of midpoints, examining their relationship with other statistical concepts and discovering their practical significance.
The midpoint represents the halfway point between two values, often the upper and lower limits of a class interval in a frequency table. It serves as a measure of central tendency, alongside the familiar concepts of mean, median, and mode. Understanding the relationship between these statistical measures enhances our ability to analyze and interpret data.
The formula for calculating the midpoint is straightforward:
Midpoint = (Upper limit + Lower limit) / 2
This formula provides the exact value that divides the class interval into two equal parts based on frequency. It’s important to note that the midpoint is distinct from the mean, median, and mode, each of which represents different aspects of central tendency.
While the mean calculates the average value across all data points, the median identifies the middle value when the data is arranged in ascending order. The mode, on the other hand, represents the value that occurs most frequently. The quartile and interquartile range provide additional insights into the distribution of data.
The midpoint plays a crucial role in statistical analysis by facilitating comparisons between different classes or groups and providing a basis for constructing histograms and other graphical representations of data. Its versatility allows it to be applied across a wide range of fields, from life sciences to business and social sciences.
Understanding the concept of midpoint is essential for effective data analysis and interpretation. By grasping the relationship between the midpoint and other statistical measures, we can gain a deeper understanding of the distribution and characteristics of our data. This knowledge empowers us to make informed decisions based on reliable statistical information.
Frequency in Context
- Define frequency and its relevance in data analysis.
- Explain how to determine frequency from a frequency table.
Frequency in Context: A Journey to Understanding Data
In the realm of statistics, where data analysis unfolds, frequency emerges as a pivotal concept, orchestrating the dance of numbers to reveal patterns and insights. It paints a picture of how often specific values or intervals appear within a dataset.
Imagine yourself as a detective, meticulously examining a crime scene. The scattered evidence—the frequency of each piece of information—guides your search for the truth. Similarly, in data analysis, frequency unravels the secrets hidden within the labyrinth of numbers.
A frequency table serves as a treasure chest of this crucial information. Each row documents a specific interval of values, while the corresponding column reveals how many times that interval appears. By deciphering this table, you unlock the coded messages of your data.
For instance, a frequency table detailing the heights of students in a classroom might showcase a range of intervals from 48 inches to 60 inches. The frequency column adjacent to each interval would indicate the number of students within that height range. Armed with this data, you can begin to understand the distribution of student heights and identify patterns or outliers.
The significance of frequency extends far beyond mere observation. It lays the foundation for exploring more advanced statistical concepts, guiding you along the path to statistical mastery. So, embrace the role of a data detective and embark on a journey to uncover the power of frequency in statistical analysis.
Determining Class Size: A Key Step in Finding the Midpoint
Understanding the midpoint of a frequency table is crucial for statistical analysis. But before we dive into its calculation, let’s explore another essential concept: class size.
Defining Class Size
Class size represents the width of each interval in a frequency table. It determines the range of values that fall within each class. A smaller class size provides more detail, while a larger one groups values together for a broader overview.
Determining Class Size from a Frequency Table
To determine the class size, we use the following formula:
Class Size = (Upper Limit of Frequency Table - Lower Limit of Frequency Table) / Number of Classes
For example, consider a frequency table with the following data:
| Class Interval | Frequency |
|---|---|
| 10 – 20 | 15 |
| 20 – 30 | 20 |
| 30 – 40 | 25 |
In this case, the upper limit is 40, the lower limit is 10, and the number of classes is 3. Plugging these values into the formula, we get:
Class Size = (40 - 10) / 3 = 10
Therefore, the class size for this frequency table is 10.
Related Concepts and Their Role in Midpoint Calculation
In the realm of statistical analysis, a host of related concepts intertwine to paint a comprehensive picture of data distribution. Chief among them are mean, median, mode, quartiles, and interquartile range. Understanding their interplay can greatly aid in finding the midpoint within a frequency table.
The mean, or average, represents the aggregate of all data points divided by their count. It provides a central tendency of the data. The median, on the other hand, is the value that divides the data into two equal halves when arranged in ascending order. It’s a stronger indicator of the typical value compared to mean.
The mode is the most frequently occurring value in a dataset, often reflecting a dominant pattern. It can provide insights into the most common observation. Quartiles are values that divide the data into four equal parts. They can be used to assess the spread of data.
The interquartile range measures the dispersion of data between the third and first quartiles. A larger interquartile range indicates a wider spread.
By considering these related concepts, we can gain valuable clues about the midpoint’s location within the frequency table. For instance, if the mean and median are close together, it suggests that the data is spread relatively evenly around the midpoint. Conversely, a large difference between mean and median indicates a skewed distribution, with the midpoint possibly closer to one extreme.
Moreover, the quartiles can help estimate the midpoint’s range. If the interquartile range is small, the midpoint is likely to be relatively close to both the median and mean. If the interquartile range is large, the midpoint may be further removed from these central values.
By synthesizing these insights, we can effectively pinpoint the midpoint within the frequency table. It serves as a valuable reference point for further statistical analysis, providing a snapshot of the data’s central tendency.
Best Blog Post Outline: Find the Midpoint in a Frequency Table with Ease
Understanding Midpoint
In the realm of statistical analysis, midpoint holds great significance. It represents the average of the upper and lower limits of a class interval in a frequency table. By grasping the concept of midpoint, you can unlock a deeper understanding of statistical measures such as mean, median, mode, quartiles, and interquartile range. The formula for calculating midpoint is elegantly simple:
Midpoint = (Upper Limit + Lower Limit) / 2
Frequency in Context
In statistical analysis, frequency plays a crucial role. It refers to the number of occurrences of a particular value or range of values within a dataset. In a frequency table, each class interval represents a range of values, and the corresponding frequency indicates how often values within that range occur.
Determining Class Size
Before calculating the midpoint, we need to determine the class size, which is the width or span of each class interval. Class size is calculated as follows:
Class Size = Upper Limit - Lower Limit
Related Concepts and Midpoint
Understanding the relationship between midpoint and other statistical measures can aid in calculating midpoint effectively.
- Mean: The average of all values in a dataset.
- Median: The middle value when the dataset is arranged in ascending or descending order.
- Mode: The most frequently occurring value in a dataset.
- Quartiles: Divide a dataset into four equal parts.
- Interquartile Range: The difference between the upper and lower quartiles.
Examples and Applications
Let’s dive into practical examples to illustrate the calculation of midpoint.
Example 1:
Consider a class interval with an upper limit of 10 and a lower limit of 5. The midpoint can be calculated as:
Midpoint = (10 + 5) / 2 = 7.5
Example 2:
In a survey, the following frequency table shows the distribution of ages:
| Age Range | Frequency |
|---|---|
| 20-29 | 15 |
| 30-39 | 20 |
| 40-49 | 25 |
To find the midpoint of the age range 20-29, we calculate:
Midpoint = (29 + 20) / 2 = 24.5
Midpoint plays a vital role in various statistical applications, such as:
- Grouping Data: Midpoint helps group data into meaningful intervals for analysis.
- Central Tendency: Midpoint provides a measure of central tendency, along with mean and median.
- Statistical Analysis: Midpoint aids in statistical tests and inference, such as hypothesis testing.