Reversing The Order Of Integration: An Efficient Approach To Double Integrals

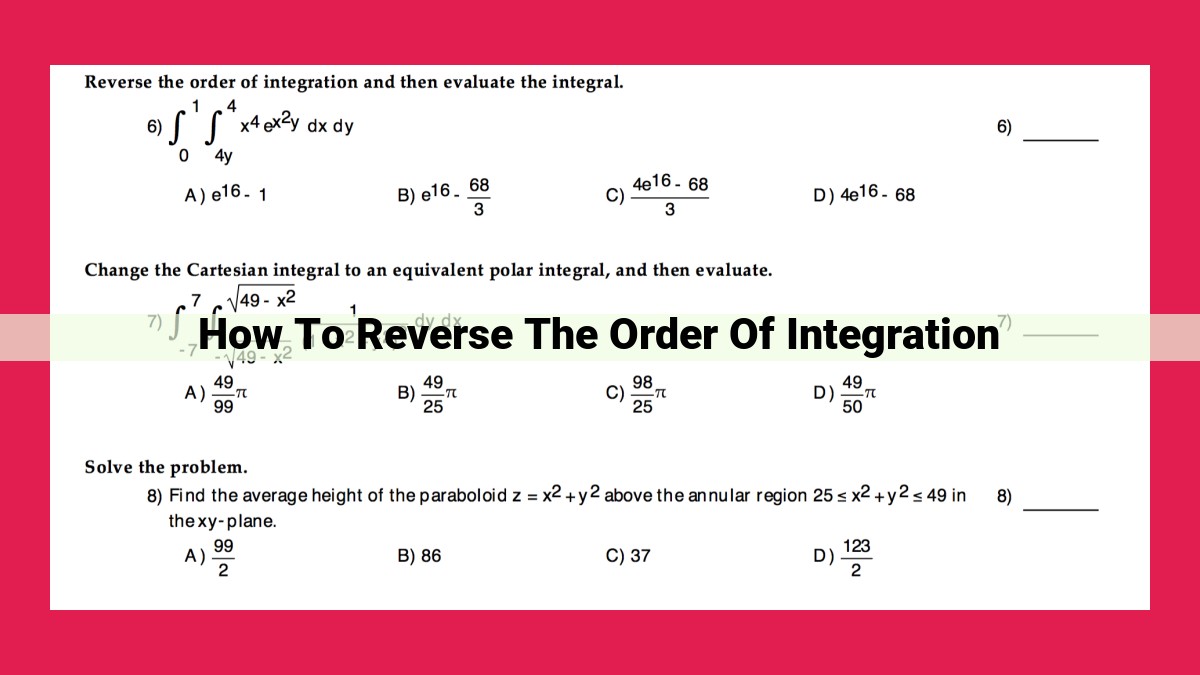
Reversing the order of integration involves changing the order in which the inner and outer integrals in a double integral are evaluated. It offers advantages in simplifying the integration process and is particularly useful when the limits of integration are easier to express in one order than the other. To reverse the order, the region of integration is described in terms of the other variable, and the absolute value of the Jacobian is used to account for the change in order. Conditions for reversing the order of integration include continuity of the function and the boundaries of the region. By following these steps, it becomes possible to evaluate double integrals more efficiently and intuitively.

Understanding Reversing the Order of Integration

• Explain the concept of reversing the order of integration in double integrals.

Understanding the Power of Reversing Order of Integration

When venturing into the realm of double integrals, understanding the concept of reversing the order of integration is crucial for unlocking its full potential. This technique involves switching the order in which you integrate a function over a region. By doing so, you can often simplify the integration process and obtain solutions more efficiently.

Embracing the Advantages of Reversed Integration

There are countless scenarios where reversing the order of integration offers significant advantages. For instance, it can:

• Eliminate the need for complex variable substitutions or partial derivatives.
• Reduce the number of calculations required, saving you time and effort.
• Improve the accuracy and efficiency of your integrations.

Exploring the Two Paths of Integration

To reverse the order of integration, you can choose between two approaches:

1. Integration with Respect to y First

This method involves integrating the function over the region with respect to y first. This is commonly used when the region is defined by horizontal lines.

2. Integration with Respect to x First

In this case, you integrate over the region with respect to x first. This is preferred when the region is defined by vertical lines.

The Change of Order of Integration Theorem

The change of order of integration theorem is the foundation upon which the technique rests. This theorem states that regardless of the order of integration, the value of the double integral remains the same.

The Absolute Value of the Jacobian: A Key Player

The absolute value of the Jacobian, denoted as |J|, plays a vital role in reversing the order of integration. It represents the change in the order of the variables and ensures that the integrated value is unaffected.

Essential Conditions for a Successful Reversal

For the reversal of order of integration to be valid, certain conditions must be met:

• The region of integration must be bounded.
• The function must be continuous over the region.
• The partial derivatives of the function must exist.

Mastering the Art with Example Problems

To solidify your understanding, try practicing with real-world example problems. These problems will showcase the practical applications of reversing the order of integration and boost your confidence in solving double integrals.

Advantages and Convenience of Reversing Integration

Reversing the order of integration in double integrals can offer significant benefits and simplify the integration process in many situations. Here’s why:

• Improved Integration: In certain cases, reversing the order of integration can lead to an integral that is easier to evaluate. By changing the order of integration, you may be able to factor out constants, eliminate nested integrals, or simplify the integrand.

• Feasibility: Reversing the order of integration can make a previously intractable integral comprehensible. Some integration techniques, such as Fubini’s Theorem, require reversing the order of integration to make the integral feasible.

• Applications to Specific Regions: Reversing the order of integration is particularly useful when integrating over non-rectangular regions. By switching the order of integration, you can align the limits of integration with the boundaries of the region, making the integration process more straightforward.

• Enhanced Geometrical Interpretation: Reversing the order of integration can provide a clearer geometrical interpretation of the region of integration. This can be especially helpful in visualizing the integration problem and understanding the relationship between the variables of integration.

In conclusion, reversing the order of integration in double integrals can greatly simplify the integration process, expand the range of problems that can be solved, and provide a more intuitive understanding of the region of integration. By understanding the advantages and convenience of reversed integration, you can unlock a powerful tool for solving double integrals efficiently and accurately.

Integration with Respect to y First

• Describe the process of integrating a function over a region with respect to y first, and provide examples of its applications.

Integration with Respect to y First: A Comprehensive Guide

When tackling double integrals, we have the flexibility to integrate with respect to either y first or x first. Integrating with respect to y first, known as the reversed order of integration, offers several advantages and can significantly simplify the integration process in certain situations.

Advantages of Integrating with Respect to y First:

• Improved readability: The integrated expression becomes more manageable and easier to understand.
• Simplified limits of integration: The limits of integration for y are often simpler and more straightforward.
• Efficient computation: Reversing the order can make the integration process more efficient, especially when the integrand is more complex with respect to x.

Steps for Integration with Respect to y First:

1. Identify the bounds of integration: Determine the range of y values for which the integration will be performed.
2. Integrate with respect to y: Perform the integral with respect to y, treating x as a constant.
3. Substitute the limits of integration: Replace the variable y with its lower and upper bounds in the integrated expression.
4. Evaluate: Calculate the final result of the integration.

Example Applications:

Consider the double integral:

∫∫R (x + y²) dA

Here, integrating with respect to y first yields:

∫[a,b] ∫[c,d] (x + y²) dy dx

This often results in simpler limits of integration and a more manageable integrand.

Conditions for Reversing Order of Integration:

To ensure the validity of reversing the order of integration, the following conditions must be met:

• The region of integration must be Type 1, where the boundaries are vertical lines and horizontal lines.
• The integrand must be continuous in the region of integration.
• The partial derivatives of the integrand with respect to x and y must be continuous in the region of integration.

Integrating with Respect to y First: Its Importance in Solving Double Integrals:

Integrating with respect to y first provides a powerful tool for solving double integrals, especially when the integrand is more complex with respect to x or when the limits of integration are simpler with respect to y. This technique can significantly enhance the efficiency and accuracy of the integration process, making it an essential technique for mastering double integrals.

Integrating with Respect to x First: Simplifying Double Integrals

In the realm of calculus, double integrals are powerful tools for evaluating the area, volume, or other properties of regions in the plane. However, sometimes the standard approach of integrating with respect to y first can lead to complex calculations. In such cases, reversing the order of integration can provide a simpler and more convenient solution.

When integrating a function over a region, we can choose to integrate with respect to either the x or y variable first. Integrating with respect to x first involves breaking the region into vertical strips and integrating the function along each strip with respect to y. This approach can be particularly useful when the lower and upper bounds of the function are functions of x.

Consider the example:

Evaluate the double integral:

$$\int_0^2\int_0^{x^2} e^{y^2}dy dx$$

Using the standard approach of integrating with respect to y first would result in:

$$\int_0^2\left[\int_0^{x^2} e^{y^2}dy\right] dx$$

This inner integral is not straightforward to evaluate. However, by reversing the order of integration, we can simplify the process:

$$\int_0^2\int_0^{x^2} e^{y^2}dy dx = \int_0^2\left[\int_y^2 e^{y^2}dx\right] dy$$

Now, the inner integral is a simple exponential function:

$$\int_y^2 e^{y^2}dx = e^{y^2}x\bigg|_{y}^2 = e^{y^2}(2-y)$$

Substituting this into the outer integral, we get:

$$\int_0^2\left[\int_y^2 e^{y^2}dx\right] dy = \int_0^2 e^{y^2}(2-y)dy$$

This integral is much easier to evaluate than the original.

In summary, integrating with respect to x first can simplify double integrals when the lower and upper bounds of the function are functions of x. By breaking the region into vertical strips and integrating along each strip with respect to y, we can often obtain more manageable and straightforward integrals.

Understanding the Change of Order of Integration Theorem

In the realm of calculus, we often grapple with integrals over complex regions. One powerful tool we have at our disposal is the change of order of integration theorem, which allows us to switch the order of integration in double integrals. This theorem is a game-changer, simplifying complex integrations and shedding light on the beauty of mathematical interdependencies.

Imagine a double integral where we integrate over a region that’s not so easily tamed. Reversing the order of integration can be like finding a hidden path that leads us to a simpler solution. The change of order of integration theorem says that as long as the region of integration is a type I or type II region, we can switch the order of integration without affecting the final value of the integral.

Preserving the Integrated Value

This theorem emphasizes one crucial aspect: conservation of the integrated value. No matter the order in which we integrate, the net result remains the same. This is a fundamental property that ensures the integrity of our calculations, regardless of the path we take.

Unlocking the Power of Reversal

The change of order of integration theorem opens up new possibilities for solving double integrals. By carefully considering the region of integration, we can choose the order that makes the integration process more manageable. In some cases, reversing the order can simplify complex integrals, while in others, it can provide a new perspective that leads to a quicker solution.

Remember: To ensure the validity of reversing the order of integration, the region of integration must be a type I or type II region. These regions are defined by boundaries that are either vertical or horizontal lines. By adhering to these conditions, we can confidently apply the change of order of integration theorem and unlock the full potential of this mathematical technique.

The Absolute Value of the Jacobian: A Gateway to Orderly Integration

In the realm of double integrals, there exists a pivotal concept that serves as a gateway to unraveling integration’s complexities: the absolute value of the Jacobian. This powerful tool empowers us to transpose the order of integration, offering a flexible and often advantageous approach to solving intricate integrals.

Imagine yourself embarking on an expedition through a dense forest. As you navigate its winding paths, you encounter a towering tree that blocks your way. Instead of hacking through the dense undergrowth, you spot a perfectly preserved trail just to the side. This trail, the absolute value of the Jacobian, provides an elegant passageway around the obstacle, allowing you to seamlessly continue your integration journey.

The absolute value of the Jacobian is a determinant, a mathematical construct that characterizes the transformation between two coordinate systems. In the context of reversing the order of integration, the Jacobian quantifies the geometric distortion caused by the transformation. By employing this value, we can ensure that the integrated result remains unchanged despite the order of integration.

The formula for the absolute value of the Jacobian is given by:

|J| = |∂(x,y)/∂(u,v)|

where |J| is the absolute value of the Jacobian, x and y represent the original coordinates, and u and v represent the transformed coordinates. The partial derivatives in the formula measure the rate of change between the two coordinate systems.

By understanding the role of the absolute value of the Jacobian, we gain the ability to reverse the order of integration judiciously. This technique proves particularly beneficial when one variable exhibits a more straightforward or convenient integration path. By transposing the order, we can align the integration process with the more tractable variable, simplifying the integration and expediting our journey towards the desired result.

Conditions for Reversing the Order of Integration

When considering reversing the order of integration in a double integral, it’s crucial to ensure that certain conditions are met to guarantee the validity of the process. These conditions serve as the bedrock upon which the reversal of integration rests.

Continuity and Partial Derivatives:

The functions involved in the integral must be continuous within the region of integration. Additionally, the partial derivatives of the functions with respect to the variables of integration (x and y) must also be continuous.

Non-Zero Determinant of the Jacobian:

The determinant of the Jacobian matrix, which arises from the transformation of variables in reversing the order of integration, must not be zero at any point within the region of integration. The Jacobian matrix is a matrix of partial derivatives, and its determinant measures the rate of change of the transformation. A non-zero determinant ensures that the transformation is non-degenerate and preserves the area elements.

Bounded Region:

The region of integration should be bounded, meaning it should have well-defined boundaries. This ensures that the integral remains finite and the limits of integration are clear.

Satisfying the Clairaut’s Theorem:

Clairaut’s theorem states that if the mixed partial derivatives of a function are continuous, then they are equal. This condition ensures that the order of differentiation does not affect the result. In the context of reversing integration, it implies that the integrand function must satisfy Clairaut’s theorem.

Reversing the Order of Integration: A Comprehensive Guide

Understanding the Concept

In the realm of calculus, double integrals involve integrating a function over two variables within a specific region. Sometimes, reversing the order of integration can simplify or improve the integration process. This blog post will delve into the concept of reversing the order of integration, explaining its advantages, outlining the processes, and providing practical examples.

Advantages and Convenience of Reversed Integration

Reversing integration can offer several benefits:

• It can make integration easier by reducing the number of steps or simplifying the integrand.
• It can help avoid convergence issues that may arise when integrating with respect to one variable first.

Integration with Respect to y First

In this method, we integrate the function with respect to y first, holding x constant. This approach is particularly useful when the integrand is simpler in terms of y.

Integration with Respect to x First

Alternatively, we can integrate with respect to x first, holding y constant. This method is preferred when the integrand is simpler in terms of x.

Change of Order of Integration Theorem

The Change of Order of Integration Theorem states that the value of a double integral is not affected by the order of integration, provided that certain conditions are met.

Absolute Value of the Jacobian

The absolute value of the Jacobian is a determinant that transforms the differential area element from one order of integration to the other.

Conditions for Reversing Order of Integration

To ensure the validity of reversing the order of integration, the following conditions must be met:

• The region of integration must be a type I or type II region.
• The function being integrated must be continuous on the region of integration.

Example Problems and Applications

Let’s illustrate the practical applications of reversing order of integration with an example:

Consider the double integral:

∬_R (x^2 + y^2) dx dy

Over the region:

R = {(x, y) | 0 ≤ x ≤ 1, x^2 ≤ y ≤ 1}

Original Order of Integration:

Integrating with respect to x first:

∫[0,1] ∫[x^2,1] (x^2 + y^2) dy dx

Reversed Order of Integration:

Integrating with respect to y first:

∫[0,1] ∫[x^2,1] (x^2 + y^2) dx dy

In this case, reversing the order of integration simplifies the integration process because the integral with respect to x is now a simple linear integral.

Reversing the order of integration can be a powerful tool for simplifying double integrals. By understanding the concept, advantages, processes, and conditions involved, you can effectively utilize this technique to solve integrals more efficiently and accurately.