Real Solutions In Mathematics: Understanding The Basics

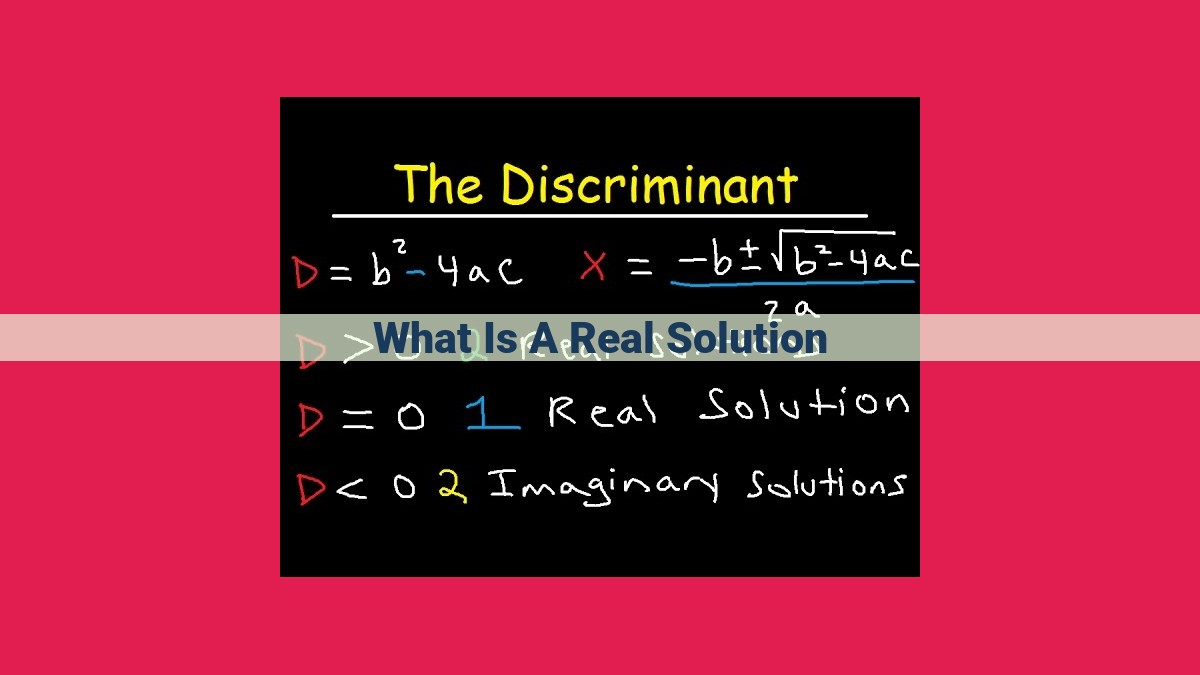
A real solution is a solution to an equation or inequality that is a real number, which can be represented on the number line. Real numbers include integers, rational numbers (fractions), and irrational numbers (non-repeating, non-terminating decimals). In contrast, complex solutions involve imaginary numbers and extend the concept of real numbers. Complex solutions are not considered real solutions because they cannot be represented on the number line. Understanding real solutions is crucial for solving mathematical problems and helps avoid common errors.

What is a Real Number and a Real Solution?

• Define a real number as a number that can be represented on the number line.
• Introduce the concept of a real solution as a solution to an equation or inequality that is a real number.

Real Numbers and Real Solutions: The Cornerstones of Mathematical Problem-Solving

Mathematics is the language of the universe, and numbers are its alphabet. Among the vast array of numbers, real numbers hold a special place, representing the cornerstone of our mathematical understanding. They are the numbers that we encounter in our everyday lives, the numbers we use to count objects, measure distances, and calculate quantities.

What is a Real Number?

A real number is any number that can be represented on a number line. This includes whole numbers (integers), such as 1, 2, and -5; fractions (rational numbers), such as 1/2, 3/4, and -1.25; and non-repeating, non-terminating decimals (irrational numbers), such as π, √2, and -0.1010010001….

Real Solutions

In mathematics, we often seek solutions to equations and inequalities. A real solution is a solution that is a real number. Real solutions can be found for a wide variety of equations and inequalities, including linear equations, quadratic equations, and even some trigonometric equations.

Complex Numbers: A Brief Digression

While real numbers form the foundation of our mathematical system, there are also numbers that lie beyond the realm of real numbers. These are known as complex numbers. Complex numbers are numbers that have both a real part and an imaginary part. The imaginary part is a number that is multiplied by the imaginary unit, i, which is defined as the square root of -1. Complex numbers are used to solve certain types of equations that do not have real solutions, such as x² + 1 = 0.

Real Solutions vs. Complex Solutions

The distinction between real solutions and complex solutions is crucial in mathematics. Real solutions are numbers that can be represented on the number line, while complex solutions are numbers that involve the imaginary unit. It is important to note that complex solutions are not considered real solutions.

Examples of Real Solutions

• The solution to the equation x + 2 = 5 is x = 3, which is a real number.
• The solution to the inequality x > 1 is all real numbers greater than 1, which is a set of real numbers.

Examples of Complex Solutions

• The solution to the equation x² + 1 = 0 is x = ±i, which is a complex number.
• The solution to the inequality x² < -1 has no real solutions, but the complex solution is x = ±i.

Understanding real solutions is essential for solving a wide range of mathematical problems. It allows us to find solutions that are meaningful and relevant to the real world. By distinguishing between real solutions and complex solutions, we can avoid common errors and enhance our problem-solving abilities. Real solutions are the foundation of our mathematical understanding and the key to unlocking a deeper appreciation of the beauty and power of mathematics.

Types of Real Numbers:

• Explain that real numbers include integers (whole numbers), rational numbers (fractions), and irrational numbers (non-repeating, non-terminating decimals).
• Provide examples of each type.

Types of Real Numbers: Unraveling the Number Spectrum

In the realm of mathematics, real numbers reign supreme, encompassing a vast spectrum of values that we encounter daily. These numbers paint the canvas upon which we solve equations, performing calculations, and make sense of the world around us. But what exactly are real numbers, and how do they differ?

Real numbers can be broadly categorized into three distinct groups: integers, rational numbers, and irrational numbers.

Integers: The cornerstone of our number system, integers represent the whole numbers we use to count objects and perform basic arithmetic operations. They stretch infinitely across the number line, encompassing all positive and negative whole numbers, as well as zero.

Rational Numbers: Dwelling between the integers, rational numbers are those that can be expressed as a fraction of two integers. They emerge when we divide one integer by another, creating numbers like 1/2, -3/4, or 5.6 (using decimal notation). Rational numbers occupy a dense space on the number line, filling the gaps between integers.

Irrational Numbers: Evading both integer and rational domains, irrational numbers cannot be expressed as a fraction of integers. They possess an enigmatic quality: they are non-repeating and non-terminating decimals. Pi (π), the ratio of a circle’s circumference to its diameter, is a prime example of an irrational number. Its unending decimal expansion reveals the elusive nature of these numbers.

Understanding the types of real numbers empowers us to navigate the mathematical world with precision. It allows us to identify the appropriate solutions to equations, unravel the mysteries of complex calculations, and appreciate the intricate tapestry of our numerical system.

Real Numbers: The Foundation of Numerical Solutions

In the vast ocean of mathematics, real numbers stand as a cornerstone, representing quantities that can be depicted on the number line. From simple integers to complex decimals, real numbers play a pivotal role in solving a wide range of equations and inequalities.

Types of Real Numbers

Real numbers encompass a diverse family of number systems, including:

• Integers: Whole numbers like -3, 0, and 5

• Rational Numbers: Fractions such as 1/2, -3/4, and 0.75

• Irrational Numbers: Non-repeating, non-terminating decimals like π (3.14…) and √2 (1.41…)

Complex Numbers: A Glimpse Beyond

While real numbers form a sturdy foundation, complex numbers venture into a more ethereal realm. These numbers possess both real and imaginary components, introducing a fascinating dimension to the mathematical landscape.

Complex numbers extend the concept of real numbers, allowing us to find solutions to certain equations that would otherwise remain elusive. For instance, x² + 1 = 0 has no real solutions, but the complex solution is the imaginary number i (with the property that i² = -1).

However, it’s important to note that complex solutions, despite their mathematical validity, are not considered real solutions. They reside outside the confines of the real number line.

Real Solutions vs. Complex Solutions

The distinction between real and complex solutions is crucial. Real solutions are those that can be represented on the number line, while complex solutions involve imaginary numbers and fall outside its domain.

Examples of Equations and Inequalities

Real Solutions:

• x + 2 = 5 (x = 3)

• x² – 4 = 0 (x = 2 or x = -2)

• |x| = 3 (x = 3 or x = -3)

No Real Solutions:

• x² + 1 = 0 (complex solution: x = i)

• |x| < 0 (no real solution)

Understanding real solutions is pivotal for solving mathematical problems effectively. By grasping this concept, we can avoid common errors and enhance our problem-solving abilities. While complex numbers extend the boundaries of numerical solutions, their distinction from real solutions remains a fundamental pillar of mathematical knowledge.

Real Solutions vs. Complex Solutions: Unraveling the Mystery

In the realm of mathematics, numbers play a pivotal role in unraveling the complexities of the world around us. Among these numbers, real numbers hold a special place, representing the quantifiable aspects of our physical universe. Real solutions, therefore, are solutions to equations or inequalities that can be represented on the number line.

In contrast, complex numbers introduce a new dimension to the world of numbers. Unlike real numbers, complex numbers include imaginary numbers, denoted by the symbol i or j. Imaginary numbers are numbers that, when squared, produce a negative result. This peculiar property allows complex numbers to solve equations that have no real solutions.

Complex solutions are solutions to equations or inequalities that involve imaginary numbers. It’s important to note that complex solutions are not considered real solutions since they cannot be represented on the number line.

To illustrate the distinction between real and complex solutions, let’s consider the equation x^2 + 1 = 0. This equation has no real solutions because the square of any real number is always positive or zero. However, the equation has two complex solutions, x = i and x = -i. These complex solutions allow us to solve the equation even though there are no real solutions.

Understanding the difference between real and complex solutions is crucial for solving mathematical problems and avoiding common errors. By recognizing that some equations may not have real solutions and require complex solutions, we can broaden our thinking and explore a wider range of mathematical possibilities.

Examples of Equations and Inequalities with Real Solutions

Understanding real solutions is vital in mathematics, as they represent numbers that can be plotted on the number line. To solidify this concept, let’s explore some examples of equations and inequalities that produce real solutions.

Equations

• Linear Equation: x + 5 = 10
  > Solving this equation gives x = 5, which is a real number.
• Quadratic Equation: x^2 – 4 = 0
  > Using the quadratic formula, we find x = 2 and x = -2, both of which are real.

Inequalities

• Linear Inequality: 2x – 1 < 7
  > Solving this inequality, we get x < 4, which represents all real numbers less than 4.
• Quadratic Inequality: x^2 + 2x + 1 > 0
  > This quadratic inequality has no real solutions because (x + 1) is always positive.

The key to identifying real solutions is that they must be numbers that can be represented on the number line. In all the above examples, the solutions are real numbers that can be plotted on a line.

Examples of Equations and Inequalities without Real Solutions

In the realm of mathematics, not all equations and inequalities can be solved with real numbers. Some require a different approach, venturing into the world of complex numbers. These equations and inequalities, without real solutions, provide an interesting challenge for math enthusiasts.

Consider the equation x^2 + 1 = 0. This equation does not have any real solutions. Mathematicians have discovered that there are numbers called imaginary numbers, represented by the symbol i, which have the unique property of i^2 = -1. Using imaginary numbers, we can find solutions to this equation in the form of x = ±i. These solutions are not real numbers because they cannot be represented on the number line.

Another example is the inequality x^2 < -1. This inequality also has no real solutions. The square of any real number is always non-negative. Therefore, there is no real number that can satisfy this inequality.

Complex numbers come into play when solving these types of equations and inequalities. When an equation or inequality involves the square root of a negative number, we introduce the concept of the imaginary unit, denoted by the symbol i. This allows us to extend the concept of real numbers to include numbers with both real and imaginary parts.

By using complex numbers, we can find solutions to equations and inequalities that do not have real solutions. For example, the equation x^2 + 1 = 0 has two complex solutions, x = i and x = -i. Similarly, the inequality x^2 < -1 has an infinite number of complex solutions, which are all numbers of the form x = a + bi, where a and b are real numbers and b ≠ 0.

Understanding the concept of real solutions and their limitations is crucial for problem-solving in mathematics. It helps us recognize when equations or inequalities cannot be solved with real numbers and when complex numbers must be introduced. This knowledge enhances our problem-solving abilities and allows us to tackle a wider range of mathematical challenges.