Unraveling The Intricacies Of Radioactive Decay: The Decay Constant, Half-Life, And Its Applications

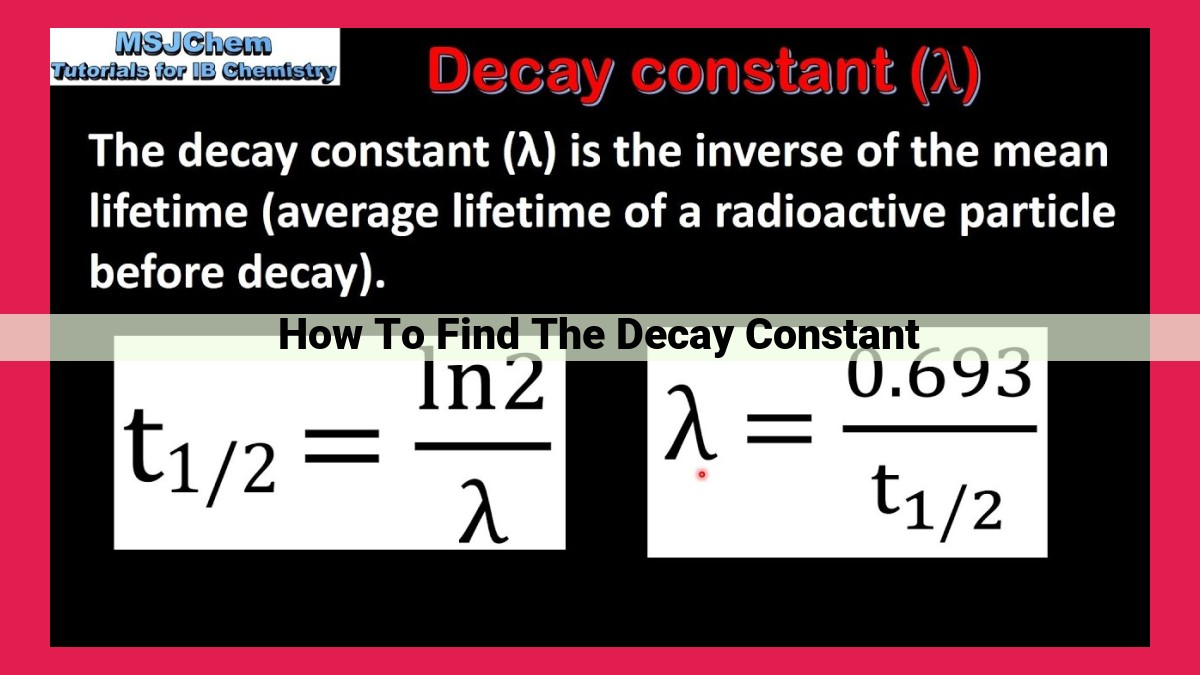
Understanding radioactive decay is crucial for analyzing the behavior of unstable atomic nuclei. The decay constant (λ) is a key parameter that represents the probability of decay per unit time. It is inversely related to the half-life (t1/2), the time it takes for half of a sample to decay. The relationship between λ and t1/2 is given by λ = (ln 2) / t1/2. The exponential decay equation, N = N0e^(-λt), can be used to calculate the decay constant based on the initial and final amounts of radioactive atoms (N0 and N, respectively). Understanding the decay constant allows scientists to predict and analyze the behavior of radioactive substances in various applications, such as nuclear medicine, environmental monitoring, and dating techniques.

Radioactive Decay: A Journey of Atomic Transformation

Unstable Atoms and the Quest for Stability

The atomic world is a dynamic realm, where atoms strive for stability. However, some atoms are born with an imbalance within their nuclei, rendering them unstable. These restless atoms embark on a journey of transformation known as radioactive decay, where they emit radiation in an attempt to achieve a more stable state.

Understanding the Language of Radioactive Decay

Radioactive decay is a fascinating process that can be analyzed using two key metrics: the decay constant and half-life. The decay constant, denoted by the Greek letter lambda (λ), represents the probability of an unstable atom decaying per unit time. The shorter the half-life, the more rapidly the atom undergoes decay.

The Decay Constant: A Measure of Radioactive Activity

The decay constant provides a direct measure of the rate of radioactive decay. It is the fraction of unstable atoms in a sample that decay per second. A radioactive substance with a large decay constant undergoes rapid decay, while one with a small decay constant experiences a slower decay rate.

The Half-Life: A Time Capsule of Change

The half-life (t1/2) is another crucial parameter in understanding radioactive decay. It is the time it takes for half of the unstable atoms in a sample to decay. This concept is analogous to flipping a coin – each flip represents a radioactive atom, and the half-life is the number of flips it takes to obtain half heads and half tails.

Radioactive Decay

• Explain the process of radioactive decay, where unstable nuclei release energy to become more stable.
• Introduce related concepts of half-life (time taken for half of a sample to decay) and decay constant (probability of decay per unit time).

Radioactive Decay: Unraveling the Mysteries of Unstable Nuclei

Imagine a world where everything is in constant flux, where elements transform and energy is released as a result of unstable atomic nuclei. This fascinating phenomenon is known as radioactive decay. Understanding the intricacies of radioactive decay, including the decay constant and half-life, is crucial for unraveling the mysteries of unstable nuclei.

The Journey of Radioactive Decay

Radioactive decay is the process by which unstable atomic nuclei release energy in order to achieve a more stable state. During radioactive decay, particles or radiation are emitted from the nucleus, transforming the atom into a different element. Two key concepts associated with radioactive decay are half-life and decay constant.

The Half-Life: Nature’s Clock for Decay

Half-life is the time it takes for half of a sample of radioactive atoms to decay. It essentially measures the rate at which the unstable atoms transform. A shorter half-life indicates a faster decay rate, while a longer half-life signifies a slower decay rate.

The Decay Constant: Measuring the Probability of Decay

The decay constant, symbolized by the Greek letter lambda (λ), represents the probability of decay per unit time. It describes the rate at which radioactive atoms spontaneously decay. A larger decay constant corresponds to a higher probability of decay and, consequently, a shorter half-life.

Linking the Decay Constant and Half-Life

The decay constant and half-life are inversely related. A shorter half-life implies a larger decay constant, while a longer half-life corresponds to a smaller decay constant. This relationship is mathematically expressed by the equation: λ = (ln 2) / t1/2, where ln 2 is the natural logarithm of 2.

The Exponential Decay Equation: Tracking the Decline

The exponential decay equation, N = N0e^(-λt), describes how the number of radioactive atoms (N) decreases over time (t). N0 represents the initial number of atoms, and e is the base of the natural logarithm. The decay constant (λ) determines the rate of decay.

Calculating the Decay Constant

The decay constant can be calculated using either the half-life equation or the exponential decay equation. When using the half-life equation, λ = (ln 2) / t1/2. When utilizing the exponential decay equation, the decay constant is calculated as λ = -(ln(N / N0)) / t. The condition N0 > N must be met when using the exponential decay equation.

Understanding radioactive decay, the decay constant, and half-life is essential for unraveling the behavior of unstable nuclei. These concepts play a crucial role in applications such as nuclear power generation, medical imaging, and radioactive waste management. By grasping the intricate relationship between the decay constant and half-life, we gain a deeper appreciation for the transformative power of radioactive decay in our world.

Decay Constant: A Measure of Radioactive Decay Rate

In the realm of radioactivity, understanding the decay constant is crucial for unraveling the mysteries of unstable atomic nuclei. The decay constant, denoted as λ (lambda), serves as a quantifier of how rapidly a radioactive substance undergoes decay, providing a measure of its decay rate per unit time.

Visualize radioactive nuclei as tiny, unstable entities, eager to release excess energy and attain stability. Each nucleus possesses a unique probability of disintegrating during a given time interval. The decay constant captures this probability, offering a window into the intrinsic instability of the atom.

Inverse Relationship with Half-Life: A Dance of Time and Decay

The decay constant and half-life (t1/2) share an intriguing inverse relationship. Half-life, as its name suggests, represents the time it takes for half of a radioactive sample to decay. A shorter half-life implies a more rapid decay, resulting in a larger decay constant. Conversely, a longer half-life corresponds to a smaller decay constant.

Imagine two radioactive substances, A and B. Substance A boasts a shorter half-life than substance B. This implies that substance A’s nuclei are more eager to decay, releasing their excess energy more rapidly. Consequently, substance A possesses a larger decay constant compared to substance B.

So, while the half-life measures the passage of time until decay, the decay constant quantifies the inherent instability that governs the decay process. Together, they provide a comprehensive understanding of radioactive decay, aiding scientists in unraveling the secrets of unstable atomic nuclei.

Half-Life: The Key to Understanding Radioactive Decay

When it comes to the fascinating world of radioactivity, understanding half-life is crucial. Half-life, denoted as t1/2, represents the time it takes for half of a radioactive substance to decay. It’s like the radioactive clock that tells us how long it takes for a collection of unstable atoms to settle into a more stable state.

Here’s a simplified analogy: Imagine a group of radioactive atoms as a room full of people with unstable energy levels. If the half-life is a minute, it means that in just one minute, half of the people in that room will release their excess energy and become more stable. The other half remains unstable, waiting for their turn to decay.

Fascinatingly, the shorter the half-life, the more unstable the atoms are. Think of it as a ticking clock; a shorter half-life means the clock is ticking faster, indicating that the atoms are more eager to shed their excess energy and reach stability.

The Inverse Relationship: A Tale of Decay and Time

The relationship between half-life and decay constant (λ) is inversely proportional. This means that as the half-life decreases (becomes shorter), the decay constant increases (becomes larger). It’s like a seesaw: when one end goes up, the other goes down.

Imagine a scenario where you have two radioactive samples, Sample A and Sample B. Sample A has a half-life of 10 minutes, while Sample B has a half-life of 5 minutes. According to our inverse relationship, Sample B will have a larger decay constant than Sample A. This means that Sample B’s atoms are more likely to decay in a given amount of time compared to Sample A.

Understanding this relationship is essential for analyzing and predicting radioactive decay. Scientists use it to calculate the decay constant, determine the amount of time it will take for a sample to decay to a certain level, and estimate the age of radioactive materials.

The Inverse Relationship between Decay Constant and Half-Life

In the realm of radioactivity, understanding decay constant and half-life is crucial for unraveling the mysteries of nuclear decay. These concepts hold the key to comprehending the behavior of unstable atomic nuclei as they strive to attain stability.

Inverse Relationship Unveiled

The decay constant, denoted by the Greek letter lambda (λ), represents the probability of radioactive decay per unit time. Imagine a radioactive sample like a collection of impatient atoms, eager to shed their excess energy and transform into a more stable form. The decay constant measures how quickly these atoms make their transition.

On the other hand, half-life (t₁/₂) is the time it takes for half of the radioactive atoms in a sample to undergo decay. Think of this as a cosmic countdown, where half of the restless atoms surrender to the allure of stability within a specific time frame.

Remarkably, these seemingly independent concepts are intertwined in an inverse relationship. A shorter half-life implies a larger decay constant, and vice versa. This means that atoms with a shorter half-life are more prone to decay, while those with longer half-lives take their time, releasing their energy at a slower pace.

The Equation Connecting the Two

The mathematical equation that governs this inverse relationship is:

λ = (ln 2) / t₁/₂

Where:

• λ is the decay constant
• t₁/₂ is the half-life

This equation serves as a bridge between the two concepts, revealing the intimate connection between the rate of decay and the time it takes for it to occur.

Exponential Decay Equation

• Introduce the exponential decay equation: N = N0e^(-λt).
• Define and explain each variable in the equation.

Understanding the Exponential Decay Equation in Radioactive Decay

In the fascinating world of nuclear physics, radioactive decay plays a crucial role in phenomena ranging from nuclear power plants to medical imaging. Understanding the intricate nature of radioactive decay requires us to delve into concepts like decay constant and half-life. But what’s the key that unlocks the secrets hidden within these abstract terms? It’s the exponential decay equation.

The exponential decay equation, expressed as N = N0e^(-λt), provides a mathematical framework for modeling the rate at which radioactive atoms decay. It’s a fundamental equation in nuclear physics, capturing the essence of radioactive decay in an elegant and precise manner. Let’s dissect this equation to unravel its meaning:

• N represents the number of radioactive atoms remaining at time t.
• N0 denotes the initial number of radioactive atoms at time 0.
• e is the **base of the natural logarithm,* approximately equal to 2.71828.
• λ represents the decay constant,* a measure of the **probability of decay per unit time.
• t is the elapsed time since the initial measurement.

The exponential decay equation embodies the concept of radioactive decay. As time progresses, the number of radioactive atoms decreases exponentially. The rate of decay is directly proportional to the decay constant λ. A larger decay constant signifies a higher decay rate, resulting in a shorter half-life. Conversely, a smaller decay constant corresponds to a slower decay rate and a longer half-life.

This equation proves invaluable in calculating and analyzing radioactive decay in various applications. From determining the age of archaeological artifacts to predicting the useful lifespan of radioactive isotopes in medical treatments, the exponential decay equation empowers scientists and researchers with a powerful tool.

Radioactive Decay: Unraveling the Mysteries of Unstable Atoms

Imagine a universe where matter is constantly evolving, not in a rapid burst, but in a gradual dance of transformation. This is the world of radioactive decay, a fundamental process where unstable atomic nuclei release energy in the form of radiation to achieve stability.

Understanding the Puzzle of Radioactive Decay

Radioactive decay is not a random event but a highly predictable process governed by two crucial concepts: decay constant and half-life. The decay constant, denoted by the Greek letter lambda (λ), measures the probability of an atom decaying per unit time. The shorter the half-life (t1/2), the more rapidly an atom decays, and the larger the decay constant; the longer the half-life, the slower the decay, and the smaller the decay constant.

Calculating the Decay Constant: Unveiling the Unseen

To determine the decay constant, scientists have devised two methods: the half-life equation and the exponential decay equation. The half-life equation is a simple yet powerful formula that relates the decay constant to the half-life:

λ = ln(2) / t1/2

For example, if an element has a half-life of 100 days, its decay constant would be:

λ = ln(2) / 100 days ≈ 0.00693 per day

Alternatively, the exponential decay equation provides a versatile tool for calculating the decay constant:

N = N0 * e^(-λt)

where N is the number of radioactive atoms at time t, N0 is the initial number of atoms, and e is the base of the natural logarithm. This equation is particularly useful when the initial number of atoms (N0) is greater than the number of atoms at a later time (N).

Applications of the Decay Constant: Unlocking Secrets and Solving Mysteries

The decay constant is an indispensable tool in various fields, from archaeology to medicine. In archaeology, measuring the decay of radioactive isotopes in ancient artifacts helps determine their age. In medicine, the decay constant of radioactive isotopes enables scientists to calculate the dose of radiation needed for medical imaging and cancer treatment.

Understanding radioactive decay is not merely an academic pursuit but a vital aspect of our world. It empowers us to unravel the mysteries of the cosmos, solve historical enigmas, and harness the potential of nuclear energy. So next time you hear the term “radioactive decay,” remember the intricate dance of instability and transformation, and the power of the decay constant that guides its path.