Master The Art Of Radical Subtraction: A Comprehensive Guide

To subtract radicals, start by isolating any radicals with the same radicand (number inside the square root). Subtract their coefficients directly. For different radicands, find the least common multiple (LCM) of the radicands, multiply the radicals by expressions that make them similar, and then subtract the coefficients. If the denominator contains a radical, multiply and divide by its conjugate to rationalize it. Conjugates are radical expressions that differ only in the sign between the terms. Multiplying and dividing by the conjugate can simplify radical expressions by eliminating irrational numbers in the denominator.

Radical Subtraction: A Comprehensive Guide for Beginners

In the realm of mathematics, we often encounter the concept of radicals, which are used to represent roots of numbers. Subtracting radicals is a crucial skill for simplifying and combining radical expressions.

Subtracting Radicals with Same Radicand

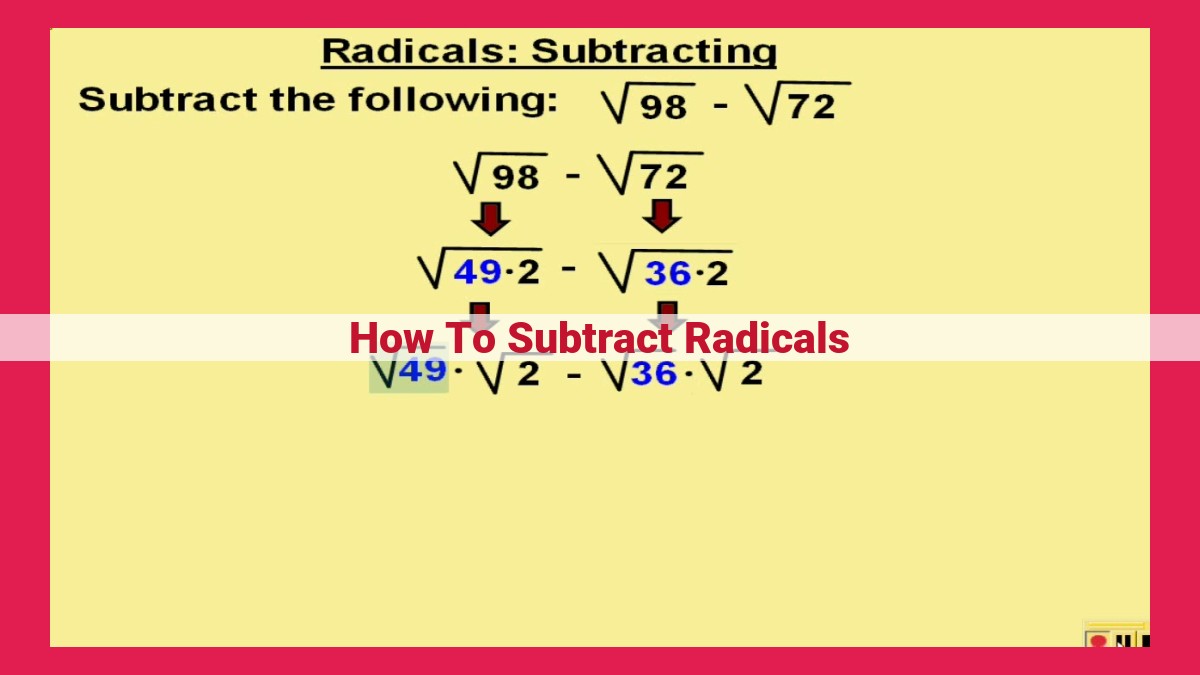
When the radicals have the same number inside the square root symbol, it’s a straightforward subtraction. Simply add or subtract the coefficients of the radicals, much like we do with other algebraic terms.

For example, if we have 5√2 – 3√2, we can simply subtract the coefficients to get 2√2.

Subtracting Radicals with Different Radicands

Things get a bit more interesting when the radicals have different numbers inside the square root symbol. In such cases, we introduce the concept of multiplying radicals to obtain similar terms.

We first find the least common multiple (LCM) of the radicands and use it to multiply the radicals. This creates a common denominator that allows us to perform subtraction.

Rationalizing the Denominator

If we have a fraction with a radical denominator, we can often simplify it by rationalizing the denominator. This involves multiplying and dividing by the conjugate of the denominator.

The conjugate of a radical expression is an expression that differs only in the sign between the terms. Multiplying the radical by its conjugate gives us a rational number.

For example, if we have 1/√2, we can rationalize the denominator by multiplying and dividing by √2:

1/√2 * √2/√2 = √2/2

Simplifying Conjugates

Conjugates play a crucial role in simplifying radical expressions. Multiplying and dividing by a conjugate can simplify expressions by eliminating irrational numbers in the denominator.

Remember, conjugates always give us rational numbers when multiplied.

Mastering radical subtraction requires understanding same radical, different radical, rationalizing the denominator, and conjugates. These techniques empower us to manipulate and simplify radical expressions efficiently, unlocking doors to deeper mathematical concepts.

Subtracting Radicals with Same Radicand

In the realm of mathematics, radicals are used to represent the roots of numbers. When we want to subtract radicals that have the same radicand, that is, the same number inside the square root symbol, we can directly add or subtract their coefficients.

Embracing Simplicity

When dealing with radicals that share the same root, the process of subtraction becomes a breeze. Just as you would add or subtract regular numbers, you can perform the same operations on the coefficients of the radicals. Consider the following example:

√16 - √9 = 4 - 3 = **1**

It’s as simple as that!

Illustrating the Concept

To further solidify our understanding, let’s explore a few more examples:

• √25 – √16 = 5 – 4 = **1
• √81 – √49 = 9 – 7 = **2
• 3√27 – 2√12 = 3√9 – 2√4 = 9 – 8 = **1

As you can see, subtracting radicals with the same radicand is a straightforward process. It’s all about recognizing the common root and performing operations on the coefficients accordingly.

Subtracting Radicals with Different Radicands

Envision yourself in a mathematical realm where numbers dance and symbols whisper secrets. Today, we’ll delve into the captivating world of radical subtraction, where we’ll unravel the mysteries of subtracting radicals with different radicands.

Radicals, those enigmatic symbols, represent the roots of numbers. But what happens when we want to subtract radicals that don’t have matching radicands? It’s like trying to compare apples and oranges! Fortunately, we have a secret weapon: multiplication.

Multiplying Radicals

Just as multiplying fractions with different denominators requires us to find a common denominator, so too does subtracting radicals with different radicands. The key lies in finding the least common multiple (LCM) of the radicands.

The LCM is the smallest number that both radicands divide into evenly. Once we have the LCM, we multiply both radicals by the appropriate factors to ensure they have the same radicand.

For example, to subtract √2 – √3, we first find the LCM of 2 and 3, which is 6. Then, we multiply √2 by √3 / √3 and √3 by √2 / √2 to obtain:

√2 * √3 / √3 – √3 * √2 / √2 = (√6 – √6) / 3 = 0

Rationalizing the Denominator

Sometimes, we encounter radicals in the denominator of a fraction. This can be a bit tricky, but it’s where our understanding of conjugates comes in handy.

Conjugates are radical expressions that differ only in the sign between the terms. Multiplying and dividing a radical by its conjugate simplifies the expression.

For instance, to rationalize the denominator of 1 / (√2 – 1), we multiply and divide by the conjugate (√2 + 1):

1 / (√2 – 1) * (√2 + 1) / (√2 + 1) = (√2 + 1) / (2 – 1) = √2 + 1

Simplifying Conjugates

Conjugates play a significant role in simplifying radical expressions. Multiplying and dividing by a conjugate can eliminate irrational numbers in the denominator. This makes it easier to work with and compare radical expressions.

Remember, the key to subtracting radicals with different radicands lies in finding the LCM, multiplying the radicals to obtain similar terms, and using conjugates to simplify expressions. With these techniques, you’ll be a master of this mathematical enigma!

Rationalizing the Denominator

• Discuss the issue of subtracting radicals with fractions in the denominator.
• Explain the concept of rationalizing the denominator by multiplying and dividing by the conjugate.
• Provide examples to demonstrate the process.

Rationalizing the Denominator: Making Roots Respectful

When dealing with radical expressions, we sometimes encounter fractions where the denominator contains a stubborn root. This unruly denominator needs to be tamed, a process known as rationalizing the denominator. It’s like teaching the denominator some manners, making it more civilized and easier to work with.

To rationalize the denominator, we employ a clever trick: we multiply and divide by the conjugate of the denominator. The conjugate is a radical expression that’s the same as the denominator except that the sign between the terms is flipped. For example, if the denominator is √2 – √3, its conjugate is √2 + √3.

Multiplying and dividing by the conjugate is like multiplying and dividing by 1; it doesn’t change the value of the fraction. But here’s the magic: the result is a fraction with a rational denominator, meaning no more roots!

Let’s illustrate this with an example. Suppose we have the fraction:

1 / (√2 - √3)

We multiply and divide by the conjugate, √2 + √3:

(1 / (√2 - √3)) * (√2 + √3 / √2 + √3) = (√2 + √3) / (2 - 3) = -(√2 + √3)

Voilà! The denominator is now a neat and tidy -1, and the fraction simplifies to -(√2 + √3).

Rationalizing the denominator is a valuable technique that unlocks a world of possibilities when working with radicals. It allows us to add, subtract, and compare radical expressions with ease, and it’s a key step in solving many radical equations and inequalities.

Simplifying Conjugates: The Magic of Eliminating Irrationals

In the realm of radical subtraction, where numbers dance under the square root symbol, the concept of conjugates emerges as a powerful tool for simplifying complex expressions. Conjugates are like two sides of the same coin – radical expressions that differ only by the sign between their terms.

Imagine a radical expression like √a − √b. Its conjugate would be √a + √b. Now, let’s perform the magic trick of multiplying and dividing by this conjugate. We get:

(√a − √b) * (√a + √b) / (√a + √b)

The denominator √a + √b magically cancels out. And voila! We’re left with the simplified expression:

a − b

This technique proves invaluable when irrational numbers lurk in the denominator of a fraction. For instance, let’s tame the unruly denominator in the fraction 1 / (√2 + √3). Multiplying and dividing by the conjugate √2 – √3, we cast out the irrationality:

1 / (√2 + √3) * (√2 - √3) / (√2 - √3) = (√2 - √3) / (2 - 3) = √2 - √3

By harnessing the power of conjugates, we not only simplify radical expressions but also eliminate the nuisance of irrational denominators. So, the next time you find yourself wrestling with radical subtraction, remember the magic of conjugates. They’ll help you conquer those pesky square root symbols and bring order to the chaos!