Radians Vs. Degrees: Units For Measuring Angles In Mathematics
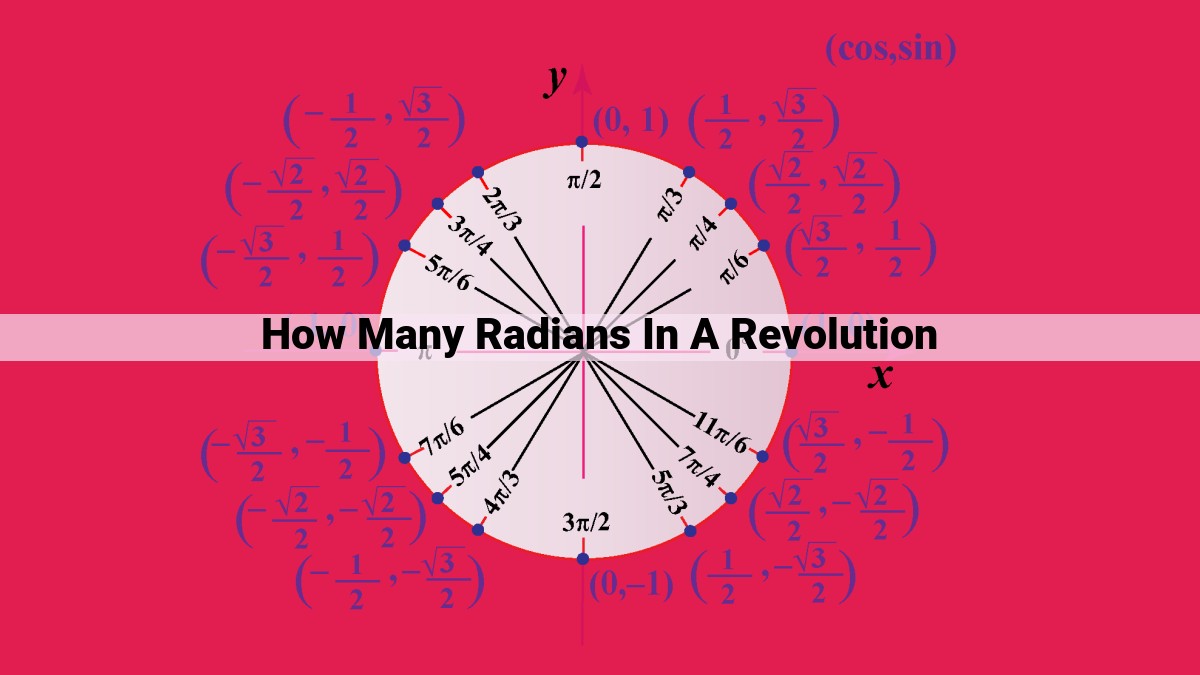
Radians and degrees are two units for measuring angles, with radians being based on the radius of a circle. A revolution, or full circle, measures 2π radians, where π is a mathematical constant approximately equal to 3.14. To convert radians to revolutions, divide the radian value by 2π. Conversely, to convert revolutions to radians, multiply the revolution value by 2π. Radians are commonly used in trigonometry and other mathematical applications because they provide a more natural and intuitive way of measuring angles, particularly when dealing with circular functions and the unit circle.
Understanding the Radian-Degree Connection: A Journey through Angles
In the realm of geometry, the measurement of angles is crucial. Two fundamental units for expressing angles are radians and degrees. Though they may seem interchangeable at first glance, there’s a subtle yet profound relationship between these two units that unveils a world of mathematical elegance and practical applications.
Definition of Radians and Degrees:
A radian is defined as the angle subtended at the center of a circle by an arc whose length is equal to the circle’s radius. On the other hand, a degree is a unit of measurement that divides a full rotation (360°) into 360 equal parts.
Overview of the Relationship:
While radians and degrees measure angles in different ways, they are inextricably connected. The conversion between these units is facilitated by the following formula:
1 radian = π/180 degrees
This means that a complete circle, which equals 2π radians, is equivalent to 360°. This relationship opens up a pathway to understanding and converting between radians and degrees seamlessly.
Radians in a Circle: Unveiling the Essence
In the mesmerizing world of geometry, radians play a pivotal role in describing the measurement of angles. Unlike degrees, which divide a circle into 360 equal parts, radians adopt a more intricate and insightful approach.
Let’s embark on a journey to unravel the enigmas surrounding radians in a circle. Delve into the fascinating world of radians and discover the secrets they hold.
Halfway Around the Circle: Embracing π Radians
Imagine a circle, a mesmerizing embodiment of symmetry and perfection. Now, let’s trace a path from one point on the circumference, traveling halfway around the circle until we return to our starting position. This majestic arc subtends an angle measured in radians. It’s a staggering π radians, approximately 3.14, that captures the essence of this semicircular journey.
Quarter of a Circle: Navigating with π/2 Radians
Let’s venture into the realm of quadrants. As we traverse a quarter of a circle, we encounter an angle adorned with π/2 radians, equivalent to 1.57. This value represents the harmonious balance between the straight line and the arc’s curvature, a captivating union of angles and circles.
Encircling the Circle: Embracing 2π Radians
Now, let’s embark on the ultimate journey of circling the entire circle. This extraordinary odyssey unveils an angle measured in 2π radians, approximately 6.28. It’s a testament to the cyclical nature of radians, where the end of one revolution seamlessly transitions into the beginning of the next.
Degrees vs. Radians: Understanding the Angle Measurement Dichotomy
In the world of geometry, angles play a crucial role in describing the orientation and relationship between lines and planes. While we often encounter angles measured in degrees, there’s another equally significant unit of angular measurement: radians. Understanding the connection between degrees and radians is essential for navigating the nuanced realm of trigonometry and its applications in various fields.
What Are Degrees and Radians?
- Degrees: We all know the concept of degrees (°) from our school days. A complete circle contains 360 degrees, divided into 60 minutes (‘) and 60 seconds (“).
- Radians: Less familiar but equally significant, radians (rad) are the measure of an angle formed by an arc whose length is equal to the radius of the circle.
Conversion Factors: The Bridge Between Degrees and Radians
To effectively work with both degrees and radians, we need to know the conversion factors between them:
- From degrees to radians: π/180°
- From radians to degrees: 180°/π
These factors allow us to seamlessly convert between the two units, opening up a wider range of possibilities in problem-solving.
Visualizing the Relationship
Imagine a circle with a radius of 1 unit. If we take a portion of the circle’s circumference, say an arc whose length is also 1 unit, the angle formed at the center of the circle is 1 radian. This means that one radian corresponds to the angle formed by an arc that is equal in length to the circle’s radius.
Significance of Radians
While degrees are more familiar in everyday conversation, radians often provide advantages in scientific and mathematical calculations. Radians are particularly useful in trigonometry, where they simplify the definitions of trigonometric functions. They also play a vital role in fields like physics and engineering, where quantities often have natural descriptions in terms of angles rather than linear distances.
Understanding the relationship between degrees and radians is a cornerstone of mathematical and scientific exploration. Embracing radians alongside degrees expands our problem-solving abilities and deepens our comprehension of the geometrical world around us. Remember the conversion factors and the visualization technique to effortlessly navigate between these two angle measurement systems.
Understanding the Relationship between Radians and Degrees
Definition of a Radian
In our daily lives, we measure angles using a familiar unit called degrees. However, in many scientific and mathematical fields, a different unit is commonly used: the radian. A radian is defined as the subtended angle by an arc equal to the circle’s radius. In other words, it is the angle formed when you take a portion of a circle’s circumference that matches the length of the circle’s radius.
Derivation of Conversion Factors
This definition of a radian leads us to a fundamental relationship between radians and degrees. Consider a circle with a radius of r. The circumference of this circle is given by 2πr. If we divide the circumference by the radius, we get the result 2π. This means that the subtended angle by the entire circle, measured in radians, is 2π.
Since there are 360 degrees in a complete circle, we can derive conversion factors between radians and degrees. One radian is equivalent to 360/2π = 180/π degrees. Conversely, one degree is equal to π/180 radians. These conversion factors allow us to easily translate angles between these two units of measurement.
By understanding the definition of a radian and its relationship to degrees, we can confidently navigate between these two systems and leverage the advantages that radians offer in various scientific and engineering applications.
Converting Radians to Revolutions
Embark on a Mathematical Adventure
In the realm of angles, we encounter two units of measurement: radians and degrees. While degrees are more familiar in our daily lives, radians hold a special significance in the world of trigonometry and beyond.
Unraveling the Relationship
Imagine a circle. Its circumference, the distance around its edge, is divided into radians. Each radian represents an angle that intercepts an arc equal in length to the circle’s radius.
Now let’s consider a complete revolution. It’s the full 360-degree turn around the circle. The key to converting from radians to revolutions lies in the fact that 1 complete revolution is equal to 2π radians.
The Conversion Formula
To convert radians to revolutions, we need to first convert them to degrees:
Degrees = Radians x (180°/π)
Once we have the number of degrees, we can then convert them to revolutions:
Revolutions = Degrees / 360°
Example
Let’s say we have an angle of π/3 radians. To convert it to revolutions:
- Convert to degrees:
Degrees = π/3 radians x (180°/π) = 60°
- Convert to revolutions:
Revolutions = 60° / 360° = 1/6 revolution
Applications in the Real World
Radians play a crucial role in various fields, from trigonometry to engineering. They offer several advantages over degrees, including:
- Simplicity: Radians are defined in terms of the circle’s radius, making them more intuitive for understanding angles.
- Calculus: Radians are essential for calculus, where they are used to measure the rate of change of angles.
- Physics: Radians are used to describe angular velocity, acceleration, and other rotational motion parameters.
Embrace the Adventure
Converting between radians and revolutions is an essential skill for anyone exploring the world of angles and trigonometry. With a clear understanding of the relationship and the conversion formula, you can confidently embark on your mathematical adventures and gain a deeper appreciation for the fascinating world of angles.
Understanding the Relationship Between Radians and Revolutions
Radians and degrees are two different units used to measure angles. While degrees are more commonly used in everyday life, radians are often preferred in scientific and engineering applications. This post will delve into the relationship between radians and revolutions, providing you with a comprehensive understanding of these units.
Radians in a Circle
In a circle, the number of radians in a half-circle is π radians, in a quarter-circle it is π/2 radians, and in a complete circle it is 2π radians. For instance, a full rotation of a wheel corresponds to 2π radians or 360 degrees.
Degrees vs. Radians
A complete circle measures 360 degrees. The conversion factor from degrees to radians is π/180°, while the conversion factor from radians to degrees is 180°/π. For example, to convert 45 degrees to radians, we multiply by π/180° to get π/4 radians.
Explanation of the Relationship
A radian is defined as the subtended angle by an arc equal to the circle’s radius. This means that the fraction of the circle’s circumference corresponding to a particular angle is equal to the angle in radians. This concept forms the basis of the conversion factors between degrees and radians.
Converting Radians to Revolutions
To convert radians to revolutions, we first multiply by the conversion factor π/180° to convert to degrees. Then, we divide by 360° to obtain the number of revolutions. For instance, to convert π/2 radians to revolutions, we get (π/2 radians) * (π/180°) / 360° = 1/4 revolution.
Example Calculations
Converting Radians to Revolutions:
- 3π/4 radians = (3π/4 radians) * (π/180°) / 360° = 3/8 revolution
- 5π/6 radians = (5π/6 radians) * (π/180°) / 360° = 5/12 revolution
Converting Revolutions to Radians:
- 1/2 revolution = 1/2 revolution * 360° / (π/180°) = π radians
- 1/4 revolution = 1/4 revolution * 360° / (π/180°) = π/2 radians
Applications and Significance of Radians
In the realm of mathematics, engineering, and physics, radians emerge as a valuable tool, offering unique advantages over degrees. Understanding the relationship between radians and degrees is crucial for unlocking the practical applications and profound insights that radians provide.
Trigonometry
In the world of trigonometry, radians shine as the natural unit of measure for angles. The trigonometric functions, such as sine, cosine, and tangent, exhibit a remarkable symmetry and simplicity when expressed in radians. This simplicity stems from the fact that radians are based on the circle’s radius, which serves as a fundamental geometric constant.
Engineering and Physics
Radians also play a pivotal role in engineering and physics. In rotational motion, radians serve as the standard unit for angular displacement. The calculations of speed, acceleration, and torque become more precise and intuitive when using radians. This is because radians provide a direct measure of the angle of rotation, eliminating the need for additional conversion factors.
Moreover, radians are essential for understanding circular motion in areas such as mechanics, electromagnetism, and astronomy. The equations governing these phenomena simplify significantly when radians are employed, allowing for more accurate analysis and deeper insights.
Advantages of Radians
The use of radians offers several advantages over degrees. Firstly, radians are unitless, unlike degrees which are measured in degrees. This eliminates the need for constant conversion between units, reducing the risk of errors and simplifying calculations.
Secondly, radians provide a continuous measure of angles. Degrees, on the other hand, are discrete units which can lead to inaccuracies when dealing with small angles. Radians allow for a more precise representation of angles, enabling more accurate analysis and predictions.
Insights Gained
By using radians, we gain deeper insights into the nature of angles and their relationship with other mathematical concepts. The conversion factor between radians and degrees highlights the connection between linear and circular measure. This connection provides a deeper understanding of the geometry of circles and the nature of rotation.
Radians also facilitate the exploration of the unit circle, a fundamental concept in trigonometry. By expressing angles in radians, we can visualize the unit circle as a continuous path, where the coordinates of points correspond to the sine and cosine values of the angles. This provides a powerful tool for analyzing trigonometric identities and other advanced mathematical relationships.





