Understand Radian Measure: Formula, Calculation, And Applications

To determine the radian measure of a central angle, we use the formula θ = s/r, where θ is the radian measure, s is the arc length, and r is the radius. The radian measure represents the ratio of arc length to radius and is a unitless quantity. By measuring the arc length and radius, we can calculate the radian measure, which is essential for various applications in engineering, navigation, and physics.

• Define radian measure and explain its importance in trigonometry.
• State the purpose of finding the radian measure of a central angle.

Understanding Radian Measure: A Gateway to Trigonometry

Imagine yourself as an explorer in the vast realm of trigonometry. To successfully navigate this unfamiliar territory, you’ll need a reliable measuring tool—the radian measure. Radian measure is a unitless quantity that helps us quantify angles, a fundamental concept in trigonometry.

The purpose of finding the radian measure of a central angle, an angle formed by two radii of a circle, is to establish a standardized way of measuring angles. This measure allows us to compare and relate angles regardless of the size of the circles they belong to. Radian measure plays a crucial role in trigonometry, providing a common language for angles in various applications, from navigation to physics.

Defining Radian Measure: The Arc-Radius Ratio

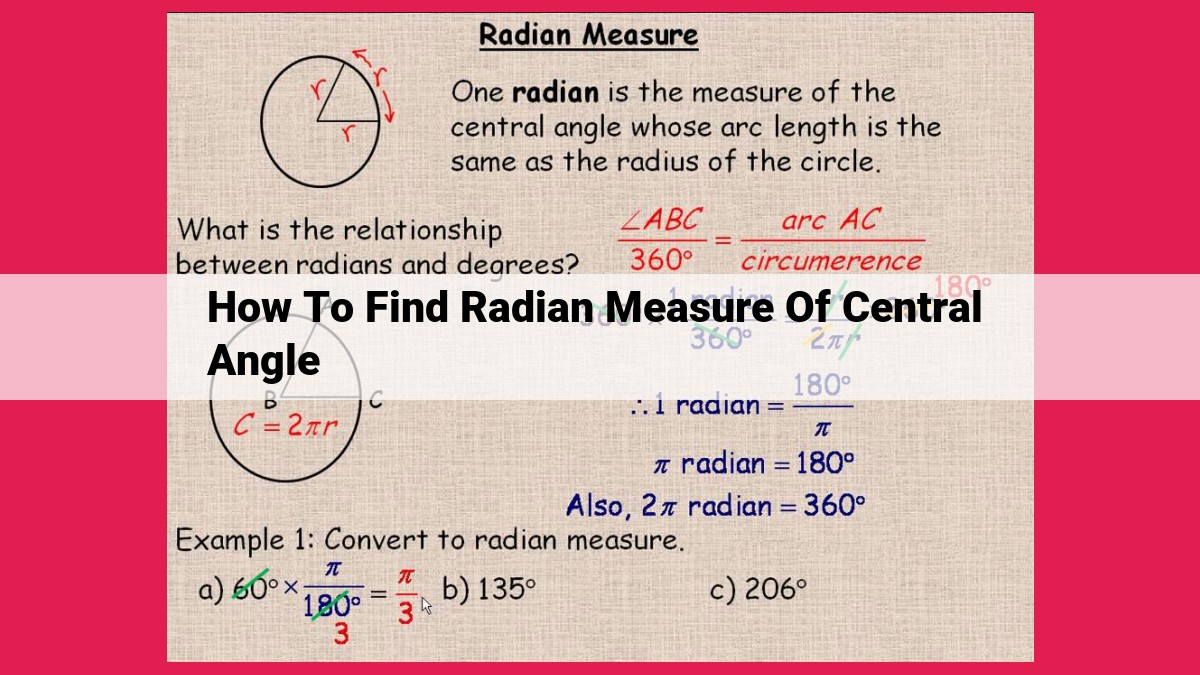
The radian measure (θ) of a central angle is defined as the ratio of its corresponding arc length (s) to the circle’s radius (r):

θ = s / r

This means that an angle of one radian is the angle formed when the arc length is equal to the radius of the circle.

Unlocking the Secrets of Central Angles and Arc Length

A central angle is formed when two radii of a circle intersect at the center. The arc length is the distance along the circumference of the circle that subtends the central angle.

The radian measure of a central angle provides valuable insights into its geometry. A larger arc length corresponds to a larger radian measure, indicating a wider angle. Similarly, a larger radius leads to a smaller radian measure for the same angle, illustrating the inverse relationship between radius and radian measure.

Understanding Radian Measure: Essential Concepts and Relationships

Radian Measure: The Unitless Angle

Imagine a circle as a slice of pizza, with its center representing the point where you hold it. The circumference of the pizza represents the entire outer edge. Now, let’s say you measure the length of the crust along a certain portion of the circle. This length is called the arc length.

The radian measure is a special unit used to measure angles that relates the arc length to the radius of the circle. It’s defined as the ratio of arc length (s) to radius (r):

θ = s/r

Central Angle: Formed by Radii

The central angle is the angle formed when two radii (lines connecting the center to points on the circle’s edge) intersect. The size of a central angle determines the length of the corresponding arc. In other words, the larger the central angle, the longer the arc length.

Arc Length: Distance Along the Circumference

Arc length measures the distance along the circle’s circumference. It’s important to note that the arc length is not the same as the chord length (the straight line connecting two points on the circle), even though they may look similar.

Radius: Center to Edge

The radius is the distance from the circle’s center to any point on its circumference. It serves as the “measuring stick” for radian measure and arc length.

Finding the Radian Measure of a Central Angle: A Step-by-Step Guide

In the realm of trigonometry, the radian measure holds great significance. It’s the key to unlocking the mysteries of angles and their relationship with circles. Understanding how to find the radian measure of a central angle empowers us to unravel the intricacies of geometry and delve into the practical applications that span across diverse fields.

The Formula: A Guiding Light

At the heart of determining the radian measure lies a simple yet profound formula: θ = s/r

This formula embodies the essence of a radian – the ratio of arc length (s) to the radius (r) of the circle.

Steps to Success: A Journey of Discovery

To embark on the journey of finding the radian measure of a central angle, we follow a series of steps:

1. Measure the Arc Length (s): Determine the distance along the circumference of the circle that corresponds to the angle.

2. Identify the Radius (r): Measure the distance from the center of the circle to any point on the arc.

3. Calculate the Radian Measure (θ): Divide the arc length (s) by the radius (r) using the formula θ = s/r.

Practical Applications: Where Radians Shine

The radian measure finds its practical applications in a multitude of fields:

• Engineering: Radians are indispensable in calculating the angles of gears, pulleys, and other mechanical components.

• Navigation: They play a crucial role in determining the course and position of ships and airplanes.

• Physics: Radians facilitate the understanding of angular velocity, acceleration, and other concepts related to circular motion.

Unraveling the mysteries of the radian measure unlocks a world of geometric and practical applications. Embracing the concepts of arc length, radius, and the formula θ = s/r empowers us to navigate the intricate landscapes of trigonometry and beyond.