Quartic Equation Solution Methods For Physics And Engineering

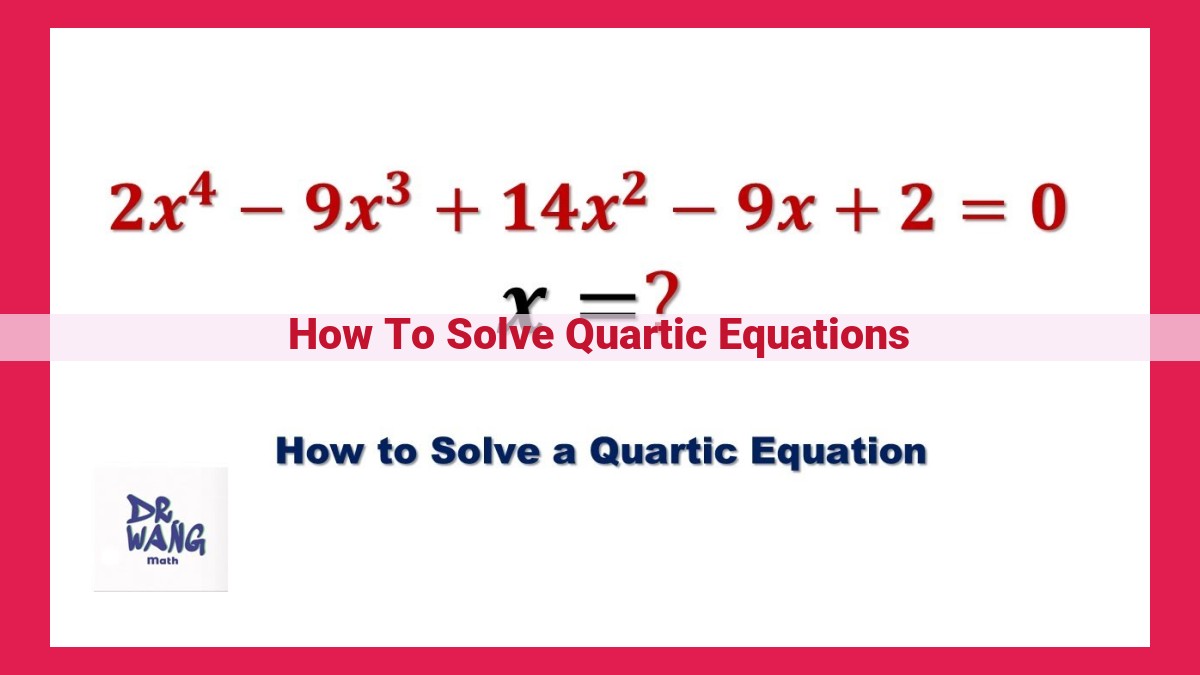
To solve quartic equations, various methods exist. Completing the square simplifies them. The factor theorem aids in factorization. The fundamental theorem of algebra ensures solvability. Gauss’s method provides a systematic approach. Lagrange’s method utilizes transformations. Descartes’ rule of signs determines root counts. Sturm’s theorem analyzes root intervals. Numerical methods, such as Newton’s, secant, and bisection, offer approximate solutions. These methods empower the exploration of quartic equations’ solutions, with applications in fields like physics and engineering.

Unveiling the Enigmatic World of Quartic Equations

In the realm of mathematics, quartic equations stand as enigmatic puzzles, beckoning scholars to unravel their secrets. Defined by their fourth degree, these equations find critical applications in diverse fields, from physics and engineering to economics and biology. They govern the trajectories of celestial bodies, model financial markets, and decipher the intricacies of chemical reactions.

Embarking on a mathematical expedition, we shall explore the captivating methods devised to solve these enigmatic equations. From the simplicity of completing the square to the power of the fundamental theorem of algebra, each technique unveils a unique path to uncovering the roots of quartic equations.

As we traverse this mathematical odyssey, we’ll encounter the ingenuity of Gauss and Lagrange as they devised elegant methods for solving these complex equations. We’ll delve into the astuteness of Descartes’ rule of signs, which provides clues to the number of positive and negative roots.

Numerical methods, such as Newton’s method and the bisection method, offer alternative approaches to approximating solutions with increasing accuracy. Each method unveils a different perspective, providing a kaleidoscope of insights into the nature of quartic equations.

Join us on this captivating journey as we unravel the intricacies of quartic equations, unlocking their hidden truths and revealing their profound significance in shaping our world.

Method 1: Completing the Square

Unveiling the Secrets of Quartic Equations

In the realm of algebra, quartic equations stand as enigmatic giants, their enigmatic nature often leaving mathematicians in a state of perplexity. However, amidst the challenges they present, the method of completing the square emerges as a beacon of hope, illuminating a path toward their resolution.

Understanding the Essence of Completing the Square

At its core, completing the square involves transforming a quadratic expression into an equivalent expression that is a perfect square. This transformation simplifies the equation, making it more amenable to solving. Consider a general quartic equation in the form:

ax^4 + bx^3 + cx^2 + dx + e = 0

Applying Completing the Square to Quartic Equations

The first step in applying completing the square is to divide both sides of the equation by a, the coefficient of the highest power:

x^4 + (b/a)x^3 + (c/a)x^2 + (d/a)x + (e/a) = 0

Next, we focus on the quadratic term x^2 + (b/a)x. Our goal is to add and subtract a constant that will complete the square:

x^4 + (b/a)x^3 + (c/a)x^2 + (d/a)x + (b^2/4a^2) - (b^2/4a^2) + (e/a) = 0

The added constant, (b^2/4a^2), completes the square. We can now factor the left-hand side as follows:

(x^2 + (b/a)x + b^2/4a^2) + (d/a)x + (e/a) - (b^2/4a^2) = 0

(x + b/2a)^2 + (d/a)x + (e/a) - (b^2/4a^2) = 0

Simplifying the Equation

The equation is now in a more manageable form. We can further simplify it by letting:

u = x + b/2a

Substituting u into the equation, we get:

u^2 + (d/a)u + (e/a) - (b^2/4a^2) = 0

This equation is now a quadratic equation in u, which can be solved using standard techniques, such as the quadratic formula. Once u is determined, we can substitute it back into x = u - b/2a to find the solutions to the original quartic equation.

Method 2: Unraveling the Secrets of Quartic Equations with the Factor Theorem

In our quest to conquer the world of quartic equations, let’s set sail for the shores of the Factor Theorem. This powerful tool will serve as our compass, guiding us towards the hidden factors that lurk within these complex polynomials.

The Factor Theorem states that if a polynomial f(x) has a factor (x - a), then substituting x = a into f(x) will yield zero. Armed with this knowledge, we can embark on a factoring adventure, strategically testing different values of a until we stumble upon the elusive factors.

For instance, let’s consider the quartic equation: x^4 - 2x^3 + x^2 - 2x + 1. By substituting x = 1 into this equation, we find that f(1) = 1^4 - 2(1)^3 + 1^2 - 2(1) + 1 = 0. This tells us that (x - 1) is a factor of our quartic equation.

Once we’ve found one factor, we can use polynomial division or synthetic division to extract the remaining factors. It’s like peeling back the layers of an onion, gradually revealing the inner workings of our quartic equation.

By skillfully applying the Factor Theorem, we can decompose quartic equations into manageable chunks, unlocking their secrets and revealing their hidden structure. It’s a technique that transforms complex polynomials into more approachable forms, making their analysis and solution a much less daunting task.

Method 3: Unveiling the Roots with the Fundamental Theorem of Algebra

The Fundamental Theorem of Algebra is a cornerstone of mathematics, asserting that every non-zero polynomial with real or complex coefficients has at least one root in the complex plane. This profound theorem has profound implications for quartic equations, equipping us with a powerful tool to unravel their hidden roots.

Let’s consider a quartic equation:

$$ax^4 + bx^3 + cx^2 + dx + e = 0$$

where a ≠ 0. According to the Fundamental Theorem of Algebra, this equation has at least one complex root. This means that there exists a complex number z = a + bi (where a and b are real numbers and i is the imaginary unit) such that:

$$a(a + bi)^4 + b(a + bi)^3 + c(a + bi)^2 + d(a + bi) + e = 0$$

By expanding and equating the real and imaginary parts to zero, we can obtain a system of equations that can be solved to find the values of a and b. This unveils the complex roots of the quartic equation.

The Fundamental Theorem of Algebra is a pivotal tool in the exploration of quartic equations, providing a fundamental understanding of their root structure. By harnessing its power, we can unravel the intricacies of these enigmatic equations and delve into the realm of their solutions.

Method 4: Gauss’s Method

Embarking on a Systematic Quest to Conquer Quartics

Prepare to delve into the intricate world of Gauss’s method, a systematic approach that empowers us to unravel the mysteries of quartic equations. This method, meticulously crafted by the legendary mathematician Carl Friedrich Gauss, provides a structured and efficient framework for solving these complex equations.

The Essence of Gauss’s Method

Gauss’s method is a masterful blend of algebraic transformations and numerical approximations. It begins by skillfully transforming the quartic equation into an auxiliary quadratic equation. This transformation, akin to a magician’s sleight of hand, dramatically simplifies the equation, making it more manageable.

A Step-by-Step Expedition

Let’s embark on a step-by-step expedition to witness the power of Gauss’s method firsthand:

• Step 1: Laying the Foundation

  We begin by expressing the quartic equation in its general form, ax^4 + bx^3 + cx^2 + dx + e = 0.

• Step 2: The Transformative Leap

  Gauss’s method hinges on a clever substitution that introduces a new variable, z = x + b/4a. This substitution, like a key unlocking a hidden treasure, transforms the quartic equation into an auxiliary quadratic equation, z^2 + pz + q = 0.

• Step 3: Solving the Auxiliary Equation

  With the auxiliary equation in our grasp, we can effortlessly solve it using the trusty quadratic formula. This yields two possible values for z, which we’ll call z1 and z2.

• Step 4: The Resolution

  Finally, we skillfully employ the reverse substitution to express the two solutions of the quartic equation in terms of the original variable x.

Harnessing the Power of Gauss’s Method

Gauss’s method stands out as a remarkable tool for tackling quartic equations, offering several distinct advantages:

• Systematic Approach: It provides a structured and step-by-step approach, ensuring accuracy and minimizing the risk of errors.
• Transformative Power: The transformative substitution simplifies the quartic equation, making it more accessible and manageable.
• Numerical Flexibility: Gauss’s method can be seamlessly combined with numerical techniques, enhancing its versatility and applicability.

While Gauss’s method shines in solving quartic equations, it’s important to note that it may not be the most efficient approach for all cases. The choice of method ultimately depends on the specific equation and the desired level of accuracy.

Lagrange’s Method: Unlocking the Secrets of Quartic Equations

In the realm of quartic equations, where the unknown variable hides behind intricate expressions, Lagrange’s method emerges as a beacon of simplicity. It employs a clever trick, a transformation that metamorphoses a seemingly formidable equation into a more manageable form.

Imagine a sculptor transforming a rough block of marble into a graceful masterpiece. Lagrange’s method does something similar. It takes the unyielding quartic equation and chisels away its complexities, revealing the hidden roots beneath.

The essence of Lagrange’s method lies in its ability to replace the quartic equation with a quadratic equation. By introducing a new variable and performing a series of substitutions, it effectively reduces the degree of the equation, making it more amenable to solving.

The transformation is akin to a magician’s sleight of hand. With a wave of his wand, the quartic equation vanishes, replaced by a quadratic counterpart that beckons for an immediate solution.

Lagrange’s method is not merely a mathematical tool; it is an art form. It empowers mathematicians to tame the untamed and illuminate the unknown. It is a testament to the human mind’s ability to find elegance in complexity and order in chaos.

Descartes’ Rule of Signs: Unveiling the Secrets of Quartic Equations

In the realm of mathematics, quartic equations hold a captivating allure, their intricacy beckoning mathematicians to unravel their hidden depths. Among the arsenal of methods employed to solve these enigmatic equations, Descartes’ Rule of Signs emerges as an elegant tool, empowering us to determine the elusive number of positive and negative real roots lurking within.

Picture a quartic equation, a polynomial of the fourth degree, gracing the pages of your notebook. Descartes’ Rule of Signs becomes your guide, a compass navigating the uncharted waters of the equation’s solution set. It whispers secrets, revealing the number of real roots that bask in positivity’s embrace and those that reside in negativity’s shadowy realm.

At its core, Descartes’ Rule of Signs operates on a simple premise: the number of sign changes in the coefficients of the equation’s terms corresponds to the number of positive real roots. Conversely, the number of sign changes in the coefficients of the terms excluding the first term reveals the number of negative real roots.

Consider, for example, the quartic equation x^4 – 3x^3 + 2x^2 – x + 1 = 0. Its coefficients, arranged in descending order, are (-1, -3, 2, -1, 1). Notice the single sign change from -3 to 2, indicating the presence of one positive real root. Moreover, the coefficients excluding the first term (-3, 2, -1, 1) exhibit no sign changes, suggesting the absence of negative real roots.

Descartes’ Rule of Signs, like a mystical oracle, offers invaluable insights into the nature of quartic equations. It empowers us to discern the equation’s solution set without delving into the complexities of solving the equation itself. Its simplicity and elegance render it an indispensable tool in the mathematical toolbox, a beacon of knowledge guiding us through the enigmatic world of quartic equations.

Sturm’s Theorem: Unveiling the Secrets of Real Roots

In the realm of quartic equations, where the search for solutions can be an arduous quest, there exists a powerful tool known as Sturm’s Theorem. This theorem, like a guiding light, illuminates the path to determining the number of real roots that lie hidden within a given interval.

Sturm’s Theorem operates on the principle that the number of real roots in an interval is equal to the difference in the number of sign changes between the coefficients of two consecutive derivatives of the polynomial. By carefully analyzing this sign change pattern, we can gain invaluable insights into the behavior of the quartic equation.

To apply Sturm’s Theorem, we first construct a sequence of polynomials:

$$P(x), P'(x), P''(x), ..., P^{(n-1)}(x)$$

where (n) is the degree of the quartic equation. We then evaluate these polynomials at the endpoints of the interval of interest. If the resulting sequence of values changes sign an odd number of times, then the interval contains an odd number of real roots. Conversely, if the sign change occurs an even number of times, then the interval contains an even number or zero real roots.

Example:

Consider the quartic equation (x^4 – 2x^3 – 5x^2 + 6x + 4). Let’s apply Sturm’s Theorem to the interval ([-1, 1]).

$$P(x) = x^4 - 2x^3 - 5x^2 + 6x + 4$$
$$P'(x) = 4x^3 - 6x^2 - 10x + 6$$
$$P''(x) = 12x^2 - 12x - 10$$

At (x = -1), the values of the polynomials are:

$$P(-1) = 4$$
$$P'(-1) = -2$$
$$P''(-1) = 6$$

The sign change pattern is (4 \to -2 \to 6), which has an odd number of sign changes (one).

At (x = 1), the values of the polynomials are:

$$P(1) = 2$$
$$P'(1) = 2$$
$$P''(1) = 2$$

The sign change pattern is (2 \to 2 \to 2), which has an even number of sign changes (zero).

Therefore, by Sturm’s Theorem, the interval ([-1, 1]) contains one real root.

Numerical Methods: Approximating the Roots of Quartic Equations

In the realm of mathematics, solving quartic equations can present a formidable challenge. Yet, through the ingenuity of mathematicians, a myriad of numerical methods have emerged as powerful tools to approximate the roots of these enigmatic equations. Let us delve into the captivating world of these numerical methods.

Newton’s Method

Imagine embarking on a thrilling treasure hunt, guided by a series of clues. Newton’s Method is akin to such a quest, where each step brings you closer to the elusive treasure—the root of the quartic equation. Starting with an initial guess, the method iteratively refines its estimate, guided by the slope of the graph of the equation at the current guess. With each iteration, the approximation becomes ever more precise, leading you closer to the hidden treasure.

Secant Method

The Secant Method shares similarities with Newton’s Method but employs a slightly different approach. Instead of relying solely on the slope at the current guess, it incorporates the slope at a previous guess as well. This refined approach often leads to more rapid convergence, reducing the number of iterations needed to reach the desired accuracy.

Bisection Method

The Bisection Method stands apart from the previous two methods as it does not require any knowledge of the graph or its slope. It operates on the principle of divide and conquer. The method repeatedly divides the interval containing the root in half, discarding the half that does not contain the root until the desired accuracy is achieved. Although slower in convergence compared to Newton’s Method or the Secant Method, Bisection Method offers the advantage of guaranteed convergence.

The arsenal of numerical methods provides invaluable tools for tackling the complexities of quartic equations. Each method offers unique advantages and limitations, making them suitable for different scenarios. Newton’s Method and the Secant Method shine in their rapid convergence, while the Bisection Method provides the comforting assurance of guaranteed convergence.

The choice of which numerical method to employ depends on factors such as the desired accuracy, the nature of the equation, and the available computational resources. By understanding the nuances of each method, we can harness their power to unlock the mysteries hidden within quartic equations.