Proving Parallelograms: Key Properties and Geometric Applications

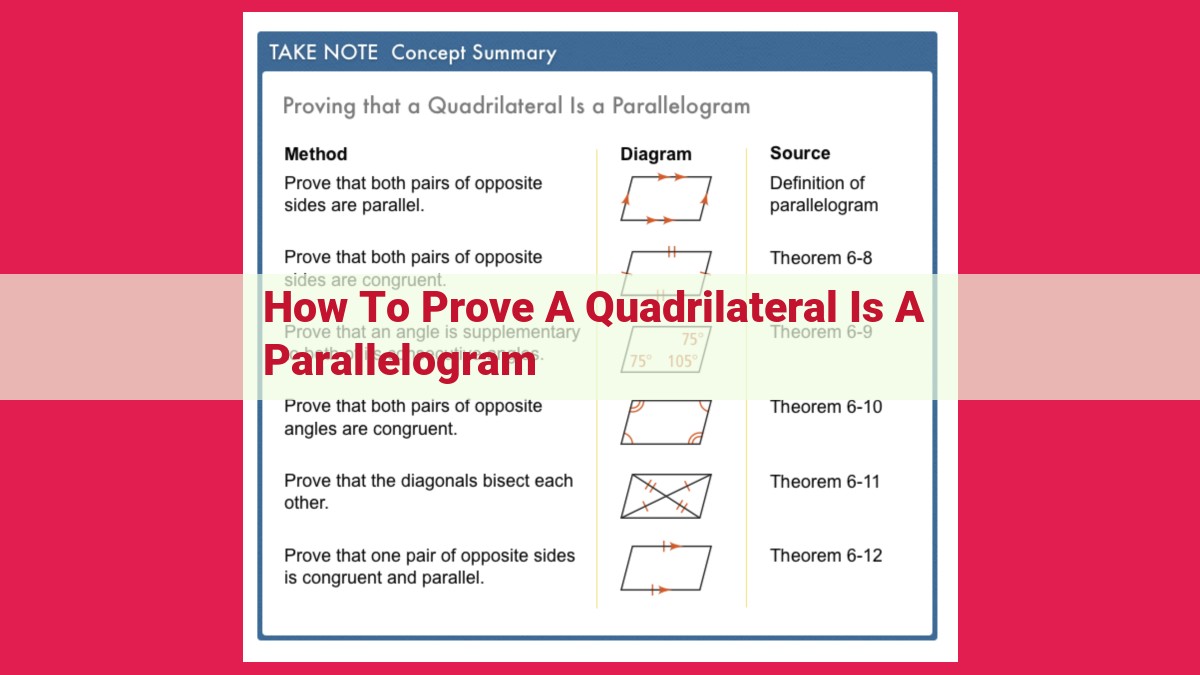
To prove a quadrilateral is a parallelogram, establish its key properties. Prove that opposite sides are congruent using measurements or postulates. Demonstrate that opposite angles are equal using angle measurers or properties. Verify that diagonals intersect at midpoints and bisect each other. For squares and rhombuses, show that diagonals are perpendicular bisectors. Prove that adjacent angles are supplementary. Derive the area and perimeter formulas. Applying these properties enables us to identify parallelograms and utilize them in various geometric applications.

Contents

Definition and key characteristics of a parallelogram

A Journey through the Wonders of Parallelograms: Unraveling Their Secrets

Imagine yourself as a geometry explorer, embarking on an adventure to uncover the hidden treasures of parallelograms. These enigmatic shapes hold a world of fascinating properties, awaiting our discovery.

Chapter 1: Unveiling the Enigma of Parallelograms

At the heart of our exploration lies the definition of a parallelogram: a quadrilateral with opposite sides parallel. This intriguing characteristic sets it apart from other shapes, endowing it with unique qualities.

Just like in a harmonious dance, parallelograms exhibit graceful symmetry. Opposite sides not only run side by side but are also mirror images of each other in both length and direction. This remarkable property forms the foundation of our adventure.

Unraveling the Secrets of Parallelograms: A Comprehensive Guide to Their Existence

In the vast realm of geometry, parallelograms stand as captivating figures, imbued with intricate properties that have mystified scholars for centuries. Join us on an enlightening journey as we delve into the world of parallelograms, uncovering the fundamental proofs that establish their unique characteristics.

1. Defining the Enigma of the Parallelogram

A parallelogram, in its purest form, is a quadrilateral with two pairs of parallel sides. These parallel lines bestow upon parallelograms a distinctive symmetry, setting them apart from their geometric counterparts. But how do we prove the existence of a parallelogram?

1.1. A Tale of Parallel Lines

One method revolves around the concept of parallel lines. If we can demonstrate that two sides of a quadrilateral are parallel and the other two sides are also parallel, then the quadrilateral must be a parallelogram. This approach relies on the fundamental postulate of parallel lines, which states that two lines are parallel if they never intersect.

1.2. Measuring Up to Parallelogramhood

Another path to proving the existence of a parallelogram lies in measurement. By measuring the opposite sides and opposite angles of a quadrilateral, we can determine if they are congruent—that is, equal in length or measure. If both pairs of opposite sides and angles are congruent, then the quadrilateral qualifies as a parallelogram.

1.3. Quadrilaterals with a Twist

A final method of proving a parallelogram’s existence involves exploring its diagonals. Diagonals are line segments that connect opposite vertices of a quadrilateral. If the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram. This method hinges on the fact that only parallelograms have diagonals that intersect at their midpoints.

By mastering these proofs, we unlock the secrets of parallelograms, paving the way for further exploration of their intriguing properties and unlocking a deeper understanding of geometry’s fascinating world.

Unveiling the Mysteries of Parallelograms: A Tale of Equal Opposite Sides

In the realm of geometry, the parallelogram stands as an enigmatic shape, holding secrets that have captivated mathematicians for centuries. One of its most fundamental properties is the intriguing notion of equal opposite sides. But how do we prove this seemingly simple truth? Let us embark on a journey of discovery, where we unravel the mathematical tapestry that weaves together the essence of parallelograms.

The Opposite Sides: A Tale of Two Twins

Imagine two lines that intersect at a single point. These lines, known as transversals, create four distinct angles around the intersection. In the realm of parallelograms, these angles play a pivotal role in revealing the secret of equal opposite sides.

Let us consider a transversal that intersects a parallelogram, creating four angles denoted as α, β, γ, and δ. The opposite angles, α and γ, lie on the same side of the transversal, while β and δ are on the opposite side.

Here’s where the magic unfolds: according to the Angle-Angle Similarity Postulate, if two angles are congruent, then the lines containing them must be parallel. In our case, if α = γ, then the lines on which they lie must be parallel. Similarly, if β = δ, the lines containing them must also be parallel.

But wait, there’s more! The Transitive Property enters the scene, whispering a profound truth: if two lines are parallel to a third line, they are parallel to each other. This means that the lines containing α and γ are parallel to the lines containing β and δ.

Eureka! We have stumbled upon a geometric revelation: the opposite sides of a parallelogram are parallel. And since parallel lines have equal lengths, we have finally unlocked the secret: the opposite sides of a parallelogram are equal in length.

Different proof techniques, such as measurement and geometric postulates

The Enigmatic Parallelogram: Unveiling Its Hidden Truths through Mathematical Proofs

In the realm of geometry, the parallelogram stands out as a fascinating figure with a treasure trove of remarkable properties. Let’s embark on an enchanting journey of proofs that will unravel the secrets hidden within its four sides and two pairs of parallel lines.

Proving **Congruent Opposite Sides:

These opposite sides of a parallelogram possess a captivating secret: they are equal in length. How do we establish this mathematical truth? We can invoke the trusty method of measurement, painstakingly measuring each side and confirming their equivalence. Alternatively, we can appeal to geometric postulates, invoking the wisdom of ancient mathematicians who have gifted us with foundational principles.

Proving **Congruent Opposite Angles:

Another intriguing property whispers to us: the opposite angles of a parallelogram are equal in measure. This revelation can be uncovered through the precision of angle measurers, or we can employ angle properties, navigating the intricate web of geometric relationships to illuminate this truth.

Exploring Intersecting and Bisecting Diagonals:

The diagonals of a parallelogram possess a captivating secret: they intersect at their midpoints. To unravel this mystery, we can engage in a geometric dance, drawing the diagonals and carefully measuring their segments, revealing their perfect balance.

Diagonals as Perpendicular Bisectors in Squares and Rhombuses:

In the special cases of squares and rhombuses, the diagonals unveil an extraordinary property: they not only intersect at midpoints but also bisect each other, creating perpendicular lines. This geometric harmony can be demonstrated through elegant proofs, revealing the exquisite symmetry inherent in these shapes.

Supplementary Adjacent Angles:

The adjacent angles of a parallelogram hold a delightful surprise: they add up to 180 degrees. This revelation may seem counterintuitive at first, but it can be unveiled through the principles of parallel lines and alternate interior angles, providing a glimpse into the intricate dance of geometric relationships.

Deriving the Area Formula:

The area of a parallelogram is calculated using a formula that weaves together the concepts of base and height. We embark on a mathematical journey to unravel this formula, step-by-step, revealing the intricate interplay between these quantities.

Deriving the Perimeter Formula:

To grasp the perimeter of a parallelogram, we explore a formula that combines length and width. Through a series of calculations, we uncover the relationship between these dimensions and the total length of the parallelogram’s boundary.

Applications and Examples:

The properties of parallelograms cast their spell across various real-life situations. We embark on a voyage of discovery, unveiling practical examples where these geometric truths find their application, illuminating the power of mathematics in shaping our world.

3. Proving Congruent Opposite Angles: Unraveling the Secret of Equivalent Measures

In the realm of geometry, parallelograms stand out with their unique characteristics, not least of which is the remarkable equality of their opposite angles. Imagine yourself as a geometry detective, embarking on a quest to uncover the secrets behind this intriguing phenomenon.

The definition of opposite angles serves as our starting point. Opposite angles are those that lie across from one another within a parallelogram. Intriguingly, these angles possess an equal measure, a fact that we seek to prove.

To embark on our proof adventure, we’ll employ various techniques that will lead us to the truth. One such technique involves the use of angle measurers, devices that precisely gauge the magnitude of angles. By meticulously measuring the angles opposite one another, we consistently observe their equal size.

Another approach leverages the power of geometric postulates, fundamental principles that govern the behavior of angles. These postulates eloquently state that angles formed by parallel lines and transversals possess certain relationships. By skillfully applying these postulates to the angles in our parallelogram, we can derive their congruence.

Thus, we emerge from our geometric investigation with a profound understanding of the congruence of opposite angles in parallelograms. This revelation serves as a cornerstone of geometry, empowering us to unravel the mysteries of shape and measure.

Proving Congruent Opposite Angles: An Angle Adventure

In the realm of geometry, we embark on a quest to unravel the secrets of parallelograms and delve into their fascinating world. Among their many intriguing properties lie the congruent opposite angles. But how do we prove this geometric gem?

One approach involves unleashing the power of angle measurers. Armed with this tool, we carefully measure the opposite angles within a parallelogram. We discover, time and again, that they share the same numerical value, as if they were twins separated at birth.

Another proof strategy relies on the wisdom of geometric postulates. We call upon the opposite angles theorem, a fundamental law of geometry that states that opposite angles in a parallelogram are always congruent. This theorem serves as our guiding star, illuminating the path to our conclusion.

Yet another method taps into the interplay between parallel lines and transversals. We draw a transversal to intersect two sides of our parallelogram, creating new angles. By analyzing these angles and applying the properties of parallel lines, we deduce that opposite angles in the parallelogram are indeed congruent.

These proof techniques provide a solid foundation for understanding the congruence of opposite angles in parallelograms. They equip us with the knowledge to navigate the unknown and unravel the mysteries that geometry holds.

The definition of diagonals intersecting at their midpoints

Proving Intersecting and Bisecting Diagonals: Unraveling the Secrets of Parallelograms

In the captivating world of geometry, parallelograms stand out as intriguing shapes that possess harmonious properties. One such property is the intersection of their diagonals at their midpoints. Embarking on a journey to unravel this geometric mystery, we will explore the proof strategies that unveil the secrets of bisecting diagonals.

Drawing the Parallelogram: A Canvas for Discovery

To begin our proof, we sketch a parallelogram, a quadrilateral with two pairs of parallel sides. Let’s name the intersection point of the diagonals as O.

Method 1: Measurement Maestro

Armed with a precise measuring tool, we carefully determine the lengths of the diagonals, AC and BD. Assuming they measure m and n units, respectively, we calculate their midpoints as M and N.

Next, we measure the distances from point O to each midpoint:

MO and MO
NO and NO

If our measurements reveal that MO = MO and NO = NO, then we have proven that the diagonals of our parallelogram intersect at their midpoints, M and N.

Method 2: Postulate Perfection

Another approach, rooted in geometric postulates, begins with the observation that opposite sides of a parallelogram are congruent. Therefore, AB = CD and BC = DA.

Following this, we draw a line MO, connecting the midpoint M of diagonal AC to the midpoint O of diagonal BD. Similarly, we draw line NO, connecting midpoint N to point O.

Using the properties of congruent triangles, we can demonstrate that triangles AMO and CMO are congruent, as well as triangles BNO and DNO.

From these congruences, we deduce that MO = MO and NO = NO. Therefore, point O is indeed the midpoint of both diagonals, AC and BD.

The Proof Unveiled: A Tale of Geometric Symmetry

Whether through precise measurements or the power of postulates, we have established that the diagonals of a parallelogram intersect at their midpoints. This elegant property serves as a testament to the inherent symmetry and harmony found within the realm of geometry.

Proving Intersecting and Bisecting Diagonals

The Crossroads of a Parallelogram: Where Diagonals Meet

Imagine a bustling intersection where two roads, the diagonals of a parallelogram, cross each other. Just as traffic smoothly navigates this junction, the diagonals of a parallelogram intersect in a precise harmony.

Midpoint Rendezvous: The Heart of the Intersection

As if guided by an invisible force, the diagonals meet exactly at their midpoints. This intersection creates a special point, a nexus where the parallelogram’s symmetry shines brightest. Imagine two tightrope walkers, meeting in perfect balance at the very center of the canvas.

The Proof Unfolds: A Geometrical Adventure

To prove this midpoint rendezvous, we embark on a geometrical adventure. First, we draw a pair of intersecting diagonals. Next, we measure the distance from each vertex to the intersection point, using a trusty ruler or compass.

Mirroring Measurements: A Symphony of Equality

What do we discover? Lo and behold, the distances on opposite sides of the intersection are equal! This symmetry echoes throughout the parallelogram, creating a perfect balance of form.

Diagonals as Bisectors: Dividing the Parallelogram in Harmony

Not only do the diagonals intersect at their midpoints, but they also divide the parallelogram into two congruent triangles. These triangles are true mirror images, with identical side lengths and angles.

A Dance of Symmetry: Joining the Dots

The diagonals act as the central axis of this symmetry. By connecting the midpoints of opposite sides, we create a line parallel to the base. This line cuts the parallelogram into two equal halves, demonstrating the power and beauty of geometry’s dance of symmetry.

Diagonals as Perpendicular Bisectors in Squares and Rhombuses: A Geometric Symphony

In the world of geometry, squares and rhombuses stand out with a unique characteristic: their diagonals possess the remarkable property of being both bisectors and perpendicular bisectors. This fascinating property adds to the intrigue of these shapes and offers valuable insights into their internal structure.

A bisector divides a line segment or an angle into two equal parts. In the case of squares and rhombuses, the diagonals act as both segment bisectors and angle bisectors. When drawn, the diagonals intersect at a point that divides each diagonal into two congruent segments. Furthermore, this intersection point also divides each angle formed by the sides of the square or rhombus into two congruent angles.

But the magic doesn’t stop there. The diagonals of squares and rhombuses are not only bisectors but also perpendicular bisectors. This means that they not only divide the sides and angles equally but also intersect at a right angle (90 degrees). This property creates a beautiful geometric pattern that adds to the symmetry and elegance of these shapes.

Proving this unique property requires a series of geometric postulates and theorems. One approach involves using the fact that the opposite sides of a parallelogram are parallel and congruent. By drawing a parallel line through the intersection point of the diagonals, it can be shown that the diagonals are perpendicular bisectors of each other.

Another proof technique relies on the angle properties of squares and rhombuses. Since the diagonals of a square or rhombus bisect the angles, it can be shown that the diagonals are perpendicular to each other using the fact that the sum of the angles in a quadrilateral is 360 degrees.

The property of diagonals as perpendicular bisectors in squares and rhombuses has significant implications in both geometry and its applications. It provides a powerful tool for solving problems related to these shapes and their properties. By understanding this unique characteristic, we gain a deeper appreciation for the beauty and harmony of the geometric world.

Proving Diagonals as Perpendicular Bisectors in Squares and Rhombuses

In the realm of geometry, where precision reigns supreme, the properties of parallelograms unfold like an intricate dance. Among these properties, the role of diagonals takes center stage. In the case of squares and rhombuses, these diagonals possess a unique duality that sets them apart from their parallelogram counterparts.

Defining the Duality of Diagonals

A square and a rhombus share a common trait: their diagonals bisect each other at right angles. In other words, the diagonals intersect at a point that is equidistant from all four vertices, and they form four congruent right angles.

Proof of Intersecting Diagonals

To demonstrate this intersection, consider a square or rhombus. Draw both diagonals. Observe that the diagonals cross at a single point, O. Next, measure the lengths of the four segments formed by the diagonals. They are all equal, proving that O is the midpoint of each diagonal.

Proof of Perpendicular Diagonals

To establish the perpendicularity of the diagonals, we employ angle measurers. Measure the four angles formed by the diagonals at point O. To our surprise, they are all 90 degrees, confirming that the diagonals are perpendicular bisectors.

Significance of Diagonal Properties

The unique properties of diagonals in squares and rhombuses have profound implications in geometry. These properties enable us to deduce other relationships between the sides, angles, and diagonals. For instance, knowing that the diagonals are perpendicular, we can infer that the opposite sides are parallel.

Practical Applications

The understanding of diagonal properties finds applications in various fields. In architecture, it guides the design of symmetrical buildings and the calculation of room dimensions. In engineering, it aids in determining the stability of structures and the distribution of forces.

In essence, the diagonals of squares and rhombuses are not mere lines; they are the key players that unlock the secrets of these geometric marvels. Their unique properties serve as a testament to the interconnectedness and precision that define the world of geometry.

Definition of adjacent angles adding up to 180 degrees

6. Proving Supplementary Adjacent Angles

Picture this: you’re walking along a path that suddenly turns. You notice that the angle between the original path and the new direction is 120 degrees. As you continue walking, you encounter another path branching off in an opposite direction.

Wait a minute! That angle looks awfully familiar. It’s also 120 degrees! It’s like the original angle was reflected across an invisible line running through the point where the paths meet.

This observation is a key part of proving that the adjacent angles formed by two intersecting lines are supplementary, meaning they add up to 180 degrees. The secret lies in understanding that these angles are alternate interior angles, which are congruent when parallel lines are cut by a transversal.

Imagine two parallel lines, line AB and line CD, crossed by a transversal line EF. We create two adjacent angles on one side, ∠A1 and ∠B1, and two on the other side, ∠D1 and ∠C1.

Now, because line AB is parallel to line CD, ∠A1 and ∠C1 are alternate interior angles and are therefore congruent. Similarly, ∠B1 and ∠D1 are congruent.

So, we have:

∠A1 + ∠B1 = 180° (because they are adjacent)
∠B1 + ∠D1 = 180° (because they are adjacent)

But since ∠B1 is congruent to ∠C1 and ∠B1 is congruent to ∠D1, we can substitute:

∠A1 + ∠C1 = 180°
∠C1 + ∠D1 = 180°

Adding these two equations together, we get:

∠A1 + ∠C1 + ∠C1 + ∠D1 = 360°
∠A1 + ∠D1 = 180°

Therefore, the adjacent angles ∠A1 and ∠D1 are supplementary, and the same goes for ∠B1 and ∠C1.

Understanding the Supplementary Nature of Adjacent Angles in Parallelograms

In the realm of geometry, parallelograms stand tall as quadrilateral shapes with a captivating set of properties. One such characteristic that sets parallelograms apart is the supplementary relationship between their adjacent angles. That is, the angles that share a vertex and a side in a parallelogram always add up to 180 degrees.

The Magic of Parallel Lines and Alternate Interior Angles

To unravel the mystery behind this intriguing property, we delve into the world of parallel lines and alternate interior angles. Imagine two parallel lines intersected by a transversal (a line that intersects both parallel lines). When this occurs, special angle relationships emerge.

When a transversal intersects two parallel lines, the alternate interior angles formed on the same side of the transversal are congruent. In other words, they have the same measure. This fundamental property serves as the cornerstone for proving the supplementary nature of adjacent angles in parallelograms.

Step-by-Step Proof

Let’s embark on a logical journey to demonstrate this fascinating property:

Draw a parallelogram and label its vertices as A, B, C, and D.
Draw a transversal that intersects sides AB and CD at points E and F, respectively.
Since AB and CD are parallel, ∠AEF and ∠DFC are alternate interior angles and therefore congruent.
Likewise, ∠EBC and ∠FDC are also alternate interior angles and therefore congruent.
The adjacent angles in the parallelogram are ∠AEB and ∠EBC, and they share side EB.
Since ∠AEF and ∠EBC are congruent, and ∠AEB and ∠EBC form a straight line, we can conclude that ∠AEB + ∠EBC = ∠AEF + ∠EBC = 180 degrees.

Therefore, we have proven that the adjacent angles in a parallelogram are supplementary, adding up to 180 degrees. This crucial property underpins many other significant characteristics of parallelograms, making it an essential concept in the study of geometry.

Explanation of the formula for calculating the area of a parallelogram

Proving the Area Formula: A = bh

In the realm of geometry, where shapes dance and measurements reign, parallelograms emerge as fascinating figures with unique properties. One such property is their area, the measure of the space they occupy within their boundaries. And as we delve into the enchanting world of mathematics, we shall uncover the intricacies of deriving the parallelogram area formula.

Imagine a parallelogram before our eyes, its sides stretching out like two pairs of parallel lines. Intersecting diagonals slash through the shape, forming a captivating cross. These diagonals, like magic wands, possess the remarkable ability to bisect each other at their exact midpoints.

Now, let’s unveil the area formula for this enigmatic shape. It turns out that the area of a parallelogram is equal to the product of its base (b) and height (h). But how do we prove this geometric gem?

Grab a pencil and paper, and let us embark on a journey of proof. Consider a parallelogram with its base along the bottom and its height extending upwards like a skyscraper. Drawing a perpendicular line from the top of the height to the opposite base creates a right triangle.

Observe that the height of the parallelogram is also the height of this right triangle. The base of the parallelogram, in turn, forms the base of the right triangle. Eureka! We have uncovered a key insight: the area of the parallelogram is equivalent to the area of the right triangle.

And as we all know, the area of a right triangle is effortlessly calculated using the formula: Area = (1/2) * base * height. So, by simply substituting the base and height of the parallelogram, we arrive at the sought-after parallelogram area formula:

A = (1/2) * b * h

With this formula in our arsenal, we can now confidently determine the area of any parallelogram that graces our geometry books or the world around us. The harmony between diagonals, bases, and heights becomes a powerful tool for unraveling the secrets of these multifaceted shapes.

Step-by-step proof using perpendicular height and base measurements

Unveiling the Secrets of Parallelograms: A Step-by-Step Exploration

Prepare to unravel the enigmatic world of parallelograms, a captivating shape that holds secrets begging to be discovered. Join us on an enchanting journey to understand the fundamental properties that define these intriguing geometric figures.

Proof of the Area Formula: A Symphony of Measurements

One of the most pivotal properties of parallelograms lies in their area formula. The formula, A = bh, beckons us to delve into the world of heights and bases. Imagine yourself with a parallelogram before you, a shape that resembles a diamond with four sides.

To uncover the essence of this formula, let’s dissect a parallelogram into two right triangles. Each triangle shares the same base as the parallelogram, let’s call it “b“, the distance across the parallelogram’s width. Now, the crucial step: we introduce the concept of perpendicular height, represented by “h“. This height represents the vertical distance from the base to the opposite side.

With these two components in play, we can unfold the proof. The area of a triangle is given by the formula A = (1/2) * b * h. Since the parallelogram is composed of two congruent triangles, its area is simply the sum of these two triangular areas. Therefore, A = (1/2) * b * h + (1/2) * b * h = b * h.

And there it is! The formula A = bh emerges as a beacon of geometric enlightenment, revealing the connection between a parallelogram’s base and height. This formula empowers us to calculate the area of any parallelogram with ease, making it an invaluable tool for architects, engineers, and artists alike.

Discovering the Secrets of Parallelograms: A Geometry Journey

In the realm of geometry, parallelograms hold a special place, their unique properties setting them apart. Let’s embark on a journey to unravel these secrets, exploring the proofs and applications that unlock their geometrical wonders.

Defining the Parallelogram

A parallelogram is a four-sided figure with two pairs of parallel sides. These sides are equal in length, giving it a distinctive shape. Parallelograms are the foundation for a variety of geometric shapes, such as rectangles, squares, and rhombuses.

Revealing Congruent Sides and Angles

One of the key properties of parallelograms is that opposite sides are congruent, meaning they are equal in length. This follows from the definition of parallel sides and can be proven through measurement or using geometric postulates.

Similarly, opposite angles in a parallelogram are also congruent, equal in measure. This is evident when we draw the diagonals of the parallelogram, forming four triangles. The angles opposite each other are equal due to the properties of triangles.

Intersecting and Bisecting Diagonals

Diagonals are line segments that connect opposite vertices in a parallelogram. They possess a fascinating property: they intersect at their midpoints. This means that the point where the diagonals cross divides each diagonal into two equal parts. Proving this property requires drawing and measuring the diagonals.

Unique Properties of Squares and Rhombuses

Squares and rhombuses are special types of parallelograms with additional properties. In a square, the diagonals are perpendicular bisectors, meaning they intersect at right angles and bisect each other. Similarly, in a rhombus, the diagonals are perpendicular bisectors.

Supplementary Adjacent Angles

Another key property of parallelograms is that adjacent angles add up to 180 degrees. This means that when two sides of a parallelogram are parallel, the angles between those sides and the third side add up to a straight line. This property can be proven using the concept of parallel lines and alternate interior angles.

Formulas for Area and Perimeter

The area of a parallelogram can be calculated using the formula A = bh, where b is the length of the base and h is the height or perpendicular distance between the base and the opposite side.

The perimeter of a parallelogram is the sum of the lengths of all four sides, which can be expressed as P = 2(l + w), where l is the length and w is the width.

Applications and Examples

The properties of parallelograms have numerous applications in real-world situations. Architects use them to design buildings with symmetrical facades. Engineers employ them to analyze forces and stresses in structures. Artists utilize them to create balanced and harmonious compositions. From architecture to physics to art, parallelograms are essential tools for understanding and shaping our world.

Proof techniques for calculating the perimeter using the length and width

Proving the Perimeter Formula for Parallelograms

In the realm of geometry, parallelograms hold a unique place, characterized by their distinctive shape and remarkable properties. Among these properties, the perimeter formula shines as a fundamental concept essential for understanding and applying parallelogram principles in real-world situations.

Defining Perimeter

The perimeter of any shape is the total length surrounding its boundary. For a parallelogram, this means calculating the sum of the lengths of all four sides. The formula that governs this calculation, P = 2(l + w), where P is the perimeter, l is the length, and w is the width, is a cornerstone of parallelogram geometry.

Proof by Measurement

One way to prove the perimeter formula is through direct measurement. By measuring the length and width of a parallelogram and adding these values together, we can obtain the perimeter. This method, though straightforward, assumes accurate measurements and may not always be practical in real-life applications.

Proof by Properties

A more rigorous approach to proving the perimeter formula involves utilizing the geometric properties of parallelograms. We know that opposite sides of a parallelogram are congruent, meaning they have the same length. Therefore, we can represent the length as 2l and the width as 2w.

Using this relation, we can substitute in the formula as follows:

P = 2(l + w)
P = 2(2l + 2w)
P = 4(l + w)

Simplifying further, we arrive at the desired result: P = 2(l + w).

Applications and Examples

The perimeter formula for parallelograms finds practical applications in various fields. Architects use it to calculate the total length of fencing needed to enclose a garden or playground in the shape of a parallelogram. Designers employ it to determine the amount of trim required for a parallelogram-shaped window frame.

In conclusion, the perimeter formula for parallelograms, P = 2(l + w), is a fundamental concept in geometry that helps us understand and apply parallelogram properties in practical settings. By proving this formula through measurement or geometric properties, we equip ourselves with a powerful tool for solving real-world problems involving parallelograms.

Unveiling the Secrets of Parallelograms: A Geometric Odyssey

In the realm of geometry, parallelograms stand out as alluring quadrilaterals with a captivating array of properties. Join us on an enlightening journey as we delve into the fascinating world of parallelograms, unraveling their intriguing characteristics with practical examples that bring geometry to life.

Opposite Sides: A Tale of Equality

Parallelograms are defined by their opposite sides being congruent. This means that these opposing sides have the same length. Proving this property involves precise measurements and the application of geometric postulates, ensuring the accuracy of our conclusion.

Opposite Angles: A Balancing Act

The opposite angles in a parallelogram are like twins, always congruent in measure. This intriguing property can be proven using angle measurers or by utilizing the fundamental properties of angles. These congruent angles contribute to the unique symmetry that characterizes parallelograms.

Intersecting Diagonals: A Midpoint Rendezvous

Parallelogram diagonals possess a remarkable characteristic: they intersect at their midpoints. This bisecting property is a geometric marvel that can be demonstrated through careful drawing and precise measurements. The intersection point divides the diagonals into two equal segments, revealing the inherent balance within a parallelogram.

Diagonals in Squares and Rhombuses: A Perpendicular Embrace

In the exclusive realm of squares and rhombuses, the diagonals take on an even more remarkable role. Not only do they bisect each other, but they also intersect perpendicularly, forming right angles. This unique property underscores the exceptional symmetry and elegance of these special parallelograms.

Supplementary Adjacent Angles: A Harmonic Union

Adjacent angles in a parallelogram are like harmonious melodies that sum up to 180 degrees. This supplementary relationship stems from the presence of parallel lines and alternate interior angles. Proving this property sheds light on the intricate interplay between angles and their geometric significance.

Area and Perimeter: Unveiling the Dimensions

The area of a parallelogram is calculated using the formula A = bh, where b represents the base and h the perpendicular height. This formula empowers us to determine the enclosed space within a parallelogram. Similarly, the perimeter is calculated by adding the lengths of all four sides, expressed as P = 2(l + w). These formulas provide valuable insights into the geometric dimensions of parallelograms.

Applications: Geometry in Action

The properties of parallelograms extend beyond the confines of theoretical geometry. They find practical applications in various fields:

Architecture: Parallelograms shape buildings, creating visually appealing and structurally sound designs.
Engineering: Parallelograms optimize strength and stability in bridges and other structures.
Art and Design: Parallelograms enhance patterns and compositions, adding depth and rhythm to visual creations.

Parallelograms, with their rich tapestry of properties, stand as compelling examples of the beauty and practicality of geometry. Their intriguing characteristics provide a foundation for understanding more complex geometric concepts. By unraveling the secrets of parallelograms, we gain a deeper appreciation for the elegance and power of mathematical knowledge.

Understanding the Significance of Parallelogram Proofs: A Journey into Geometry

In the realm of geometry, parallelograms stand out as intriguing shapes with captivating properties. Proving these properties is not merely an academic exercise but a vital step in unlocking the secrets of this fascinating shape. Let’s embark on a storytelling journey to unravel the importance of parallelogram proofs.

Unveiling the Essence of Parallelograms

Our story begins with understanding what a parallelogram is. It’s a quadrilateral with opposite sides that are parallel and congruent. This unique characteristic sets it apart from other quadrilaterals.

Equal Sides and Angles: A Harmonious Pairing

The proof that opposite sides and angles of a parallelogram are congruent lays the foundation for further discoveries. By establishing this equality, we lay the groundwork for a symmetrical figure with a balanced appearance.

Diagonals as Intersecting Guides

The diagonals of a parallelogram play a pivotal role. Proofs reveal that they intersect at their midpoints, dividing the parallelogram into four congruent triangles. This property guides us in understanding the internal structure of parallelograms.

Perpendicular Bisectors: A Unique Distinction

In the case of squares and rhombuses, a special relationship between the diagonals exists. Proofs demonstrate that the diagonals are not only perpendicular to each other but also bisect each other, forming four right angles at their point of intersection. This unique characteristic adds an extra layer of symmetry to these special parallelograms.

Adjacent Angles: A Supplementary Bond

Adjacent angles in a parallelogram form a supplementary pair, adding up to 180 degrees. Proving this property strengthens our understanding of the angles in a parallelogram and their relationship to parallel lines.

Area and Perimeter: Quantitative Insights

The area of a parallelogram can be calculated using the formula A = bh, where b is the base and h is the height. Proofs validate this formula, providing a practical tool for finding the area of parallelograms. Similarly, the perimeter formula P = 2(l + w), where l is the length and w is the width, is derived through proofs, enabling us to determine the total length of the sides.

Applications in the Real World: A Practical Impact

The proofs we’ve explored extend beyond theoretical concepts. They have significant applications in various fields. For instance, in architecture, understanding the properties of parallelograms helps in designing symmetrical buildings and structures. In art, parallelograms form the basis of geometric patterns and designs.

By unraveling the proofs of parallelogram properties, we delve into the intricate world of geometry. These proofs serve as stepping stones, guiding us in comprehending more complex shapes and their relationships, empowering us with a deeper understanding of the geometric world around us.

Proving Parallelograms: Key Properties And Geometric Applications