Unlocking The Secrets Of The Point Of Tangency: Its Significance In Geometry And Beyond

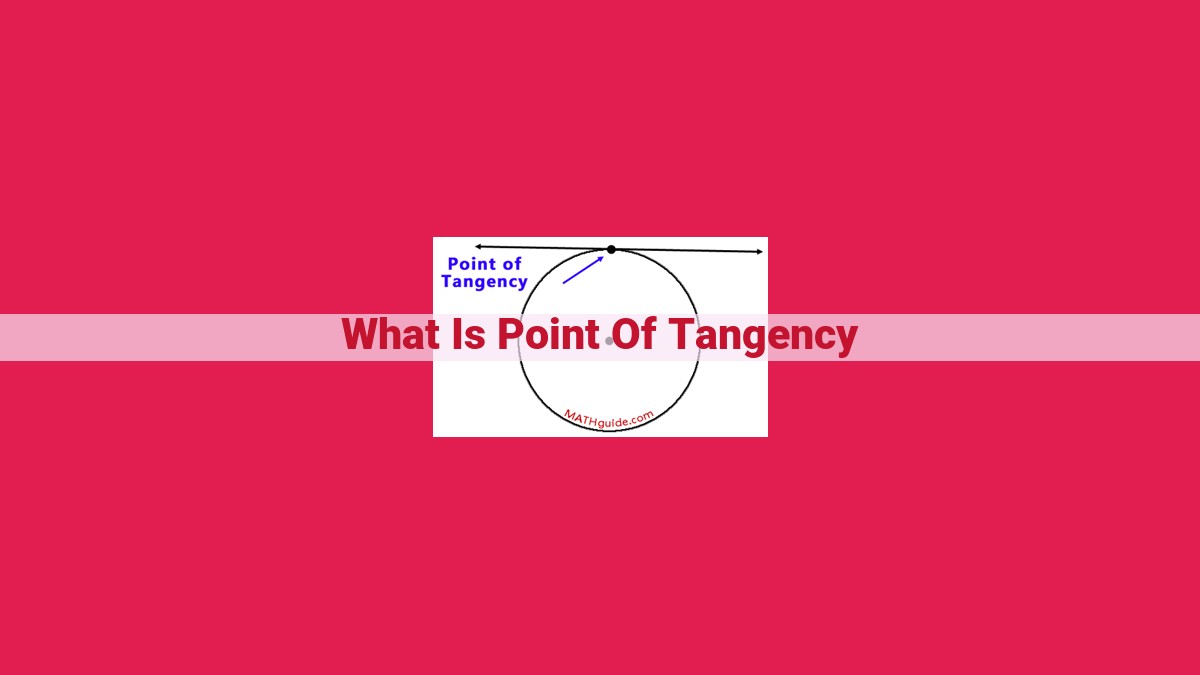
The point of tangency, where a line or plane just touches a curve or surface, holds significant importance in geometry. It represents the instant of contact, marking the point where the tangent line is perpendicular to the normal line at that point. Understanding the point of tangency is crucial for analyzing curves and planes, determining curvature and slope, and modeling motion and forces in physics and engineering.

Point of Tangency: A Gateway to Understanding Geometry and Beyond

Imagine a graceful curve, like the smooth arc of a rainbow. At a single point, this curve meets a straight line, kissing it gently. This meeting point is known as the point of tangency, a concept of profound significance in geometry.

Defining the Point of Tangency

A point of tangency is the point where a curve and a straight line intersect, such that the straight line is tangent to the curve. The tangent line is a line that touches the curve at only one point and doesn’t cross it.

Cruciality of Understanding the Concept

Understanding the point of tangency is crucial for several reasons:

• It unlocks a deeper comprehension of curves and their behavior.
• It enables us to analyze the curvature and slope of curves.
• It forms the foundation for advanced geometric concepts, such as derivatives and integrals.

Definition and Properties of the Point of Tangency

A point of tangency is the point at which a line or curve touches another line or curve without crossing it. It’s a crucial concept in geometry, as it helps us understand the relationship between two objects.

We define a point of tangency as the point where the tangent line to a curve at that point intersects the curve. The tangent line is the line that is parallel to the curve at that point.

There are several important properties of the tangent line and its relationship to the curve at the point of tangency:

• The tangent line is perpendicular to the normal line to the curve at that point.
• The slope of the tangent line is equal to the derivative of the curve at that point.
• The radius of curvature of the curve at that point is inversely proportional to the slope of the tangent line.

These properties make the point of tangency a useful tool for analyzing curves and planes. By understanding the properties of the tangent line, we can learn more about the behavior of the curve at that point.

Significance in Analysis

• Explain how the point of tangency is used to analyze curves and planes.
• Provide examples of how to use the tangent line to determine curvature and slope.

Significance in Analysis: The Power of Tangents

In the realm of geometry, the point of tangency holds immense significance in analyzing curves and planes. It serves as a crucial tool for mathematicians, physicists, and engineers alike, providing insights into the behavior and characteristics of various surfaces.

The tangent line at a point of tangency is a straight line that touches the curve or plane at a single point. This line is perpendicular to the normal line, which is a line passing through the point of tangency and perpendicular to the curve or plane at that point. Understanding the relationship between the tangent line and the curve allows us to delve deeper into the properties of the surface.

One key application of the point of tangency is in determining the curvature of a curve. The curvature measures the rate at which a curve changes direction. The tangent line at a point of tangency provides a local approximation of the curve, and the angle between the tangent lines at two nearby points of tangency gives an estimate of the curvature. By examining the curvature, we can gain insights into the behavior of the curve and its overall shape.

Another important aspect of analysis is the slope of a curve at a given point. The slope represents the rate of change of the curve with respect to the horizontal axis. The tangent line at a point of tangency provides a direct measure of the slope at that point. By calculating the slopes at various points of tangency, we can construct a graph that shows how the slope of the curve changes over the entire domain. This information can be crucial for understanding the behavior of the curve and its potential applications.

The Tangent’s Tale: A World of Physical Applications

The point of tangency, a special place where a curve and a line meet gently, plays a pivotal role in the realm of physics and engineering. It’s here, at this delicate intersection, that we unravel the secrets of motion and the forces that shape our world.

Consider the graceful arc of a projectile soaring through the air. As it traces its path, its trajectory intersects a tangent line at its highest point. This point of tangency marks the moment of zero velocity, the instant when the projectile’s upward momentum gives way to gravity’s relentless pull. By analyzing the slope of this tangent line, we can decipher the projectile’s speed and direction at that particular moment.

Moving beyond celestial mechanics, the point of tangency also finds its footing in the world of structures. Imagine a beam supported at both ends. As a load is applied, the beam bows under the weight. At the point of tangency, where the beam meets the supporting surface, the shear forces are at their peak. By studying this point, engineers can design structures that can withstand extreme loads without collapsing.

Another captivating application of the point of tangency lies in the field of fluid dynamics. When a fluid flows over a surface, it creates a velocity gradient. The tangent line at a given point on the surface represents the direction of flow at that point. By analyzing the tangent lines at various points along the surface, engineers can optimize the design of aircraft wings, ship hulls, and other objects that interact with fluids to minimize drag and maximize efficiency.

The point of tangency, seemingly a simple geometric concept, holds immense power in the world of physics and engineering. It’s a crucial tool for understanding motion, analyzing forces, and designing structures that can withstand the test of time. So next time you’re admiring the smooth curve of a racetrack or marveling at the graceful flight of a bird, remember the unsung hero behind it all: the point of tangency, the silent guide that helps us unravel the mysteries of our physical world.

Examples of Point of Tangency Applications

In the realm of geometry, the concept of a point of tangency shines as a beacon of elegance and practical significance. Its applications extend far beyond the pages of textbooks, into the dynamic world of engineering, physics, and everyday encounters. Let’s explore some captivating examples that illustrate the power and versatility of this geometrical cornerstone.

Engineering Marvels:

The Eiffel Tower, a towering testament to human ingenuity, stands tall with its graceful curves. At the point where each curved beam meets the horizontal supports, the point of tangency dictates the tower’s stability. Engineers meticulously calculated these points to ensure the tower’s ability to withstand wind forces and maintain its iconic shape.

Automotive Innovations:

In the realm of automotive design, the concept of tangency plays a crucial role. The sleek and aerodynamic curves of a car’s body are carefully engineered to reduce drag. The point of tangency where the front bumper meets the hood optimizes airflow, minimizing resistance and enhancing fuel efficiency.

Motion Analysis in Physics:

The point of tangency also finds its place in the intricate world of physics. It aids in analyzing the motion of objects that follow curved trajectories, such as a thrown projectile or a swinging pendulum. By determining the point of tangency on the path of the object, scientists can pinpoint the moment when its velocity is perpendicular to the curve.

Everyday Applications:

Even in our daily routines, we encounter the point of tangency. Think of the humble bicycle wheel. The point where the tire makes contact with the ground is the point of tangency. Understanding this point is crucial for optimizing traction and balancing the forces acting on the bike.

Finding and Interpreting the Point of Tangency:

Identifying and interpreting the point of tangency in real-world situations can be a rewarding endeavor. Often, it involves employing mathematical concepts such as derivatives and differential calculus. However, in many cases, a keen eye and a grasp of the underlying geometry can suffice. By carefully observing the shape and curvature of an object, you can often pinpoint the point of tangency, unlocking a wealth of valuable insights.