How To Determine The Equation Of A Perpendicular Line: A Step-By-Step Guide

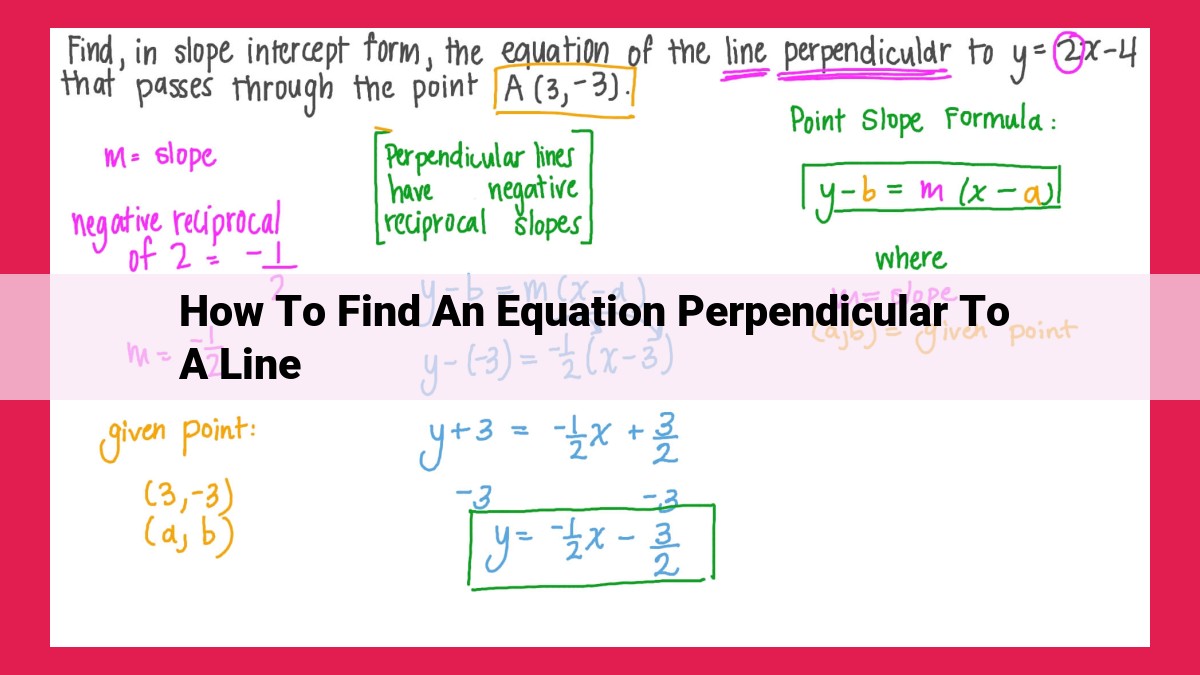
To find the equation of a line perpendicular to a given line, determine the slope of the given line. The slope of the perpendicular line will be the negative reciprocal of the given line’s slope. Use point-slope form to write the equation of the perpendicular line using a point on the given line and its slope. To convert to slope-intercept form, solve for y and rearrange. This method provides a systematic approach to finding equations of perpendicular lines, which is useful in geometry, architecture, and other fields where angle relationships are crucial.

Unlocking the Power of Perpendicular Lines: A Journey into Geometry and Beyond

In the realm of geometry, perpendicular lines reign supreme, their perpendicularity forming the cornerstone of countless applications in our world. Join us on an enlightening journey as we explore the intriguing relationship between perpendicular lines and slope—the measure of a line’s steepness. Along the way, we’ll unravel the mysteries of line equations, enabling you to confidently find the equation of a line perpendicular to any given line.

Perpendicular Lines: The Cornerstone of Geometry

Imagine a city grid, where streets intersect at right angles, forming neat perpendicular lines. These perpendicular lines create an organized and navigable system, allowing us to determine distances and navigate with ease. In geometry, perpendicular lines hold the same fundamental importance. They are defined as lines that intersect at a right angle, forming a 90-degree angle.

In real-world applications, perpendicular lines play a crucial role in architecture, engineering, and design. From towering skyscrapers to intricately designed bridges, perpendicular lines ensure structural stability and aesthetic appeal. They help us create square corners, level surfaces, and symmetrical patterns, bringing harmony and order to our surroundings.

The Enigma of Slope: Measuring Line Steepness

To fully grasp the relationship between perpendicular lines, we must first understand the concept of slope. Slope is a numerical value that measures the steepness of a line. It is calculated as the ratio of the change in vertical height (y-axis) to the change in horizontal distance (x-axis). Lines with a positive slope rise from left to right, while lines with a negative slope fall from left to right. Horizontal lines have a slope of 0, while vertical lines have an undefined slope.

Unraveling the Secret of Perpendicular Lines

Here lies the key to our exploration: the connection between perpendicular lines and slope. Perpendicular lines have a remarkable property—their slopes are negative reciprocals of each other. In simpler terms, if one line has a slope of m, the slope of its perpendicular line will be -1/m. This inverse relationship ensures that when two perpendicular lines intersect, they form a right angle.

Navigating the Maze of Line Equations

To effectively find the equation of a line perpendicular to a given line, we must master the language of line equations. Line equations can be expressed in various forms, including point-slope form and slope-intercept form.

Point-slope form is useful when we have a point on the line and its slope. The equation is written as:

y - y1 = m(x - x1)

where (x1, y1) is the given point and m is the slope.

Slope-intercept form is convenient when we know the line’s slope and y-intercept (the point where the line crosses the y-axis). The equation is written as:

y = mx + b

where m is the slope and b is the y-intercept.

Finding the Equation of a Perpendicular Line: A Step-by-Step Guide

Now, let’s put our knowledge into practice. To find the equation of a line perpendicular to a given line, follow these simple steps:

1. Determine the slope of the given line. If the line’s equation is in slope-intercept form (y = mx + b), the slope is simply m. Otherwise, use the point-slope form to calculate the slope.
2. Calculate the negative reciprocal of the given slope. This will be the slope of the perpendicular line.
3. Choose a point on the perpendicular line. This can be any point, but it will simplify your calculations if you choose a point that is not on the given line.
4. Write the equation of the perpendicular line in point-slope form. Use the point you chose and the slope you calculated in step 2.
5. Convert the equation to slope-intercept form (optional). This is useful if you want to find the y-intercept of the perpendicular line.

Perpendicular lines and slope are intertwined concepts that provide a powerful tool for understanding and manipulating lines. The ability to find the equation of a perpendicular line is essential in various fields, including architecture, engineering, design, and surveying. By mastering this skill, you can harness the power of geometry to unlock countless possibilities.

Understanding the Essence of Perpendicular Lines: A Geometric Journey

In the realm of geometry, the concept of perpendicular lines holds immense significance, both in theoretical understanding and practical applications. It’s a tale of lines intersecting at right angles, creating a symphony of perpendicularity.

a. The Tale of Slope: Measuring a Line’s Steepness

Imagine a line traversing the plane like a daring adventurer. Its slope, a numerical value, measures the line’s steepness. It’s like the slant of a hill, except here the hill is a line. A positive slope indicates a line that rises as it moves from left to right, while a negative slope tells the tale of a line descending.

b. Perpendicular Lines: A Dance of Right Angles

Now, let’s introduce perpendicular lines, the ballet dancers of geometry. These lines meet at a 90-degree angle, forming a perfect right triangle. It’s like two lines embracing, their intersection creating a graceful ‘T’ shape.

But here’s where the magic happens: perpendicular lines have a special relationship with their slopes. The slopes of perpendicular lines are negative reciprocals of each other. What does that mean? It’s like a game of opposites. If one line has a positive slope, its perpendicular partner will have an equal and opposite negative slope. It’s like balancing scales, where one side goes up while the other goes down to maintain equilibrium.

Slope of Perpendicular Lines: The Negative Reciprocal Rule

In the realm of geometry, perpendicular lines hold a pivotal position, intersecting each other at right angles (90 degrees). Understanding their behavior is crucial for unraveling various problems in geometry and beyond. One of the most fundamental properties of perpendicular lines is the negative reciprocal rule for their slopes.

Slope and its Significance

Before delving into the negative reciprocal rule, let’s first shed light on the concept of slope. Slope measures the steepness or inclination of a line. It is defined as the ratio of the vertical change (rise) to the horizontal change (run) between any two points on the line. A line with a steeper incline has a greater slope.

Negative Reciprocal Rule

Now, let’s unveil the intriguing negative reciprocal rule. For two perpendicular lines, their slopes are not just any numbers; they are specifically linked by this rule. The slope of one line is the negative reciprocal of the slope of the other. This means that if one line has a positive slope, the perpendicular line has a corresponding negative slope, and vice versa.

Imagine two lines, Line A and Line B, that intersect perpendicularly. Let’s denote their slopes as m_A and m_B, respectively. According to the negative reciprocal rule, we have:

m_A = -1/m_B

In other words, m_A is the negative reciprocal of m_B. This relationship provides us with a powerful tool for analyzing perpendicular lines.

Example

Consider the following example:

• Line A: passes through points (2, 3) and (4, 7)
• Line B: is perpendicular to Line A

To find the slope of Line A, we use the slope formula:

m_A = (y_2 - y_1) / (x_2 - x_1)
= (7 - 3) / (4 - 2)
= 4 / 2
= 2

Now, applying the negative reciprocal rule, the slope of Line B will be:

m_B = -1/m_A
= -1/2

Therefore, the slope of Line B is -1/2, confirming the negative reciprocal relationship.

Unveiling the Secrets of Line Equations: Exploring Point-Slope and Slope-Intercept Forms

In the realm of geometry, lines play a pivotal role, and understanding their equations is paramount. Among the various line equations, point-slope form and slope-intercept form stand out as essential tools in describing the relationship between points on a line and its slope.

Point-Slope Form: Capturing the Essence of a Line

The point-slope form of a line equation revolves around a specific point on the line and its slope. This form reads as:

y - y1 = m(x - x1)

where:

• (x1, y1) represents the given point on the line
• m is the slope of the line

To illustrate, let’s consider a line passing through the point (2, 3) with a slope of 2. Using the point-slope form, the equation of the line becomes:

y - 3 = 2(x - 2)

Slope-Intercept Form: Unveiling the Line’s Trajectory

The slope-intercept form of a line equation offers a different perspective. This form highlights the slope and the y-intercept of the line, expressed as:

y = mx + b

where:

• m is the slope of the line
• b is the y-intercept (the point where the line intersects the y-axis)

Returning to our previous example, the equation of the line in slope-intercept form would be:

y = 2x + 1

These two forms provide powerful tools for describing and analyzing lines. So, the next time you encounter a line equation, you’ll be equipped with the knowledge to decipher its secrets and uncover the underlying geometry.

Unveiling the Equation of a Line: Embarking on a Journey in Point-Slope Form

In the realm of geometry and beyond, lines have always fascinated us with their mesmerizing symmetries and practical applications. When it comes to understanding the intricacies of lines, the concept of perpendicularity stands out as a fundamental pillar. Dive into this enlightening journey as we unravel the secrets of finding the equation of a perpendicular line, employing the versatile point-slope form.

Point-Slope Form: A Gateway to Line Equations

Imagine a line stretching out before you, like an uncharted path waiting to be explored. The point-slope form, akin to a guiding light, empowers us to illuminate this path, transforming it into an equation that precisely captures its essence.

To embark on this journey, you’ll need two essential pieces of information: a point ((x_1, y_1)) that lies on the line and the slope (_m_) that governs its inclination. With these beacons in hand, you can craft the equation using the following formula:

**\((y - y_1) = m(x - x_1)\)**

This equation serves as a blueprint for the line, describing the relationship between any point _((x, y)) on the line and the given point _((x_1, y_1)).

Illustrating the Point-Slope Form in Action

Let’s embark on a practical example to solidify our understanding. Suppose we have a line passing through the point ((2, 5)) with a slope of _3. Using the point-slope form, we can write the equation of this line as:

**\((y - 5) = 3(x - 2)\)**

This equation precisely captures the geometry of the line, providing a roadmap for locating any point that resides on it.

The Equation of a Perpendicular Line: Unveiling the Hidden Connection

Now, let’s delve into the captivating world of perpendicular lines. When two lines intersect, forming a right angle, their slopes engage in an intriguing dance. The slope of a perpendicular line is always the negative reciprocal of the original line’s slope.

For instance, if our original line has a slope of 3, its perpendicular counterpart will have a slope of -1/3. This mathematical relationship opens a gateway to finding the equation of a perpendicular line with ease.

The ability to find the equation of perpendicular lines unlocks a treasure chest of practical applications across diverse fields. In engineering, architects rely on perpendicular lines to ensure structural stability. In design, artists utilize them to create visually appealing compositions. And in countless other realms, perpendicular lines play a pivotal role in problem-solving and innovation.

Mastering the point-slope form empowers you to navigate the world of geometry with confidence, unraveling the mysteries of perpendicular lines and unlocking their transformative potential in the real world.

Slope-Intercept Form of a Line: Simplifying Line Equations

In the realm of geometry, the equation of a line is a crucial tool that describes its path and behavior. One of the most common and useful forms of a line equation is the slope-intercept form. This form provides a straightforward representation of a line’s slope and y-intercept, making it easy to analyze and understand.

The slope-intercept form of a line equation is written as:

y = mx + b

where:

• y represents the dependent variable, which is the vertical coordinate of any point on the line.
• x represents the independent variable, which is the horizontal coordinate of any point on the line.
• m represents the slope of the line, which measures its steepness.
• b represents the y-intercept of the line, which is the point where the line crosses the y-axis.

Understanding the slope-intercept form is essential for manipulating and interpreting line equations. The slope, m, determines the line’s direction and steepness. A positive slope indicates a line that rises from left to right, while a negative slope indicates a line that falls from left to right. The y-intercept, b, represents the point where the line intersects the y-axis, providing insight into the line’s vertical position.

Finding the Equation of a Perpendicular Line

In the tapestry of geometry, understanding the relationship between perpendicular lines is paramount. Whether in the intricate blueprints of skyscrapers or the harmonious curves of nature’s designs, perpendicular lines play a crucial role in creating structure, balance, and aesthetic appeal.

To delve deeper into this fascinating concept, let’s embark on a journey to discover the secrets of perpendicular lines and unravel the method to calculate their equations.

Step 1: Determine the Slope

The slope of a line measures its steepness. For perpendicular lines, their slopes are intimately intertwined. Imagine two intersecting lines forming an “X” shape. The slope of one line is the negative reciprocal of the slope of the other. If one line has a slope of 2, for instance, its perpendicular counterpart will have a slope of -1/2.

Step 2: Write the Equation in Point-Slope Form

The point-slope form of a line equation is:

y - y1 = m(x - x1)

where:

• (x1, y1) is a point on the line
• “m” is the slope of the line

For a perpendicular line, we already know the slope and have a point on the given line. Plugging these values into the point-slope form, we can write the equation of the perpendicular line.

Step 3: Convert to Slope-Intercept Form (Optional)

The slope-intercept form of a line equation is:

y = mx + b

where:

• “m” is the slope of the line
• “b” is the y-intercept (where the line crosses the y-axis)

To convert the point-slope form to slope-intercept form, we can solve for “b” by subtracting “mx” from both sides of the equation.

Mastering the art of finding the equation of a perpendicular line empowers us with a valuable tool for shaping our surroundings. From designing bridges that defy gravity to creating architectural wonders that inspire awe, understanding perpendicularity is essential in countless fields. The next time you encounter lines that meet at right angles, remember the steps outlined in this guide and unlock the secrets of geometry’s captivating dance.