Discover The Period Of The Cosecant Function: A Guide To Its 2Π Rhythm

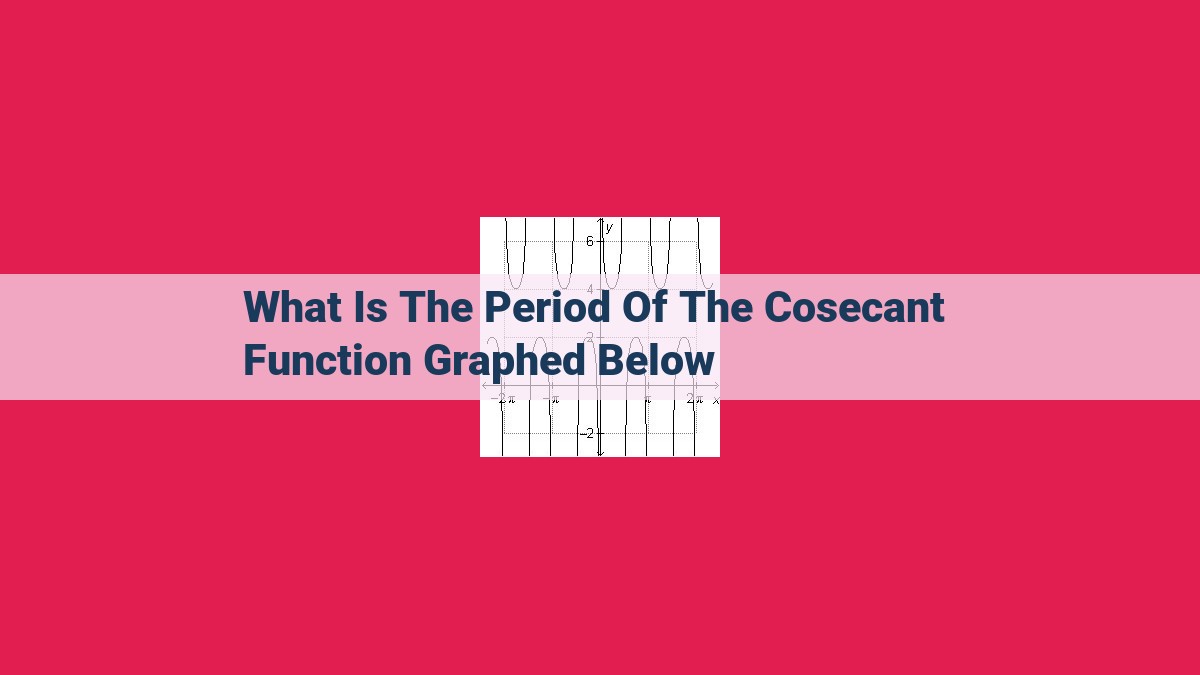
The period of the cosecant function is the horizontal distance between any two consecutive peaks or troughs. It represents the time it takes for one complete cycle of the function. In the given graph, the distance between any two adjacent peaks (maxima) is 2π units, indicating that the period of the cosecant function graphed is 2π.

Understanding Amplitude: The Measure of Vertical Fluctuations

In the realm of trigonometry, functions like cosecant describe periodic oscillations, resembling waves that ebb and flow. A crucial aspect of these waves is their amplitude, which captures the extent of their vertical movement.

Amplitude, represented by the letter A, is the measure of the vertical distance between the highest and lowest points of a cosecant wave. It provides a numerical value that quantifies the “height” of these oscillations. A larger amplitude indicates more pronounced fluctuations, while a smaller amplitude results in shallower waves.

By understanding amplitude, we gain insights into the intensity of the cosecant function. A cosecant wave with a large amplitude will have sharp peaks and deep troughs, while a wave with a small amplitude will exhibit more gentle variations. This knowledge is fundamental for analyzing the behavior of trigonometric functions and predicting their graphical representations.

Real-World Applications

The concept of amplitude finds practical applications in various fields:

• Music: Amplitude determines the loudness of a musical note. Instruments with higher amplitudes produce louder sounds.
• Physics: In wave phenomena, amplitude represents the energy or intensity of the wave. Larger amplitude waves carry more energy.
• Acoustics: Amplitude is crucial in designing sound systems to ensure optimal volume and clarity.

Understanding amplitude empowers us to delve deeper into the intricacies of trigonometric functions and their versatile applications. By grasping this fundamental concept, we unlock the key to deciphering the patterns and predicting the behavior of these essential mathematical tools.

Period: The Rhythm of Trigonometric Motion

In the symphony of trigonometric functions, the cosecant dances with a distinct rhythm that we call its period. Imagine a pendulum swinging back and forth, tracing out a graceful arc. The period of the pendulum is the time it takes for the bob to move from its maximum height, through its lowest point, and back to its maximum height again.

Similarly, the period of the cosecant function is the horizontal distance between any two consecutive peaks or troughs on its graph. This distance represents the time it takes for the function to complete one full cycle.

Think of a wave crashing on the shore. The period of the wave is the time between the arrival of two consecutive crests. In the graph of the cosecant function, the peaks and troughs correspond to the crests and troughs of the wave. The period of the cosecant function, therefore, tells us how long it takes for the function to complete one oscillation, or wave.

Understanding the period of the cosecant function is crucial for analyzing periodic phenomena such as sound waves, light waves, and even the heartbeat. By knowing the period, we can predict when the function will reach its maximum or minimum values, and how often it will repeat its pattern.

Phase Shift: A Journey Along the X-Axis

In the realm of graphs, the cosecant function embarks on a captivating dance, swaying between its maximum and minimum values. But what happens when this graceful movement is interrupted by an unexpected shift? That’s where phase shift enters the scene, introducing a fascinating twist to the cosecant’s trajectory.

Imagine the cosecant graph as a graceful wave, its peaks and troughs rising and falling rhythmically. Now, picture a mischievous fairy dusting the graph with a touch of magic, causing it to glide gracefully to the left or right along the x-axis. This magical shift is known as phase shift.

The effect on the graph is mesmerizing. The starting point of the wave shifts in time, altering the initial position of its peaks and troughs. If the shift is to the left, the graph appears to start at a more advanced point in its cycle, as if it has already completed a portion of its journey. Conversely, if the shift is to the right, the graph seems to delay its ascent, as its peaks and troughs emerge later along the x-axis.

This shift has profound implications for the interpretation of the graph. Suppose we are analyzing the cosecant function of a periodic phenomenon, such as the tides. By understanding the phase shift, we can determine when the high and low tides occur relative to a specific reference point. This knowledge is invaluable for predicting the timing of these crucial events.

Phase shift is an enchanting concept that transforms the cosecant graph into a dynamic and responsive entity. It allows us to tailor the function to specific scenarios, empowering us to explore the intricate interplay between time and periodic events. So, the next time you encounter a cosecant graph, remember its hidden dance partner, phase shift, and appreciate its subtle yet profound influence on the graph’s behavior.

Vertical Shift: Moving Up or Down

• Explain that vertical shift moves the graph vertically along the y-axis.
• Discuss how it changes the midline of the graph.

Vertical Shift: Moving Up or Down

Imagine a Ferris wheel that slowly rises and lowers, carrying its passengers on an exhilarating ride. The vertical shift of a graph, like the Ferris wheel, is a transformation that moves the graph up or down along the y-axis.

Just as the Ferris wheel alters the starting height of the ride, vertical shift changes the midline of the graph. The midline, represented by a horizontal line, indicates the average value of the function.

When the graph is shifted upward, the midline moves closer to the top of the coordinate plane, increasing the minimum and maximum values of the function. Think of the Ferris wheel slowly rising, gradually lifting the passengers higher above the ground.

Conversely, when the graph is shifted downward, the midline moves closer to the bottom of the coordinate plane, decreasing the minimum and maximum values. Picture the Ferris wheel gently lowering, bringing the passengers closer to the ground.

By understanding vertical shift, we can visualize how the graph moves and the impact it has on the function’s overall behavior. It’s like being able to control the height of the Ferris wheel, adjusting the intensity of the ride for a thrilling or calming experience.

Midline: The Center Line

• Define the midline as the horizontal line through the center of the graph.
• Explain that it indicates the average value of the function.

Understanding the Midline: The Centerline of Functions

In the world of mathematics, functions are a powerful tool for modeling and analyzing patterns. They describe the relationship between an input (x) and an output (y). Every function has certain characteristics that define its behavior, and one of the most important is the midline.

The midline is a horizontal line that runs through the center of a function’s graph. It divides the graph into two equal parts and serves as a reference point for understanding the function’s behavior. The midline is crucial for visualizing the function’s average value, which is the average of all the output values.

To find the midline, simply determine the average of the minimum and maximum values of the function’s range. The midline will be located halfway between these two points. For instance, if a function’s range is from -5 to 5, the midline will be at 0 (the average of -5 and 5).

The midline is particularly significant in sinusoidal functions like the sine, cosine, and cosecant functions. These functions exhibit cyclic behavior, with their graphs repeating over a certain interval. The midline in sinusoidal functions represents the midpoint of the wave, from which the graph oscillates above and below.

Understanding the midline is essential for analyzing functions and making accurate predictions about their behavior. By identifying the midline, you can determine the function’s average value, which provides insights into its overall behavior. Furthermore, the midline helps you visualize the function’s symmetry and its relationship with other key characteristics like amplitude and period.

Unraveling the Mysteries of the Cosecant Function: A Comprehensive Guide

Embark on an enchanting journey into the world of the cosecant function, where we will unravel its secrets and decode its enigmatic properties. From its towering heights to its elusive coastline, we will explore the hidden dimensions that govern this mathematical marvel.

Amplitude: Unveiling the Vertical Scale

Picture the cosecant function as a symphony of waves, rhythmically rising and falling. The amplitude measures the grandiosity of these waves, capturing the vertical distance between the lofty peaks and the depths of the troughs. It determines the “height” of the waves, swaying the graph into mesmerizing patterns.

Period: Embracing the Horizontal Cycle

Imagine each wave as a chapter in a captivating story. The period marks the distance between any two consecutive chapters, representing the time it takes for the story to complete one full cycle. This mesmerizing rhythm dictates the rate at which the cosecant function repeats its intricate dance.

Phase Shift: A Horizontal Journey

Envision the cosecant function embarking on a horizontal odyssey, shifting along the x-axis. This phase shift alters the starting point of the graph, creating a tantalizing misalignment between the expected and the observed. It’s as if the story begins at a different chapter, leaving us in a state of delightful suspense.

Vertical Shift: Ascending and Descending

Now, let’s elevate our perspective and consider the cosecant function’s ascent and descent along the y-axis. The vertical shift propels the graph vertically, like a majestic eagle soaring through the sky. It shifts the graph’s midline, the horizontal line that bisects the graph, indicating the function’s average altitude.

Midline: The Center of Gravity

The midline is the equilibrium of the cosecant function, the calm before the storm and the tranquility after the tempest. It represents the center line of the graph, around which the waves gracefully oscillate. This midline serves as a stable reference point, guiding our understanding of the function’s behavior.

Domain: Unveiling the Realm of Possible Inputs

The cosecant function, like a discerning traveler, has preferences for certain input values. Its domain is the realm of all admissible inputs, the values that the function gracefully accepts. For the cosecant function, all real numbers are welcome guests, with one notable exception: zero. Zero, the elusive point of division, remains off-limits, creating a captivating mystery within the domain.

The cosecant function, with its enchanting blend of amplitude, period, phase shift, and domain, paints an unforgettable canvas of mathematical beauty. By unraveling its secrets, we gain a deeper appreciation for the complexities and wonders that lie within the realm of trigonometric functions.

Range: Possible Outputs

• Define the range as the set of all possible output values.
• State that the range of the cosecant function is all real numbers greater than or equal to -1 and less than or equal to 1.

Unlock Your Understanding of the Cosecant Function: A Comprehensive Guide

Embark on a fascinating journey into the realm of trigonometric functions, where we’ll delve into the intricacies of the cosecant function. This versatile function, defined as the reciprocal of the sine function, plays a crucial role in modeling periodic phenomena and solving real-world problems. In this comprehensive guide, we’ll unravel the key characteristics of the cosecant function, empowering you with a thorough understanding of its behavior and applications.

Amplitude: The Vertical Scale

Picture the cosecant function as a symphony of sinusoidal curves. The amplitude of a cosecant curve, measured as the vertical distance between its maximum and minimum values, determines the height of its peaks and troughs. This parameter gauges the extent of the function’s oscillations, providing insights into the intensity or magnitude of the phenomenon under study.

Period: The Horizontal Cycle

Analogous to the rhythm of a musical beat, the period of a cosecant function represents the distance between any two consecutive peaks or troughs. It measures the time it takes for the function to complete one full cycle. Understanding the period is essential for anticipating the timing of events or predicting periodic patterns in data.

Phase Shift: Horizontal Movement

Imagine sliding a cosecant curve along the x-axis. This horizontal displacement, known as phase shift, alters the starting point of the graph. By shifting the function to the left or right, we effectively adjust the timing of its oscillations, aligning them with real-world events or specific conditions.

Vertical Shift: Moving Up or Down

Envision lifting or lowering a cosecant curve along the y-axis. This vertical shift adjusts the midline of the graph, which represents the average value of the function. By shifting the midline upward or downward, we can account for offsets or biases in the data being modeled.

Midline: The Center Line

The midline of a cosecant function serves as a reference point, dividing the graph into two equal halves. It indicates the average value of the function, providing a benchmark against which to compare its variations. The midline plays a pivotal role in determining the overall shape and behavior of the cosecant curve.

Domain: Possible Inputs

Consider the set of all possible input values for the cosecant function. This set, known as the domain, reveals the range of values over which the function is defined. For the cosecant function, the domain excludes zero as it corresponds to an undefined value. Understanding the domain ensures that we operate within the function’s valid range of inputs.

Range: Possible Outputs

Finally, let’s explore the set of all possible output values generated by the cosecant function. This set, known as the range, defines the limits within which the function oscillates. For the cosecant function, the range is bounded between -1 and 1, indicating that its output values never fall outside this interval. This characteristic is crucial for understanding the function’s behavior and its potential applications.