Unveil The Secrets Of Finding Parabola X-Intercepts: A Comprehensive Guide

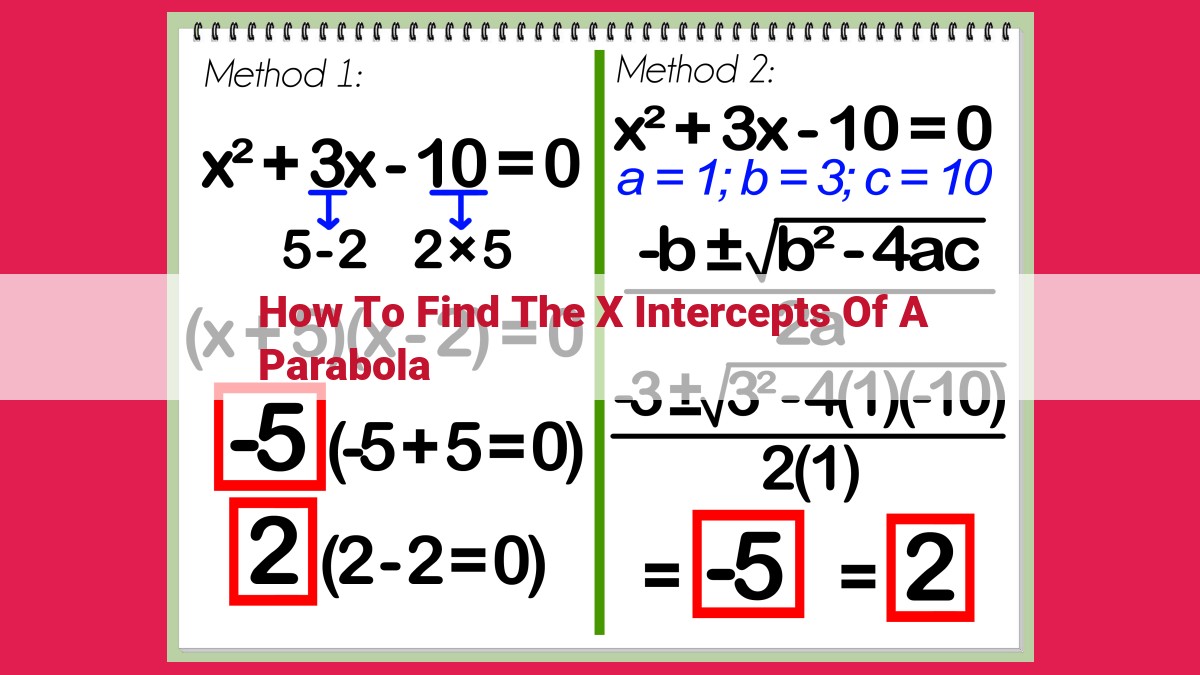
To find the x-intercepts of a parabola, set y = 0 in its standard form equation (y = ax² + bx + c), resulting in a quadratic expression. Factor it into linear factors, set each factor to zero using the zero product property, and solve for x. The resulting values are the x-intercepts, representing the points where the parabola crosses the x-axis and y is equal to 0.

In the realm of mathematics, parabolas stand as picturesque curves that dance gracefully across the coordinate plane. Their distinct U-shaped silhouette captivates the imagination, inviting us to delve into their enchanting world. Imagine a parabolic arch, gracefully curving overhead, or a comet streaking across the night sky, leaving behind a luminescent parabolic trail. Parabolas permeate our surroundings, from the gentle slopes of a rolling hill to the trajectory of a perfectly tossed ball. Their alluring shape and mathematical significance have intrigued scholars for centuries, making them an indispensable part of our understanding of the world.

As we embark on this adventure, we’ll unravel the secrets of parabolas, focusing on their intriguing x-intercepts. These points, where the parabola intersects the x-axis, hold profound insights into the curve’s characteristics and behavior. By understanding how to find and interpret x-intercepts, we’ll gain a deeper appreciation for the beauty and versatility of parabolas.

X-Intercepts: The Points Where Parabolas Meet the X-Axis

Picture this: you’ve got yourself a beautiful parabola, gracefully curving its way across the coordinate plane. Now, let’s take a closer look at those special points where this parabola intersects the x-axis. These points are called x-intercepts.

Think about it this way: when the parabola crosses the x-axis, it’s like it’s saying “Hey, I’m touching the ground right here!” And what’s special about the ground? Well, the ground is where y equals zero. So, at an x-intercept, the y value of the parabola is exactly zero.

These x-intercepts are not just random points; they hold important information. They tell us where the parabola crosses the horizontal line of the x-axis. These points represent the values of x for which the parabola has a height of zero.

Solving for the X-Intercepts: A Step-by-Step Guide to Unraveling the Roots of Parabolas

In the realm of mathematics, parabolas reign supreme, capturing our attention with their U-shaped curves. To delve deeper into the secrets of these enchanting curves, we must first understand their x-intercepts, the points where they gracefully intersect the x-axis.

The x-intercepts hold a special significance, representing the y = 0 values where the parabola kisses the horizontal line. To unveil these enigmatic points, we embark on a mathematical quest, guided by the standard form equation of a parabola:

y = ax² + bx + c

Our journey begins by setting y equal to zero in this equation, as if we are commanding the parabola to reveal its x-axis rendezvous points:

0 = ax² + bx + c

Solving for x in this equation is akin to unlocking a treasure chest. But fear not, dear reader, for we have a trusty companion – the zero product property. This mathematical rule whispers: if the product of two expressions is zero, then at least one of those expressions must be zero.

With this newfound insight, we carefully factor the quadratic expression (ax² + bx + c) into two linear factors. It’s like taking apart a complex puzzle to reveal its simpler pieces. This step is crucial, as it allows us to equate each linear factor to zero and solve for x.

Different factors lead to different x-intercept values, each representing a distinct point where the parabola meets the x-axis. It’s as if we are uncovering the hidden gems scattered along the parabola’s curve.

If factoring proves to be a daunting task, we can turn to the quadratic formula, a mathematical lifeline that can be used to find x-intercepts without factoring. Simply plug the values of a, b, and c into the formula, and it will effortlessly yield the x-intercept values.

Now, armed with the power to solve for x-intercepts, we can confidently analyze any parabola, unlocking its secrets and appreciating its mathematical beauty. So, let us continue our mathematical exploration, unraveling the mysteries of parabolas one x-intercept at a time.

Factoring the Quadratic Expression

The quadratic expression (ax² + bx + c) that defines a parabola can be factored into two linear factors. This process allows us to break down the parabola’s equation into simpler terms that make finding its x-intercepts easier.

Imagine the quadratic expression as a rectangle. Its area is represented by (ax² + bx + c), and the two dimensions of the rectangle are linear factors. For instance, (x + 3)(x - 2) is a factored form of the quadratic expression (x² + x - 6). The dimensions of this rectangle are (x + 3) and (x - 2).

Visual Representation

The factoring process visually resembles finding two numbers that multiply to c and add to b. For the expression (x² + x - 6), these numbers are 3 and -2, as seen in the factored form (x + 3)(x - 2). The factors (x + 3) and (x - 2) represent the two dimensions of the rectangle whose area is equal to the quadratic expression.

Zero Product Property: Unraveling the X-Intercepts of Parabolas

In our journey to comprehend the captivating world of parabolas, we arrive at a pivotal concept known as the Zero Product Property. This property serves as a guiding principle, illuminating the path to uncovering the enigmatic x-intercepts that define these parabolic curves.

The Zero Product Property asserts that when the product of two factors equates to zero, it inevitably implies that one of those factors must also be zero. This fundamental principle plays a crucial role in our quest to determine the x-intercepts of parabolas.

To untangle the intricacies of this concept, let us delve into the standard form equation of a parabola: y = ax² + bx + c. Our primary objective lies in identifying the x-values where the parabola intersects the x-axis, which corresponds to the points where y = 0.

Through a series of meticulous steps, we transform our parabolic equation into a more tractable form. By substituting y = 0, we effectively isolate the quadratic expression ax² + bx + c on one side of the equation.

Now, we invoke the power of factoring, a technique that enables us to decompose the quadratic expression into the product of two linear factors. This process unveils the underlying structure of the parabola and provides a clearer understanding of its behavior.

Armed with our factored linear factors, we apply the Zero Product Property with surgical precision. It dictates that if the product of these factors vanishes, it necessarily follows that one of the factors must be zero.

This crucial realization allows us to isolate each linear factor and set it independently equal to zero. By solving for x in each case, we uncover the x-intercepts that define the parabola. Each unique linear factor yields a distinct x-intercept value, providing valuable insights into the shape and orientation of the curve.

In essence, the Zero Product Property serves as a bridge between the factored form of the quadratic expression and the x-intercepts of the parabola. It underscores the fundamental principle that when a product equates to zero, one of its constituents must inevitably share the same fate, guiding us towards a comprehensive understanding of parabolic behavior.

Equating Factors to Zero: Unveiling X-Intercept Values

Once we have successfully factored the quadratic expression into two linear factors, we embark on the final step of our journey to determine the x-intercepts. In this crucial stage, we harness the power of the zero product property. This principle dictates that if the product of two factors is zero, at least one of those factors must also be zero.

Armed with this knowledge, we turn our attention to our factored linear factors. We set each factor meticulously equal to zero. By solving each linear equation for x, we uncover the hidden treasures – the x-intercept values.

Example:

Consider the quadratic expression ax² + bx + c. Upon factoring, we obtain:

(ax + d)(ex + f) = 0

To find the x-intercepts, we set each factor equal to zero:

ax + d = 0
ex + f = 0

Solving each equation, we find:

x = -d/a
x = -f/e

These two distinct values of x represent the x-coordinates of the parabola’s intercepts with the x-axis.

It’s important to note that different factors will lead to different x-intercept values. For instance, a parabola with factors (x - 3) and (x + 2) will have x-intercepts at x = 3 and x = -2, respectively.

In conclusion, the process of equating factors to zero is a critical step in determining the x-intercepts of a parabola. By harnessing the zero product property and meticulously solving linear equations, we uncover the points where the parabola gracefully touches the x-axis.

Finding X-Intercepts: The Alternative Method Using the Quadratic Formula

In our quest to unravel the secrets of parabolas, we encounter a scenario where the trusty method of factoring falls short. Fear not, dear reader, for we have another powerful tool at our disposal – the quadratic formula.

The quadratic formula is a mathematical equation that can solve any quadratic equation, regardless of its complexity. It may seem daunting at first, but with a little guidance, we’ll navigate it together.

The quadratic formula is written as:

x = (-b ± √(b² - 4ac)) / 2a

Here, a, b, and c represent the coefficients of the quadratic equation in the standard form y = ax² + bx + c.

To find the x-intercepts using the quadratic formula, we simply substitute the values of a, b, and c into the formula and solve for x. The x-intercepts are the points where the parabola crosses the x-axis, and they occur when the y-value is zero.

For example, consider the parabola y = x² – 4x + 3. To find its x-intercepts, we plug a = 1, b = -4, and c = 3 into the quadratic formula:

x = (-(-4) ± √((-4)² - 4(1)(3))) / 2(1)
x = (4 ± √(16 - 12)) / 2
x = (4 ± 2) / 2

Solving for x, we find two x-intercepts: x = 1 and x = 3. This means that the parabola crosses the x-axis at the points (1, 0) and (3, 0).

So, while factoring may be our preferred method, the quadratic formula provides a reliable backup option when factoring is not possible. With this tool in our arsenal, we can conquer any quadratic equation that comes our way.