Number Models: Essential Tools For Math Literacy And Problem-Solving

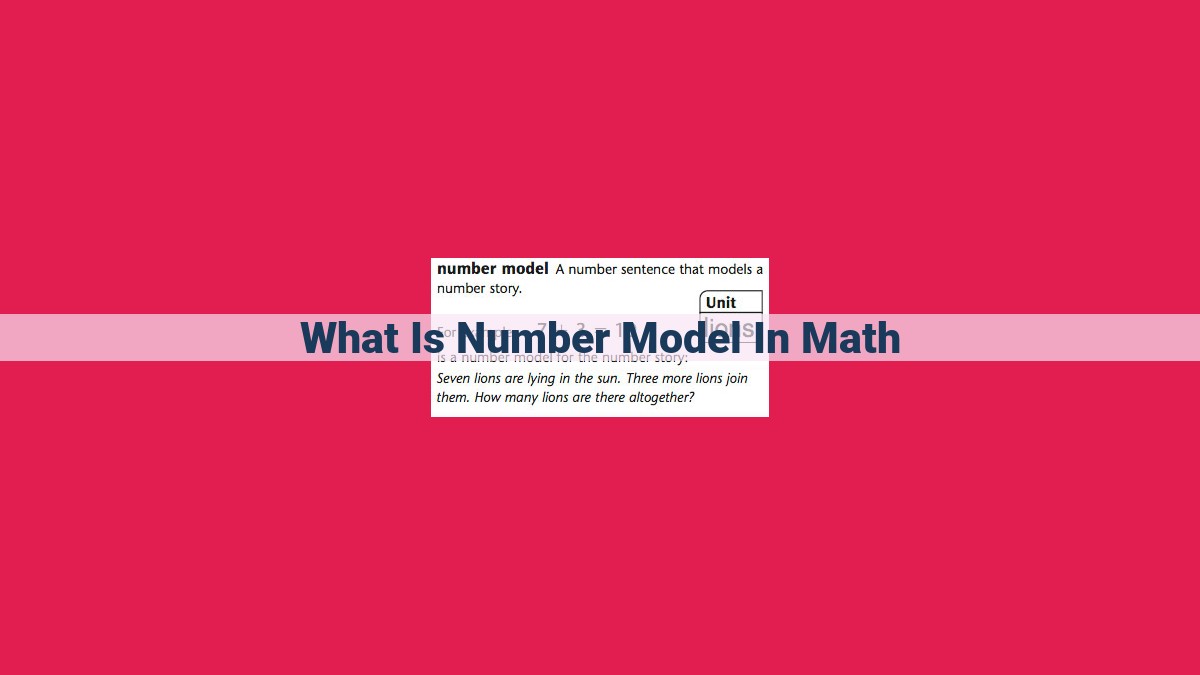
A number model is a conceptual representation of a number that helps us understand its relationships and operations. It includes place value, which shows the position of digits and their impact on number value, and various forms like standard form, expanded form, and word form. Number models also include the number line, which visually represents numbers and their relationships, number patterns that explore regularities, and number bonds that show sums and differences. These models are crucial in math education, fostering number sense, comprehension of number relationships, and problem-solving abilities.

• Define number models as conceptual representations of numbers
• Highlight their significance in comprehending number relationships and operations

Understanding Number Models: The Essential Foundation for Math Literacy

In the realm of mathematics, numbers are more than just digits; they are ideas, concepts, and tools that shape our understanding of the world around us. To grasp the true essence of numbers, it is imperative to comprehend number models, which are conceptual representations that bring numbers to life.

Number models empower us to visualize numbers, see their relationships, and perform operations with ease. They act as bridges connecting the abstract world of numbers to the tangible experiences we can relate to. By unraveling these models, we unlock the secrets of number sense, the foundation upon which all mathematical knowledge is built.

From place value to the ubiquitous number line, number models take various forms, each with its unique purpose. They help us understand how numbers are structured, how they interact with each other, and how they can be used to solve real-world problems. By embracing number models, we cultivate a deep and intuitive understanding of the language of mathematics, enabling us to navigate the world of numbers with confidence and proficiency.

Place Value: The Brickwork of Number Models

In the world of numbers, place value stands as a pivotal concept, the foundation upon which number models are built. It’s like the blueprint of a house, dictating the position of each digit and its impact on the value of the number.

Think of the number 234. The digit 2 holds the hundreds place, representing 200. The digit 3 settles in the tens place, worth 30. And the digit 4 occupies the ones place, contributing 4.

Base 10: The Common Thread

The base 10 number system, the cornerstone of our number system, plays a starring role in place value. Each place represents a multiple of 10. The hundreds place is 100, the tens place is 10, and the ones place is 1. This base 10 system allows us to easily compare and manipulate numbers.

Standard Form: The Number’s Suit

Standard form is the way we conventionally write numbers based on place value. For example, we write the number above as 234. Standard form makes calculations and number comparisons a breeze.

Expanded Form: The Number’s Ingredients

Expanded form, on the other hand, expresses a number as a sum of its individual place values. So, 234 becomes 200 + 30 + 4. Expanded form helps us understand the composition of a number and allows us to break it down into its component parts.

Word Form: The Number’s Narrative

Word form represents numbers using words. For example, we would write 234 as two hundred thirty-four. Word form is essential for developing number sense and improving our ability to communicate about numbers.

By mastering place value, we lay the groundwork for a strong understanding of number models and all the mathematical adventures that lie ahead!

Standard Form: The Concise Representation of Numbers

In the realm of mathematics, numbers dance in a symphony of representation. Among the various forms, standard form stands tall as the conventional way of expressing numbers. It’s the arrangement of digits that we all know and love, with each digit occupying its designated place in the number family.

Defining Standard Form

Standard form, simply put, is a system where each digit holds a specific value based on its position. Starting from the right, the first digit represents the ones, then tens, hundreds, and so on. This structure empowers us to easily understand the magnitude and relationship between numbers.

The Power of Simplicity

Standard form’s simplicity shines through in both calculations and number comparisons. When adding or subtracting numbers, the values of individual digits line up perfectly, making it a breeze to perform operations. Similarly, when comparing numbers, the standard form allows us to quickly discern which number is greater or smaller.

Example:

Let’s imagine two numbers, 256 and 347. In standard form, we can instantly recognize that 347 is greater than 256 because the digit in the hundreds place (3) is larger than the corresponding digit in 256. This same principle applies to all arithmetic operations, making standard form an indispensable tool for mathematical calculations.

Expanded Form: Breaking Down Number Structure

• Explain expanded form as the expression of a number as a sum of individual place values
• Discuss its use in understanding number composition and decomposition

Expanded Form: Unveiling the Inner Workings of Numbers

When faced with a number, it’s easy to see it as a single entity. But what if we could peel back the layers and reveal its inner structure? That’s where expanded form comes in.

Expanded form is a mathematical tool that allows us to break down a number into its individual place values. For example, the number 456 in expanded form becomes 400 + 50 + 6. Each digit is multiplied by its respective place value: 4 × 100, 5 × 10, and 6 × 1.

By breaking down numbers this way, we can better understand their composition and decomposition. We can easily identify the value of each digit based on its position. For instance, in the expanded form of 456, we know that 4 represents 400, highlighting the significance of its place in the hundreds column.

Unlocking Hidden Patterns:

Expanded form goes beyond mere representation. It opens up a world of pattern recognition. By analyzing the place values of different digits, we can discern relationships and predict how numbers will behave in operations.

For example, consider the numbers 105 and 108. In expanded form, we see:

• 105 = 100 + 0 + 5
• 108 = 100 + 0 + 8

Notice that both numbers have the same hundreds place value (100) and the same tens place value (0). Only the ones place value differs, indicating that the difference between the two numbers lies solely in their ones digit. This understanding aids in quick mental calculations and number comparisons.

A Bridge to Deeper Number Sense:

Expanded form serves as a crucial bridge in developing a strong number sense. It helps students visualize numbers, comprehend their structure, and manipulate them with confidence.

By breaking down numbers into their component parts, expanded form demystifies mathematical concepts, making them more accessible and meaningful. It fosters a deeper understanding of number relationships, paving the way for advanced mathematical thinking.

Word Form: Expressing Numbers through Language

Words have the power to convey complex ideas, and numbers are no exception. Word form refers to the representation of numbers using words, a method that plays a crucial role in our understanding and communication of mathematical concepts.

Defining Word Form

Word form, as the name suggests, involves writing numbers as words. For instance, the number 123 in word form would be “one hundred twenty-three.” This format offers a more intuitive and familiar way of representing numbers, particularly for large or complex values.

Importance for Number Sense

Word form fosters the development of number sense, the ability to understand and manipulate numbers flexibly. By associating words with numbers, we can better grasp their magnitude, relationships, and patterns. It allows us to make sense of numbers in real-life contexts, such as counting objects, measuring quantities, and solving word problems.

Enhancing Communication

Numbers convey information, but words can add depth and clarity. Word form facilitates communication, especially when discussing numbers in verbal settings or written documents. It helps us avoid confusion and misinterpretation by providing a common language for representing numerical values.

In summary, word form is not merely a different way of writing numbers but a vital tool for enhancing our mathematical literacy. It builds number sense, improves communication, and empowers us to engage with numbers in a more meaningful way.

Number Lines: Visualizing the World of Numbers

Numbers, the building blocks of mathematics, can be abstract and challenging for young learners. Number lines provide a tangible and visual representation of numbers, making them more relatable and accessible. It’s like a number playground where numbers can dance and interact!

Imagine a long, straight path with numbers marked at regular intervals. This is our number line. It’s a map that helps us explore the world of numbers. By visualizing numbers on the line, we can understand their relationships and operations in a much more intuitive way.

Number sequences, like a marching band, move along the number line in a predictable pattern. We can see the gaps between numbers, helping us to predict the next number in the sequence. It’s like a number dance that we can follow step by step.

Mathematical operations, such as addition and subtraction, become less daunting on a number line. Instead of manipulating abstract digits, we can watch numbers move and adjust on the line, making calculations feel more tangible and less intimidating.

Comparisons between numbers are also a breeze on the number line. The distance between numbers tells us which one is greater or smaller. It’s like a race where the number that’s farther along the line takes the lead!

Number lines are not just mathematical tools; they are magical wands that transform abstract numbers into a visual wonderland. By making numbers more relatable and engaging, number lines empower young learners to embrace the world of mathematics with confidence and understanding. They are a key to unlocking the door to mathematical mastery.

Number Patterns: Unraveling the Mathematical Symphony

In the tapestry of numbers, patterns dance like ballerinas, weaving together an intricate symphony of mathematical relationships. These enigmatic sequences, known as number patterns, are like hidden codes, inviting us to decipher their underlying rules.

The Magic of Number Prediction:

Embarking on the journey of number patterns is akin to becoming a mathematical detective. By observing the pattern, we become adept at predicting the next number in the sequence. It’s like a game of puzzles, where each solution unravels a deeper understanding of the pattern’s hidden logic.

Unveiling Relationships:

Number patterns reveal the intricate connections between numbers. They illuminate how numbers relate to one another, forming mathematical threads that bind them together. By uncovering these relationships, we gain a deeper insight into the structure and dynamics of the number system.

Problem-Solving Mastery:

In the realm of problem-solving, number patterns are invaluable tools. They empower us to approach complex numerical challenges with finesse. By recognizing patterns in word problems, we can devise strategies that lead us to elegant solutions, transforming us into mathematical wizards.

Types of Number Patterns:

The mathematical landscape is adorned with diverse number patterns, each with its unique characteristics. Some of the most common include:

• Arithmetic patterns (addition or subtraction of a constant)
• Geometric patterns (multiplication or division of a constant)
• Fibonacci sequence (addition of two preceding terms)

Benefits of Exploring Number Patterns:

Delving into the realm of number patterns bestows upon us a myriad of benefits. It sharpens our cognitive skills, fostering logical reasoning and critical thinking. Additionally, it enhances our mathematical fluency, enabling us to navigate numerical challenges with ease.

Number patterns are the hidden treasures within the mathematical universe. They invite us to embark on a journey of discovery, leading us to a deeper understanding of numbers and their captivating relationships. Embracing the adventure of number patterns unlocks the door to a world of mathematical enlightenment.

Number Bonds: Building Number Sense through Number Relationships

Numbers are more than just symbols; they represent ideas and relationships that shape our understanding of the world. Number bonds are a crucial tool for building number sense, as they provide a tangible representation of the connections between numbers.

What are Number Bonds?

Number bonds are diagrams that show the relationships between numbers as sums and differences. They typically consist of a circle representing the whole number, with smaller circles attached representing the parts. For example, the number bond 5 + 2 = 7 would be shown as a circle with 5 smaller circles inside and 2 smaller circles attached outside.

Using Number Bonds to Develop Number Sense

Number bonds help children develop number sense in several ways:

• Visualizing Number Relationships: Number bonds provide a visual representation of the relationships between numbers, making it easier for children to understand how numbers can be broken down and recombined.
• Exploring Number Combinations: Number bonds help children explore different combinations of numbers that add up to a given total. This helps them develop flexibility in their thinking and supports the development of mental math skills.
• Reinforcing Addition and Subtraction Facts: Number bonds reinforce the relationship between addition and subtraction, as the same diagram can be used to represent both the sum and the difference of two numbers.

Number Bonds in Addition and Subtraction Strategies

Number bonds can also be used to support addition and subtraction strategies:

• Counting On and Counting Back: Number bonds help children visualize the process of counting on (adding) and counting back (subtracting). They can see how adding or removing a certain number of objects changes the total.
• Number Bond Break Apart: The break apart strategy involves breaking a number into two smaller numbers and then adding or subtracting them. Number bonds can help children visualize this process and make it easier to understand.
• Compensation: Compensation is a strategy where children adjust one number slightly to make the calculation easier. Number bonds can help children understand how to make these adjustments and find equivalent number sentences.

Number bonds are an essential tool for building number sense and supporting addition and subtraction strategies. By providing a visual representation of number relationships, they help children develop a deeper understanding of numbers and their properties. Incorporating number bonds into math instruction can significantly enhance children’s mathematical learning and empower them to become confident and competent mathematicians.