Measuring Curve Radius: Understanding Central Angle, Arc Length, And Intercepted Chord

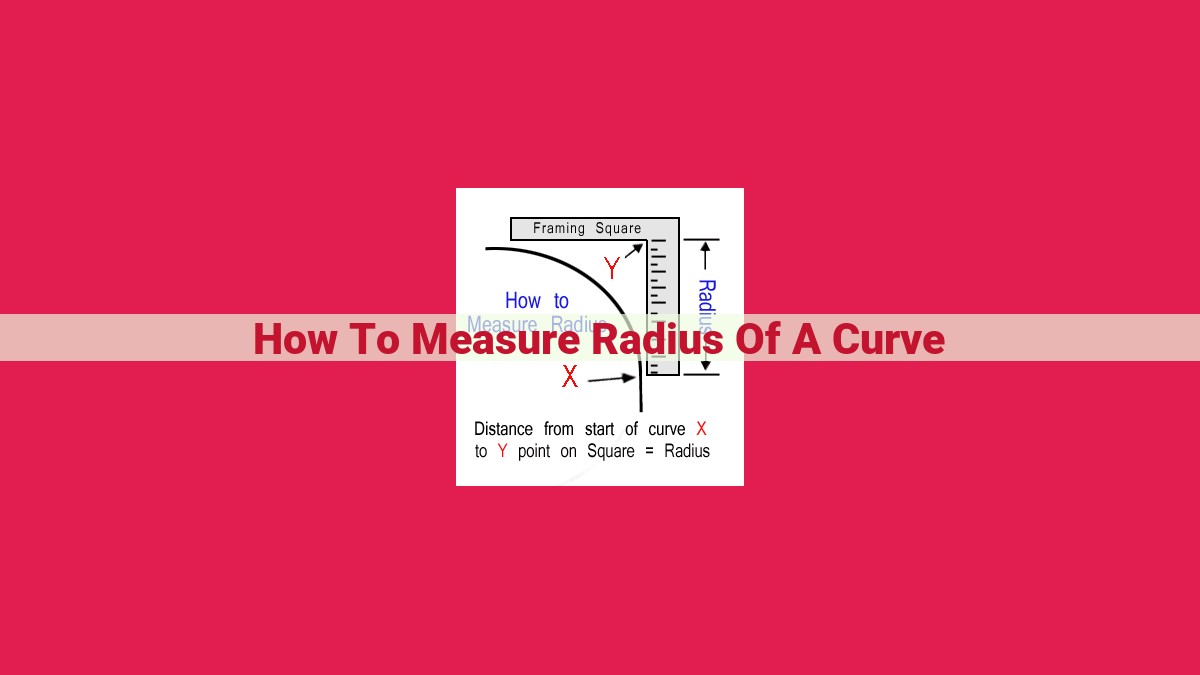
To measure the radius of a curve, it is important to understand its central angle, arc length, and intercepted chord. The radius represents the distance from the center of the curve to its perimeter, where the central angle measures the angle at the center of the circle that intercepts the curve. The arc length refers to the distance along the curve’s perimeter, while the intercepted chord is the straight line connecting two points on the curve. By considering these elements and their interrelationships, one can accurately determine the radius and curvature of the curve.

The Secrets of Curves: Understanding Radius of Curvature and Beyond

Get ready for an exciting exploration into the fascinating world of curves! This journey will unravel the mysteries of radius of curvature, a crucial concept in geometry that unlocks the secrets of curves.

Radius of Curvature: The Key to the Center

Picture a beautiful curve, like the arc of a rainbow or the slope of a hill. Imagine a radius of curvature as the distance from any point on this curve to a center of curvature. This center is like the heart of the curve, the point from which every other point is equidistant.

The radius of curvature tells us how tight or gentle a curve is. A smaller radius indicates a tighter curve, while a larger radius represents a more gradual bend.

Tangent and Normal: Touching and Crossing

As we dive deeper into curves, we encounter two other important lines: tangents and normals. A tangent is like a friendly neighbor, gently touching the curve at just one point. A normal, on the other hand, is a bit more standoffish, crossing the curve at the same point but perpendicularly, like a line that has had enough.

These lines are like detectives, helping us decipher the secrets of curves. The direction of the curve at a particular point is determined by the tangent line, while the normal line provides information about the curvature of the curve at that point.

So, there you have it! This is just a glimpse into the captivating realm of curves. We’ve explored the radius of curvature, along with its trusty companions, tangents and normals. Stay tuned for more adventures in this exciting world!

Central Angle: Measuring the Arc’s Embrace

In the realm of geometry, the central angle holds a pivotal role in defining the arc of a circle or curve. Imagine a circle, like a celestial sphere. Its radii, emanating from the center like spokes of a wheel, form a network that encompasses the circle. Now, if you connect two radii, you create what we call a central angle. This angle is measured at the circle’s center.

The central angle plays a crucial role in determining the arc length. The arc length, as its name suggests, measures the distance along the curve of the circle or curve. The relationship between the central angle and arc length is proportional. The larger the central angle, the longer the corresponding arc length. Think of it as stepping around the circumference of a circle: the more steps you take (central angle), the greater the distance you cover (arc length).

Furthermore, the central angle is intimately connected to the sector. A sector, like a slice of pie, is the region bounded by the radii and the corresponding arc. The area of a sector is also directly proportional to the measure of its central angle. So, the larger the central angle, the larger the sector area. This principle finds practical applications in various fields, such as architecture and engineering, where precise measurements of arc lengths and sector areas are essential.

Understanding the central angle is pivotal in deciphering the intricacies of circles and curves. It empowers us to accurately determine arc lengths and sector areas, unlocking a deeper comprehension of geometric shapes.

Arc Length: Measuring the Curve

• Define arc length and explain how it can be calculated.
• Discuss the difference between arc length and chord length.

Arc Length: Measuring the Curve

Let’s venture into the captivating world of geometry and unravel the mysteries of arc length. Imagine yourself standing before a beautiful arch or a winding road. How would you measure the exact distance along its graceful curve?

Defining Arc Length

An arc length is simply the distance along a portion of a curve, measured from one end to the other. It differs from the chord length, which is the straight-line distance between the endpoints of the curve.

Calculating Arc Length

For small arcs, you can approximate the arc length by using the chord length. However, for more precise measurements, we need to delve into the wonders of calculus. The integral is a powerful mathematical tool that allows us to compute the exact arc length for any continuous curve.

The Integral and Arc Length

The integral formula for arc length is:

Arc Length = ∫√(dx²/dt² + dy²/dt²) dt

where:

• dx/dt and dy/dt are the first derivatives of the curve’s parametric equations
• dt is the differential of the parameter

Example: Circular Arc

Let’s consider a circular arc of radius r and central angle θ. The parametric equations for this arc are:

x = r cos(θ)
y = r sin(θ)

The integral for the arc length becomes:

Arc Length = ∫√(r² sin²(θ) + r² cos²(θ)) dθ = r * θ

Relevance to Real-World Applications

Arc length finds numerous applications in various fields:

• Architecture: Calculating the length of archways, balconies, and other curved structures
• Transportation: Determining the distance traveled along winding roads or railway tracks
• Engineering: Estimating the length of cables, pipes, and other curved components
• Computer Graphics: Creating realistic curved shapes for animations and virtual environments

Understanding arc length is essential for accurately measuring the distance along curves. From measuring roads to designing structures, the concept of arc length plays a vital role in shaping our world. So the next time you encounter a curve, remember the magical integral that allows us to unveil its intricate geometry.

Chord: A Line Connecting Points on a Curve

In the realm of geometry, curves gracefully twist and turn, captivating our senses with their intriguing shapes. Amidst this dynamic landscape, the chord emerges as an essential element, stretching between two points on a curve, like a taut string connecting two vibrant notes.

Beyond its fundamental definition, the chord plays a vital role in deciphering the curvature of a curve. Imagine a finely crafted bow, poised to glide across the taut strings of a violin. Its curvature is subtly hinted at by the shape of the chord, a telltale sign of the curve’s underlying geometry.

The shorter the chord relative to its corresponding arc length, the tighter the curvature of the curve. Conversely, longer chords imply gentler curves, akin to the graceful arc of a delicate arch. By analyzing the length of chords and their relationship to the curve they touch, we gain a deeper understanding of the intricate dance of shapes.

Sector: A Slice of the Circle or Curve

Imagine a slice of a pie, a triangular portion of dough that tantalizes your taste buds. In the realm of geometry, we encounter a similar concept called a sector, a region bounded by a central angle and its corresponding arc.

A central angle is formed when two radii (lines connecting the center to points on a circle or curve) intersect within the circle or curve. The corresponding arc is the portion of the circumference (for circles) or curve that lies between the endpoints of the radii. Together, the central angle and arc define a sector.

The most captivating aspect of a sector is its area, which is directly proportional to the measure of its central angle. Picture a birthday cake with a dozen candles, each representing a sector with an equal central angle. The larger the central angle, the larger the sector’s slice of the cake. This relationship is mathematically expressed as:

Sector Area = (Central Angle Measure / 360°) × πr²

Where r is the radius of the circle or curve.

In other words, the sector area is a fraction of the entire circle’s area, determined by the fraction of the 360° central angle it occupies. For example, a sector with a central angle of 60° would have an area of one-sixth of the entire circle’s area.

So, there you have it, the sector, a region of a circle or curve bounded by a central angle and its arc, with an area that dances in harmony with the measure of its central angle.

Intercept: Where Chord and Tangent Meet

• Define intercept as the point where a chord intersects a tangent line.
• Explain its significance in determining the curvature of a curve.

The Intercept: A Critical Intersection in Curvature

In the realm of geometry, curves and their properties play a fascinating role. One such element is the intercept, a crucial intersection point that sheds light on the curvature of a curve.

Definition and Significance

The intercept is the point where a chord, a line segment connecting two points on a curve, meets a tangent line, a line that touches the curve at a single point perpendicularly. The intercept’s significance lies in its ability to determine the curvature of the curve at that specific point.

Determining Curvature

The curvature of a curve describes how sharply it bends. A curve with high curvature has a relatively small radius of curvature, indicating that it turns quickly. Conversely, a curve with low curvature has a larger radius of curvature, indicating a more gradual turn.

The intercept provides valuable information about the curvature. The angle between the intercept and the tangent line is known as the angle of intersection. Curves with a smaller angle of intersection have a sharper curvature, while curves with a larger angle of intersection have a flatter curvature.

Visualizing the Intercept

Imagine a curve resembling a roller coaster track. The points where the track changes direction most abruptly have smaller angles of intersection and, therefore, sharper curvature. At these points, the intercept will be closer to the curve, indicating a more pronounced change in direction.

Conversely, on sections of the track where the curve is more gradual, the angle of intersection will be larger, and the intercept will be farther away from the curve. This represents a less pronounced change in direction and, hence, a flatter curvature.

Significance in Applications

The intercept has practical applications in various fields, including engineering, architecture, and computer graphics. For instance, in designing roads or bridges, engineers consider the curvature of the curve to ensure smooth transitions and minimize wear and tear.

In architecture, the intercept is used to determine the shape of arches and domes, ensuring structural integrity and visual aesthetics. Computer graphics rely on intercepts to create realistic curves and surfaces in 3D models.

Understanding the Intercept

By grasping the concept of the intercept and its role in determining curvature, we gain a deeper understanding of the complex nature of curves. It’s a tool that unlocks valuable insights into the shape and behavior of curves, empowering us to create and interpret geometric structures with precision.

The Tangent Line: Unveiling the Curve’s Direction

In the realm of geometry, curves dance across the plane, their intricate shapes revealing hidden insights. Among the essential elements that shape these curves, the tangent line stands out as a beacon of direction. It’s a line that touches the curve at a single point, perpendicularly.

Imagine a curve as a winding road, with its twists and turns reflecting the changing direction of the road. The tangent line acts like a signpost, pointing us towards the immediate direction of the curve at a specific point.

Discovering the Tangent’s Importance

The tangent line holds profound significance for understanding curves. It provides a window into the slope of the curve at a particular point, which is crucial for describing how the curve rises or falls. By analyzing the slope of the tangent line, we can determine whether the curve is increasing, decreasing, or remaining constant.

A Compass for Curve Analysis

Furthermore, the tangent line serves as a compass, guiding us through the curvature of the curve. Its angle with the radius of curvature (the line from the curve’s center to the point of tangency) measures the curvature of the curve at that point. The tighter the radius of curvature, the sharper the curve, and the larger the angle between the tangent line and the radial line.

The tangent line is an indispensable tool for unraveling the mysteries of curves. By comprehending its role in determining the direction and curvature of a curve, we gain a deeper understanding of the fascinating world of geometry. So, the next time you encounter a curve, remember the tangent line as your trusted guide, leading you through its hidden depths.

Normal Line: Perpendicular to the Tangent

• Define normal line as a line perpendicular to the tangent line at a specific point.
• Explain its relationship to the radius of curvature and the direction of the curve.

Normal Line: Perpendicular to the Tangent

Imagine you’re walking along a winding road. As you turn, you can sense the direction of the road changing beneath your feet. This direction is determined by the tangent line at each point. But there’s another line that plays a crucial role in understanding the curve of the road—the normal line.

The normal line is like a guardian angel, always standing perpendicular to the tangent line at any given point. This special relationship means that the normal line is always pointing directly towards the center of curvature, the point around which the curve is bending.

But why is the center of curvature so important? Because it determines the radius of curvature, which measures the tightness of the curve. The smaller the radius of curvature, the sharper the turn.

Now, let’s visualize an arching bridge. As you drive along it, the tangent line at any point will indicate the direction of your car. However, the normal line will be pointing towards the center of the bridge, which is the point around which the bridge curves. By aligning your car with the normal line, you can navigate the curve smoothly and safely.

In summary, the normal line is a geometric guardian that provides crucial information about the direction and curvature of a path. It helps us navigate the complexities of curves and understand the underlying geometry that shapes our world.