Understanding Mathematical Properties For Accurate Calculations And Problem-Solving

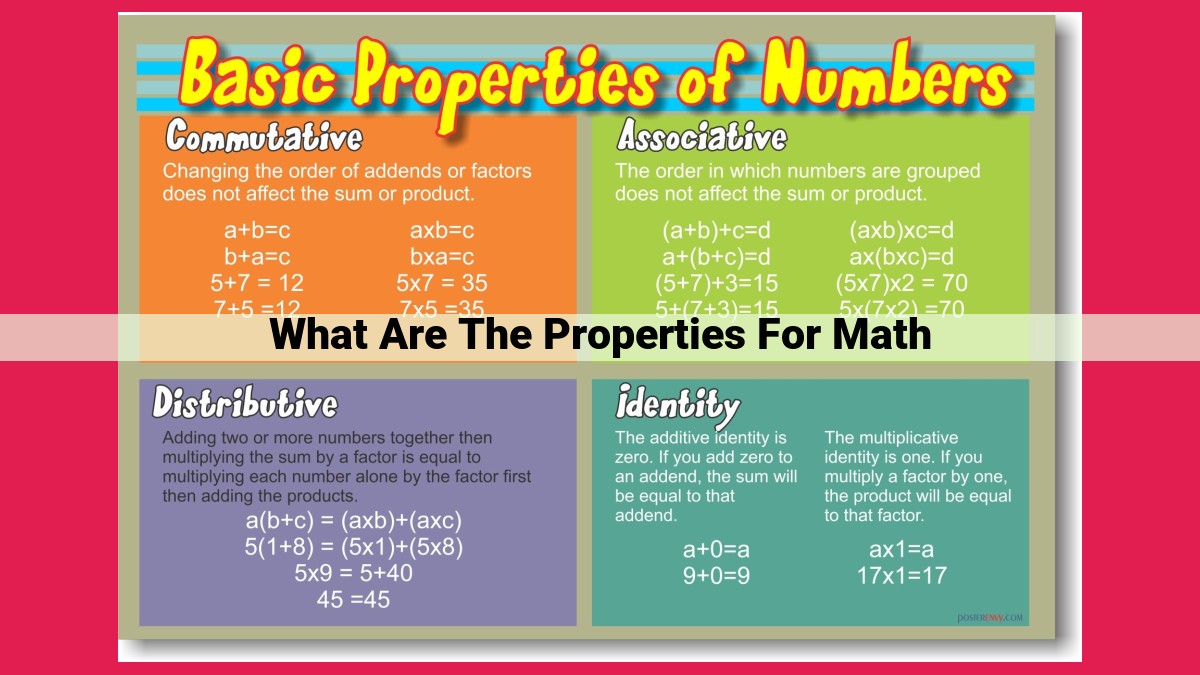
Mathematical properties are fundamental rules that govern mathematical operations, simplifying calculations and ensuring consistent results. Key properties include the associative, commutative, and distributive properties, which relate to the grouping and order of operations. The identity and inverse properties provide multiplicative and additive identities, respectively, while the transitive property establishes relationships between equations and inequalities. These properties have practical applications in real-world scenarios, enabling efficient problem-solving and accurate calculations.

• Definition of mathematical properties and their importance in mathematical operations.

In the captivating world of mathematics, mathematical properties serve as the foundational principles that govern the operations we perform. Like the rules of a game, these properties provide clarity and structure, enabling us to navigate the complexities of numbers and equations with confidence. They empower us to simplify calculations, solve problems, and unravel mathematical mysteries.

Mathematical properties are inherent characteristics of numbers and operations, guiding their behavior in predictable ways. They ensure consistent results regardless of the order or grouping of elements, making them indispensable tools for efficient and accurate mathematical endeavors. Understanding these properties not only enhances our computational abilities but also deepens our appreciation for the elegance and interconnectedness of mathematics.

Key Mathematical Properties: Unlocking the Secrets of Mathematical Operations

In the realm of mathematics, properties are the guiding principles that govern how operations behave. These properties provide a solid foundation for understanding how mathematical expressions work, enabling us to solve problems efficiently and accurately.

Associative Property: Where Order Doesn’t Matter

Imagine you’re adding three numbers: 5, 7, and 9. You can group them in any order you like – (5 + 7) + 9 or 5 + (7 + 9) – and you’ll always get the same result, 21. This is because of the associative property, which states that when performing the same operation on a series of numbers, the order in which you group them doesn’t affect the outcome.

Commutative Property: Swapping Partners, Same Result

In the addition example above, you can also swap the order of the numbers without changing the result. This is the commutative property, which applies to both addition and multiplication. It means that, for instance, 5 + 7 is the same as 7 + 5, and 3 × 4 is the same as 4 × 3. This property simplifies calculations and makes it easier to find mental shortcuts.

Distributive Property: Multiplying is Easier Than You Think

Often, you may encounter expressions like 3 × (4 + 5). The distributive property allows you to break this down into simpler operations: 3 × 4 + 3 × 5. This property is especially useful when you need to factorize expressions or perform calculations mentally. It’s a powerful tool that makes mathematical operations more manageable.

Identity Property: Zero and One, the Special Numbers

In mathematics, there are two special numbers: zero and one. Zero, when added to any number, doesn’t change its value. This is the zero property of addition. Similarly, multiplying any number by one gives you the same number back. This is the one property of multiplication. These properties serve as the foundation for many other mathematical operations.

Inverse Property: Undoing Operations

In the world of mathematics, every operation has an inverse, an operation that undoes the original one. For example, the inverse of addition is subtraction, and the inverse of multiplication is division. The inverse property ensures that every mathematical operation has a companion that can reverse its effect.

Transitive Property: Connecting the Dots

Imagine you have three points on a number line: A, B, and C. If A is less than B, and B is less than C, then the transitive property tells us that A must be less than C. This property extends to other mathematical operations as well, such as equality and inequality. It allows us to chain together relationships and draw logical conclusions.

Mathematical Properties: Foundations of Mathematical Operations

Introduction:
Mathematical properties are fundamental principles that govern mathematical operations. They dictate how numbers and variables interact and simplify calculations. Understanding these properties is crucial for developing problem-solving skills and grasping the intricacies of mathematics.

Key Mathematical Properties:

• Associative Property:
  • This property allows you to change the grouping of numbers in an expression without altering its value. For instance, (a + b) + c = a + (b + c).
  • Commutative Property:
    • Related to the Associative Property, this property states that you can switch the order of numbers in an expression without changing its value. Example: a + b = b + a.
• Distributive Property:
  • This powerful property connects the operations of addition and multiplication. It states that a(b + c) = ab + ac, allowing for efficient factorization and simplification of expressions.
• Identity Property:
  • Every number has an identity element that, when combined, does not change its value. For addition, the identity element is 0, and for multiplication, it is 1.
• Inverse Property:
  • This property pairs numbers that, when combined, result in the identity element. For example, the inverse of 5 for addition is -5, and the inverse of 4 for multiplication is 1/4.
• Transitive Property:
  • This property plays a pivotal role in understanding equality. It states that if a = b and b = c, then a = c. It establishes a chain of equality that simplifies comparisons.

Applications of Mathematical Properties in Real-World Scenarios:

• Grocery Shopping:
  • Associative Property: You can group your grocery items in any order while calculating the total cost without affecting the result.
• Construction:
  • Distributive Property: When calculating the area of a rectangular room, you can break it down into separate lengths and widths and apply the formula efficiently.
• Recipe Scaling:
  • Identity Property: Doubling a recipe means using twice the amount of each ingredient, preserving the proportions.
• Investment Planning:
  • Inverse Property: To find the original investment amount from a given return, simply divide the return by the interest rate.
• Sports Betting:
  • Transitive Property: If Team A beats Team B, and Team B beats Team C, you can deduce that Team A will likely beat Team C.

Conclusion:
Mathematical properties are the backbone of mathematical operations, providing a solid foundation for problem-solving and real-world applications. Mastering these properties empowers individuals to navigate complex calculations, analyze data, and make informed decisions in various fields. By understanding and utilizing these principles, we unlock the power of mathematics to unravel the mysteries of the world around us.