Mastering Weak Acid Ph Calculations: A Comprehensive Guide
To calculate the pH of a weak acid, first understand the significance of pH and acid dissociation constants. Use ICE tables to determine equilibrium concentrations of the acid, conjugate base, and hydrogen ions. Solve for hydrogen ion concentration ([H+]) using either the equilibrium concentrations or the quadratic equation. Finally, calculate pH using the formula pH = -log[H+]. This process requires a clear understanding of the weak acid’s dissociation behavior and the equilibrium principles governing weak acid solutions.
Understanding pH: A Gateway to Chemical Solution Insights
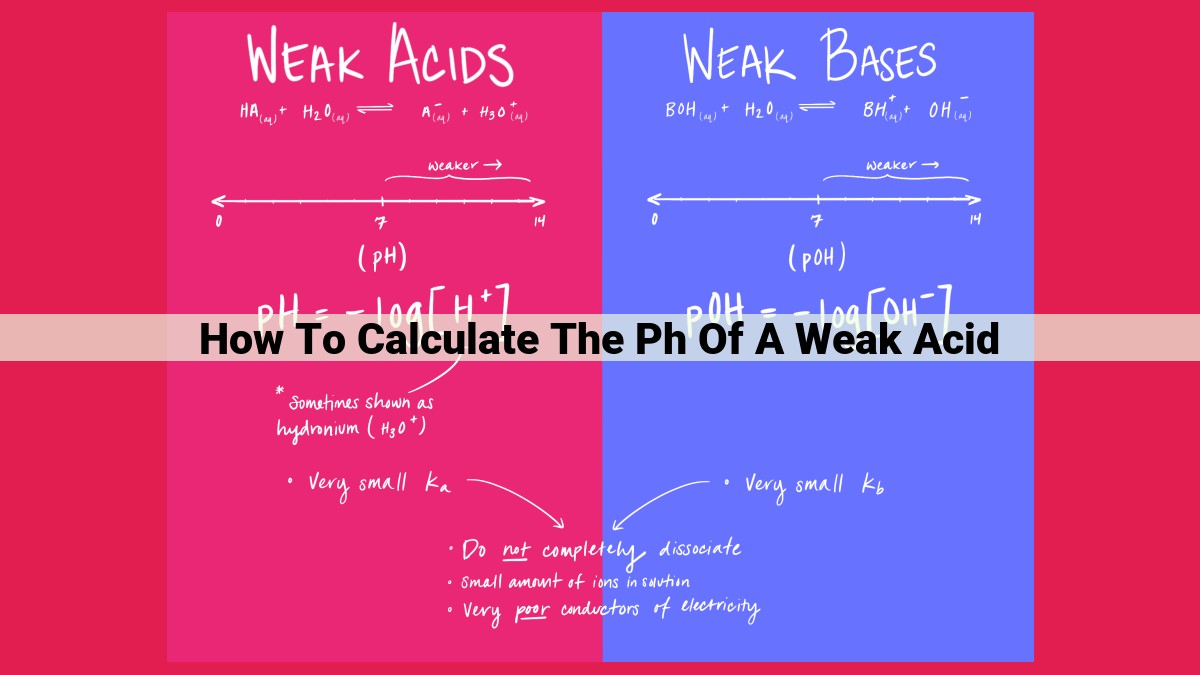
In the realm of chemistry, comprehending pH is paramount. pH (potential of hydrogen) serves as a crucial indicator of a solution’s acidity or basicity, shedding light on its chemical nature.
pH is measured on a scale of 0 to 14, with 0 representing extreme acidity and 14 denoting high basicity. Neutral solutions fall in the middle, with a pH of 7. Understanding pH is essential for various scientific disciplines, including chemistry, biology, and environmental studies.
Acidity and Basicity Defined
Weak acids, unlike their strong counterparts, only partially dissociate in water, releasing hydrogen ions (H+) and their corresponding conjugate bases. This partial dissociation leads to a lower concentration of H+ ions in solution, resulting in a higher pH value.
Unveiling the Fundamental Concepts: pH, Ka, and ICE Tables
Ka (acid dissociation constant) is a numerical value that quantifies the strength of an acid. A higher Ka value indicates a stronger acid, resulting in a lower pH. ICE tables (Initial, Change, Equilibrium) provide a systematic approach to determining equilibrium concentrations in weak acid solutions.
Calculating Equilibrium Concentrations of Weak Acids
To determine equilibrium concentrations, Ka and initial concentrations are utilized. With the help of ICE tables, the initial concentrations of reactants and products are tracked, along with changes in these concentrations as the reaction progresses, ultimately revealing the equilibrium concentrations.
Solving for Hydrogen Ion Concentration: A Quadratic Equation
In certain cases, a quadratic equation is required to solve for the hydrogen ion concentration [H+]. This occurs when the equilibrium constant expression involves a quadratic equation. By solving the quadratic equation, the [H+] value can be obtained, which is crucial for pH calculations.
Calculating pH from Hydrogen Ion Concentration
Once the hydrogen ion concentration [H+] is determined, pH can be calculated using the formula pH = -log[H+]. By plugging in the [H+] value, the pH of the solution can be obtained.
Understanding the concepts outlined in this blog post is vital for accurately calculating the pH of weak acid solutions. By comprehending the interplay between pH, Ka, and ICE tables, and by employing the appropriate equations and techniques, scientists can effectively analyze and interpret chemical solutions.
Weak Acids: A Somewhat Dissociated Species
In the realm of chemistry, we encounter various types of acids, each with distinct characteristics and behaviors. Among them, weak acids stand out for their partial dissociation in aqueous solutions, a phenomenon that sets them apart from their more assertive counterparts, strong acids.
In contrast to strong acids, which completely dissociate, releasing all their hydrogen ions (H+) into the solution, weak acids only partially undergo this process. This incomplete dissociation results in the release of only a fraction of the potential H+ ions. The remaining H+ ions remain bound to the weak acid molecule, forming conjugate bases.
The extent to which a weak acid dissociates is governed by its acid dissociation constant (Ka), a measure of its strength. A larger Ka value indicates a stronger acid, while a smaller Ka value indicates a weaker acid.
The partial dissociation of weak acids can be visualized using the following chemical equation:
HA(aq) + H2O(l) ⇌ H+(aq) + A-(aq)
In this equation, HA represents the weak acid, H+ represents the hydrogen ion, A- represents the conjugate base, and the arrows indicate a reversible reaction.
The equilibrium position of this reaction, which determines the extent of dissociation, is influenced by the Ka value of the weak acid. A higher Ka value shifts the equilibrium to the right, favoring the formation of more H+ ions and conjugate bases. Conversely, a lower Ka value shifts the equilibrium to the left, favoring the formation of less H+ ions and more undissociated weak acid molecules.
Understanding the behavior of weak acids is crucial in various chemical applications, such as pH calculations, buffer solutions, and chemical reactions. By comprehending their partial dissociation and the factors that influence it, we gain valuable insights into the intricate world of acid-base chemistry.
Fundamental Concepts: pH, Ka, and ICE Table
- Explain the concept of pH and its relationship with [H+]: pH = -log[H+].
- Introduce the acid dissociation constant (Ka) as a measure of acid strength.
- Discuss the use of ICE tables to determine equilibrium concentrations in weak acid solutions.
Fundamental Concepts of Weak Acids: pH, Ka, and ICE Table
In the realm of chemistry, understanding the behavior of weak acids is crucial for deciphering the secrets hidden within chemical solutions. These elusive acids, unlike their strong counterparts, showcase a unique ability to partially dissociate, releasing hydrogen ions (H+) and their conjugate bases.
To navigate this intricate dance between weak acids and their ions, we introduce three fundamental concepts: pH, Ka, and ICE tables. pH (potential of hydrogen) measures the acidity or basicity of a solution on a scale of 0 to 14, with 7 representing neutrality. Ka (acid dissociation constant) is the equilibrium constant that quantifies the strength of an acid. A smaller Ka indicates a stronger acid.
Finally, ICE tables (Initial, Change, Equilibrium) provide a systematic approach to determine the equilibrium concentrations of a weak acid solution. These tables track the changes in the concentrations of reactants and products as the system reaches equilibrium.
Understanding the Role of pH and Ka
The relationship between pH and [H+] is inverse and logarithmic: pH = -log[H+]. This means that as the concentration of hydrogen ions increases, the pH decreases, indicating a more acidic solution. Ka, on the other hand, is a measure of how readily an acid dissociates into its ions. A higher Ka value corresponds to a greater extent of dissociation and thus a lower pH.
The Power of ICE Tables
ICE tables serve as valuable tools for unraveling the equilibrium concentrations of a weak acid solution. By setting the initial concentrations, accounting for the changes due to dissociation, and then determining the equilibrium concentrations, we can gain insights into the behavior of the system.
For example, consider a 0.1 M solution of acetic acid (CH3COOH), a weak acid with a Ka of 1.8 x 10^-5. Using an ICE table, we find that at equilibrium, [H+] = 1.34 x 10^-3 M, and thus the pH is 2.87.
Mastering the concepts of pH, Ka, and ICE tables is essential for comprehending the intricacies of weak acid solutions. By unraveling the interplay between these concepts, we unlock the secrets of chemical solutions, empowering us to predict and understand the behavior of these elusive acids.
Calculating Equilibrium Concentrations of Weak Acids
- Explain the use of Ka and initial concentrations to determine equilibrium concentrations.
- Step-by-step guide on setting up and solving ICE tables for weak acids.
Calculating Equilibrium Concentrations of Weak Acids
In chemistry, understanding the behavior of acids and their solutions is crucial. When it comes to weak acids, the key lies in determining their equilibrium concentrations. Weak acids, unlike their strong counterparts, only partially dissociate in water, releasing a limited number of hydrogen ions (H+). This partial dissociation can be quantified using an important concept called the acid dissociation constant (Ka).
Ka, a characteristic of each weak acid, measures the extent to which it dissociates. A higher Ka value indicates a stronger acid that dissociates more extensively. To determine the equilibrium concentrations of a weak acid, we employ a useful tool: the ICE table.
Setting Up an ICE Table for Weak Acids
An ICE table, or Initial-Change-Equilibrium table, is a tabular representation of the changes in concentrations of the reactants and products as a reaction progresses towards equilibrium.
| | Initial | Change | Equilibrium |
|---|---|---|---|
| HA(aq) | [HA]i | -x | [HA]eq |
| H+(aq) | 0 | +x | [H+]eq |
| A-(aq) | 0 | +x | [A-]eq |
In this table:
HA(aq) represents the initial concentration of the weak acid, [HA]i
H+(aq) represents the equilibrium concentration of hydrogen ions, [H+]eq
A-(aq) represents the equilibrium concentration of the conjugate base, [A-]eq
x represents the change in concentration
Solving the ICE Table
To solve an ICE table for a weak acid, follow these steps:
- Substitute Ka and Equilibrium Concentrations: Replace the [HA]eq, [H+]eq, and [A-]eq with their respective equilibrium concentrations.
- Simplify and Solve for x: Solve the resulting equation for x, which represents the change in concentration.
- Calculate Equilibrium Concentrations: Use x to calculate the equilibrium concentrations of [HA]eq, [H+]eq, and [A-]eq.
Example:
Consider a weak acid, HA, with an initial concentration of 0.1 M and a Ka of 1.0 x 10^-5. Using the ICE table and the steps described above, we can calculate the equilibrium concentrations:
| | Initial | Change | Equilibrium |
|---|---|---|---|
| HA(aq) | 0.1 M | -x | 0.1 - x |
| H+(aq) | 0 | +x | x |
| A-(aq) | 0 | +x | x |
Substituting the values and solving for x:
1.0 x 10^-5 = (x^2) / (0.1 - x)
Solving for x, we get x = 1.0 x 10^-3 M.
Therefore:
[HA]eq = 0.1 M - 1.0 x 10^-3 M = 0.099 M
[H+]eq = [A-]eq = 1.0 x 10^-3 M
Understanding the concept of equilibrium concentrations for weak acids empowers chemists to accurately analyze and predict the behavior of acid solutions.
Solving for Hydrogen Ion Concentration: Unraveling the Quadratic Equation
In our quest to understand the intricate world of weak acids, we encounter a crucial scenario where we need to determine the elusive hydrogen ion concentration ([H+]) using the quadratic equation. This equation emerges when the traditional approach, known as the ICE table, fails to provide a straightforward solution.
Imagine a weak acid, let’s call it HA, dissolving in water. As it dissociates, it releases a certain amount of hydrogen ions (H+) and its conjugate base (A-). The equilibrium expression for this dissociation reaction is:
HA(aq) ⇌ H+(aq) + A-(aq)
The equilibrium constant, also known as the acid dissociation constant (Ka) for this reaction is defined as:
Ka = [H+][A-] / [HA]
To determine the equilibrium concentrations of all species involved, we construct an ICE table. However, sometimes, solving for [H+] using this method may not be immediately apparent, especially when [HA] is not negligible compared to [H+] and [A-].
In such cases, we resort to the quadratic equation, which arises from the equilibrium expression:
[H+]^2 + Ka[HA] - Ka[A-] = 0
This quadratic equation can be solved using standard methods, such as factoring or the quadratic formula.
Let’s consider an example to illustrate the process. Suppose we have a 0.1 M solution of HA with a Ka value of 1.0 x 10^-5. Using an ICE table, we initially assume that [H+] = x, [A-] = x, and [HA] = 0.1 – x.
Substituting these values into the quadratic equation and solving for x, we get:
x^2 + (1.0 x 10^-5)(0.1 - x) - (1.0 x 10^-5)x = 0
x^2 + (1.0 x 10^-6) - (1.0 x 10^-5)x = 0
x = 1.0 x 10^-3 M
Therefore, [H+] = 1.0 x 10^-3 M. This value represents the equilibrium concentration of hydrogen ions in the weak acid solution, providing valuable insights into its acidity.
Calculating pH from Hydrogen Ion Concentration
To complete our exploration of weak acids, let’s delve into the final step: calculating pH from the determined hydrogen ion concentration [H+]. This step is crucial for understanding the acidity or basicity of a solution.
Formula for Calculating pH
The pH scale measures the acidity or alkalinity of a solution on a scale of 0 to 14. The formula for calculating pH is a logarithmic function:
pH = -log[H+]
Where:
- pH is the measure of acidity or basicity
- [H+] is the molar concentration of hydrogen ions in the solution
Understanding the Logarithmic Relationship
The logarithmic function means that a small change in [H+] can result in a significant change in pH. For example, a solution with [H+] = 10^-1 M will have a pH of 1, while a solution with [H+] = 10^-10 M will have a pH of 10.
Using the Formula
To calculate the pH of a solution, simply plug the [H+] value into the formula:
pH = -log(0.001) = 3
This means that a solution with [H+] = 0.001 M has a pH of 3, indicating an acidic solution.
Understanding how to calculate pH from hydrogen ion concentration is essential for accurately determining the acidity or basicity of a solution. By following the steps outlined in this article, you can confidently analyze weak acid solutions and apply these concepts in various chemical applications.