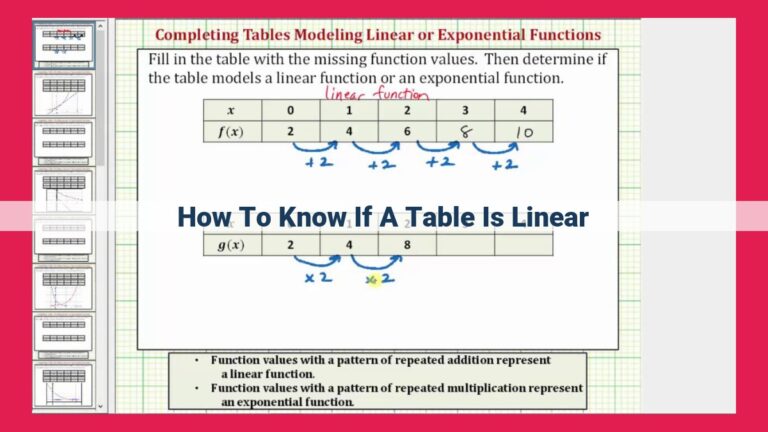
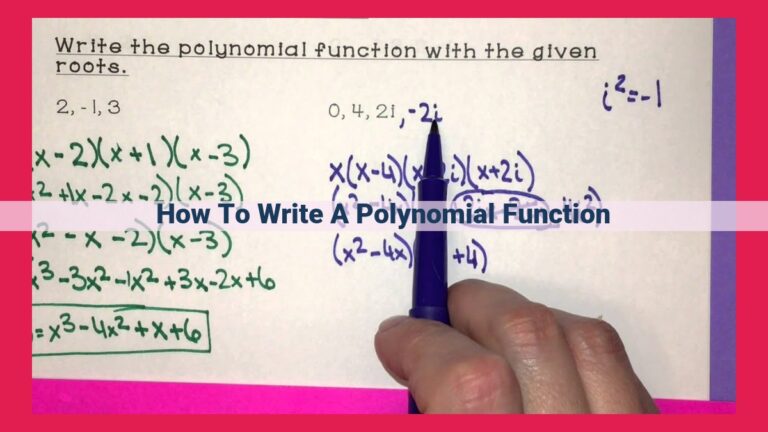
Master Standard Form Polynomials: A Comprehensive Guide For Mathematical Mastery

To write polynomials in standard form, first understand the basics (coefficients, terms, etc.) and the benefits of standard form. Then, rearrange terms in descending degree order, combine like terms, omit leading zeros and decimals, and write in the form ax^n + bx^(n-1) + … + c. Examples include 3x^2 + 2x – 5 and 2.5x^4 – x^2 + 1. By writing polynomials in standard form, mathematical operations become easier and polynomial properties can be more easily identified.

Understanding Polynomial Basics

• Explain the concepts of coefficients, constants, degree, and exponents.
• Define monomials, polynomials, and terms as components of polynomials.

Understanding Polynomial Basics: Demystifying the Building Blocks

In the realm of mathematics, polynomials hold a fundamental place, acting as powerful tools for expressing complex mathematical concepts and solving a wide array of problems. To master the art of manipulating polynomials, it’s essential to delve into their underlying structure, beginning with the comprehension of key terms.

Coefficients, Constants, and Degree

Polynomials are constructed from individual terms, each of which possesses three primary attributes:

• Coefficients: The numeric factors that multiply the variable(s).
• Constants: Terms without any variable factors.
• Degree: The highest exponent of the variable in the term.

For instance, in the term 3x^2, the coefficient is 3, the variable is x, and the degree is 2.

Monomials, Polynomials, and Terms

• Monomials: Single-term polynomials.
• Polynomials: Expressions consisting of multiple terms.
• Terms: Individual components of a polynomial, separated by addition or subtraction signs.

To illustrate, the expression 2x^3 – 5x^2 + 7 is a polynomial with three terms: 2x^3, -5x^2, and 7.

The Significance of Standard Form

When dealing with polynomials, it becomes imperative to adopt a consistent format, known as the standard form, which establishes a universal way of organizing and representing these expressions. This convention offers numerous advantages:

• Clarity: It facilitates ease of reading and comprehension.
• Simplification: It allows for efficient manipulation and simplification.
• Uniformity: It fosters consistency in mathematical communication.

Writing Polynomials in Standard Form: A Step-by-Step Guide

Transforming polynomials into standard form involves several crucial steps:

1. Arrange Terms in Descending Degree Order: List all terms in order, with the highest degree term first.
2. Combine Like Terms: Group and combine terms with the same variable and degree.
3. Simplify: Remove leading zeros and decimal points in coefficients.

For example, the polynomial 5x – 3x^2 + 8 – 2x^2 can be written in standard form as -5x^2 + 5x + 8.

The Significance of Standard Form in Polynomial Expressions

In the realm of polynomials, standard form reigns supreme as the key to unlocking mathematical clarity and computational efficiency. It’s more than just a stylistic choice; it’s a fundamental convention that streamlines polynomial operations, making them a breeze.

Standard form serves as a structured blueprint, organizing polynomial terms in descending order of degree. This organization allows us to quickly identify key features such as the highest degree term and the number of terms, which are crucial for algebraic manipulations.

Moreover, standard form facilitates mathematical operations, such as addition, subtraction, and multiplication. By lining up like terms vertically, we can combine them effortlessly, resulting in simplified and accurate expressions. This orderliness also prevents calculation errors, ensuring reliable results.

In short, standard form is the cornerstone of polynomial manipulation. It simplifies calculations, reduces errors, and enhances our understanding of polynomial expressions. Embracing this convention unlocks a new level of mathematical proficiency, making polynomials less daunting and more manageable.

Writing Polynomials in Standard Form: A Step-by-Step Guide

Understanding the Basics

Before we dive into the intricacies of writing polynomials in standard form, let’s brush up on some essential concepts. A polynomial is an expression consisting of one or more terms, where each term comprises coefficients, constants, and variables raised to various exponents. Coefficients are numerical factors multiplied by variables, while constants are numbers not associated with variables. The degree of a polynomial is determined by the highest exponent of any variable it contains.

Organizing Polynomials in Standard Form

For mathematical operations, it’s crucial to organize polynomials in standard form. Standard form dictates that terms are arranged in descending order of degree, starting with the term containing the highest degree. Coefficients and variables are written explicitly, and like terms (terms with the same variable and exponent) are combined.

Rearranging Polynomial Terms

To rearrange polynomial terms, follow this step-by-step process:

1. Identify the Term with the Highest Degree: Locate the term with the largest exponent and place it at the beginning of the polynomial.
2. Arrange Remaining Terms in Descending Degree Order: Order the remaining terms from highest degree to lowest degree, moving from left to right.

Combining Like Terms

When combining like terms, add or subtract their coefficients while retaining the original variable and exponent. For instance, 3x + 2x simplifies to 5x. It’s essential to remember that variables with different exponents are considered unlike terms and cannot be combined.

Omitting Leading Zeros and Decimal Points

Leading zeros and decimal points in coefficients should be omitted in standard form. For example, instead of 0.5x, write the coefficient as 1/2x. Similarly, 2.0x should be simplified to 2x. This practice ensures consistency and clarity in mathematical expressions.

Examples

Let’s consider some examples to illustrate standard form:

• Polynomial: 3x² + 5x – 2

• Standard form: 3x² + 5x – 2

• Polynomial: x³ – 2x² + 4x – 1

• Standard form: x³ – 2x² + 4x – 1

• Polynomial: 2 – 3y + y³

• Standard form: y³ – 3y + 2

Writing polynomials in standard form is a fundamental skill for mathematical proficiency. By following the outlined steps and adhering to the principles of standard form, you can effectively communicate and manipulate polynomial expressions. Remember to arrange terms in descending degree order, combine like terms, and omit leading zeros and decimal points to ensure clarity and consistency in your mathematical work.

Examples of Standard Form: Unveiling the Essence of Polynomials

Expanding Polynomial Horizons

In the realm of mathematics, polynomials stand as powerful tools for expressing algebraic relationships. To harness their full potential, it’s essential to understand the concept of standard form, a structured format that organizes polynomial terms in a consistent manner.

Dissecting Standard Form

Polynomials written in standard form adhere to the following key principles:

• Terms are arranged in descending order of degree, which represents the highest exponent on the variable.
• Like terms, variables with the same exponent, are combined to simplify the polynomial.
• Leading zeros and decimal points in coefficients are omitted.

Illustrative Examples

Let’s explore a few examples to clarify standard form:

• Polynomial 1: 2x³ – 5x² + 7x + 3

  • Highest degree term: 2x³
  • Number of terms: 4

• Polynomial 2: -4y⁴ + 3y³ – 2y² + 6

  • Highest degree term: -4y⁴
  • Number of terms: 3

• Polynomial 3: 0.5z² – 0.25z + 1

  • Highest degree term: 0.5z²
  • Number of terms: 3

Identifying Key Features

As you examine these examples, note that:

• The highest degree term appears first, followed by terms in descending order of degree.
• Coefficients are simplified, without leading zeros or decimal points.
• The number of terms simply counts the individual terms in the polynomial.

A Journey into Standard Form Proficiency

Mastering standard form empowers you with a deeper understanding of polynomials. It facilitates algebraic operations, such as addition, subtraction, and multiplication, allowing you to solve equations and derive new relationships. Moreover, standard form serves as a universal language in mathematics, enabling clear communication of polynomial expressions.

So embrace the power of standard form and unlock the secrets of polynomials. By presenting them in a structured and consistent format, you can conquer algebraic challenges with confidence and precision.