Mastering Square Root Operations: Unrooting, Eliminating, And Solving
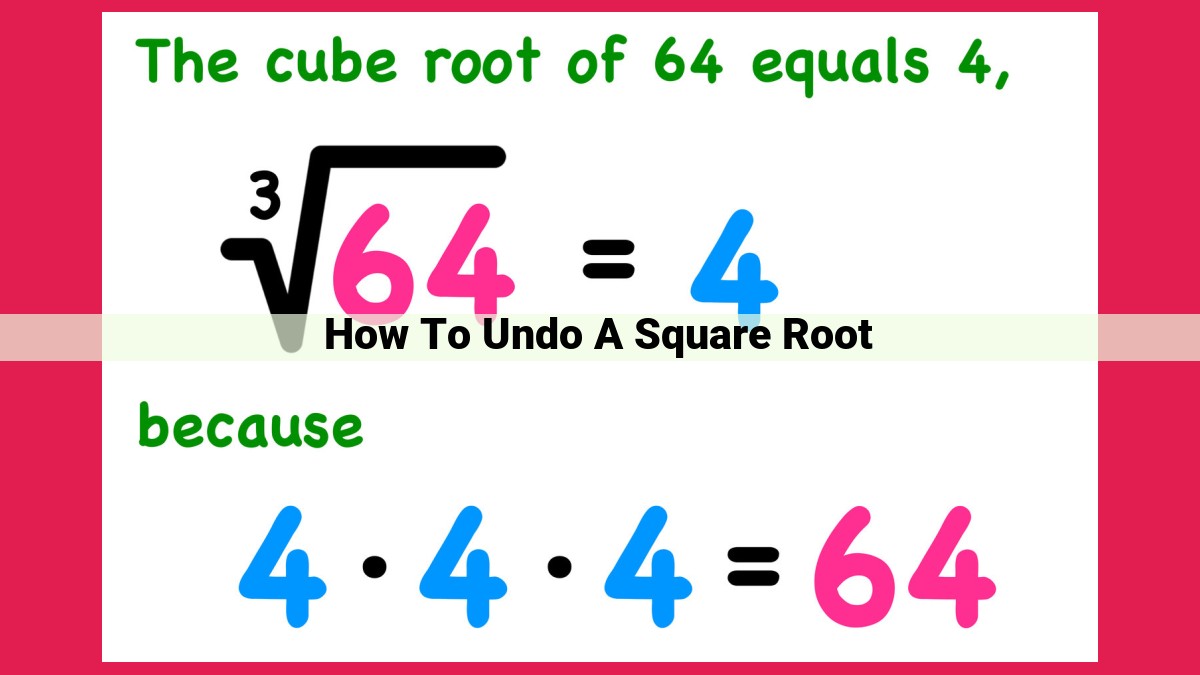
To “undo” a square root (a√b), you perform the “unroot” operation (a√b)¹. This involves multiplying the square root by itself, effectively reversing the squaring operation that created the square root in the first place. Alternatively, you can square both sides of an equation to eliminate a variable within a square root, isolating it for solution. These techniques find application in many fields, including engineering and physics, where solving equations with square roots is essential for calculations and problem-solving.
The Mysterious Case of the Unsquarer: Unraveling the Inverse Operation
In the enigmatic realm of mathematics, square roots and their enigmatic inverse, the unsquarer, reign supreme. Embark on a captivating journey as we delve into the techniques that unravel the secrets of “undoing” a square root, revealing the hidden wonders that lie within.
Square roots, the enigmatic counterparts of squaring, represent a captivating operation that unveils the hidden depths of a number’s essence. We’re familiar with finding square roots, but what if we seek to reverse the process? Enter the unsquarer, the elusive operation that unravels the mysteries left behind by square roots.
Inverting a Square Root: Mastering the “Unroot” Operation
In the realm of mathematics, square roots play a pivotal role in unraveling equations and understanding complex phenomena. But what happens when we need to reverse this operation, akin to unravelling a Gordian knot? Enter the enigmatic concept of the “unroot” operation, the inverse of square rooting, which empowers us to undo the enigmatic embrace of square roots.
Defining the “Unroot” Operation
To comprehend the essence of the “unroot” operation, we must envision it as the antidote to square rooting. Just as division reverses multiplication, the “unroot” operation unravels the effects of square rooting. It is the mathematical equivalent of a time-traveler, transporting us back to the pristine world before square roots were applied.
Unveiling the “Unroot” of a Square Root Expression
To master the “unroot” operation, we embark on a journey that begins with a square root expression. Let’s consider the enigmatic expression √(x). The corresponding “unroot” expression is x^2, where x represents the unknown value hidden beneath the square root’s veil. This relationship stems from the fundamental property of square rooting: when a number is squared, the result is the square root of its square.
Exploring Related Concepts for a Deeper Understanding
To fully grasp the “unroot” operation, we delve into related concepts that illuminate its intricacies. Among these concepts, two stand out: squaring both sides of an equation and isolating the variable.
Squaring both sides of an equation, like a magical incantation, transforms the equation into an abode where variables can be unearthed. This technique allows us to exorcise the square root’s hold on variables, revealing their true identities.
Isolating the variable is akin to a detective isolating a suspect. By manipulating the equation, we isolate the variable within the square root, making it vulnerable to the transformative power of the “unroot” operation.
Calculating the “Unroot”: An Alternative Explanation for Inverting Square Roots
In our quest to unravel the mysteries of mathematics, we often encounter equations with square roots, leaving us wondering how to “undo” them. Enter the concept of the “unroot” operation, an indispensable tool for navigating these mathematical challenges.
Imagine, if you will, a square root as a cloak of secrecy concealing the true value of a hidden number. The “unroot” operation serves as the key to unlock this secret, revealing the number that, when squared, produces the original square root expression.
To grasp the essence of the “unroot,” let’s break it down into a simple formula:
Unroot(√x) = x^(1/2)
Here, √x represents the square root of x, and the term “unroot” is used to denote the inverse operation.
Just as addition and subtraction are inverse operations, so too are squaring and taking square roots. The act of “unrooting” is essentially the inverse of squaring. By squaring a number, we create its square, and by taking the square root, we undo this operation.
To illustrate this concept, consider the following example:
Unroot(√16) = 16^(1/2) = 4
In this case, we start with the square root of 16, which is 4. By applying the “unroot” operation, we square the hidden number, which in this instance is 4, and recover the original value of 16.
This alternative explanation provides a comprehensive understanding of the “unroot” operation, demonstrating how it unveils the secret hidden within square roots, allowing us to navigate complex equations and unlock the mysteries of mathematics.
Squaring Both Sides of an Equation: Unlocking Hidden Variables
In the realm of mathematics, square roots often hold variables captive, obscuring their true values. But fear not, for like a key that unlocks a secret chamber, squaring both sides of an equation can release these imprisoned variables, revealing their hidden secrets.
Consider the enigmatic equation:
√x = 5
How do we liberate the elusive x? Squaring both sides of the equation is our secret weapon. By doing so, we elevate the square root to the power of 2, effectively “undoing” its operation:
(√x)² = 5²
Simplifying further, we obtain:
x = 25
x has been set free! Squaring both sides of the equation transformed the square root into its inverse operation, unveiling the hidden variable.
Another captivating example:
√(x + 3) = 4
Squaring both sides, we tame the square root:
(√(x + 3))² = 4²
Simplifying, we unravel the mystery:
x + 3 = 16
x = 13
This technique is particularly valuable when solving equations with variables buried within multiple square roots. Squaring both sides aligns the square roots, allowing us to unravel their entangled secrets.
Key takeaways:
- Squaring both sides of an equation can undo square roots, revealing hidden variables.
- This technique is especially useful for solving equations with variables within multiple square roots.
- By squaring both sides, we effectively invert the square root operation, transforming it into its inverse.
**Undoing Square Roots: Unraveling the Inverse Operation**
Square roots, the inverse of squaring, are fundamental mathematical operations. To fully comprehend this concept, understanding their inverse operation – unsquarer – is crucial. This guide explores techniques for “undoing” a square root, empowering readers to tackle complex equations and real-world applications.
Inverting a Square Root – The “Unroot” Operation
The unroot operation is the inverse of square rooting. To find the “unroot” of a square root expression, simply take the square of the expression. For example, the “unroot” of √9 is 9. This relationship is mathematically expressed as:
(√x)² = x
Understanding the “unroot” requires relating it to the concept of inverting a fraction. Just as multiplying a fraction by its reciprocal results in 1, inverting a square root involves multiplying it by its reciprocal (i.e., squaring it).
Calculating the “Unroot” – Alternative Explanation
An alternative approach to calculating the “unroot” involves the following steps:
- Find the square of the square root expression.
- Take the square root of the result from step 1.
For instance, to find the “unroot” of √16:
- Square √16 to get (√16)² = 16
- Take the square root of 16 to get √16 = 4
Squaring Both Sides of an Equation
Squaring both sides of an equation is a powerful technique when dealing with equations containing square roots. This approach helps eliminate the square root, allowing for the solution of the variable within the square root.
Consider the equation:
√x = 5
Squaring both sides:
(√x)² = 5²
Simplifying:
x = 25
This technique allows for the isolation of the variable within the square root, making it easier to solve.
Applications in Real-World Scenarios
“Undoing” square roots finds practical applications in various fields:
- Engineering: Calculating the forces acting on structures and determining the stresses within materials often involves using square roots.
- Physics: Understanding the motion of objects, such as projectile trajectories, requires the use of square roots.
- Finance: Evaluating investment returns and calculating the time required for investments to double or triple involves using square roots.
Mastering the techniques for “undoing” square roots is essential for solving complex equations and tackling real-world applications. Understanding the concept of unsquarer and its relation to squaring both sides of an equation empowers individuals to approach mathematical problems with confidence. By embracing these techniques, readers can unlock the full potential of square roots, enhancing their problem-solving abilities and gaining a deeper appreciation for this fundamental mathematical operation.