Mastering Simplifying Fractions With Square Roots For Mathematical Proficiency
Simplifying fractions with square roots involves breaking down complex fractions into their simplest form. Understanding fractions and square roots is crucial. Multiplying and dividing fractions, simplifying square roots by factoring, and combining like radicals are key steps. Rationalizing the denominator eliminates irrational denominators. By following these steps, you can simplify fractions with square roots effectively, ensuring correct representation and accurate calculations. Mastering this technique empowers you to solve mathematical problems and advance your understanding of rational and irrational numbers.
Simplifying Fractions with Square Roots: A Guide to Mastery
In the realm of mathematics, fractions with square roots can be daunting, but their simplification is crucial for unlocking their full potential. These fractions arise in various scenarios, from geometry to physics, demanding our ability to handle them seamlessly. Embark on this journey to unravel the art of simplifying fractions with square roots, empowering you to conquer this mathematical challenge with ease.
Understanding the Components
Before delving into simplification, let’s establish a solid understanding of fractions. A fraction, denoted as a/b, consists of two parts: the numerator a and the denominator b. The numerator represents the number of equal parts in a whole, while the denominator indicates the total number of such parts. Simplifying fractions involves finding their equivalent simplest form with the smallest possible numerator and denominator.
The Significance of Square Roots
Square roots are numbers that, when multiplied by themselves, produce another number. Understanding square roots is essential for simplifying fractions. A perfect square is a number that can be written as the square of an integer. For instance, 16 is a perfect square because it can be expressed as 4², where 4 is the square root of 16.
Purpose of Simplifying Fractions with Square Roots
Simplifying fractions with square roots serves several purposes:
- Making fractions more manageable and easier to work with
- Facilitating comparisons and ordering of fractions
- Extracting numerical information from expressions with square roots
- Solving equations and inequalities involving fractions with square roots
Understanding Fractions: A Path to Mathematical Mastery
Fractions, the enigmatic building blocks of mathematics, hold a special place in our numerical world. They embody the concept of representing parts of a whole, revealing the intricate relationships between quantities. At their core lie two essential components: the numerator and the denominator. The numerator, perched atop the fraction bar, represents the number of parts being considered, while the denominator, residing below, indicates the total number of parts.
Fractions serve as a versatile tool for expressing ratios, or the comparison of two quantities. For instance, the fraction 2/3 signifies that the ratio of the first quantity to the second is 2 to 3. Furthermore, fractions can take on multiple forms that are equivalent to each other. For example, the fractions 1/2, 2/4, and 3/6 all represent the same ratio, indicating that half of the whole is being considered.
Simplifying fractions is an essential skill that unlocks their true potential. By reducing fractions to their simplest form, we eliminate unnecessary complexities and reveal their inherent beauty. This process involves finding the greatest common factor (GCF) between the numerator and denominator and dividing both by the GCF. Through simplification, we uncover the most concise representation of a fraction, making calculations and comparisons more manageable.
Understanding Square Roots: The Key to Simplifying Fractions
Do you recall struggling with simplifying fractions involving square roots? Let’s dive into the fascinating world of square roots to unravel this mystery and make these fractions a piece of cake!
Defining Square Roots:
At the heart of square roots lies the concept of a perfect square. A perfect square is a number that results from multiplying any whole number by itself. For instance, 16 is a perfect square because it’s 4 x 4. The square root of a number is the number that, when multiplied by itself, gives us the original number. So, the square root of 16 is 4.
Significance of Square Roots:
Square roots are more than just mathematical symbols. They’re indispensable tools in various fields, from architecture to physics. In geometry, square roots help us calculate the lengths of diagonals and find the areas of triangles, squares, and rectangles. They empower engineers to design structures with precise angles and dimensions. And in physics, square roots are essential for understanding concepts such as velocity and acceleration.
Perfect Squares and Irrational Numbers:
Not all numbers have nice, whole-number square roots. Sometimes, we encounter irrational numbers, which are numbers that cannot be expressed as a simple fraction. For example, the square root of 2 is an irrational number. This means that it cannot be written as a/b, where a and b are integers. Instead, irrational numbers are non-terminating and non-repeating decimals.
Examples:
Let’s consider the fraction √16/4. We can simplify this fraction by recognizing that √16 is simply 4. So, we have 4/4, which equals 1.
On the other hand, √8/2 cannot be simplified further. This is because √8 is an irrational number, and we cannot express it as a simple fraction.
Operating with Fractions: A Guide to Simplifying Expressions
Understanding fractions is crucial in mathematics, and it becomes even more essential when dealing with square roots. In this section, we’ll delve into the rules of multiplying and dividing fractions, as well as introduce techniques like cross-multiplication and factoring to help us simplify these expressions.
Multiplying Fractions
When multiplying fractions, we simply multiply their numerators together and their denominators together. For instance, if we want to multiply 1/2 and 3/4, we get (1 x 3) / (2 x 4) = 3/8. Remember, the order of multiplication doesn’t matter, so 1/2 x 3/4 is the same as 3/4 x 1/2.
Dividing Fractions
Dividing fractions is a bit trickier. Instead of dividing the first fraction by the second, we flip the second fraction and multiply. So, to divide 1/2 by 3/4, we flip 3/4 to 4/3 and multiply: 1/2 x 4/3 = 2/3.
Cross-Multiplication
Cross-multiplication is a shortcut we can use to simplify fractions before multiplying them. Instead of multiplying the numerators and denominators separately, we can simply multiply the numerator of each fraction by the denominator of the other. For example, to simplify 1/2 x 3/4, we cross-multiply to get (1 x 4) / (2 x 3) = 2/6.
Factoring
Factoring is another technique that can help us simplify fractions. By factoring out any common factors between the numerator and denominator, we can reduce the fraction to its simplest form. For example, if we have the fraction 12/24, we can factor out a common factor of 12 from both the numerator and denominator: 12/24 = (12/12) / (24/12) = 1/2.
Simplifying Square Roots: A Step-by-Step Guide
In the world of mathematics, fractions and square roots often go hand in hand. Simplifying these expressions allows us to understand them better and perform calculations more efficiently. Let’s dive into the exciting journey of simplifying square roots!
What is a Square Root?
A square root is a number that, when multiplied by itself, gives you another number. For example, the square root of 9 is 3 because 3 x 3 = 9. When we write a square root mathematically, we use the symbol √.
Simplifying Square Roots
To simplify a square root, we look for perfect squares, which are numbers that can be written as the square of an integer. For example, 16 is a perfect square because it can be written as 4², where 4 is an integer.
Steps to Simplify Square Roots:
- Factor out any perfect squares from the radicand (the number under the square root symbol).
- Take the square root of each perfect square factor.
- Combine the simplified radicals using the rules of addition or subtraction.
For instance, let’s simplify the square root of 18.
√18 = √(9 x 2)
= √9 × √2
= 3√2
You can factor out 9 because it is a perfect square, and its square root is 3. Then, you take the square root of 2, which is √2. Finally, you combine them using the multiplication rule for radicals.
Prime Factorization and Perfect Squares
Prime factorization is a technique for writing a number as a product of prime numbers. Prime numbers are numbers that can only be divided by themselves and 1.
By using prime factorization, we can more easily identify perfect squares. If the prime factorization of a number contains only perfect squares, then the number itself is a perfect square.
For example, the prime factorization of 18 is 2 x 3². Since both 2 and 3² are perfect squares, we know that 18 is also a perfect square. This understanding helps us simplify square roots even further.
Combining Like Radicals:
- Define like radicals and explain how to combine them.
- Introduce monomials, polynomials, and the distributive property.
Combining Like Radicals: The Harmony of Similar Roots
In the world of mathematics, where numbers dance and equations weave intricate tales, there exists a captivating concept known as “like radicals.” Just as musical notes can harmonize when they share the same root, so too can radicals with similar roots be combined to create a more elegant and simplified expression.
Defining Like Radicals
Like radicals are radicals that have the same radicand. The radicand is the number or expression inside the radical symbol. For example, √2 and √8 are like radicals because they both have the radicand 2.
Combining Like Radicals
To combine like radicals, we simply add or subtract their coefficients, the numbers in front of the radical symbol. The radicand remains unchanged. For instance, we can combine 3√2 and 2√2 as follows:
3√2 + 2√2 = (3 + 2)√2 = **5√2**
Monomials and Polynomials
In algebra, a monomial is an expression with only one term, such as 3√2 or 2x. A polynomial is an expression with two or more terms, such as 3√2 + 2√2 or 2x + 5.
The Distributive Property
Combining like radicals often involves using the distributive property, which states that a(b + c) = ab + ac. Applying this property, we can split the square root into individual terms and combine the coefficients. For example:
√(x + 3) - √x = √(x) + √(3) - √x = **√3**
Simplifying Result
After combining like radicals, we may be able to simplify the result further. For instance, if we have 2√12, we can factor out √4 from the radicand:
2√12 = 2√(4 × 3) = 2 × 2√3 = **4√3**
Combining like radicals is a fundamental skill in algebra that allows us to simplify and manipulate expressions involving square roots. By understanding the concept of like radicals, monomials, polynomials, and the distributive property, we can conquer the complexities of fractional expressions and unlock their true beauty. Remember, the harmony of similar roots is the key to unraveling the mysteries of fractions with square roots.
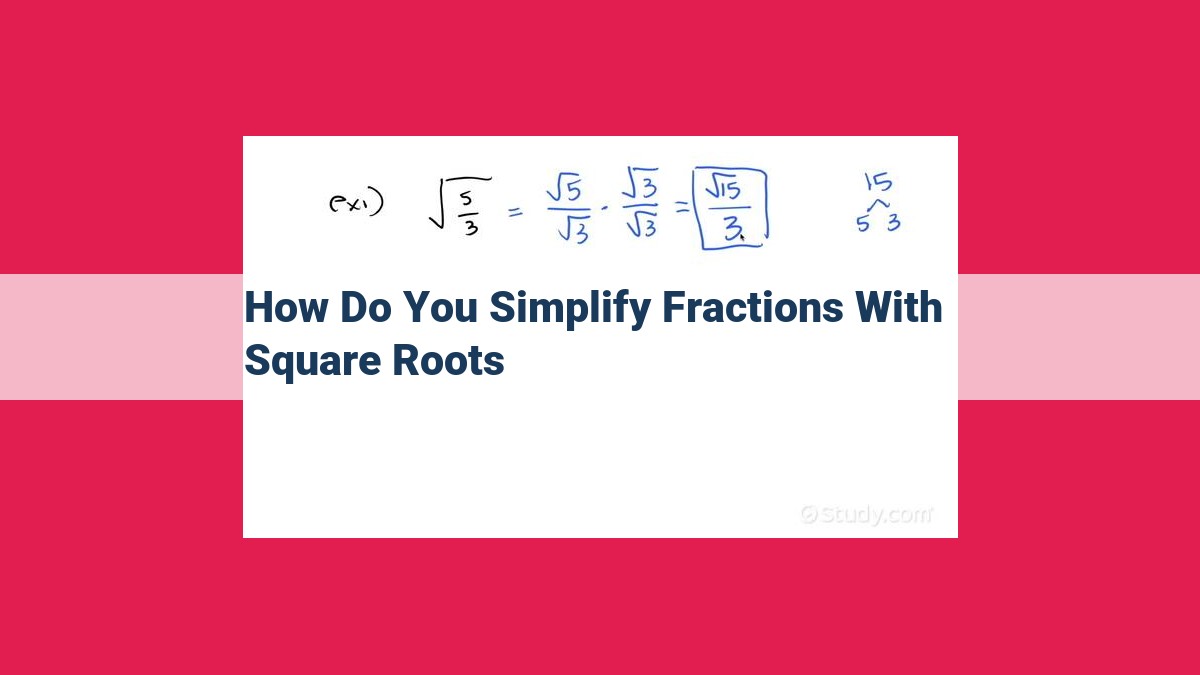
Rationalizing the Denominator: Bringing Fractions to a Whole New Level
In the realm of mathematics, fractions often rear their enigmatic heads, especially when compounded with the complexities of square roots. To conquer such fractions, a technique known as rationalizing the denominator emerged as a beacon of clarity.
Rationalizing the denominator is a process that transforms a fraction with a denominator containing square roots into an equivalent fraction with a rational denominator (one without square roots). This transformation is achieved through the clever use of conjugate expressions and perfect square trinomials.
Conjugate Expressions: The Superhero Sidekicks of Square Roots
Conjugate expressions are two expressions that differ only by the sign between them. For example, (\sqrt{2} – \sqrt{2}) is a conjugate expression. The product of conjugate expressions has a special property: it results in a perfect square trinomial.
Perfect Square Trinomials: The Building Blocks of Rationalization
Perfect square trinomials are quadratic expressions that can be factored into the square of a binomial. For instance, ((a + b)^2 = a^2 + 2ab + b^2) is a perfect square trinomial.
The Rationalization Process: Unveiling the Rational Denominator
To rationalize a denominator, we multiply both the numerator and denominator by the conjugate of the denominator. This operation eliminates the square root from the denominator and transforms it into a perfect square trinomial.
For example, to rationalize the fraction (\frac{1}{\sqrt{2}}), we multiply both the numerator and denominator by (\sqrt{2}), the conjugate of (\sqrt{2}):
$$\frac{1}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}$$
The denominator is now rational, and the transformed fraction is equivalent to the original fraction.
Rationalizing denominators is a crucial skill in mathematics, enabling us to simplify fractions, solve equations, and unlock the mysteries of higher-level math. It’s like having a secret weapon in your mathematical arsenal, giving you the confidence to tackle any fraction that dares to stand in your way.