Mastering Line Integrals: Essential Techniques And Applications

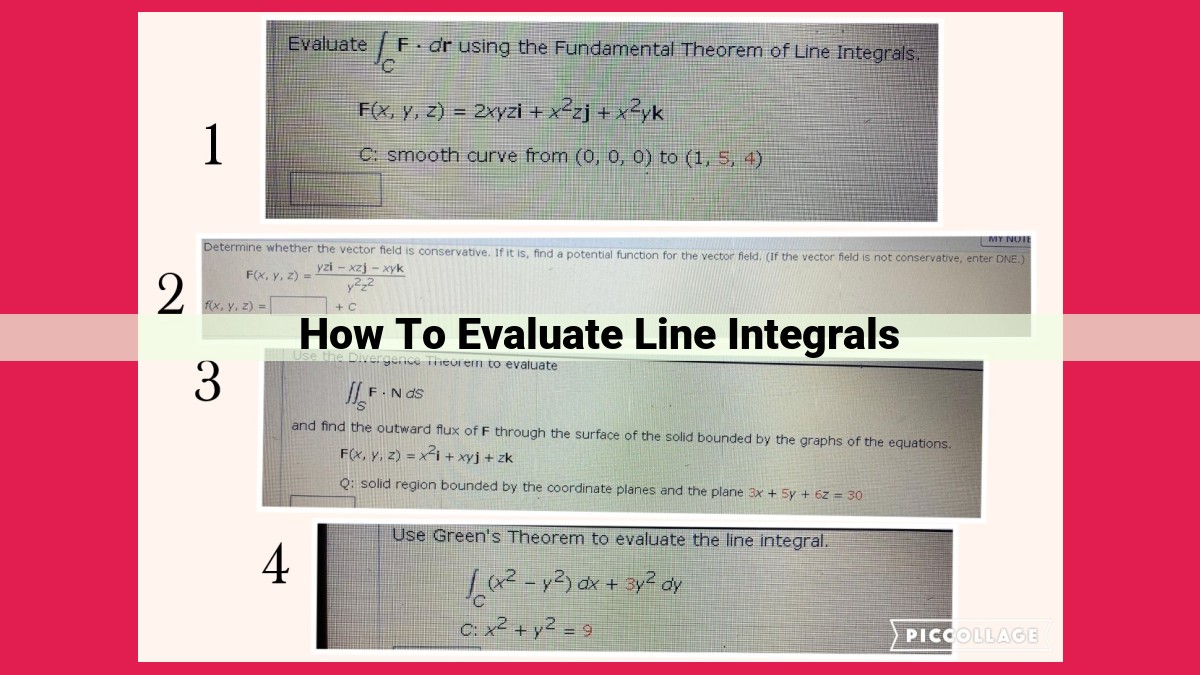
To evaluate line integrals, parameterize the curve, evaluate the integrand along the curve, and integrate with respect to the parameter. If the curve is closed, the line integral can be evaluated using the fundamental theorem of line integrals. If the vector field is conservative, the line integral is path-independent and can be evaluated using the gradient theorem. Green’s theorem, Stokes’ theorem, and the divergence theorem provide techniques to evaluate line integrals in two and three dimensions using surface integrals and triple integrals, respectively.

• Definition and mathematical representation of line integrals
• Concept of paths and vector fields

In the realm of mathematics, line integrals play a pivotal role in unlocking the secrets of curves and vector fields. Imagine a path winding its way through space, like a thread weaving through a tapestry. Along this path, a vector field, a symphony of arrows, flows and ebbs.

Decoding Line Integrals

Line integrals capture the essence of this dynamic interplay between paths and vector fields. They are mathematical tools that allow us to quantify the cumulative effect of the vector field along the path. In essence, a line integral calculates the net value of the vector field as it “travels” along the path.

Represented symbolically, a line integral takes the form of an integral sign placed over a path, denoted by the symbol ∫_C. Within this integral, we encounter a function called F(x,y,z), which represents the vector field, and an infinitesimal displacement vector dr, which traces the direction and magnitude of the path.

Paths and Vector Fields: A Symphony of Movement

Paths, the foundations of line integrals, can take on diverse forms. They can be smooth and graceful, like the arc of a rainbow, or jagged and intricate, like the silhouette of a lightning bolt. The parameterization of a path defines its specific shape, providing a mathematical recipe for tracing its every twist and turn.

Vector fields, on the other hand, are collections of vectors assigned to each point in space. They bring the path to life, imbuing it with direction and magnitude. Each vector in the field represents the strength and orientation of a force, a flow, or some other physical quantity.

Unveiling the Fundamental Concepts

To delve deeper into the world of line integrals, we must first lay the groundwork with essential concepts. Curves, parameterizations, orientations, vector fields—these building blocks form the framework for understanding the intricate dance between paths and fields.

Once these concepts are firmly grasped, we embark on the exploration of theorems such as the Fundamental Theorem of Line Integrals, Green’s Theorem, Stokes’ Theorem, and the Divergence Theorem. These theorems bridge the gap between line integrals and other mathematical constructs, paving the way for a deeper comprehension of complex physical phenomena.

Essential Concepts for Understanding Line Integrals

Embark on a mathematical journey as we explore the captivating realm of line integrals. To grasp this concept fully, let’s delve into three fundamental elements:

Curves: The Contours of Integration

Contours, paths, or curves are the trajectories along which line integrals are calculated. We classify them based on their characteristics:

• Closed Curves: Paths that begin and end at the same point, forming a continuous loop.
• Open Curves: Paths having distinct endpoints, with no self-intersections.
• Smooth Curves: Curves that are continuous and have well-defined tangents at each point.
• Piecewise Smooth Curves: Curves that may have sharp corners or kinks, but are smooth over each individual segment.

Vector Fields: The Essence of Movement

Vector fields are functions that assign a vector to each point in a region. These vectors represent various physical quantities, such as velocity, force, or temperature.

• Components: Vector fields have x, y, and z components, indicating the direction and magnitude of the vector at each point.
• Representations: Vector fields can be graphically represented as arrows or streamlines, providing a visual representation of the field’s behavior.

Path Integrals: Simplifying the Journey

Path integrals are a special type of line integral that involve functions along a specific path. By parameterizing the path as a function of a single variable, we can simplify the evaluation of line integrals.

• Parameterization: Finding a function that represents the path in terms of a single parameter.
• Line Integral as Path Integral: Expressing the line integral as an integral over the path parameter.

The Fundamental Theorem of Line Integrals: Unraveling the Mystery

In our exploration of line integrals, we encounter a pivotal theorem that bridges the gap between complex calculations and elegant solutions. The Fundamental Theorem of Line Integrals unlocks a profound connection between line integrals and path integrals, a connection that simplifies our journey and sheds light on the underlying principles.

Consider a vector field, a representation of a force or velocity acting at every point in space. Imagine a particle traversing a path in this vector field. The line integral measures the total effect of this force or velocity along the path. However, evaluating a line integral can be cumbersome.

Enter the Fundamental Theorem of Line Integrals. This theorem asserts that, under certain conditions, the line integral of a vector field along a path is equivalent to the path integral of the same vector field along the same path. In other words, instead of integrating along the entire intricate path, we can simply integrate along the endpoints of the path.

The key to using this theorem lies in identifying conservative vector fields. These are vector fields where the line integral around any closed path is always zero. For conservative vector fields, the path integral depends only on the endpoints of the path, allowing us to bypass the complexities of the actual path.

The Fundamental Theorem of Line Integrals is not merely a mathematical trick; it has profound implications in physics and engineering. It enables us to calculate work, circulation, and fluid flow with ease, providing valuable insights into real-world phenomena. It also serves as a testament to the power of mathematics to simplify the complex world around us.

Green’s Theorem: A Bridge Between Line Integrals and Area Integrals

In the realm of mathematics, Green’s Theorem emerges as a cornerstone concept for understanding the interplay between line integrals and area integrals. Imagine a two-dimensional region bounded by a closed curve. Green’s Theorem provides a powerful tool to evaluate the line integral around this curve in terms of an integral over the enclosed area.

The theorem states that the line integral around a positively oriented, piecewise smooth boundary curve of a region R is equal to the area integral of the curl of the vector field over the region R. In other words:

∮C F · dr = ∬R (∂Q/∂x – ∂P/∂y) dA

where:

• C is a positively oriented boundary curve of the region R
• F = P i + Q j is a vector field
• dr is a differential displacement vector along the curve C
• dA is a differential area element in the region R

Green’s Theorem offers a convenient method to evaluate line integrals by converting them into area integrals. This is particularly useful when the vector field has a curl that is easy to calculate, while the line integral itself may be more complex.

For example, if you have a vector field F = (x^2 + y) i + (x – y^2) j and want to evaluate the line integral around a square with vertices at (0, 0), (1, 0), (1, 1), and (0, 1), you can apply Green’s Theorem to simplify the calculation.

In this scenario, the curl of F is 2, which is constant throughout the region. Applying Green’s Theorem, you can evaluate the line integral as:

∮C F · dr = ∬R (∂Q/∂x – ∂P/∂y) dA = ∬R 2 dA = 2(area of square) = 2

Therefore, the line integral around the square is equal to 2.

Green’s Theorem finds wide applications in physics and engineering. It is used to calculate work done by forces, fluid circulation, and heat transfer, among other phenomena. By providing a connection between line integrals and area integrals, Green’s Theorem simplifies the analysis of physical systems and enhances our understanding of the underlying mathematical principles.

Stokes’ Theorem: A Gateway to Line Integrals in Three Dimensions

Stokes’ Theorem: Bridging the Gap between Curved Lines and Surfaces

As we delve deeper into the realm of line integrals, we encounter Stokes’ Theorem, a remarkable extension of Green’s Theorem to three dimensions. This theorem provides a powerful tool to evaluate line integrals in the context of surfaces.

Stokes’ Theorem states that the line integral of a vector field around the boundary of an oriented surface is equal to the surface integral of the curl of the vector field over that surface. In other words, it relates the behavior of a vector field along a curve that encloses a surface to the intrinsic properties of the surface itself.

Unlocking the Potential of Stokes’ Theorem

Stokes’ Theorem opens up a whole new world of possibilities for evaluating line integrals. It allows us to convert complex line integrals on arbitrary surfaces into simpler surface integrals that are often easier to compute.

The surface integral in Stokes’ Theorem involves the curl of the vector field, which measures the amount of “circulation” or “rotation” around a given point on the surface. This concept is crucial for understanding the behavior of vector fields in three dimensions.

Applications in Various Fields

Stokes’ Theorem finds applications in a wide array of fields, including:

• Fluid dynamics: Calculating the flow rate of fluids through pipes or around objects
• Electromagnetism: Determining the magnetic field generated by current-carrying wires
• Material science: Analyzing the strain and stress distributions in deformed structures

Stokes’ Theorem: A Cornerstone of Vector Calculus

Stokes’ Theorem is an essential tool for understanding and manipulating line integrals in three dimensions. It provides a framework for relating the behavior of vector fields on surfaces to their behavior along the boundaries of those surfaces. As you continue your exploration of vector calculus, Stokes’ Theorem will undoubtedly play a pivotal role in expanding your understanding and problem-solving abilities.

The Divergence Theorem: A Bridge Between Surface and Triple Integrals

In the realm of calculus, line integrals play a pivotal role in understanding the behavior of vector fields along paths. One of the most profound theorems in this domain is the Divergence Theorem, which establishes a crucial connection between surface integrals and triple integrals.

Imagine a fluid flowing through a three-dimensional region. The divergence of the fluid’s velocity field measures the net flow of fluid out of a small volume around a point. The Divergence Theorem asserts that the surface integral of the divergence of a vector field over a closed surface enclosing a region is equal to the triple integral of the divergence of the field throughout the region.

In mathematical terms, let F be a vector field defined in a simply connected region R bounded by a closed surface S. Then, the Divergence Theorem states:

∫∫_S F · n dS = ∭_R ∇ · F dV

where:

• n is the outward unit normal to the surface S
• ∇ · F is the divergence of the vector field F
• dS is the surface element
• dV is the volume element

The Divergence Theorem finds numerous applications in physics and engineering. For instance, it can be used to calculate the total flux of a fluid through a surface, the net electric charge within a volume, or the rate at which heat is flowing out of a region.

By understanding the connection between surface and triple integrals established by the Divergence Theorem, we gain a deeper comprehension of the flow and behavior of vector fields in three dimensions. It empowers us to solve complex problems involving fluid dynamics, electromagnetism, and heat transfer, providing invaluable insights into the physical world around us.

Practical Applications of Line Integrals

Beyond theoretical concepts, line integrals find practical applications in various scientific and engineering fields:

• Calculating Work: Line integrals can determine the work done by a force along a particle’s trajectory. In physics, this is crucial for understanding the dynamics of moving objects, such as the energy required to lift an object against gravity.

• Circulation: Line integrals also measure the circulation of a vector field around a closed path. This concept is vital in fluid mechanics to analyze the flow of fluids, such as the velocity of water in a river or the airflow around an airplane wing.

• Real-World Examples:

  • In electrical engineering, line integrals are used to calculate the current flow in electrical circuits and the voltage drop across resistors.
  • In mechanical engineering, line integrals can determine the stress distribution in structural components and the deflection of beams under load.
  • In fluid dynamics, line integrals are employed to analyze the streamlines and predict the flow patterns of fluids in pipelines and around immersed objects.

Understanding line integrals empowers scientists and engineers to tackle complex real-world problems and design efficient and effective solutions. These applications underscore the practical significance and relevance of line integrals in various fields.