Mastering Fraction Division With Like Denominators: Essential For Real-Life Applications And Accuracy
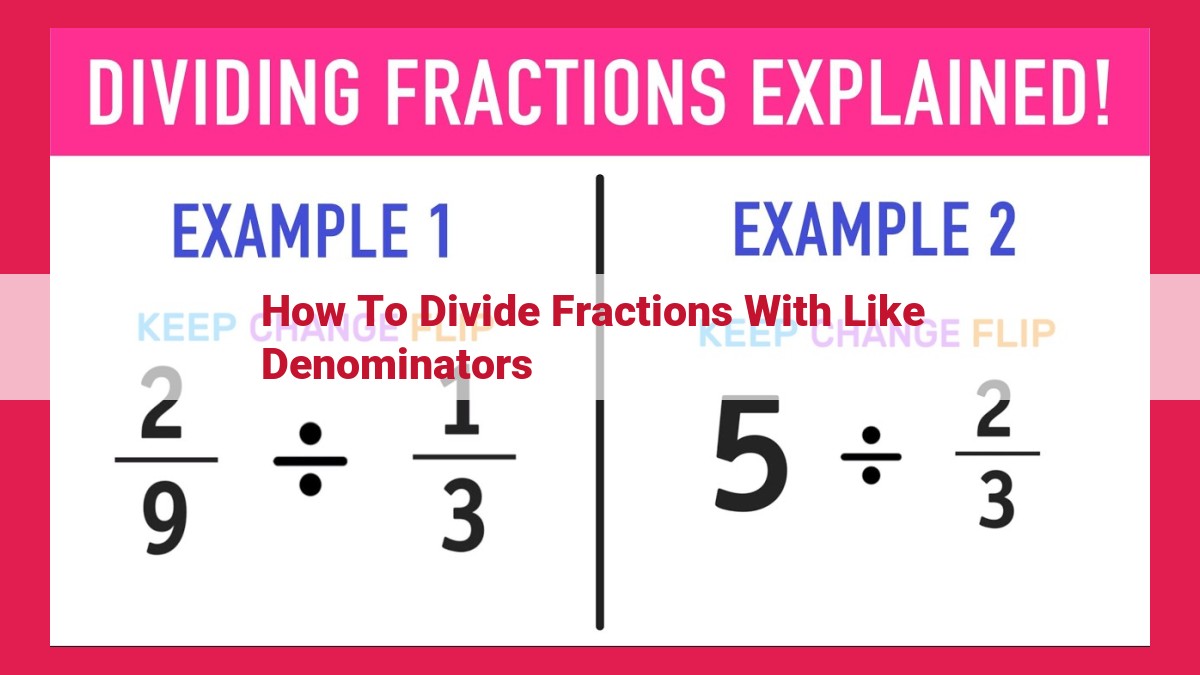
Dividing fractions with like denominators is crucial in everyday scenarios, from recipe adjustments to home improvement tasks. To divide fractions with the same denominators, flip the second fraction (making the numerator the denominator and vice versa) and multiply the fractions. The new numerator is the product of the first fraction’s numerator and the reciprocal’s numerator, while the new denominator is the product of the first fraction’s denominator and the reciprocal’s denominator. Simplifying the resulting fraction to its lowest terms is recommended for clarity and accuracy.
Fraction Division: A Practical Guide to Mastering Daily Math Challenges
In the tapestry of our daily lives, fractions are an intrinsic thread, weaving their way into countless scenarios that demand our understanding. From dividing a pizza among friends to calculating the discount on a purchase, fraction division empowers us to navigate these situations with confidence.
Imagine yourself at a family gathering, tasked with slicing a pizza for everyone. You want to ensure that each slice is of equal size, so you need to divide the pizza’s total area into fractions representing the number of people present. This is where fraction division comes into play.
Similarly, when shopping for groceries, you may encounter discounts expressed as fractions. To determine how much you save, you need to divide the discount fraction by the original price, allowing you to calculate the discounted amount with ease. These practical examples underscore the significance of fraction division in everyday life.
Understanding Fraction Division: Delving into Division with Like Denominators
In our daily encounters, fractions play a significant role, be it in measuring ingredients for a delectable dish, estimating distances on a map, or calculating the distribution of a dividend among shareholders. Among the fundamental operations involving fractions, division holds a crucial place. Let’s embark on a journey to explore the world of fraction division, specifically focusing on the case when fractions share identical denominators.
Fraction Division with Like Denominators
When dividing fractions with like denominators, we encounter a straightforward process that unravels the intricacies of this operation. The key lies in understanding the concept of a reciprocal. A reciprocal of a fraction simply involves inverting it, which means swapping its numerator and denominator. This reciprocal plays a pivotal role in the division of fractions.
Step-by-Step Division
To divide fractions with like denominators, we follow a simple two-step process:
-
Flip the Second Fraction: Convert the second fraction into its reciprocal by inverting its numerator and denominator.
-
Multiply Fractions: Multiply the first fraction by the reciprocal of the second fraction. The resulting fraction represents the quotient of our division.
Example
Let’s consider an example to illustrate this concept. Suppose we want to divide 3/4 by 1/2.
-
Flip the Second Fraction: The reciprocal of 1/2 is 2/1.
-
Multiply Fractions: Multiplying 3/4 by 2/1 gives us (3/4) * (2/1) = 6/4.
Simplifying the result, we get 6/4 = 3/2. Therefore, 3/4 divided by 1/2 equals 3/2.
Significance of Reciprocals
Reciprocals are essential in fraction division because they transform the operation into multiplication. Instead of performing division, we simply flip the second fraction and multiply it by the first. This transformation simplifies the process and enhances our understanding of fraction division.
In conclusion, mastering fraction division with like denominators empowers us with a valuable tool for solving a myriad of problems that arise in our daily lives. By embracing the concept of reciprocals and following the simple two-step process, we can confidently navigate the world of fraction division, unlocking its secrets and unraveling its practical applications.
Step 1: Flipping the Second Fraction: The Reciprocal’s Role
In the world of fraction division, there’s a magic trick that makes everything easier: the reciprocal. Imagine you’re at a bakery, and you have a luscious chocolate cake. If you wanted to divide it equally between you and your best friend, you’d simply cut it into two pieces.
Now, what if you had a delicious pizza? It’s still a circle, but instead of cutting it into equal parts, you’d divide it into slices. Think of the reciprocal as the pizza cutter that transforms a fraction into its pizza slice version.
For instance, let’s say your recipe calls for 1/2 cup of flour. But you only have 1/4 cup. Fear not! The reciprocal of 1/4 is 4/1, which is like that amazing pizza cutter. When you flip and multiply 1/2 by 4/1, you get the equivalent of four 1/2 cups, or 2 cups – just what you need!
So, in fraction division, when you have 1/2 divided by 1/4, you flip that second fraction and turn it into 4/1. This magical transformation is the first step towards dividing fractions with like denominators like a pro.
Step 2: Multiplying Fractions
Now, it’s time for the multiplication magic! In this step, we’re going to take the first fraction and multiply both its numerator and denominator by the reciprocal of the second fraction. Remember, the reciprocal is that funny name we gave to the second fraction after we flipped it.
This multiplication process is just like multiplying any other fraction, but with an extra twist. We’re going to use the “Numerator-by-Numerator, Denominator-by-Denominator” rule. It’s like a dance party where the numerators and denominators pair up and multiply each other.
For example, let’s say we’re dividing 2/3 by 1/4:
(2/3) ÷ (1/4)
First, we flip the second fraction to get its reciprocal:
(2/3) ÷ (4/1)
Now, we multiply the first fraction by the reciprocal:
(2/3) * (4/1)
Using our dance rule, we multiply the numerators (2 by 4) and the denominators (3 by 1):
(2 * 4) / (3 * 1)
And voila! We have our answer:
8/3
Don’t worry if you don’t get it right away. Just keep practicing and you’ll be a fraction division pro in no time.
Example of Dividing Fractions with Like Denominators: A Step-by-Step Guide
In the realm of fractions, division plays a pivotal role in deciphering a myriad of everyday scenarios. Whether it’s splitting a pizza among friends or calculating recipe ingredients, the ability to divide fractions with like denominators is an essential skill.
Let’s embark on a journey through the simple steps involved in dividing fractions with common denominators:
-
Meet the **Flipped Fraction: The secret to fraction division lies in flipping the second fraction upside down, essentially finding its reciprocal. A reciprocal is a fraction where the numerator and denominator have swapped places. For instance, the reciprocal of 2/3 would be 3/2.
-
Multiplication Magic: Once you have your flipped fraction, it’s time for multiplication. Multiply the numerator of the first fraction by the numerator of the flipped second fraction. Next, multiply the denominator of the first fraction by the denominator of the flipped second fraction.
-
The Grand Finale: The result of this magical multiplication is your answer. Remember to simplify your final answer by reducing any fractions to their lowest terms.
Now, let’s dive into an example to make it crystal clear:
Suppose you want to divide 3/4 by 1/2. Following our steps, we’ll first flip 1/2 to get 2/1. Then, we’ll multiply:
Numerators: 3 x 2 = 6
Denominators: 4 x 1 = 4
So, 3/4 divided by 1/2 equals 6/4. Simplifying this fraction, we get our final answer: 3/2.
The Reciprocal: A Fraction’s Flip Side
When we embark on the adventure of dividing fractions, we encounter a magical concept known as the reciprocal. Picture yourself on a seesaw, with two fractions balancing each other. The reciprocal of a fraction is like flipping one side of the seesaw to the other.
Let’s take the fraction 1/2 as an example. Its reciprocal is 2/1. By turning the fraction upside down, we create a flipped version of itself. This means that multiplying a fraction by its reciprocal is like multiplying the seasaw by two, making it perfectly balanced.
The reciprocal plays a crucial role in fraction division. When we divide fractions, we essentially flip the second fraction (the divisor) and multiply. This is akin to turning the seesaw so that the divisor ends up on the bottom, allowing us to calculate the result.
For instance, dividing 1/2 by 1/4 is the same as multiplying 1/2 by 4/1. This operation flips 1/4 and multiplies it with 1/2, resulting in the answer 2/1, which simplifies to 2. Thus, the reciprocal allows us to perform fraction division using the familiar concept of multiplication.
The Power of Fraction Division: A Guide to Simplifying Everyday Calculations
In the realm of mathematics, fraction division holds a pivotal role in unraveling the complexities of everyday life. From culinary adventures to financial dilemmas, this mathematical operation empowers us to make sense of the world around us.
One particular type of fraction division that warrants attention is the division of fractions with like denominators. This concept, though deceptively simple, forms the cornerstone of numerous calculations that shape our daily experiences.
Step 1: The Magic of Flipping
When faced with the task of dividing two fractions with identical denominators, the first step involves a clever trick known as reciprocity. Simply put, reciprocals are two numbers that, when multiplied together, yield the value of 1.
To divide Fraction A by Fraction B, we begin by flipping Fraction B. This means inverting its numerator and denominator. For instance, if Fraction B is 3/4, its reciprocal becomes 4/3.
Step 2: The Multiplication Phase
With Fraction B successfully flipped, it’s time for the multiplication dance. We multiply the numerator of Fraction A by the numerator of Fraction B’s reciprocal, and likewise for the denominators.
Step 3: Unveiling the Simplified Fraction
The result of our multiplication maneuver is a brand-new fraction. However, this fraction may not be in its simplest form. To rectify this, we embark on a quest for common factors.
Common factors are numbers that divide evenly into both the numerator and denominator of a fraction. By identifying and dividing both the numerator and denominator by these common factors, we can simplify the fraction to its purest form.
Example: Putting It All Together
Let’s put this knowledge to the test with an example. Suppose we want to know how many 1/4 cups of flour we need to make 3/2 cups of dough.
- Flip Fraction B (3/2): 2/3
- Multiply Fraction A (1/4) by the flipped Fraction B (2/3): 2/12
- Simplify: 2/12 ÷ 2 ÷ 3 = 1/6
Our calculation reveals that we need 1/6 cups of flour for this recipe.
Additional Tips and Tricks
To make fraction division even more effortless, consider these tricks:
- Cross-cancellation: When possible, identify and cancel common factors in the numerators and denominators before multiplying.
- Mental math strategies: For simple fractions, use mental math shortcuts to avoid complex calculations.
Mastering fraction division with like denominators unlocks a world of possibilities. Whether you’re planning a meal, calculating expenses, or solving mathematical puzzles, this fundamental operation will guide you towards accurate and efficient solutions. Remember, simplifying fractions is the key to unlocking the true power of this mathematical tool.
Dive into the World of Fraction Division with Like Denominators
In the realm of our daily lives, fractions often play a crucial role. From baking a cake to splitting a pizza, understanding how to divide fractions with the same denominators is a valuable skill. Let’s embark on a fraction-dividing adventure and explore this essential mathematical concept.
Defining Fraction Division with Like Denominators
When you divide fractions with like denominators, you’re essentially finding a fraction that represents the quotient of the two original fractions. Imagine you have two pizzas, each with 12 slices. If you want to share one pizza equally among 4 friends, you need to find the fraction of each pizza that represents one friend’s share.
Step-by-Step Guide to Fraction Division
Step 1: Flipping the Second Fraction
The secret to dividing fractions with like denominators lies in the concept of a reciprocal. A reciprocal is a fraction where the numerator and denominator are swapped. So, if you have a fraction like 1/2, its reciprocal would be 2/1.
Step 2: Multiplying Fractions
Once you have the reciprocal of the second fraction, you simply multiply the two fractions. To do this, multiply the numerators and multiply the denominators. In our pizza example, you would multiply 1/4 by 2/1.
Example: Divide 1/4 by 2/1
- Flip the second fraction: 2/1 becomes 1/2
- Multiply the fractions: 1/4 x 1/2 = 1/8
So, each friend gets 1/8 of the pizza. Easy as pie!
Additional Tips and Tricks
- Cross-Cancellation: If the numerators or denominators of the two fractions have common factors, you can reduce them before multiplying. This makes the calculation much simpler.
- Mental Math Magic: For simple fractions, you can use mental math tricks to divide them. For instance, 1/2 divided by 1/4 is simply 2.
Dividing fractions with like denominators is a breeze once you understand the concept of a reciprocal and the simple multiplication process. Remember, this skill is not just limited to academic math but also has practical applications in everyday life. So, the next time you’re sharing snacks or measuring ingredients, feel confident in your fraction-dividing abilities!
Additional Tips and Tricks:
- Explain the technique of cross-cancellation to simplify calculations.
- Introduce mental math strategies for efficient fraction division.
Fraction Division with Like Denominators: A Step-by-Step Guide
In everyday life, we encounter numerous situations where we need to divide fractions. From mixing ingredients for a cake to calculating travel time, understanding fraction division is crucial.
Defining Fraction Division with Like Denominators
Dividing fractions with like denominators involves breaking down the problem into simple steps. When the denominators of the fractions are the same, the process becomes much easier.
Step 1: Flipping the Second Fraction
The trick to dividing fractions with like denominators is to flip the second fraction. This means inverting the numerator and denominator. The result is called the reciprocal.
Step 2: Multiplying Fractions
Next, we multiply the first fraction by the reciprocal of the second fraction. This is the same as multiplying the numerators and denominators of the first fraction by the inverted second fraction.
Example of Dividing Fractions with Like Denominators
Let’s say we want to divide 1/4 by 1/2.
- Flip the second fraction: 1/2 becomes 2/1
- Multiply the fractions: (1/4) * (2/1) = 1 * 2 / 4 * 1 = 2/4
Simplifying the Result
The fraction 2/4 can be simplified to 1/2 by dividing both numerator and denominator by 2.
Additional Tips and Tricks
- Cross-cancellation: This technique allows you to cancel common factors between the numerators and denominators of the fractions before multiplying.
- Mental math: For simple fractions, you can use mental math strategies to divide them in your head. For instance, dividing 1/4 by 1/2 is the same as multiplying 1/4 by 2, which gives you 2/4 or 1/2.
Understanding how to divide fractions with like denominators is essential for various practical situations. By following these steps and using additional tricks, you can confidently perform these calculations and apply them in your daily life. Remember, practice makes perfect, so don’t hesitate to challenge yourself with different fraction division problems to enhance your skills.