Mastering Differential Equations: A Comprehensive Guide To General Solutions

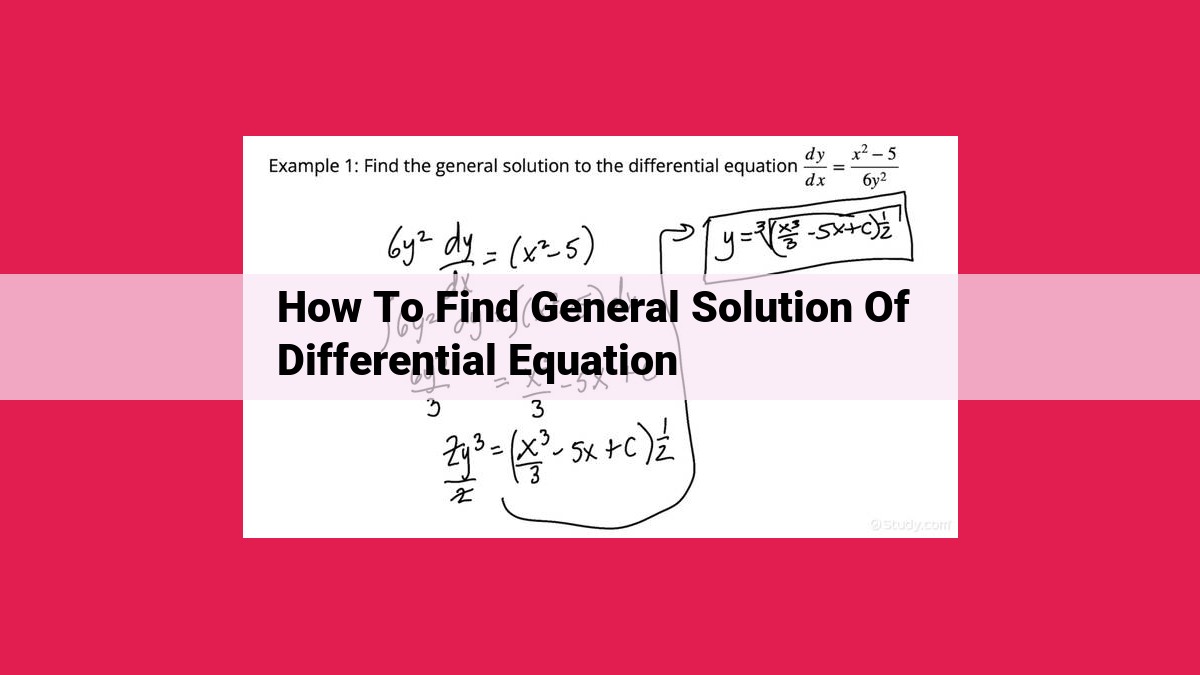
To find the general solution of a differential equation, first identify its type (homogeneous or nonhomogeneous). For homogeneous equations, solve the characteristic equation to find the roots and write the general solution. For nonhomogeneous equations, find a particular solution and combine it with the general solution of the homogeneous equation. Linearity allows for superposition, which simplifies solving systems of linear differential equations. Finally, use initial or boundary conditions to determine the particular solution that fits the specific problem.

Differential Equations: A Guide to the Fundamentals

In the realm of mathematics, differential equations hold a pivotal position. They are mathematical equations that describe how a dependent variable varies with respect to one or more independent variables. These equations are ubiquitous in our world, playing a crucial role in modeling a vast array of real-world phenomena, from the growth of bacteria to the vibrations of guitar strings.

Types of Differential Equations

Differential equations are characterized by their order, degree, linearity, and homogeneity.

• Order: The order of a differential equation refers to the highest derivative of the dependent variable that appears in the equation.

• Degree: The degree of a differential equation is the highest power to which the dependent variable or its derivatives are raised.

• Linearity: A differential equation is considered linear if the dependent variable and its derivatives appear in a linear form (e.g., as a sum of terms multiplied by constants).

• Homogeneity: Homogeneity refers to whether the differential equation has terms involving only the dependent variable or terms that also involve the independent variables.

Navigating the Maze of Homogeneous Differential Equations

In the realm of differential equations, homogeneous equations stand apart as a class of mathematical enigmas that can both captivate and perplex. They present a puzzle that demands a systematic and nuanced approach to unravel their intricate secrets. In this article, we embark on an adventure to unmask the mysteries of homogeneous differential equations, step by step.

Method of Separation of Variables: A Path to Simplicity

The method of separation of variables is a clever technique that allows us to transform a seemingly daunting differential equation into a pair of more manageable first-order equations. The key lies in identifying variables that can be separated and rewriting the equation in terms of these variables. By doing so, we create a path towards obtaining a solution.

Unveiling the Characteristic Equation and Its Roots

Once the equation is separated, we encounter the characteristic equation, a quadratic equation that holds the key to understanding the equation’s behavior. By finding the roots of this equation, we uncover essential information about the nature of the solutions.

Crafting the General Solution: A Symphony of Roots

Armed with the roots of the characteristic equation, we can construct the general solution to the homogeneous differential equation. This solution is a harmonious blend of exponential or trigonometric functions, each corresponding to a different root. By combining these functions, we capture the essence of all possible solutions.

In conclusion, solving homogeneous differential equations requires a combination of mathematical intuition, careful manipulation, and the artistry of combining solutions. By mastering these techniques, we gain the power to navigate the maze of differential equations and unlock their hidden truths. So, let us embrace the challenge and embark on this mathematical journey, one step at a time.

Solving Nonhomogeneous Differential Equations

In the world of differential equations, solving nonhomogeneous equations is like conquering a challenging puzzle. One method, the undetermined coefficients, works like a charm for certain nonhomogeneous terms. We simply guess a particular solution that matches the form of the nonhomogeneous term, and then determine its coefficients. It’s like solving a matching game, where the coefficients are the pieces that fit the nonhomogeneous term.

But what if the nonhomogeneous term doesn’t fit our pre-chosen forms? That’s where variation of parameters swoops in. This technique is a bit more intricate, but it’s like having a toolbox of solutions to choose from. By cleverly modifying solutions to the homogeneous equation, we can construct a particular solution that fits any nonhomogeneous term.

This is where the true magic of differential equations unfolds. These methods allow us to solve a vast array of equations that describe real-world phenomena. From the oscillation of springs to the radioactive decay of atoms, differential equations help us understand the dynamics of our universe.

Unveiling the Power of Linear Differential Equations

In the intricate world of mathematics, differential equations hold a captivating allure, unlocking insights into a vast array of real-world phenomena. Among these equations, linear differential equations stand out as a cornerstone of scientific inquiry, owing to their notable properties and wide-reaching applications.

Linearity and Superposition: A Symphony of Solutions

Linearity grants differential equations an exceptional characteristic: superposition. This principle asserts that any linear combination of solutions to a linear differential equation is also a valid solution. In other words, if you have two solutions, say y₁ and y₂, you can effortlessly concoct a new solution: cy₁ + dy₂, where c and d are constants. This remarkable property greatly simplifies the process of finding solutions.

Systems: A Tangled Web of Equations

A system of linear differential equations presents a more complex challenge, wherein several equations are tangled together, each governing the behavior of a distinct unknown function. Yet, linearity once again lends a helping hand. By skillfully expressing the system in matrix form, you can often leverage powerful techniques like Gaussian elimination to systematically unravel the knotted equations and unveil their solutions.

Linear differential equations, with their linearity and superposition properties, serve as indispensable tools in the mathematical toolbox. They gracefully describe a myriad of physical phenomena, from the rhythmic oscillations of pendulums to the intricate dynamics of electrical circuits. Their versatility has propelled their use across a vast spectrum of scientific disciplines, solidifying their reputation as cornerstones of mathematical modeling. Through their ability to untangle complex systems, linear differential equations empower us to gain profound insights into the workings of our intricate world.

Initial-Value and Boundary-Value Problems

• Definition and difference between initial and boundary conditions
• Using initial or boundary conditions to determine the particular solution

Initial-Value and Boundary-Value Problems

In the world of differential equations, not all solutions are created equal. Sometimes, we’re not just interested in the general solution to an equation but also in specific solutions that satisfy certain conditions. This is where initial-value problems and boundary-value problems come into play.

Initial-Value Problems (IVPs)

Let’s start with initial-value problems. Imagine you have a differential equation that describes the growth of a population. The equation might tell you how the rate of growth changes over time. But what you really want to know is the population at a specific point in time, say, the present.

This is where initial conditions come in. They’re like the initial size of the population. By setting the solution of the differential equation equal to the initial condition, we can pin down the solution to a specific value.

Boundary-Value Problems (BVPs)

Boundary-value problems, on the other hand, are a bit different. Let’s say you have a differential equation that describes the temperature of a metal rod. The equation tells you how the temperature changes along the rod. But what you’re interested in is the temperature at the ends of the rod.

This is where boundary conditions come in. They’re like the temperatures at the ends of the rod. By setting the solution of the differential equation equal to the boundary conditions, we can find the specific solution that satisfies those conditions.

Using Conditions to Find Solutions

Both IVPs and BVPs give us extra information about the solution that we can use to narrow down our search. By applying the conditions to the general solution of the differential equation, we can determine the values of the constants that make the solution satisfy those conditions.

This process helps us find the particular solution that meets our specific requirements. It’s like putting together a puzzle, where the initial or boundary conditions give us the missing pieces to complete the picture.

Step-by-Step Guide to Finding the General Solution of Differential Equations

In the vast realm of mathematics, differential equations hold a special place. They describe how rates of change govern the behavior of functions, unlocking insights into phenomena from population growth to complex vibrations. To delve into their mysteries, let’s embark on a step-by-step journey to find their general solutions.

1. Identifying the Differential Equation

As we encounter a differential equation, our first task is to classify it based on its order, degree, linearity, and homogeneity. These characteristics determine the techniques we’ll employ to conquer it.

2. Solving the Homogeneous Equation

The homogeneous equation arises when the nonhomogeneous term is absent. Its solution typically involves the separation of variables or finding the characteristic equation and its roots. This step yields the complementary solution, a fundamental building block for our general solution.

3. Finding a Particular Solution

Next, we tackle the nonhomogeneous equation. Here, we seek a particular solution that satisfies the full equation. Depending on the nonhomogeneous term, we might use the method of undetermined coefficients or variation of parameters. This solution captures the unique impact of the external force driving our differential equation.

4. Combining Solutions

Finally, we combine the complementary solution and the particular solution to obtain the general solution. This powerful formula represents the collection of all possible solutions to our differential equation.

Step-by-Step Example

Imagine we have the differential equation:

y'' + 3y' + 2y = x²

1. Identifying: This is a second-order, constant coefficient, linear, and nonhomogeneous differential equation.

2. Solving Homogeneous Equation: Separation of variables gives the complementary solution:

   y_c = Ae^(-x) + Be^(-2x)
   

3. Finding Particular Solution: Method of undetermined coefficients yields:

   y_p = Cx² + Dx + E
   

4. Combining Solutions: The general solution is:

   y = y_c + y_p = Ae^(-x) + Be^(-2x) + Cx² + Dx + E
   

By following this step-by-step process, we’ve uncovered the general solution to our differential equation, empowering us to understand and predict the behavior of complex systems.

Applications of Differential Equations

In the realm of mathematics, differential equations hold a pivotal role in unraveling the complexities of our physical world. They provide a powerful language to model intricate phenomena, empowering us to understand and predict a wide array of real-world processes.

From the rhythmic vibrations of a guitar string to the exponential growth of a bacterial colony, differential equations lie at the heart of countless natural occurrences. Engineers employ them to design efficient structures and optimize fluid flow. Physicists rely on them to probe the mysteries of particle dynamics and quantum mechanics. Biologists leverage them to investigate the intricate workings of living organisms.

Beyond these core disciplines, differential equations find applications in an astonishingly diverse array of fields. Economists utilize them to model market behavior, while sociologists apply them to understand population dynamics. Chemists employ them to unravel the mechanisms of chemical reactions, and ecologists use them to predict the evolution of ecosystems.

The versatility and power of differential equations extend far beyond theoretical domains. They serve as the backbone of technological advancements, enabling us to develop lifesaving medical devices, design cutting-edge communication systems, and explore the depths of outer space.

By harnessing the power of differential equations, we gain a deeper understanding of the natural world and our place within it. They empower us to make informed decisions, solve complex problems, and drive scientific progress. As we delve deeper into the intricate workings of our universe, differential equations will undoubtedly continue to play an indispensable role in shaping our knowledge and shaping our future.