Mastering Decimal Subtraction: Step-By-Step Guide For Accurate Results

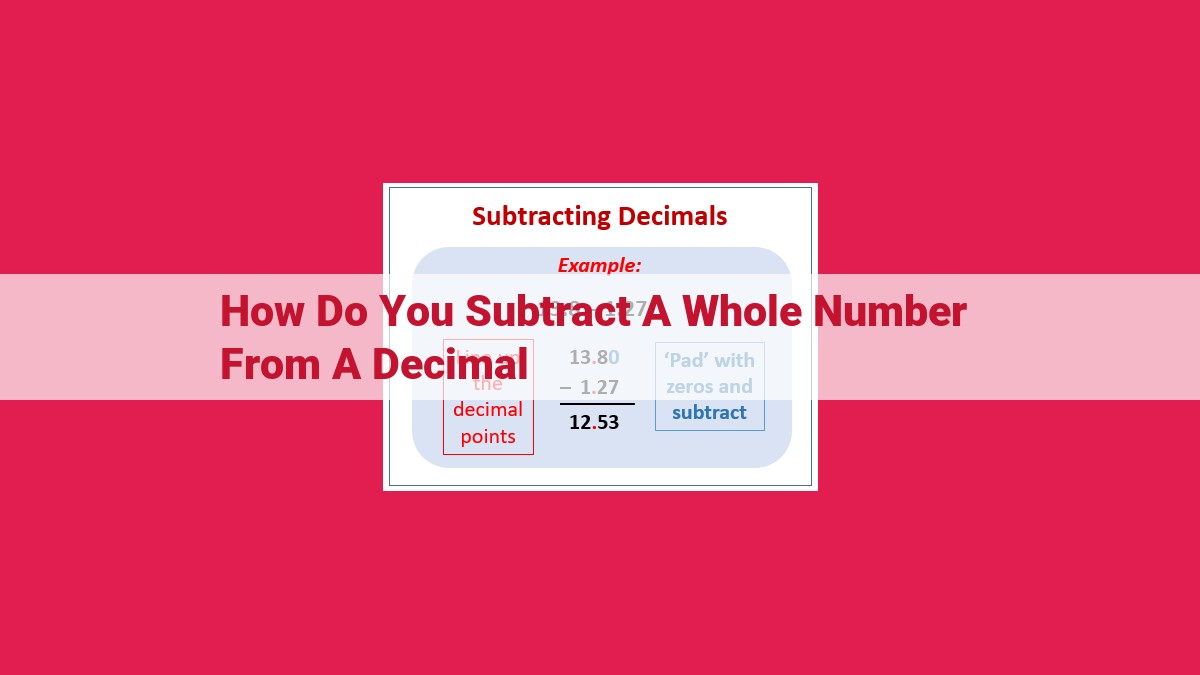
When subtracting a whole number from a decimal, it’s crucial to align the decimal points vertically. If the subtrahend (whole number) has fewer decimal places, add zeros to match the minuend (decimal). Subtract digits column by column, starting from the rightmost column. If the subtrahend digit exceeds the minuend digit, borrow 1 from the next minuend column and subtract it. Finally, bring down any remaining minuend digits after subtracting all digits to complete the result.

Decimal Alignment: The Foundation for Successful Subtraction

When embarking on the mathematical journey of subtracting a whole number from a decimal, the path to precision lies in the alignment of decimal points. This meticulous arrangement ensures the accuracy of your calculations and leads you to a confident grasp of this numerical operation.

Imagine two numbers, a minuend (the number being subtracted from) and a subtrahend (the number being subtracted). To subtract successfully, we align their decimal points vertically. This alignment ensures that numbers of the same value are subtracted from each other, regardless of their placement before or after the decimal point.

In the case of the subtrahend having fewer decimal places than the minuend, we extend it by adding zeros. These zeros act as placeholders, ensuring that the digits are subtracted in the correct order. By aligning the decimal points and adding zeros when necessary, we lay the firm foundation for error-free subtraction.

Column Subtraction: Breaking Down the Process

When it comes to subtraction, the rightmost column holds a special significance. It’s the starting point of our journey, where we begin the process of subtracting digits column by column.

Rightmost Column: Setting the Stage

Let’s say we’re subtracting 15.34 from 22.11. We’ll start with the rightmost column, where we have 4 and 1. If the digit in the subtrahend (1) is less than the digit in the minuend (4), it’s smooth sailing. We simply subtract 1 from 4 to get 3.

Borrowing: When the Going Gets Tough

But what if the digit in the subtrahend (9) is greater than the digit in the minuend (4)? This is where the concept of borrowing comes into play. We “borrow” 1 from the next column (the tenths column) and add it to the minuend digit. So, 4 becomes 14, and we can now subtract 9 from 14 to get 5.

Step-by-Step Column Subtraction

We continue this process column by column, moving from right to left, until we reach the end. With each step, we subtract the digits, being mindful of any borrowing that may arise. It’s like a mathematical treasure hunt, where we uncover the hidden differences between numbers.

Bringing Down the Remaining Digits

Once we’ve subtracted all the digits, it’s time to bring down any remaining digits in the minuend column. These digits represent the remainder of the subtraction, completing our final result.

In our example, after subtracting the rightmost columns, we have 22 – 15 = 7. We bring down the remaining 1 to get our final answer: 7.11.

So, there you have it! Column subtraction is all about breaking down a problem into smaller, manageable steps. Start with the rightmost column, handle any borrowing, and bring down the remaining digits to reach your final destination – the correct difference.

Borrowing: When the Subtrahend Exceeds the Minuend

In the realm of subtraction, we often encounter scenarios where the digit in the subtrahend (the number we’re subtracting) is greater than the digit in the minuend (the number we’re subtracting from). This is when the concept of “borrowing” comes into play.

Imagine this: You have a stack of 10 blocks, and you want to remove 7 blocks. If you try to take 7 blocks directly from the stack, you’ll end up with a deficit of 3 blocks.

To resolve this, you borrow a block from the next stack, which gives you a total of 10 + 1 = 11 blocks. Now, you can subtract the 7 blocks, leaving you with 4 blocks.

This is analogous to what we do when we subtract in math. When the digit in the subtrahend is greater than the digit in the minuend, we need to “borrow” a 1 from the next digit in the minuend.

Let’s say we want to subtract 5 from 12. Since 5 is greater than 2, we borrow a 1 from the 1 in 12. This gives us 12 + 1 = 13.

Now, we can subtract the 5 from the 13, resulting in 8. The borrowed 1 is then subtracted from the digit in the minuend column beneath the 5, which is 1. This gives us 0.

The final result is 13 – 5 = 80. You might say, “Hey, it’s a six!” And you’re right! But the 0 in the tenths place represents the borrowed 1 that we returned to the minuend.

Bringing Down Remaining Digits: Completing the Puzzle

• Discuss the importance of bringing down any remaining digits in the minuend column after subtracting all the digits.
• Explain that these digits represent the remainder of the subtraction and complete the final result.

Bringing Down Remaining Digits: Completing the Puzzle in Decimal Subtraction

When subtracting decimals, it’s crucial to ensure proper alignment to avoid errors. After aligning the decimals carefully, we embark on a step-by-step subtraction journey, starting from the rightmost column. Each column presents its own unique challenge, but what happens when we’ve subtracted all the digits?

Enter the remaining digits in the minuend column. These unsung heroes hold the key to completing the puzzle and revealing the final result. Imagine you’re searching for a hidden treasure. The first clue led you to a chest, but it’s locked with a puzzle that requires solving the decimal subtraction. Every digit you subtract is a step towards the solution, but it’s only when you bring down the remaining digits that you can unlock the chest.

These remaining digits are the remainder of the subtraction, orphaned digits that crave inclusion in the final answer. By bringing them down to join the subtracted digits, you’re completing the puzzle, revealing the treasure that lies within. It’s a moment of triumph, where the journey of subtraction culminates in a satisfying result.