Mastering Convergent Series Summation: A Comprehensive Guide

To find the sum of a convergent series, various methods can be used. Telescoping series involve canceling terms to simplify the sum, while p-series and geometric series have specific convergence properties and formulas. Alternating series alternate signs and have a controlled error bound. Comparison, ratio, and root tests analyze the terms of the series to determine convergence. The integral test converts the series into an integral to assess convergence. These methods provide a systematic approach to finding the sum of convergent series, with applications ranging from mathematics to science and engineering.

• Define a convergent series and its properties.
• Explain the importance and applications of convergent series.

Convergent Series: A Journey into the Realm of Infinite Sums

In the realm of mathematics, where numbers dance and equations ignite curiosity, we encounter the fascinating concept of convergent series. Convergent series, like a never-ending story, consist of an infinite sequence of numbers whose sum approaches a finite value as more terms are added.

Why are convergent series so important? They hold the key to unlocking complex problems in mathematics, physics, engineering, and beyond. From representing the behavior of light to calculating the probability of events, convergent series are essential tools in understanding our world.

Take a sequence for instance, a list of numbers that march in order. Convergence is like the destination of a sequence, the value it approaches as it continues its journey. When a series, the sum of a sequence, converges, it provides a way to represent infinite processes with a single finite number.

In this magical realm of convergent series, we uncover a treasure trove of methods for determining convergence and calculating sums. From the telescoping series, where terms cancel each other out, to the geometric series, with its ever-decreasing terms, each method offers a unique key to unlocking the secrets of these infinite sums.

Whether it’s the alternating series, where positive and negative terms take turns, or the p-series, which explores the limits of infinite fractions, each type of series unveils its own set of fascinating properties.

The comparison test, ratio test, and root test become our trusted guides along this mathematical expedition. They help us compare series to known convergent or divergent patterns, providing a roadmap to success.

And finally, the integral test, like a mathematician’s microscope, allows us to use continuous functions to probe the nature of series, revealing their convergence or divergence with exquisite precision.

As we delve deeper into the world of convergent series, we discover a treasure chest of applications. They unlock the secrets of power series, enabling us to represent functions as infinite polynomials. In probability, they allow us to calculate the likelihood of events, shaping our understanding of chance and uncertainty.

Convergent series, like the threads in a tapestry, weave together the fabric of mathematical knowledge. Their ability to represent infinite processes with finite values makes them indispensable tools in unraveling the mysteries of our universe. So, let us embark on this mathematical adventure, where convergence guides our steps and the sum of infinite worlds awaits our discovery.

Sequences and Convergence

• Explain the concept of a sequence and its limit.
• Relate convergence of a sequence to the sum of a series.

Sequences and Convergence: The Path to Summing Up

In the realm of mathematics, exploring the behavior of sequences and their convergence holds the key to understanding the sum of seemingly infinite series. Sequences, simply put, are ordered lists of numbers that follow a certain pattern. Each number in a sequence is called a term.

The limit of a sequence is a value that the terms of the sequence approach as the number of terms increases without bound. Convergence, in this context, means that the sequence has a limit. A convergent sequence is one whose terms eventually get arbitrarily close to the limit.

The convergence of a sequence plays a crucial role in determining whether the sum of a series exists. A series is an expression that represents the sum of an infinite number of terms. If the sequence of partial sums of a series converges, then the series itself is said to be convergent.

In essence, the convergence of a sequence provides a foundation for understanding the sum of a series. By studying the behavior of individual terms in a sequence, we can unravel the nature of the series’s sum. This concept forms the bedrock of many mathematical applications, enabling us to calculate sums that would otherwise seem impossible.

Telescoping Series

• Define a telescoping series and its characteristics.
• Show how to evaluate its sum using partial sums and telescoping sums.

Telescoping Series: A Simple Guide to Unraveling Complex Sums

In the realm of convergent series, telescoping series stand out as a unique breed. They possess a remarkable characteristic—their terms cancel out in pairs, leaving behind a simple sum to evaluate.

Defining Telescoping Series

A telescoping series is a series where each term, after the first, can be expressed as the difference between consecutive terms of a sequence. In other words, it takes the form:

a_1 + (a_2 - a_1) + (a_3 - a_2) + (a_4 - a_3) + ...

Unraveling the Sum

The magic of telescoping series lies in their ability to simplify the evaluation of their sum. By grouping the terms together, we can see that the initial terms cancel out, leaving us with the following:

a_1 + (a_2 - a_1) + (a_3 - a_2) + (a_4 - a_3) + ... = a_n - a_1

Example: A Telescoping Sum

Consider the following telescoping series:

1 - 1/2 + 1/2 - 1/4 + 1/4 - 1/8 + ...

Grouping the terms, we get:

(1 - 1/2) + (1/2 - 1/4) + (1/4 - 1/8) + ... = 1 - 0

Thus, the sum of this telescoping series is simply 1.

Applications in Mathematics and Beyond

Telescoping series find wide application in mathematics and its various branches, including:

• Calculus: Evaluating integrals and limit sums
• Number Theory: Proving properties of integers and sequences
• Physics: Solving differential equations in mechanics and electromagnetism

Unveiling the sum of a telescoping series is a straightforward process that involves pairing up and canceling terms. By understanding this technique, we unlock a powerful tool for solving complex sums efficiently. Whether in the realms of mathematics or real-world applications, telescoping series continue to play a vital role in unraveling intricate problems.

p-Series

• Introduce the p-series and its convergence properties.
• Describe its relationship with the harmonic series.
• Introduce the Zeta function as a generalization.

The Convergence of **p-Series: A Journey from Finite to Infinite**

In the realm of mathematics, series play a pivotal role in representing the sum of an infinite number of terms. Among these, the p-series stands as a fascinating subject, inviting us on an intellectual expedition to explore its convergence properties and remarkable connections.

The p-series is a special type of series defined as the sum of the reciprocals of positive integers raised to a power p:

∑[n=1 to ∞] 1/n^p

The convergence or divergence of this series depends on the value of p. If p > 1, the series converges, meaning its sum approaches a finite value as the number of terms increases. However, if p ≤ 1, the series diverges, meaning its sum does not converge to a finite value.

Relationship with the Harmonic Series

The p-series has a particularly intriguing relationship with the harmonic series, a series formed by the sum of the reciprocals of positive integers:

∑[n=1 to ∞] 1/n

The harmonic series is an example of a divergent series, meaning its sum tends towards infinity as the number of terms increases. Interestingly, the p-series converges for values of p > 1 and diverges for p ≤ 1, providing a contrasting insight into the behavior of series.

Introducing the Zeta Function

The p-series also serves as a stepping stone towards understanding the Zeta function, a generalization that extends the concept of the p-series to complex values of p. The Zeta function, denoted as ζ(p), is defined as:

ζ(p) = ∑[n=1 to ∞] 1/n^p

The Zeta function has profound applications in various fields, including number theory, physics, and computer science, making the p-series a gateway to a wider mathematical landscape.

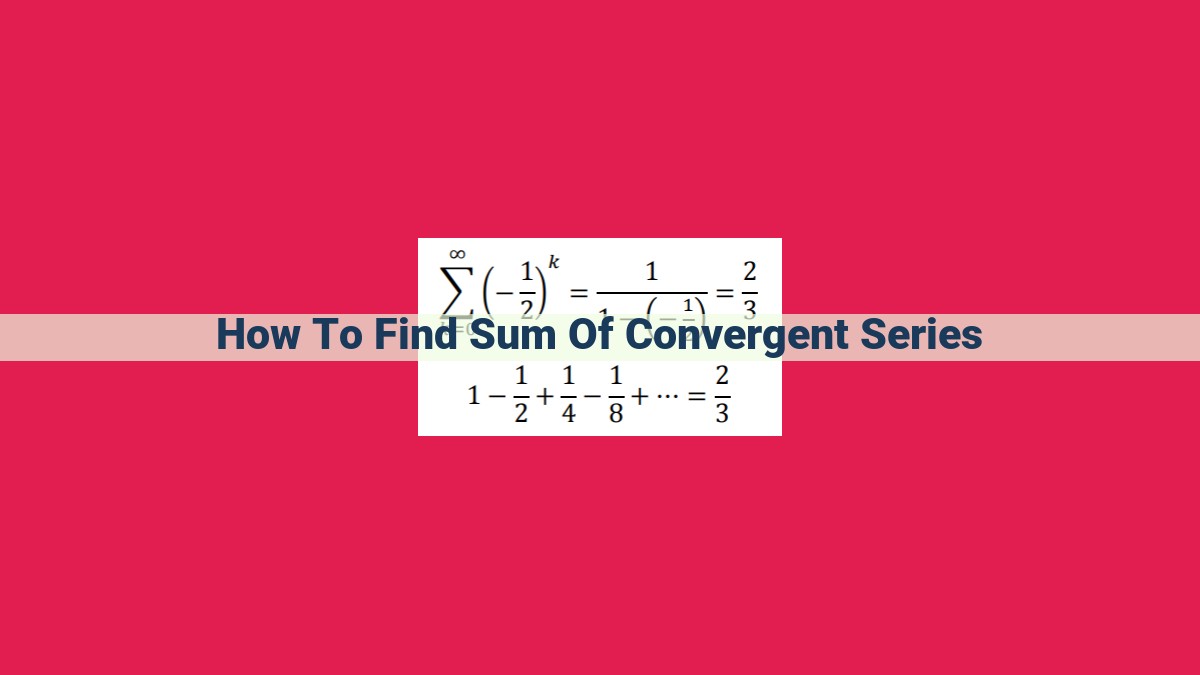
Geometric Series: A Path to Understanding Convergence

Embark on a mathematical journey to explore the geometric series, a fascinating concept that unveils the secrets of convergence. A geometric series is a beautiful dance of numbers, where each number is obtained by multiplying the preceding number by a constant factor known as the common ratio. This harmonious sequence holds the key to unlocking a plethora of mathematical mysteries.

Conditions for Convergence

For a geometric series to converge, it must adhere to certain conditions. If the absolute value of the common ratio is less than 1, the series will embark on a journey towards a finite destination. This means that the numbers in the series will gradually shrink, dancing closer and closer to zero. However, if the absolute value of the common ratio is greater than or equal to 1, the series will spiral towards infinity, forever chasing a goal it can never reach.

Finding the Sum of a Convergent Geometric Series

When a geometric series dances within the realm of convergence, we can unveil its secrets and find the sum of its numbers. Imagine a telescoping sum, where each number takes a step forward and a step back, ultimately canceling each other out. This elegant dance reveals the sum of a convergent geometric series as a simple formula:

Sum = a / (1 - r)

where a represents the first number in the series and r is the common ratio.

Applications in the Real World

Geometric series are not just mathematical curiosities; they play a crucial role in our understanding of the world around us. They power the growth of bacteria colonies, determine the half-life of radioactive isotopes, and help us calculate the interest on our savings accounts. By understanding the intricacies of geometric series, we gain a deeper appreciation for the mathematical foundations that shape our reality.

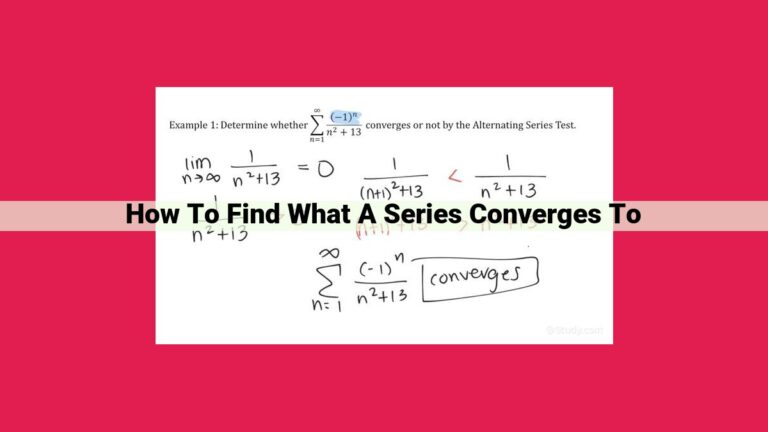
Alternating Series: A Tale of Balancing Positives and Negatives

In the realm of mathematics, we encounter series, an infinite sum of terms. Among these series, there’s a fascinating species known as alternating series, where terms alternate between positive and negative values.

An alternating series assumes the form:

∑ (-1)^n * a_n

where:

• a_n is a positive term for all n
• n is a natural number starting from 1

Absolute and Conditional Convergence

An alternating series is said to be absolutely convergent if the series of its absolute values converges. In other words, the series

∑ |a_n|

converges.

However, an alternating series can also exhibit conditional convergence. This occurs when the series of its absolute values diverges but the original alternating series converges.

The Alternating Series Test

To determine the convergence of an alternating series, we can use the Alternating Series Test, which states that an alternating series converges if the following conditions are met:

1. a_n is positive and decreases for all n
2. lim (a_n) = 0

Estimating the Sum

Even if an alternating series converges, it can be challenging to find its exact sum. However, we can estimate the sum of the first n terms using the following formula:

|R_n| ≤ a_{n+1}

where R_n represents the error between the sum of the first n terms and the actual sum of the series.

Applications and Significance

Alternating series are found in various applications, including:

• Evaluating integrals
• Approximating functions
• Solving differential equations

By understanding the behavior of alternating series, mathematicians can effectively solve problems and make accurate approximations even in the presence of alternating positive and negative terms.

The Comparison Test: A Guiding Light in the Convergence Quest

In the realm of mathematics, the pursuit of understanding the behavior of infinite series is a fundamental endeavor. One powerful tool in this quest is the Comparison Test, a beacon of guidance that helps us illuminate the convergence or divergence of a series based on the properties of another series.

Imagine a series of terms, each like a miniature step in a mathematical journey. If we can find another series, one that’s similar in nature to the original, and we know that the second series converges, then we can infer something about the first series as well.

The Logic Behind the Comparison Test

The Comparison Test relies on a simple yet profound idea: if the terms of a series are never greater than the terms of a convergent series, then the original series must also converge. Conversely, if the terms of our series are always greater than the terms of a divergent series, then the original series must also diverge.

The key to using this test effectively lies in choosing the right comparison series. It should be a series that we already know converges or diverges, and it should have some similarities with the original series.

Bounded Sequences: A Helpful Concept

In the context of the Comparison Test, we encounter the notion of bounded sequences. A sequence is said to be bounded if it has an upper bound and a lower bound. This means that there are two numbers, an upper limit and a lower limit, that no term in the sequence can exceed or fall below.

When working with the Comparison Test, if we can show that the terms of our original series are always less than or equal to the terms of a convergent comparison series that’s bounded, then we can conclude that the original series also converges.

Leveraging the Comparison Test

To illustrate the power of this test, let’s consider the series:

1 + 1/2 + 1/4 + 1/8 + 1/16 + ...

We notice that the terms of this series are decreasing and positive. We can compare this series to the convergent geometric series:

1 + 1/2 + 1/4 + 1/8 + 1/16 + ... = 2

Since the terms of our original series are smaller than or equal to the terms of the convergent geometric series, and because the geometric series is bounded, we can conclude that the original series also converges.

The Comparison Test is an invaluable tool in the exploration of convergent series. By comparing the behavior of a series to that of a known convergent or divergent series, we can shed light on its convergence properties. This test not only helps us determine if a series converges or diverges, but also provides insights into the nature of the series’ terms, ultimately enhancing our understanding of the intricate world of infinite series.

The Ratio Test: A Trick to Tame Infinite Sums

Imagine you have an infinite line of people, each holding a certain amount of money. The person at the front has the most, and each subsequent person has an amount that is a fixed fraction of the person in front. This line is what we call a geometric series.

The question is: Can you tell whether the total amount of money in this line is finite or goes to infinity as you keep adding up the people? The Ratio Test is a tool that can help you answer this question.

The trick is to look at the ratio of consecutive terms:

r = (a_n+1 / a_n)

If this ratio approaches a number less than 1 as you move down the line (i.e., n gets larger and larger), then the series converges (meaning the total amount of money is finite). If the ratio is greater than 1, the series diverges (meaning the total amount is infinite).

For example: Consider the series 1 + 1/2 + 1/4 + 1/8 + …

The ratio of consecutive terms is 1/2. Since this ratio is less than 1, the series converges.

The Ratio Test is particularly useful when:

• The terms of the series decrease rapidly, making the comparison test difficult.
• The terms do not have a simple pattern, making the integral test impractical.

Remember: The Ratio Test is just one of many tools available to determine convergence or divergence of series. By mastering this trick, you can unlock the secrets of infinite sums and gain a deeper understanding of the mathematical world.

The Root Test: Unraveling the Convergence Secrets of Series

In the world of mathematics, series are like intricate puzzles, their convergence determining whether they reveal a finite sum or an elusive infinity. Among the many tests that guide us in solving these puzzles is the root test.

Imagine a series as an endless sequence of terms, each one a piece of a larger whole. The root test focuses on the limit of the $n$th root of these terms. When this limit is less than 1, the series converges, like a gentle stream flowing towards a peaceful lake. However, when the limit is greater than 1, the series diverges, like a raging river forever cascading into the unknown.

The root test is a powerful tool because it often provides a clear-cut answer, unlike other tests that may yield inconclusive results. It works particularly well when the terms of the series involve exponential or polynomial functions. For example, consider the series

∑ (2^n) / n^2

Using the root test, we calculate the limit of the $n$th root of the terms:

lim (2^n / n^2)^(1/n) = 2/1 = 2

Since the limit is greater than 1, the series diverges.

The root test is a valuable addition to our mathematical toolbox, providing a straightforward method for determining the convergence or divergence of series. Its simplicity and effectiveness make it a favorite among mathematicians seeking to unlock the mysteries of these infinite sums.

Integral Test

• Introduce the integral test for convergence.
• Explain how to use a definite integral to determine convergence or divergence.
• Discuss its relationship with the comparison test.

The Integral Test for Convergent Series: A Gateway to Infinite Sums

In our journey through the realm of convergent series, we come across a powerful tool known as the Integral Test. This test provides a clever way to determine whether an infinite sum converges or diverges by utilizing the familiar concept of definite integrals.

The Integral Test:

For a non-negative series, that is, a series with all terms being positive, the Integral Test states that the series converges if and only if the improper integral of the corresponding function from 1 to infinity is convergent. In other words, if:

∫[1, ∞] f(x) dx converges

then:

Σ[n=1,∞] f(n) converges

Conversely, if:

∫[1, ∞] f(x) dx diverges

then:

Σ[n=1,∞] f(n) diverges

Understanding the Integral Test:

The Integral Test is based on the intuitive notion that the cumulative sum of a series can be approximated by the area under the corresponding curve. If the area under the curve is finite, then the series is likely to converge. Conversely, if the area is infinite, the series may diverge.

Relationship with the Comparison Test:

The Integral Test is closely related to the Comparison Test. If we can find a function g(x) that is continuous, positive, and satisfies g(n) ≤ f(n) for all n ≥ 1, then the convergence or divergence of the series:

Σ[n=1,∞] g(n)

can be used to determine the convergence or divergence of the series:

Σ[n=1,∞] f(n)

Applications of the Integral Test:

The Integral Test has numerous applications in evaluating convergent series. It is particularly useful for testing series that involve integrals or logarithms. For example, it can be used to show that the harmonic series:

Σ[n=1,∞] 1/n

diverges, while the p-series:

Σ[n=1,∞] 1/n^p

converges for p > 1.

The Integral Test is a versatile tool for testing the convergence or divergence of convergent series. By understanding the relationship between integrals and infinite sums, we gain a powerful means to analyze and evaluate these important sequences. Whether in the realm of mathematics or real-world applications, the Integral Test provides invaluable insights into the behavior of infinite series.