Expert Guide: Master Fraction Subtraction With Different Denominators

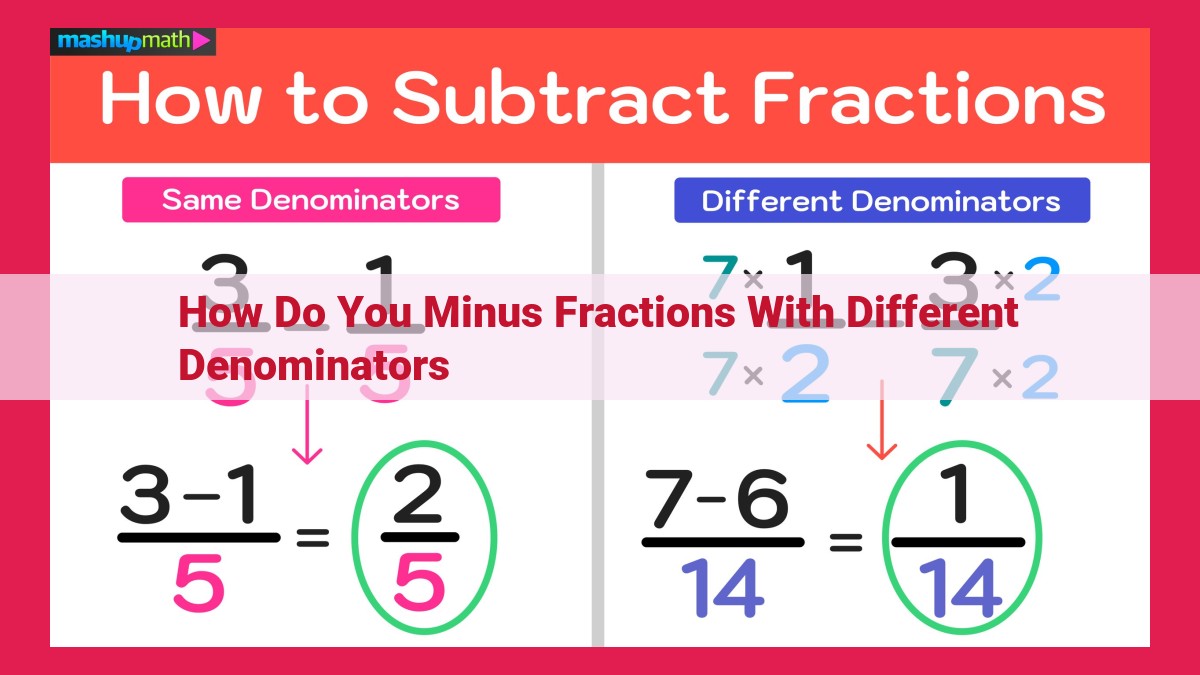
To subtract fractions with different denominators, first find their Least Common Multiple (LCM) and multiply both fractions by the same factors to create equivalent fractions with the same denominator. Then, subtract the numerators of these equivalent fractions while keeping the denominator the same. This process ensures that the denominators are aligned and the subtraction can be performed accurately.

Understanding the Concept: Denominators and LCM

• Explain the concept of a fraction and the significance of the denominator.
• Define Least Common Multiple (LCM) and its role in simplifying fractions.
• Provide examples to illustrate how to find the LCM of different denominators.

Understanding the World of Fractions and Denominators

In the realm of numbers, fractions reign supreme as the unsung heroes of mathematical adventures. A fraction, like a window into a parallel world, represents a part of a whole. Every fraction has two key components – the numerator and the denominator. The numerator, like a beacon of light, guides us to the quantity represented, while the denominator, a steadfast anchor, tells us the total number of equal parts that make up the whole.

The Magic of Least Common Multiples (LCM)

When fractions dare to venture into the world of subtraction, a special helper emerges – the Least Common Multiple (LCM). The LCM, like a master strategist, finds the smallest positive number that can be divided evenly by all the denominators of the fractions in question. Finding the LCM is like creating a common language that unites the fractions, allowing them to communicate harmoniously.

Transforming Fractions into Equivalents

To conquer the realm of fraction subtraction, we employ a secret technique known as equivalent fractions. Just like shape-shifting wizards, equivalent fractions appear different but represent the same value. We can create equivalent fractions by multiplying both the numerator and the denominator by the same non-zero number. This magical act leaves the fraction’s value unchanged, yet gives us the power to mold the fraction into a form that aligns perfectly with the others.

Subtracting Fractions: A Tale of Alignment

With our fractions now aligned, the stage is set for subtraction. We bravely subtract the numerators of the equivalent fractions, keeping the denominator intact. This act of subtraction reveals the difference between the quantities represented by the fractions. It’s like a modern-day jousting match, where the fractions battle for supremacy, and the difference emerges victorious.

Maintaining Denominational Harmony

To ensure a smooth subtraction, we must ensure that all denominators sing in unison. This harmony is achieved by converting each fraction into an equivalent form with the same denominator. It’s like giving each fraction a passport that allows them to communicate freely. With this unity, the subtraction becomes a graceful dance, leading us to the desired result.

Multiplying to Create Equivalent Fractions

Imagine you have a recipe that calls for 1/2 cup of flour. But you only have a measuring cup with markings in quarter cups. How can you measure out the right amount of flour?

The answer lies in creating equivalent fractions. An equivalent fraction has the same value as the original fraction, but the numerator and denominator are different.

To create an equivalent fraction, you can multiply both the numerator and denominator by the same number. For example, if we multiply both the numerator and denominator of 1/2 by 2, we get 2/4.

1/2 * 2/2 = 2/4

Both 1/2 and 2/4 represent the same amount of flour. This is because multiplying by 2 cancels out, leaving us with the same value.

This technique can be used to convert any fraction to an equivalent fraction with any desired denominator. For example, to convert 3/4 to an equivalent fraction with a denominator of 12, we would multiply both the numerator and denominator by 3.

3/4 * 3/3 = 9/12

Now we have an equivalent fraction (9/12) that has the denominator 12. This makes it easy to measure out the flour using our quarter-cup measuring cup.

Remember, when creating equivalent fractions, multiplying by the same number is key. This simple technique will help you convert fractions to any denominator you need, making it easier to solve problems and perform calculations.

Subtracting Numerators: The Key Step in Fraction Subtraction

In the realm of fractions, the concept of subtraction is much like a secret code, known only to those who understand the subtle art of aligning and subtracting the numerators. To grasp this hidden knowledge, let’s embark on a journey that will unveil the secrets of fraction subtraction.

At the heart of fraction subtraction lies a simple yet profound truth: only the numerators dance, while the denominators watch in silence. This means that when we subtract fractions, we focus solely on the numbers on top, leaving the numbers on the bottom untouched.

The secret to aligning the numerators is to ensure they are standing side by side like soldiers in formation. To achieve this, we employ the power of equivalent fractions. Recall that equivalent fractions are fractions with different numerators and denominators but represent the exact same value. We use these equivalent fractions to transform our fractions into identical outfits, making subtraction a breeze.

Now, with the numerators aligned and ready for action, we can perform the subtraction. Just like in ordinary number subtraction, we simply subtract the smaller numerator from the larger numerator. The result becomes the new numerator of our answer.

Example: Let’s subtract the fractions 3/4 and 1/4:
– Align the numerators: 3/4 – 1/4.
– Subtract the numerators: 2/4.

Remember: The denominator remains the same. So, our final answer is the fraction 2/4.

Mastering this technique of subtracting numerators is the key to unlocking the world of fraction subtraction. It allows us to tame the most challenging fractions and conquer any mathematical challenge that comes our way.

Keeping the Denominators Consistent

In the realm of fraction subtraction, ensuring uniformity among denominators is crucial, like fitting puzzle pieces into their designated slots. To comprehend why, let’s embark on a mathematical journey.

Imagine you’re at a market, trying to compare the prices of apples and bananas. Apples cost $0.50 each, while bananas cost $0.25 per pound. To determine which purchase offers a better deal, you need to convert the prices to a common unit of measurement, like dollars per pound.

In the same vein, when subtracting fractions with different denominators, we need to find a common denominator that acts as a level playing field for all fractions involved. This is where equivalent fractions come into play.

Equivalent fractions are fractions that represent the same value, but their numerators and denominators may differ. For instance, 1/2 and 2/4 are equivalent fractions because they both represent half of a whole.

To ensure consistent denominators, we can use equivalent fractions to convert one or both fractions to have the same denominator. Let’s say we want to subtract 1/3 – 1/4. To make the denominators the same, we can convert 1/3 to 4/12 and keep 1/4 as it is.

1/3 = 4/12
1/4 = 1/4

Now, we can subtract the numerators while keeping the denominator the same:

4/12 - 1/4 = 3/12 = 1/4

By keeping the denominators consistent, we can ensure accurate and meaningful subtraction of fractions. This concept is vital not only in mathematics but also in real-world applications, such as cooking, construction, and budget management, where precise measurements and calculations are essential.

Examples and Applications

• Provide practical examples to illustrate the subtraction of fractions with different denominators.
• Discuss the application of this concept in real-world scenarios, such as measuring ingredients or calculating time intervals.
• Include practice problems to engage readers and test their understanding.

Subtracting Fractions: A Tale of Denominator Harmony

In the realm of fractions, subtraction presents a unique challenge: aligning denominators. Just like musicians playing in an orchestra, fractions must harmonize their “bottom numbers” (denominators) before they can perform their subtraction dance.

Let’s embark on an adventure to unravel this enchanting process. First, we’ll explore the concept of the Least Common Multiple (LCM), the magic number that brings denominators into harmony. We’ll then discover how to multiply fractions by the same number, creating equivalent fractions that share the same denominator.

With our denominators aligned, we can finally waltz into the world of subtracting fractions. It’s a simple step that involves subtracting the numerators (top numbers) while keeping the denominator the same. But remember, just like in a dance, it’s crucial to align the numerators before subtracting to avoid any missteps.

To ensure perfect harmony, we may need to convert fractions to their equivalent forms. These musical chairs lead us to denominators that sing the same tune. Using examples, we’ll witness how this conversion transforms fractions into a harmonious ensemble.

To bring this concept to life, let’s venture into practical examples. From measuring ingredients in a culinary masterpiece to calculating time intervals in a grand adventure, we’ll see how subtracting fractions plays a vital role in our everyday routines.

Finally, we’ll present a symphony of practice problems for you to test your newfound skills. By solving these fraction puzzles, you’ll become a maestro of denominator harmony, effortlessly finding the difference between fractions and adding a touch of math magic to your life.