Ln: The Natural Logarithm Unveiled

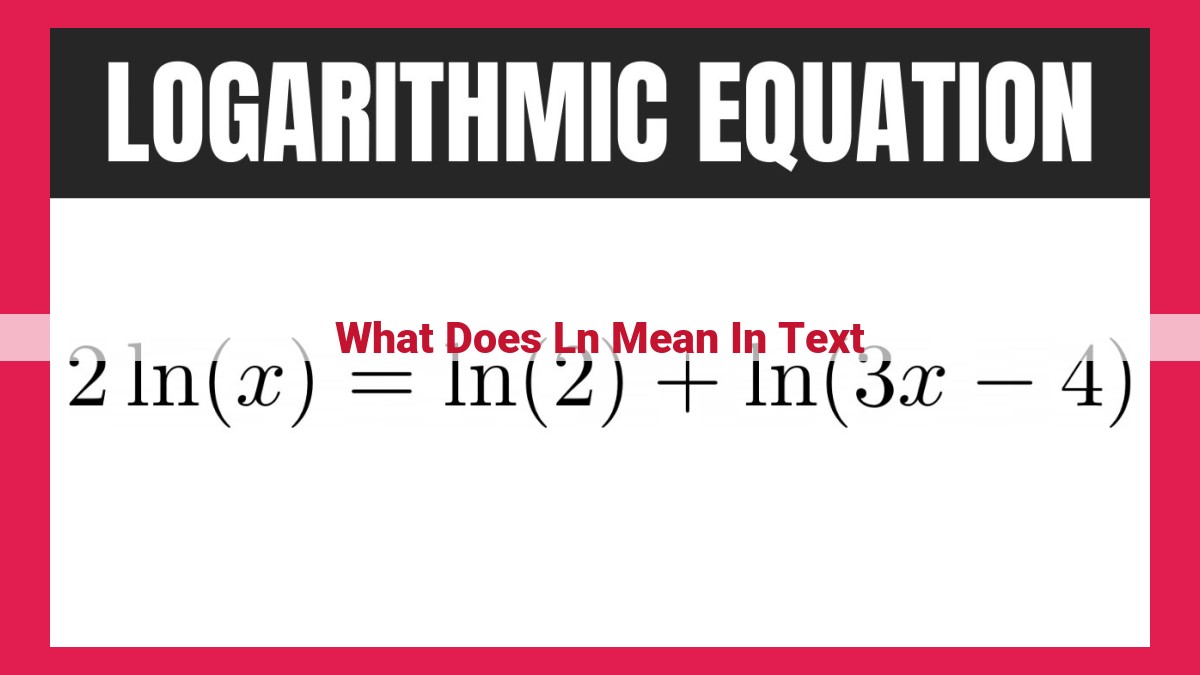
Ln Meaning in Text

Ln, or natural logarithm, is a mathematical function that takes a positive number as input and produces its exponent when expressed as a base of e. It’s related to the exponential function (e^x), with the identity e^ln(x) = x. Ln has unique properties, including ln(e) = 1, ln(1) = 0, and ln(xy) = ln(x) + ln(y). Its applications span fields like exponential growth models, probability, and calculus, making it a versatile tool in mathematics and scientific disciplines.

The Natural Logarithm: A Mathematical Enigma Unraveled

In the realm of mathematics, there exists an intriguing concept known as the natural logarithm, denoted by ln. It’s a logarithm with a unique base, the enigmatic e, which holds profound significance in the mathematical universe.

The natural logarithm, ln(x), is defined as the exponent to which e must be raised to produce x. In simpler terms, it’s the exponent that makes e equal to x. This extraordinary concept allows us to simplify complex exponential equations and delve into the intricacies of mathematical modeling.

Concept of Natural Logarithm

The natural logarithm, denoted as ln or logₑ, is a mathematical function that measures the exponent to which a number e must be raised to obtain another number x. In other words, lnx is the power to which you raise e to get x.

Consider the equation:

e^(ln(x)) = x

This equation illustrates the inverse relationship between the exponential function, denoted as e^x, and the natural logarithm. The exponential function raises e to the power of x, while the natural logarithm “undoes” this operation by finding the exponent to which e was raised to get x.

The natural logarithm has several important mathematical properties:

• ln(e) = 1: When the input to the natural logarithm is e, the output is 1. This is because e raised to the power of 1 is e itself.

• ln(1) = 0: When the input to the natural logarithm is 1, the output is 0. This is because e raised to the power of 0 is 1.

• ln(xy) = ln(x) + ln(y): The natural logarithm of a product of two numbers is equal to the sum of the natural logarithms of those numbers.

• ln(x^n) = n * ln(x): The natural logarithm of a number raised to a power is equal to the power multiplied by the natural logarithm of that number.

These properties make the natural logarithm a valuable tool for solving mathematical equations and modeling real-world phenomena.

Unveiling the Natural Logarithm: A Mathematical Journey

In the realm of mathematics, the natural logarithm, denoted by ln, stands as a pillar of logarithmic operations, with its base firmly rooted in the enigmatic mathematical constant e. This journey will delve into the essence of the natural logarithm, exploring its concept, properties, and applications.

The Heart of the Natural Logarithm

The natural logarithm is defined as the logarithm to the base e, a number approximately equal to 2.718. Just as logarithms express exponents, ln unravels the exponent to which e must be raised to yield the original number.

Related Concepts: An Interwoven Tapestry

The natural logarithm is closely intertwined with the exponential function, represented by e^x. ln and e^x form an inverse pair, with the output of one being the input of the other. This relationship is elegantly captured by the identity e^ln(x) = x.

Logarithms in General

Logarithms are essentially exponent operations that unveil the exponent of a given base to obtain another number. The natural logarithm is a specific type of logarithm that uses e as its base.

The Mathematical Constant e

e is the mathematical constant that serves as the cornerstone of the natural logarithm. It holds immense significance in exponential functions, calculus, and other mathematical realms.

Properties: Unlocking the Secrets of ln

The natural logarithm possesses a rich tapestry of properties that govern its behavior:

• ln(e) = 1, indicating that raising e to the power of 1 yields the natural logarithm of e.
• ln(1) = 0, signifying that the natural logarithm of 1 is 0.
• ln(xy) = ln(x) + ln(y), revealing that the natural logarithm of a product is the sum of the natural logarithms of its factors.
• ln(x^n) = n * ln(x), highlighting that the natural logarithm of a number raised to a power is equal to the power multiplied by the natural logarithm of the number.

Applications: A Transformative Force

The natural logarithm finds widespread applications across diverse fields, including:

• Exponential Growth and Decay Models: ln plays a crucial role in modeling exponential growth and decay phenomena, providing insights into population dynamics, radioactive decay, and financial markets.
• Probability Distributions: ln is instrumental in probability theory, particularly in the analysis of continuous distributions such as the normal distribution.
• Calculus and Integral Equations: ln serves as an indispensable tool in calculus and integral equations, facilitating differentiation, integration, and solving differential equations.

In conclusion, the natural logarithm is a mathematical bedrock that empowers us to understand and manipulate logarithmic operations. Its inverse relationship with the exponential function, intertwined concepts, distinct properties, and far-reaching applications make ln a valuable tool in the vast landscape of mathematics and its real-world applications.

Properties of the Natural Logarithm: Unveiling the Secrets of ln

The natural logarithm, denoted as ln, is a mathematical function that holds significant importance in various fields. Understanding its properties is crucial for harnessing its power and applying it effectively in practical applications.

ln(e) = 1

This property establishes that the logarithm of the base of the natural logarithm (e) is equal to 1. This is an intuitive result, as it implies that any number raised to the power of 1 is simply itself.

ln(1) = 0

Another fundamental property of ln is that the logarithm of 1 is always 0. This is because any number raised to the power of 0 equals 1, and the logarithm of 1 is the exponent that yields this result.

ln(xy) = ln(x) + ln(y)

This property, known as the product rule, states that the logarithm of a product is equal to the sum of the logarithms of the individual factors. This property is particularly useful when simplifying logarithmic expressions involving multiplication.

ln(x^n) = n * ln(x)

The power rule of ln states that the logarithm of a number raised to a power is equal to the power multiplied by the logarithm of the number. This property highlights the relationship between exponentiation and logarithmic functions.

These four properties form the foundation of working with the natural logarithm. They provide a set of rules that govern the behavior of ln and allow us to manipulate logarithmic expressions effectively. Understanding these properties is essential for unlocking the full potential of the natural logarithm in various applications, such as exponential growth and decay models, probability distributions, and calculus.

Applications of the Natural Logarithm

Step into the fascinating world of the natural logarithm (ln), where its applications extend far beyond the realm of mathematics. This logarithmic wizard plays a pivotal role in various disciplines, empowering us to understand and solve problems in a myriad of fields.

Exponential Growth and Decay Models

The natural logarithm is an indispensable tool for modeling exponential phenomena. From the growth of bacteria in a petri dish to the decay of radioactive substances, ln helps us describe and predict how quantities change over time. In these models, the exponential function (e^x) represents the growth or decay rate, while ln acts as the inverse function, revealing the underlying pattern.

Probability Distributions

In the realm of probability, the natural logarithm finds its place in describing the distribution of random variables. The normal distribution, a cornerstone of statistics, relies on ln to characterize the spread and shape of data. By applying the natural logarithm to probability density functions, we can gain insights into the likelihood of specific outcomes and the overall distribution of values.

Calculus and Integral Equations

The natural logarithm’s significance extends to the world of calculus. It serves as the fundamental tool for integrating exponential functions, unlocking the power to solve complex integrals. In differential equations, ln plays a crucial role in solving first-order linear equations and opens the door to understanding more intricate equations involving derivatives.

By delving into these practical applications, we uncover the true power of the natural logarithm. It’s not just a mathematical concept but a versatile tool that helps us model real-world phenomena, make predictions, and solve intricate problems. From exponential growth to decay models, probability distributions to calculus, ln empowers us to navigate diverse scientific and mathematical landscapes.