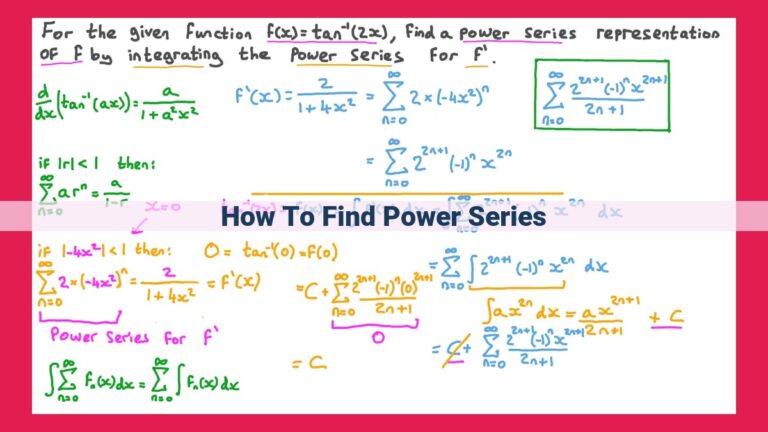
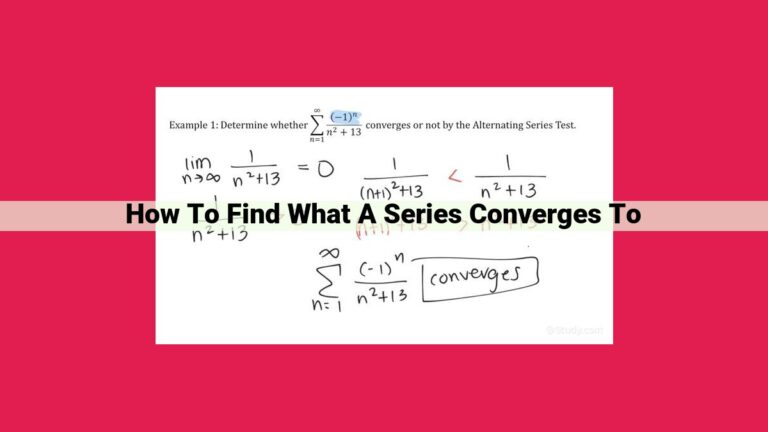
Determining Limit Existence: A Comprehensive Guide Using Continuity, Intermediate Value, And Squeeze Theorems

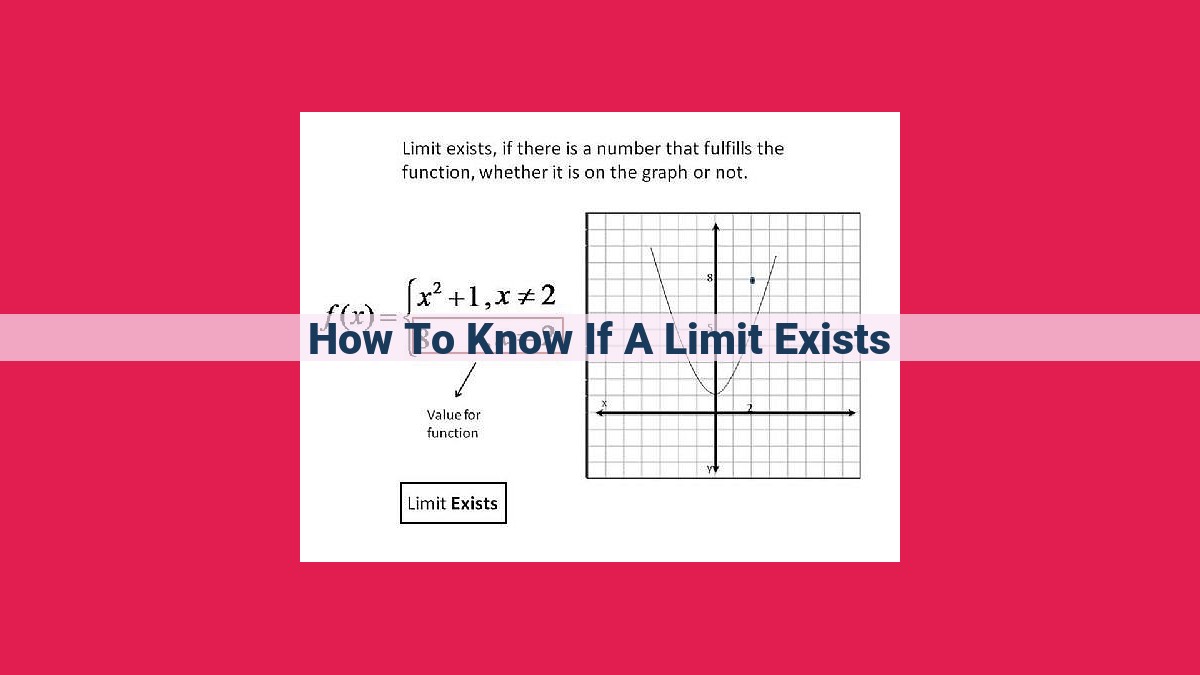
To determine if a limit exists, one can establish continuity, utilize the Intermediate Value Theorem, or apply the Squeeze Theorem. Continuity implies the existence of a limit; the Intermediate Value Theorem indicates a limit’s existence if the function is continuous on a closed interval containing the point of interest; and the Squeeze Theorem establishes the limit’s existence if it is sandwiched between two other functions with known limits.

**Unlocking the Significance of Limits: A Gateway to Calculus and Beyond**

Limits, cornerstones in the realm of calculus, serve as a gateway to unlocking profound mathematical concepts. They hold immense significance in analysis, providing a solid foundation for understanding complex functions and their behavior.

Methods for Determining the Existence of Limits

Determining the existence of limits is crucial for comprehending the continuity and behavior of functions. Various methods come to our aid in this endeavor. Direct substitution, a straightforward technique, reveals the limit’s value if the function is defined at the specific point. When direct substitution fails, algebraic manipulations, such as factoring or rationalization, can pave the way to a clearer path.

Continuity and Limits: A Harmonious Duo

In the fascinating world of calculus and analysis, limits are indispensable tools that unlock a deeper understanding of functions and their behavior. Closely intertwined with the concept of limits is the notion of continuity, a fundamental property that characterizes functions’ smoothness and absence of abrupt changes. Together, limits and continuity form an intricate partnership, aiding mathematicians and scientists in unraveling the complexities of functions.

Defining Continuity

Continuity, at its core, describes how a function behaves as its input smoothly transitions from one value to another. In mathematical terms, a function is said to be continuous at a particular point if its value at that point is equal to the limit of the function’s values as the input approaches that point.

The Interplay of Continuity and Limits

As we delve deeper into the relationship between continuity and limits, we uncover a profound connection. Any function that is continuous at a given point must also possess a limit at that point. Conversely, if a function has a limit at a specific point, it suggests that the function might be continuous at that point. This interconnectedness makes limits an invaluable tool for investigating continuity.

Limits from a Continuity Perspective

Limits offer an alternative perspective on continuity. By examining the limit of a function as its input approaches a particular point, we can determine whether the function is continuous at that point. If the limit exists and is equal to the function’s value at that point, continuity is established. This approach provides a comprehensive understanding of continuity and complements the direct definition.

Harnessing the Power of the Intermediate Value Theorem

Embarking on a Calculus Quest

As we delve deeper into the fascinating realm of calculus, we encounter a pivotal concept: limits. These enigmatic entities are the gateways to understanding the behavior of functions as inputs approach specific values. To uncover the mysteries surrounding limits, we have a trusty ally in the form of the Intermediate Value Theorem.

The Essence of the Intermediate Value Theorem

The Intermediate Value Theorem (IVT) proclaims a profound truth about continuous functions: if a function f(x) is continuous on a closed interval [a, b], then for any value c between f(a) and f(b), there exists at least one value x in the interval such that f(x) = c.

A Glimpse into the Theorem’s Implications

This theorem holds immense significance in our calculus journey. It reveals that continuous functions cannot abruptly jump from one value to another. Instead, they must take on all intermediate values as they traverse an interval. This property provides a crucial tool for proving the existence of limits.

Unveiling Limit Existence with the IVT

Consider a function f(x) that is continuous on an open interval containing the point c. If there exists a value L such that for any ε > 0, we can find a δ > 0 such that |f(x) – L| < ε whenever 0 < |x – c| < δ, then, according to the IVT, _f(x) must take on every value between f(c-δ) and f(c+δ). This implies that f(x) cannot be arbitrarily close to any value other than L within the interval, confirming the existence of the limit lim_(x→c) f(x) = L.

Exploring Related Concepts: Extreme Value Theorem and Rolle’s Theorem

The IVT is intertwined with two other remarkable theorems: the Extreme Value Theorem and Rolle’s Theorem. The Extreme Value Theorem guarantees that continuous functions on closed intervals attain both a maximum and a minimum value. Rolle’s Theorem, on the other hand, states that if a function is continuous on a closed interval and differentiable on the interval’s interior, then there exists at least one point where the function’s derivative is zero. These theorems, along with the IVT, form a formidable arsenal for exploring the behavior of functions.

Unveiling the Power of the Squeeze Theorem

In the realm of calculus, the Squeeze Theorem emerges as a formidable tool, empowering us to establish the existence of limits in scenarios where other methods may falter. Its elegance lies in its ability to sandwich a function between two other functions whose limits we already know.

The Squeeze Theorem, in its majestic simplicity, states:
If (f(x) \le g(x) \le h(x)) for all (x) in an open interval containing (c) and
If (\lim\limits_{x \to c} f(x) = \lim\limits_{x \to c} h(x) = L), then
(\lim\limits_{x \to c} g(x) = L)

This concept is akin to the classic Goldilocks tale. We have three functions: (f(x)), (g(x)), and (h(x)). (f(x)) and (h(x)) are like the porridge that’s “just right” – they both approach the same limit (L) as (x) approaches (c). (g(x)) is our Goldilocks, stuck in the middle, and the Squeeze Theorem tells us that it must also approach (L).

Applying the Squeeze Theorem is a straightforward process:

1. Find two functions, (f(x)) and (h(x)), such that (f(x) \le g(x) \le h(x)) in an open interval containing (c).
2. Calculate the limits of (f(x)) and (h(x)) as (x) approaches (c).
3. If the limits of (f(x)) and (h(x)) are equal to (L), then the limit of (g(x)) as (x) approaches (c) is also (L).

The Squeeze Theorem is a powerful tool with a wide range of applications. It can be used to establish the existence of limits in a variety of contexts, including trigonometric functions, exponential functions, and logarithmic functions. Its versatility makes it a cornerstone of calculus and mathematical analysis.

Bridging the Gap: Unveiling the Interdependence of Limit Theorems

Introduction:
In our exploration of limits, we have encountered various methods to determine their existence, each with its own strengths and applications. Now, let’s unveil the intricate connections between these methods, revealing an elegant framework that empowers us to tackle complex limit problems.

The Interplay of Methods:
The epsilon-delta definition provides a rigorous foundation for limits, defining them in terms of specific bounds and neighborhoods. The Intermediate Value Theorem helps us prove the existence of limits by leveraging the continuity of functions. The Squeeze Theorem offers a powerful tool to establish limits by bounding the function between two sequences with known limits.

Building Blocks of a Robust Framework:
These methods are not isolated entities but rather interconnected building blocks that complement each other. The epsilon-delta definition sets the stage for understanding limits, while the Intermediate Value Theorem provides a bridge to continuity. The Squeeze Theorem, in turn, utilizes the concepts of bounding functions and limits to achieve its purpose.

A Comprehensive Approach to Limit Analysis:
By combining these methods, we gain a comprehensive approach to limit analysis. The epsilon-delta definition provides the theoretical basis, the Intermediate Value Theorem connects limits to continuity, and the Squeeze Theorem offers a practical tool for establishing existence. Together, they form a coherent system that empowers us to tackle a wide range of limit problems with confidence.

Conclusion:
The interdependence of limit theorems is not merely a theoretical curiosity but a practical necessity for effective limit analysis. By understanding the connections between these methods, we gain a deeper appreciation for the subject and develop a robust framework that enables us to navigate complex mathematical problems with ease.

Applications and Impact: Expanding Horizons with Limit Theorems

The realm of mathematics extends far beyond the confines of textbooks and classrooms. Limit theorems, those indispensable tools for understanding the behavior of functions, find their applications in a myriad of mathematical and scientific disciplines.

• Calculus and Analysis: Limits are the cornerstone of calculus, enabling us to determine the rates of change (derivatives) and areas under curves (integrals). They provide the foundation for understanding advanced mathematical concepts like transcendental functions, sequences, and series.

• Physics: In physics, limits help us analyze the motion of objects, such as calculating instantaneous velocities and accelerations. They also play a crucial role in modeling physical phenomena like fluid dynamics, thermodynamics, and quantum mechanics.

• Engineering: Engineers rely on limits to design structures, predict fluid flow, and optimize energy systems. By understanding the limiting behavior of functions, they can ensure the safety and efficiency of their designs.

• Economics: Limits are used in economics to study the behavior of markets, predict consumer demand, and analyze investment strategies. They help economists understand how economies evolve over time and make informed decisions.

• Computer Science: In computer science, limits are useful in algorithm analysis, where they help determine the efficiency and time complexity of algorithms. They also play a role in computer graphics, where they allow us to create smooth and realistic animations.

Beyond their theoretical applications, limit theorems have a profound impact on our daily lives:

• Navigation: GPS devices use limits to calculate our current position by trilaterating our distance from multiple satellites.

• Medical Imaging: Medical imaging techniques like X-rays and MRIs rely on limits to create clear and accurate images of the human body.

• Weather Forecasting: Meteorologists use limits to predict weather patterns and forecast the severity of storms.

By mastering limit theorems, we not only unlock the door to advanced mathematics but also gain a deeper understanding of the world around us. They empower us to solve complex problems, design innovative technologies, and make informed decisions in various fields. Understanding these concepts is essential for anyone aspiring to make a meaningful contribution to the ever-evolving landscape of science and technology.