Understanding Net Work: Key Concepts And Calculations

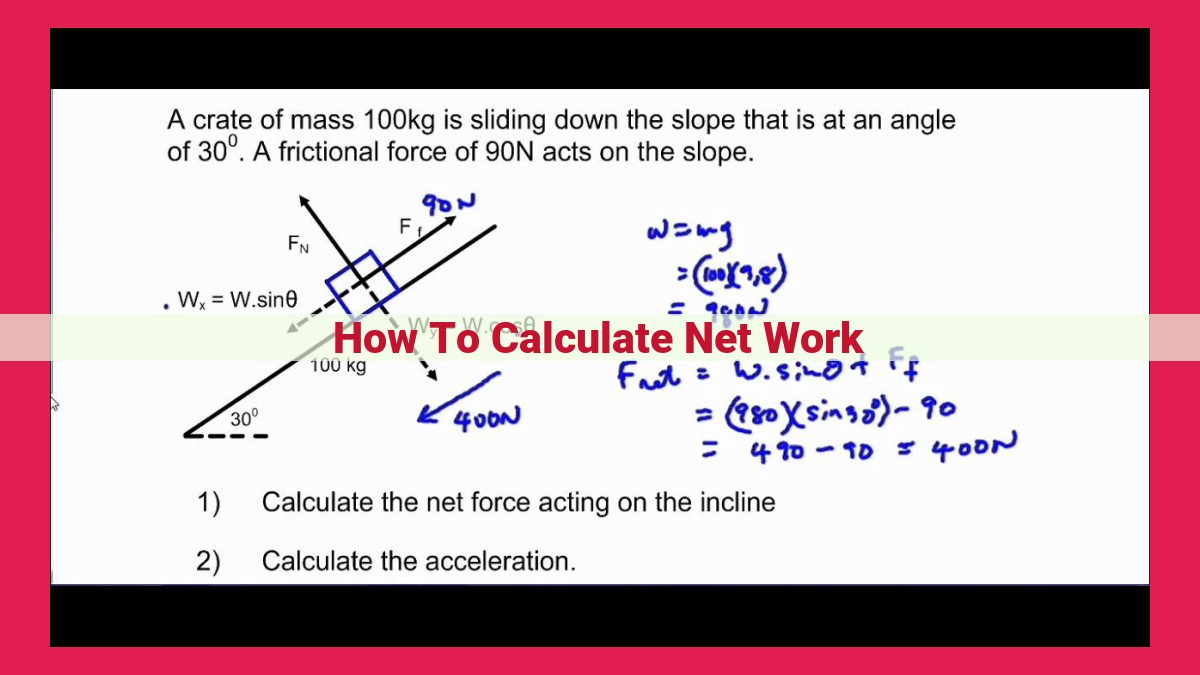
To calculate net work, sum the work of each individual force acting on an object. Net work is the overall energy transfer due to the combined effect of multiple forces. It’s calculated using the formula W_net = ΣW_i, where W_i represents the work done by each force. This concept is crucial in physics, as it aids in understanding energy transformations, problem-solving, and analyzing the behavior of objects under the influence of forces.

Work in Physics: A Cornerstone of Energy Transformations

In the vast tapestry of physics, work stands as a fundamental concept that governs energy transformations across myriad fields. It underpins our understanding of how machines operate, how energy flows, and how the universe operates on a grand scale.

Whether we are analyzing the movement of celestial bodies, the operation of an electric motor, or the simple act of pushing a book across a table, the principle of work is ever-present. It provides a quantitative measure of the energy transfer that occurs when a force is applied to move an object over a displacement.

By unraveling the intricacies of work, we gain a powerful tool for deciphering the enigmatic dance of energy in our physical world. It empowers us to design efficient engines, harness the power of nature, and even unravel the secrets of the cosmos.

Understanding the Concept of Work in Physics

Imagine yourself pushing a heavy box across the floor. As you apply force to the box and it moves, you are performing work. In physics, work is defined as the transfer of energy due to the application of a force over a distance. This concept is crucial in understanding various phenomena in fields like mechanics, engineering, and energy production.

To calculate work, we use the formula W = F * d * cos(θ), where:

• W represents the work done (in joules)
• F is the force applied (in newtons)
• d is the displacement (in meters)
• θ is the angle between the force and displacement vectors

Understanding these variables is essential for accurately determining the amount of work done. For instance, if you push the box horizontally (θ = 0°), the work done equals the product of the applied force and the distance moved. However, if you push the box at an angle, the cosine of θ will reduce the effective force, resulting in less work done.

Understanding Net Work: A Comprehensive Guide

In the realm of physics, work plays a pivotal role in describing the transfer of energy between objects. It is a fundamental concept that finds applications across various fields, including mechanics, engineering, and thermodynamics.

Defining Net Work

Net work is the total amount of work done by all the forces acting on an object. It represents the cumulative effect of individual work interactions that an object experiences. The net work done on an object is calculated as the sum of all the work interactions performed on it.

Mathematical Representation

The equation for net work is given by:

W_net = ΣW_i

where:

• W_net is the net work done
• Σ represents the summation
• W_i is the individual work done by each force

Significance of Net Work

Net work provides a quantitative measure of the total energy transferred to or from an object. It is a crucial parameter in analyzing energy transformations and problem-solving in physics. The net work done on an object can result in a change in its energy state or motion.

Understanding the Interplay of Forces and Displacement

Work is defined as the product of force and displacement. Force is a push or pull that acts on an object, while displacement is the change in position of an object. The direction of force relative to the displacement is accounted for by the cosine of the angle between them.

The Formula Unveiled

The formula for work is:

W = F * d * cos(θ)

where:

• W is the work done
• F is the force applied
• d is the displacement
• θ is the angle between the force and displacement vectors

This formula highlights the vectorial nature of work, emphasizing the importance of considering both the magnitude and direction of force and displacement.

Net work is an essential concept in physics that allows us to quantify the total energy transferred to or from an object. By understanding the interplay of forces and displacement, we can accurately calculate the net work and gain insights into energy transformations and problem-solving in various physical contexts.

Related Concepts: Force and Displacement

Understanding the concepts of force and displacement is crucial to grasping the mechanics of work. Force is a vector quantity that describes both the strength and direction of an interaction. In physics, force is responsible for applying energy to an object, causing it to undergo acceleration.

Displacement, on the other hand, is a vector quantity that represents the change in position of an object. It indicates the shortest distance between the initial and final positions of the object. In work calculations, displacement plays a key role in determining the magnitude of work performed.

Consider a simple example: when you push a book across a table, you apply a force to the book. This force causes the book to move a certain distance. The work you do on the book is equal to the force you applied multiplied by the displacement of the book.

In this scenario, the force is applied in the direction of the book’s displacement, resulting in positive work. If you were to push the book in a direction perpendicular to its displacement, the work done would be zero. This is because the force and displacement are orthogonal to each other, meaning their dot product is zero.

Power: The Measure of Work’s Efficiency

Imagine working on a project with a team of friends. Some of you slog away tirelessly, while others seem to breeze through their tasks with ease. What makes the difference? It’s not just about how much work you do, but also how quickly you do it. This is where the concept of power comes in.

Power is the rate at which work is done. It measures how much work is accomplished in a given amount of time. The formula for power is simple:

Power (P) = Work (W) / Time (t)

The unit of power is the watt (W), named after the Scottish inventor James Watt. One watt is equal to one joule of work done per second.

Implications of Power

Power is a crucial factor in many aspects of life. In engineering, power is used to design and optimize machines and systems. In sports, power is essential for achieving peak performance. And in everyday life, power helps us make decisions about how to best use our time and energy.

For example, if you’re trying to decide between two vacuum cleaners, you’ll want to consider their power ratings. A vacuum cleaner with a higher power rating will do more work in less time, making it more efficient.

Understanding Power

To calculate power, you need to know two things: the amount of work done and the time it takes to do it. The work can be measured in joules, and the time can be measured in seconds. Once you have these two values, you can simply divide work by time to get power.

The concept of power is closely related to the concept of force. Force is a push or pull that acts on an object. Displacement is the distance and direction that an object moves. When a force is applied to an object, it does work and causes the object to move. The power of the force is equal to the work done divided by the time it takes to do the work.

Power is also related to the concept of energy. Energy is the ability to do work. When an object has energy, it can exert a force and cause motion. The power of an object is equal to the rate at which it can transfer energy.

Power is a fundamental concept in physics that has applications in many different fields. By understanding power, you can better understand how the world around you works and how to use your time and energy wisely.

Other Energy Forms: Kinetic and Potential Energy

• Explain the concept of kinetic energy as energy due to motion.
• Define potential energy as energy due to position or state.

Other Energy Forms: Kinetic and Potential Energy

In the realm of physics, energy manifests in various forms, among which kinetic energy and potential energy play pivotal roles.

Kinetic energy, the epitome of energy in motion, endows an object with the ability to perform work due to its constant state of movement. The faster an object traverses, the greater its kinetic energy. Imagine a speeding car, brimming with the potential to exert significant force upon impact.

Conversely, potential energy stems from an object’s position or state. An object resting at a certain height, for instance, possesses gravitational potential energy, ready to be converted into kinetic energy as it descends. A stretched rubber band also harbors potential energy, poised to release its stored energy when set free.

The interplay between kinetic and potential energy is a captivating dance of transformation. As an object falls, its potential energy dwindles while its kinetic energy ascends, reaching its peak at the bottom of its trajectory. Conversely, when a moving object grinds to a halt, its kinetic energy vanishes, metamorphosing into potential energy.

In the tapestry of energy, the Law of Conservation of Energy reigns supreme. This immutable principle dictates that energy can neither be created nor destroyed, merely transformed from one guise to another. The sum of kinetic and potential energy remains constant, allowing scientists to meticulously analyze energy transformations and unravel the intricacies of the physical world.

Understanding the Law of Conservation of Energy in Work Calculations

In the realm of physics, energy is a fundamental concept that plays a crucial role in all physical processes. One of the most significant laws governing energy is the Law of Conservation of Energy, which postulates that energy cannot be created or destroyed, only transformed from one form to another. This principle has profound implications for our understanding of work and energy calculations.

When work is done, energy is transferred from one object to another due to the application of a force that causes displacement. The amount of work done is calculated as the product of the force applied, the displacement of the object, and the cosine of the angle between the force and displacement vectors. This formula, W = F x d x cos(θ), provides a quantitative measure of the energy transferred during the work process.

The Law of Conservation of Energy comes into play when we consider a system of multiple objects undergoing work interactions. The net work done on the system, or the W_net, is the sum of all the individual work interactions that occur within the system. Remarkably, the W_net is equal to the change in the system’s total energy.

This principle is not only conceptually elegant but also practically significant. It enables physicists and engineers to analyze energy transformations and make predictions about the behavior of systems. For instance, it allows us to calculate the power output of a machine, which is defined as the rate at which work is done. The formula for power, P = W / t, where W is the work done and t is the time taken, helps us determine how quickly energy is transferred.

The Law of Conservation of Energy reinforces the interconnectedness of energy forms, such as kinetic energy (energy due to motion) and potential energy (energy due to position or state). It illuminates the fact that energy can change its form but its total quantity remains constant. This principle underlies our understanding of energy transformations and plays a vital role in fields such as thermodynamics, mechanics, and electromagnetism.