Mastering The Art Of Isolating Variables: A Comprehensive Guide To Solving For “C”

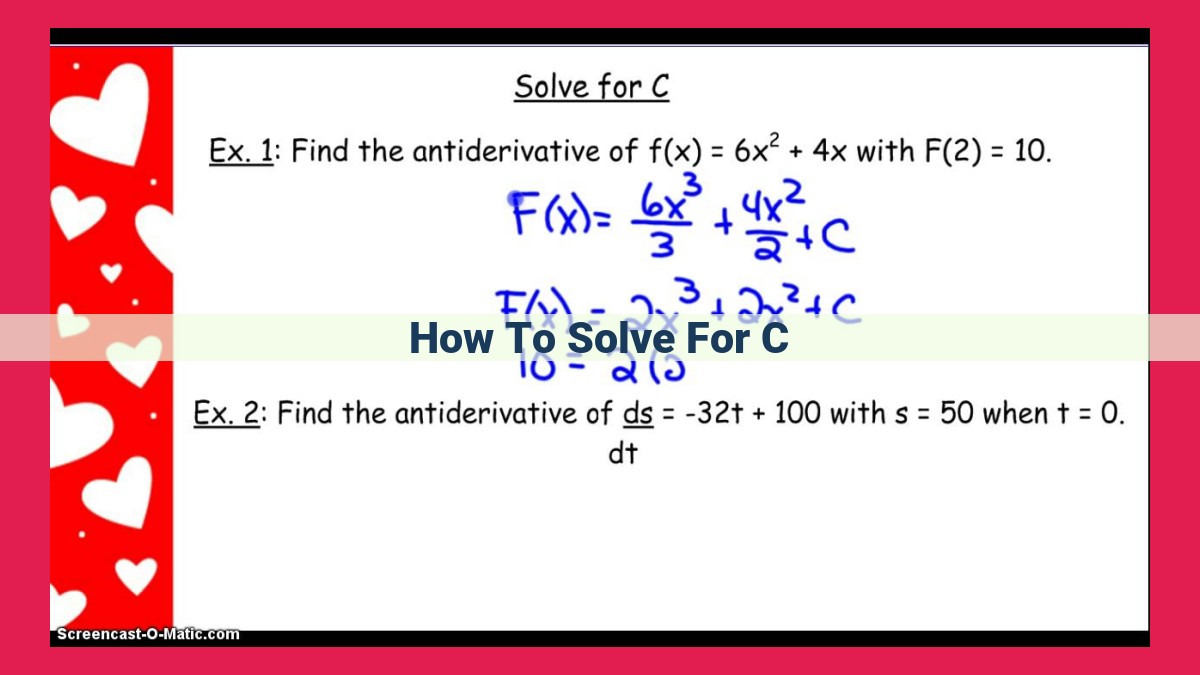
To solve for c, follow these steps: 1) Isolate c on one side of the equation by subtracting or adding constants. 2) If c has a coefficient (number in front), divide both sides by that coefficient. 3) If c’s coefficient is a fraction, multiply both sides by the reciprocal of the fraction. 4) Simplify the resulting equation using algebraic rules. For example, to solve 2c + 5 = 15, subtract 5 from both sides (2c = 10), then divide by 2 (c = 5).

Isolating c: A Step-by-Step Guide for Equation Manipulation

In the realm of mathematics, isolating variables is a fundamental skill that unlocks the ability to solve equations and decipher hidden relationships within them. Let’s embark on a storytelling journey to explore the concept of isolating a variable c and moving it to one side of the equation.

Imagine an equation as a seesaw, with numbers and variables balancing on each side. To isolate c, we need to move it to one side of the seesaw, leaving only constants on the other. This process involves two main strategies:

1. Subtracting/Adding Constants:

Think of subtracting or adding constants as adjusting the weights on the seesaw. If c is on the left side, we subtract c from both sides to neutralize its presence on the left. Conversely, if c is on the right side, we add c to both sides to shift it to the left. This manipulation keeps the equation balanced while isolating c.

2. Multiplying by the Reciprocal:

Here’s where fractions come into play. The reciprocal of a fraction is simply flipping its numerator and denominator. If c has a coefficient (a number multiplying it), we can isolate c by multiplying both sides of the equation by the reciprocal of that coefficient. This effectively divides both sides by the coefficient, canceling it out and leaving c by itself.

By carefully applying these techniques, we can effectively isolate c, leaving us with a simplified equation where c is easily identifiable and ready to be solved. Stay tuned for our next installment, where we’ll delve into the details of dividing by the coefficient and multiplying by the reciprocal to conquer even more complex equations!

Dividing by the Coefficient of c

In the realm of algebra, where equations dance and variables hide their secrets, isolating a variable can be a puzzling quest. But fear not, for we’ve stepped into the spotlight to illuminate the path of isolating the elusive “c.”

What’s a Coefficient?

Think of a coefficient as the sidekick to your variable. It’s the numerical multiplier that sits in front of the variable, like a faithful companion. In the equation 2c – 5 = 11, 2 is the coefficient of c.

Dividing the Equation

Now, to isolate c, we need to separate it from its coefficient. Imagine a seesaw with c on one side and the constant 5 on the other. To balance the seesaw, we divide both sides by 2, the coefficient of c.

Just like balancing a physical seesaw, dividing both sides of the equation by 2 keeps the equality intact. When we do this, we get:

(2c - 5)/2 = 11/2

Voilà! We’ve successfully divided by the coefficient of c, isolating c to one side of the equation.

Simplifying the Equation

Our work isn’t done yet. We need to simplify the equation to make it clear and concise. Let’s combine like terms and get rid of that pesky fraction:

2c - 5 = 22

And there you have it, my friend! We’ve isolated c, revealing its true value.

Remember, isolating a variable is a crucial step in solving equations and understanding the relationships between variables. By dividing by the coefficient, we separate the variable from its sidekick and bring it into the spotlight.

Multiplying by the Reciprocal of c’s Coefficient: Unlocking the Variable’s Isolation

In the realm of equation manipulation, fractions and their reciprocals play a pivotal role in unlocking the secrets hidden within equations. Just as a key fits a lock, the reciprocal of a coefficient can open the door to isolating a variable.

Let’s begin by understanding what a reciprocal is. In mathematics, the reciprocal of a number is simply 1 divided by that number. For instance, the reciprocal of 3 is 1/3.

Now, imagine you have an equation with a variable c that’s multiplying a constant value. To isolate c, we need to divide both sides of the equation by that constant value. However, if the constant is a fraction, we can’t simply divide by it directly.

That’s where the reciprocal comes in. By multiplying both sides of the equation by the reciprocal of the fraction, we effectively “flip” the fraction, turning it into a whole number. This allows us to divide and isolate c.

For instance, let’s say we have the equation:

5/2 * c = 10

To isolate c, we need to multiply both sides by the reciprocal of 5/2, which is 2/5:

(2/5) * (5/2 * c) = (2/5) * 10

This effectively cancels out the fraction 5/2 on the left side, leaving us with:

c = 10 * 2/5

Simplifying further, we get:

c = 4

And there you have it! By multiplying both sides of the equation by the reciprocal of the fraction, we were able to isolate c.

Simplifying the Equation

• Describe the importance of simplifying the resulting equation after isolating c.
• Explain the use of algebraic rules, such as combining like terms and expanding expressions, to simplify the equation.

Isolating c: A Step-by-Step Guide to Solving Linear Equations

In mathematics, isolating a variable is a crucial step in solving equations. Let’s delve into the process of isolating c in a linear equation step by step.

Isolating c: Moving the Variable to One Side

We start by isolating c on one side of the equation. This means getting rid of everything else that’s attached to c. We can do this by:

• Subtracting constants from both sides of the equation to get c by itself on one side.
• Adding constants to both sides of the equation to get c by itself on the other side.

Dividing by the Coefficient of c

A coefficient is the number that multiplies a variable. In the equation ax + b = c, a is the coefficient of x. To isolate c, we need to divide both sides of the equation by the coefficient of c. This gives us:

x = (b - c) / a

Multiplying by the Reciprocal of c’s Coefficient

The reciprocal of a number is the number that, when multiplied by itself, gives you 1. The reciprocal of a is 1/a. We can multiply both sides of the equation by the reciprocal of a to isolate c:

x = (b - c) * 1/a

Simplifying the Equation

Once we’ve isolated c, we can simplify the equation by removing parentheses, combining like terms, and expanding expressions. This makes the equation easier to read and understand.

Example: Solving for c in a Specific Equation

Let’s solve for c in the equation:

3x - 5 = 10

1. Isolate c: Add 5 to both sides.
2. Divide by 3: Divide both sides by 3.
3. Simplify: Remove parentheses and combine like terms.

x = 5

Unveiling the Enigmatic c: A Comprehensive Guide to Solving Linear Equations

In the realm of algebra, solving for a variable is like piecing together a puzzle, with each step bringing us closer to the solution. In this blog, we’ll embark on a captivating journey as we unravel the mysteries of isolating and solving for the elusive variable c.

Isolating c: The Art of Moving the Variable to the Spotlight

Imagine a mischievous c hiding amidst a labyrinth of numbers and symbols. To bring it into the limelight, we must move it to one side of the equation, like a solitary star in the night sky. This can be achieved by subtracting or adding constants on either side, coaxing c to take center stage.

Dividing by the Coefficient: Unleashing the Power of Division

Coefficients, the numbers multiplying c, play a crucial role in its isolation. Just like a commander leading an army, coefficients determine c’s behavior. To isolate c, we must divide both sides of the equation by its coefficient, setting it free from its numerical shackles.

Multiplicative Magic: Reciprocals to the Rescue

Enter the world of fractions, where reciprocals hold the key to further isolating c. The reciprocal of c’s coefficient is like a magic wand, transforming the equation when multiplied on both sides. With this clever trick, c stands alone, unencumbered by any numerical distractions.

Simplifying the Equation: A Path to Clarity

Once c is isolated, the equation demands a touch of algebraic finesse. We invoke algebraic rules to combine like terms and expand expressions, simplifying the equation into a streamlined masterpiece. This final step ensures that c is revealed in its purest form.

Putting Theory into Practice: Solving for c

To fully grasp this concept, let’s conjure up a practical example. Consider the equation 2c + 5 = 15. Our mission is to extract c from this numerical labyrinth.

• Isolating c: We start by subtracting 5 from both sides: 2c = 10.
• Dividing by the coefficient: Next, we divide both sides by 2: c = 5.
• Simplifying the equation: Voila! c is now isolated and unadorned.

Through this step-by-step journey, we have demystified the enigmatic process of solving for c. With practice and a touch of algebraic wizardry, you too can become a master of isolating variables and conquering linear equations.