Discover The Inverse Property: Unraveling Mathematical Operations And Problem-Solving

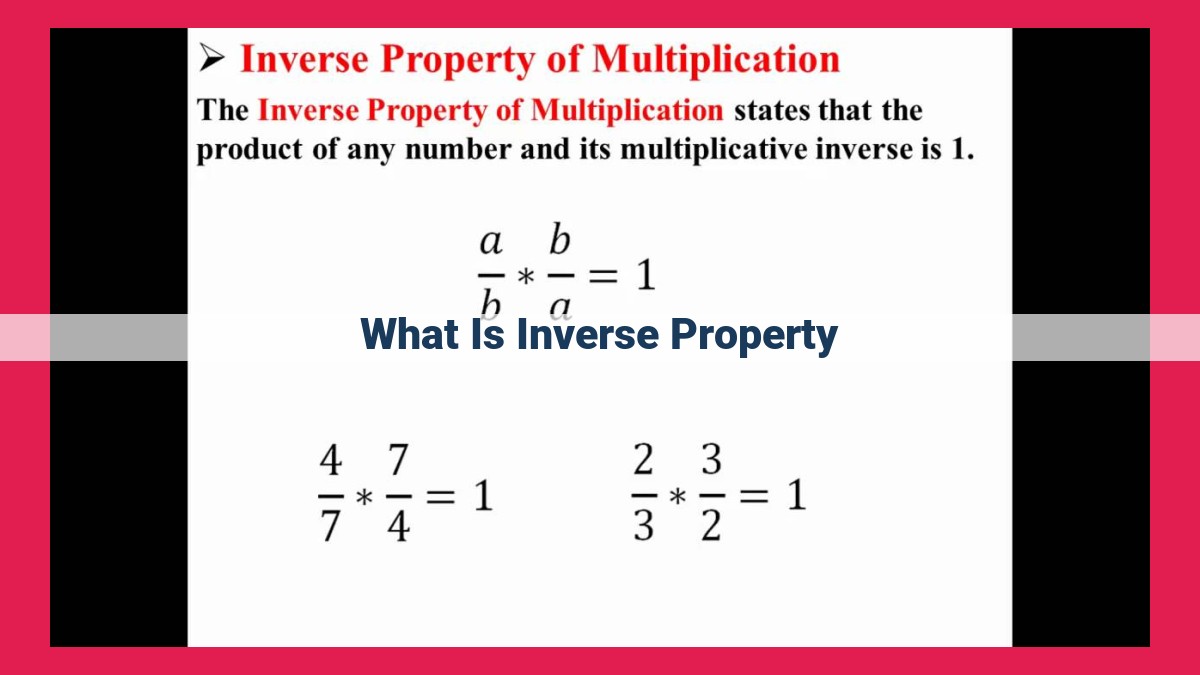
Inverse property in mathematics defines operations that undo the effects of other operations. For instance, subtraction is the inverse of addition, as it reverses the result of adding. Similarly, division undoes multiplication, and finding the square root is the inverse of squaring. Inverse operations play a crucial role in solving equations, simplifying expressions, and enhancing mathematical problem-solving skills.

Inverse Operations: A Mathematical Symphony of Undoing and Solving

In the realm of mathematics, the concept of inverse operations takes center stage as a game-changer in the world of numbers and equations. These magical operations possess the remarkable ability to undo the effects of their counterparts, like a mathematical eraser that wipes away the consequences of previous actions.

Inverse operations are like mathematical twin flames, each born with the express purpose of reversing the impact of the other. They embody the principle of balance, restoring mathematical harmony by canceling out or neutralizing the effects of their predecessors. Their presence in the world of mathematics is akin to a soothing melody, offering simplicity and elegance in a world that can often seem complex and daunting.

These mathematical wonders play a pivotal role in performing calculations and unraveling the mysteries hidden within equations. They provide a systematic approach to undoing mathematical actions, allowing us to retrace our steps and find solutions with remarkable ease. It’s as if mathematics has granted us a superpower, enabling us to reverse time within the realm of numbers and equations.

Undoing Operations: The Power of Inverse

In the realm of mathematics, understanding inverse operations is crucial. Inverse operations are mathematical operations that undo the effects of original operations. They allow us to reverse the actions we have performed, much like an eraser in the world of numbers.

One of the most common examples is that of addition and subtraction. Subtraction is the inverse operation of addition. When we add a number to another, we increase its value. Subtracting that same number effectively undoes the addition, bringing us back to the original value.

Similarly, division is the inverse operation of multiplication. Multiplying a number by another increases its value. Dividing by the same number undoes this multiplication, returning us to the original value.

For instance, if we add 5 to the number 10, we get 15. To undo this addition and return to the original value of 10, we subtract 5 from 15. In other words, 15 – 5 = 10.

Another example is multiplication by 3 and division by 3. If we multiply the number 6 by 3, we get 18. To undo this multiplication and return to the original value of 6, we divide 18 by 3. In other words, 18 ÷ 3 = 6.

Understanding the inverse property not only helps us perform calculations accurately but also plays a pivotal role in solving equations. By applying the inverse operation to both sides of an equation, we can isolate the variable and find its value.

For instance, if we have the equation 5x = 25, to find the value of x, we can undo the multiplication by 5 by dividing both sides of the equation by 5. This gives us (5x) ÷ 5 = 25 ÷ 5, which simplifies to x = 5.

In conclusion, inverse operations are the unsung heroes of mathematics. They possess the remarkable power to undo the effects of original operations, allowing us to reverse our steps and solve problems effectively. Embracing the inverse property not only enhances our mathematical skills but also instills a profound understanding of the interconnectedness of mathematical operations.

Distinguishing Inverse and Opposite Operations: A Tale of Undoing and Contrasting

In the realm of mathematics, operations play a pivotal role in manipulating numbers and solving equations. Among these operations, inverse operations stand out as the unsung heroes, capable of undoing the effects of their counterparts. But how do they differ from opposite operations? Let’s explore this distinction, unraveling the nuances that set them apart.

Inverse Operations: The Undoing Force

Imagine a mathematical journey where you embark on a series of operations. Along the way, you encounter a roadblock that requires you to reverse your steps. Inverse operations come to your aid, providing a way to undo the previous operations and return to your starting point.

For instance, if you added 5 to a number, the inverse operation, subtraction, allows you to undo the addition by subtracting 5. Similarly, if you multiplied a number by 3, division by 3 acts as the inverse operation, reversing the effect of multiplication.

Opposite Operations: Contrasting Values

In contrast to inverse operations, opposite operations do not undo the effects of their counterparts. Instead, they represent values that are diametrically opposed on the number line. Negative numbers, for example, are opposite to their positive counterparts.

Consider the number -5. It is opposite to 5, but it does not undo the addition of 5. Rather, it cancels out the effect of adding 5, resulting in the original number.

A Closer Look: Inverse vs. Opposite

The distinction between inverse and opposite operations can be further clarified through examples.

• Inverse of Addition: Subtraction

• Opposite of Addition: – (negative sign)

• Inverse of Multiplication: Division

• Opposite of Multiplication: 0 (multiplied by anything results in 0)

• Inverse of Squaring: Square Root

• Opposite of Squaring: – (negative square number)

Remember: Inverse operations undo previous operations, while opposite operations represent opposing values.

The Significance of Distinguishing

Understanding the difference between inverse and opposite operations is crucial for navigating mathematical problems with precision. By discerning which type of operation is required, you can effectively simplify expressions, solve equations, and make informed decisions.

Mastering the inverse property empowers you to undo operations, unraveling mathematical puzzles with confidence. Embrace the power of inverse and opposite operations, and embark on a journey where numbers dance to your command!

Examples of Inverse Operations in Action: Unveiling the Power of Mathematical Undoing

When it comes to math, the concept of inverse operations is a game-changer. Inverse operations are like magical wands that reverse the effects of their original counterparts, allowing us to “undo” calculations and solve equations with ease.

Addition and Subtraction:

The most straightforward example of inverse operations is the pair of addition and subtraction. Adding a number increases its value, while subtracting the same number cancels out the effect, bringing us back to the original number. For instance, if we add 5 to 10, we get 15. To undo this addition, we simply subtract 5, returning the value to 10.

Multiplication and Division:

Similar to addition and subtraction, multiplication and division are also inverse operations. Multiplying a number by a non-zero value increases its size, while dividing it by the same value reverses the increase. For example, if we multiply 8 by 3, we get 24. To undo this multiplication, we divide 24 by 3, resulting in 8 once again.

Squaring and Finding the Square Root:

Squaring a number involves multiplying it by itself, dramatically increasing its value. Finding the square root is the inverse operation, which undoes this squaring by determining the number that, when multiplied by itself, gives us the original squared number. For instance, if we square 5, we get 25. Finding the square root of 25 gives us 5, effectively “undoing” the squaring process.

Exponentiation and Taking the Logarithm:

Exponentiation is the process of raising a number to a specified power. Its inverse operation is taking the logarithm, which finds the exponent that, when applied to a base, gives us the original number. For example, if we raise 2 to the power of 3, we get 8. To undo this exponentiation, we take the logarithm with a base of 2, which gives us 3.

These examples showcase the versatility of inverse operations in various mathematical scenarios. Understanding and utilizing them empowers us to tackle complex equations, simplify expressions, and develop a deeper appreciation for the intricacies of mathematics.

Implications for Mathematical Problem Solving

Inverse operations play a pivotal role in the realm of mathematical problem solving. They provide a powerful tool to unravel complex equations and simplify intricate expressions. Let’s explore how inverse operations empower us to conquer mathematical challenges.

Solving Equations with Inverse Operations

In the equation 2x + 5 = 15, the objective is to isolate x, the unknown variable. To do so, we employ inverse operations. Subtraction undoes the addition by 5, yielding 2x = 10. Finally, division by 2 undoes the multiplication by 2, revealing the solution x = 5.

Simplifying Expressions with Inverse Operations

Simplifying complex expressions can be a daunting task. However, inverse operations offer a lifeline. Consider the expression 3(x – 2) + 5. Distributing the 3 results in 3x – 6 + 5. Combining like terms gives us 3x – 1. By factoring out the common factor of x, we obtain x(3 – 1), which simplifies to x(2) or 2x.

Inverse operations stand as the cornerstone of mathematical problem solving. They enable us to solve equations by undoing the effects of operations. Additionally, they empower us to simplify complex expressions, unlocking the path to finding solutions. Their significance cannot be understated.